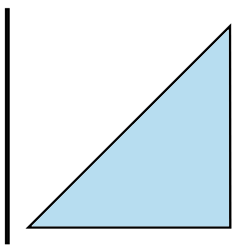
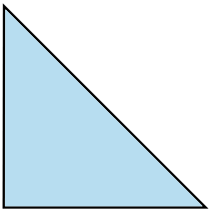
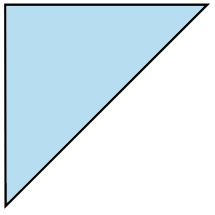
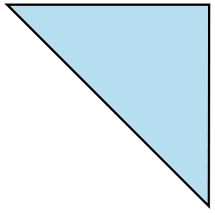
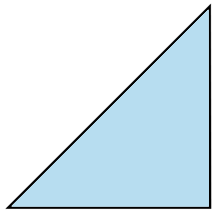
Find the value of
Trigonometry, SMB-060
Find the value of
Trigonometry, SMB-059
Find the value of
Trigonometry, SMB-058
Find the value of
Trigonometry, SMB-057
Find
Trigonometry, SMB-056
Find
Trigonometry, SMB-055
Find the value of
Trigonometry, SMB-054
Find the value of
Trigonometry, SMB-053
Find the value of
Trigonometry, SMB-052
Find the value of
Trigonometry, SMC-051
Solve for
Trigonometry, SMB-050
Solve for
Measurement, STD2 M6 2023 HSC 35
The diagram shows triangle
Calculate the area of the triangle, to the nearest square metre. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
Circles and Hyperbolas, SMB-019
Find the centre and radius of the circle with the equation
Circles and Parabolas, SMB-018
- Find the centre and radius of the circle with the equation
-
(2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Sketch the circle on the graph below. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
a.
b.
Show Worked Solution
| a. | ||
b.
Circles and Hyperbolas, SMB-017
- Find the centre and radius of the circle with the equation
-
(2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Sketch the circle on the graph below. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
a.
b.
Show Worked Solution
| a. | ||
b.
Circles and Hyperbolas, SMB-016
Find the centre and radius of the circle with the equation
Circles and Hyperbola, SMB-015
Find the centre and radius of the circle with the equation
Circles and Hyperbolas, SMB-014
- Find the centre and radius of the circle with the equation
-
(2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Sketch the circle on the graph below. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
a.
b.
Show Worked Solution
| a. | ||
b.
Circles and Hyperbolas, SMB-013
- Write down the equation of the circle with centre
and radius 2. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- On the graph, sketch the circle in part (a). (2 marks)
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
a.
b.
Show Worked Solution
a.
b.
Circles and Hyperbolas, SMB-012
Write down the equation of the circle with centre
Circles and Hyperbolas, SMB-011
Find the centre and radius of the circle with the equation
Circles and Hyperbolas, SMB-010 MC
A circle with centre
The values of
- −3 and 38
- 3 and 12
- −3 and −8
- 3 and 18
Quadratics, SMB-015
The diagram shows the curve with equation
- Find the
-coordinates of points (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Write down the coordinates of
(2 marks)
--- 4 WORK AREA LINES (style=lined) ---
Quadratics, SMB-014
The parabola
Find the
--- 5 WORK AREA LINES (style=lined) ---
Show Worked Solution
| i. |
|
Circles and Hyperbolas, SMB-009
Sketch the graph of
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Circles and Hyperbolas, SMB-008
Sketch the graph of
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Circles and Hyperbolas, SMB-007
Sketch the graph of
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Circles and Hyperbolas, SMB-006
Sketch the graph of
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Circles and Hyperbolas, SMB-004
- List all asymptotes of the graph
. (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
- Hence, sketch the graph of
. (2 marks)
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
i.
ii.
Show Worked Solution
i.
ii.
Circles and Hyperbolas, SMB-003
Sketch the graph of
Clearly mark all asymptotes. (3 marks)
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Circles and Hyperbola, SMB-002
Sketch the graph of
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Circles and Hyperbola, SMB-001
Quadratics, SMB-013
Factorise the parabola described by the equation
--- 6 WORK AREA LINES (style=lined) ---
Quadratics, SMB-012
- Factorise
(1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Find the vertex of the parabola with equation
(2 marks)
--- 4 WORK AREA LINES (style=lined) ---
Quadratics, SMB-011
- Factorise
(1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Find the vertex of the parabola with equation
(2 marks)
--- 4 WORK AREA LINES (style=lined) ---
Quadratics, SMB-010
- Factorise
(2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Find the vertex of the parabola with equation
(2 marks)
--- 5 WORK AREA LINES (style=lined) ---
Quadratics, SMB-009
By completing the square, find the coordinates of the vertex of the parabola with equation
Quadratics, SMB-008
By completing the square, find the coordinates of the vertex of the parabola with equation
Quadratics, SMB-007
By completing the square, find the coordinates of the vertex of the parabola with equation
Exponential, SMB-006 MC
Which graph best represents the equation
| A. |  |
B. |  |
| C. |  |
D. |  |
Exponentials, SMB-004
Sketch the graph of
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Exponential, SMB-003
By completing the table of values, sketch the graph of
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Transformations, SMB-024
Points
Plot the points
--- 0 WORK AREA LINES (style=lined) ---
Show Worked Solution
Transformations, SMB-023
Point
What are the coordinates of
--- 8 WORK AREA LINES (style=lined) ---
Show Worked Solution
Transformations, SMB-022
Point
What are the coordinates of
--- 8 WORK AREA LINES (style=lined) ---
Show Worked Solution
Transformations, SMB-021
Point
What are the coordinates of
--- 8 WORK AREA LINES (style=lined) ---
Show Worked Solution
Transformations, SMB-020
Points
Plot the points
--- 0 WORK AREA LINES (style=lined) ---
Show Answers Only
Show Worked Solution
Transformations, SMB-019
Transformations, SMB-018 MC
Shape 1 is rotated to look like Shape 2.
Which of these could describe the rotation?
- 90° clockwise
- 90° anticlockwise
- 45° clockwise
- 45° anticlockwise
Transformations, SMB-017
How many lines of symmetry does this shape have? (2 marks)
--- 2 WORK AREA LINES (style=lined) ---
Show Worked Solution
Transformations, SMB-016 MC
Which of the shapes below has exactly two lines of symmetry?
| A | B | C | D |
|
|
|
|
|
Show Worked Solution
Transformations, SMB-015
Gabby put 5 points on a grid and labelled them
Point
Gabby adds a sixth point,
How far is point
--- 5 WORK AREA LINES (style=lined) ---
Show Worked Solution
Transformations, SMB-014 MC
This shape is reflected across the line and then rotated 90° clockwise.
Which image shows the appearance of the shape after these transformations?
| A | B |
 |
 |
| C | D |
 |
 |
Show Answers Only
Show Worked Solution
Transformations, SMB-013
The trapezium
Which of these transformations resulted in the new position?
- Rotate
180° clockwise about the origin. - Rotate
270° clockwise about the origin. - Reflect
across the -axis, then translate 8 units left. - Reflect
across the -axis, then translate 7 units down.
Show Worked Solution
Transformations, SMB-012 MC
Rochelle drew a pattern which is pictured below.
Rochelle rotates the pattern.
How much does Rochelle to turn the pattern until it looks exactly the same?
Transformations, SMB-011 MC
Mohammed drew a shape with four lines of symmetry.
Which of these could be Mohammed's shape?
| A. | B. | C. | D. |
 |
 |
 |
 |
Show Worked Solution
Transformations, SMB-010
This shape will be translated 4 units to the right and 2 units up.
Where will the image of the point
--- 4 WORK AREA LINES (style=lined) ---
Transformations, SMB-009
The point
Find the coordinates of
Transformations, SMB-008
The point
Find the coordinates of