The region enclosed by the semicircle
The two pieces are rotated about the
Show that
--- 8 WORK AREA LINES (style=lined) ---
Aussie Maths & Science Teachers: Save your time with SmarterEd
The region enclosed by the semicircle
The two pieces are rotated about the
Show that
--- 8 WORK AREA LINES (style=lined) ---
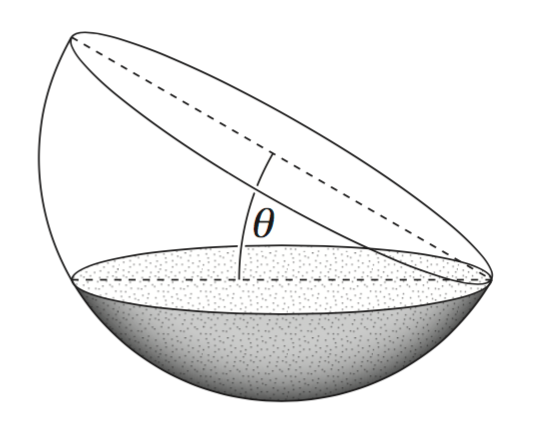
(i)
The diagram shows two curves
An egg is modelled by rotating the curves about the
Find the exact value of the volume of the solid of revolution. (4 marks)
--- 10 WORK AREA LINES (style=lined) ---
The circle
--- 8 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
i.
ii. (1)
| ii. (2) | ||