SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Calculus, 2ADV C3 2018 HSC 16a

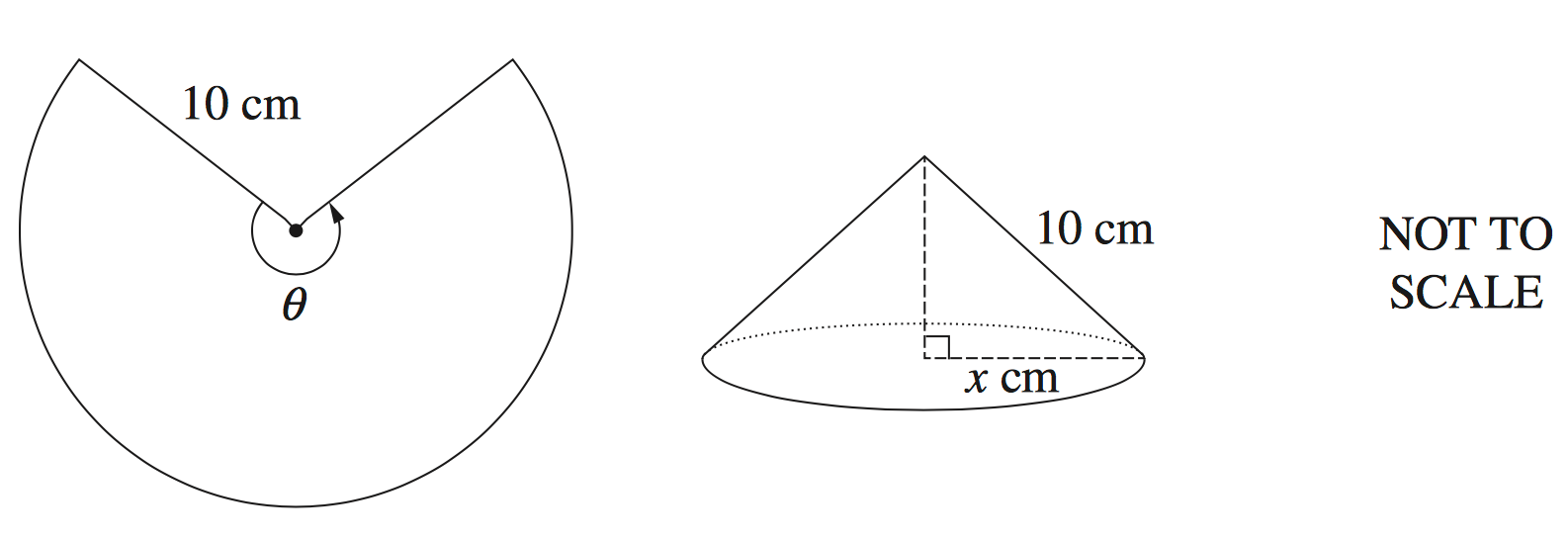
A sector with radius 10 cm and angle    is used to form the curved surface of a cone with base radius cm, as shown in the diagram.
 


 

The volume of a cone of radius and height is given by  .

  1. Show that the volume, cm³, of the cone described above is given by
     
          .   (1 mark)

  2. Show that  .   (2 marks)

  3. Find the exact value of    for which is a maximum.   (3 marks)

Show Answers Only
Show Worked Solution

i.   


 

♦ Mean mark (ii) 45%.

ii.  
 
   
   
   

 

iii. 

♦♦ Mean mark (iii) 23%.

 


 

 

Calculus, 2ADV C3 2015 HSC 16c

The diagram shows a cylinder of radius and height inscribed in a cone of radius and height , where and are constants.
  

The volume of a cone of radius and height is  

The volume of a cylinder of radius and height is  

  1. Show that the volume, , of the cylinder can be written as
     
            (3 marks)

  2. By considering the inscribed cylinder of maximum volume, show that the volume of any inscribed cylinder does not exceed of the volume of the cone.   (4 marks)

Show Answers Only
Show Worked Solution

i.   
 

♦♦ Mean mark (i) 16%.

 

 
 

 


 

♦♦ Mean mark (ii) 21%.

ii.   

 
 

 

 


 

 

 

 

 

Calculus, 2ADV C3 2006 HSC 9c

A cone is inscribed in a sphere of radius , centred at . The height of the cone is and the radius of the base is , as shown in the diagram.

  1. Show that the volume, , of the cone is given by
     
         .  (2 marks)

  2. Find the value of for which the volume of the cone is a maximum. You must give reasons why your value of gives the maximum volume.  (3 marks)

Show Answers Only
Show Worked Solution

i. 

 
 
 

 

ii.