Non Right-Angled Trig

Trigonometry, SMB-067

Find the value of , correct to the nearest minute. (3 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-066

Find the value of , correct to the nearest degree. (2 marks)

--- 5 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

Trigonometry, SMB-065

Find the value of , correct to 1 decimal place. (2 marks)

--- 5 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

Trigonometry, SMB-064

Find the value of , correct to 2 decimal places. (3 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-063

Find the value of , correct to 1 decimal place. (3 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-062

Find the value of , correct to 1 decimal place. (3 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-061

Find the value of , correct to the nearest degree. (3 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-060

Find the value of , correct to the nearest degree. (2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-059

Find the value of , correct to the nearest degree. (2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-058

Find the value of , correct to the nearest degree. (2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-057

Find , to the nearest degree, such that

(2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-056

Find , to the nearest degree, such that

(2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-055

Find the value of , correct to 1 decimal place. (2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-054

Find the value of , correct to 1 decimal place. (2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-053

Find the value of , correct to 1 decimal place. (2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-052

Find the value of , correct to 1 decimal place. (2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMC-051

Solve for , giving your answer correct to 1 decimal place.

(2 marks)

Show Answers Only

Show Worked Solution

Trigonometry, SMB-050

Solve for , giving your answer correct to 1 decimal place.

(2 marks)

Show Answers Only

Show Worked Solution

Measurement, STD2 M6 2023 HSC 35

The diagram shows triangle .

Calculate the area of the triangle, to the nearest square metre. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

Measurement, STD2 M6 2022 HSC 26

The diagram shows two right-angled triangles, and ,

where and .

Calculate the size of angle , to the nearest minute. (4 marks)

--- 8 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

♦ Mean mark 50%.

Measurement, STD2 M6 2021 HSC 32

A right-angled triangle is cut out from a semicircle with centre . The length of the diameter is 16 cm and = 30°, as shown on the diagram.

Find the length of in centimetres, correct to two decimal places. (2 marks)

--- 4 WORK AREA LINES (style=lined) ---
Hence, find the area of the shaded region in square centimetres, correct to one decimal place. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

a.

b.

♦ Mean mark part (b) 36%.

Measurement, STD2 M6 2018 HSC 30c

The diagram shows two triangles.

Triangle is right-angled, with and .

In triangle and . The area of triangle is 30 cm².

What is the value of , correct to one decimal place? (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

♦ Mean mark 39%.

Measurement, STD2 M6 2015 HSC 22 MC

The area of the triangle shown is 250 cm².

What is the value of , correct to the nearest whole number?

Show Answers Only

Show Worked Solution

♦ Mean mark 42%.

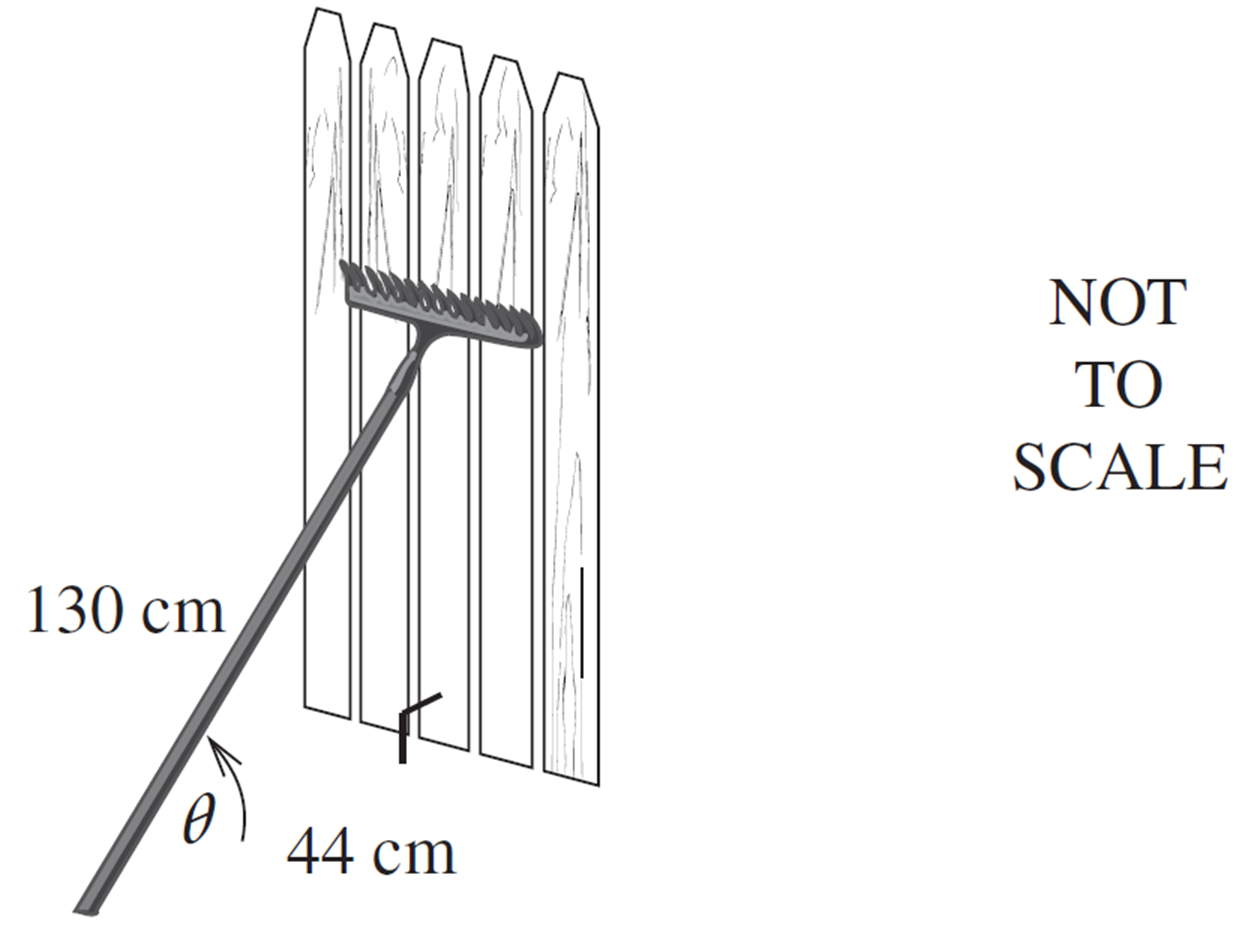
Measurement, STD2 M6 2006 HSC 24b

A 130 cm long garden rake leans against a fence. The end of the rake is 44 cm from the base of the fence.

If the fence is vertical, find the value of to the nearest degree. (2 marks)

--- 4 WORK AREA LINES (style=lined) ---
The fence develops a lean and the rake is now at an angle of 53° to the ground. Calculate the new distance ( cm) from the base of the fence to the head of the rake. Give your answer to the nearest centimetre. (2 marks)

--- 4 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

ii.

Measurement, STD2 M6 2006 HSC 9 MC

What is the area of this triangle, to the nearest square metre?

$²$
$²$
$²$
$²$

Show Answers Only

Show Worked Solution



	$²$

Measurement, STD2 M6 2005 HSC 5 MC

Which formula should be used to calculate the distance between Toby and Frankie?

Show Answers Only

Show Worked Solution

Measurement, STD2 M6 2004 HSC 9 MC

What is the area of the triangle to the nearest square metre?

$²$
$²$
$²$
$²$

Show Answers Only

Show Worked Solution



	$²$

Measurement, STD2 M6 2008 HSC 5 MC

What is the size of the smallest angle in this triangle?

Show Answers Only

Show Worked Solution

Measurement, STD2 M6 2010 HSC 26d

Find the area of triangle , correct to the nearest square metre. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

$²$ $²$

Show Worked Solution

♦♦ Mean mark 32%.
TIP: The allocation of 3 marks to this question should flag the need for more than 1 step.



	$² ²$

Measurement, STD2 M6 2010 HSC 9 MC

Three towns , and are marked on the diagram.

The distance from to is 76 km. and

What is the distance from to to the nearest kilometre?

Show Answers Only

Show Worked Solution

Measurement, STD2 M6 2012 HSC 10 MC

What is the area of this triangle, to the nearest square metre?

$²$
$²$
$²$
$²$

Show Answers Only

Show Worked Solution



	$²$