smc-1039-60-x-axis Rotation

Calculus, EXT1 C3 2024 HSC 12b

The region, , is bounded by the function, , the -axis and the lines and .

What is the volume of the solid of revolution obtained when the region is rotated about the -axis? (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

Calculus, EXT1 C3 2022 SPEC1 10

Let .

Sketch the graph of for on the set of axes below. Label any asymptotes with their equations and label any turning points and the endpoints with their coordinates.   (3 marks)
The graph of for is rotated about the -axis to form a solid of revolution.
Find the volume of this solid. Give your answer in the form , where , , . (3 marks)

Show Answers Only

Show Worked Solution

♦ Mean mark (a) 49%.

b.

♦ Mean mark (b) 55%.

Calculus, EXT1 C3 2022 HSC 13b

A solid of revolution is to be found by rotating the region bounded by the -axis and the curve , where , between and about the -axis.

Find the value of for which the volume is . (3 marks)

--- 8 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

Calculus, EXT1 C3 2021 SPEC1 4

The shaded region in the diagram below is bounded by the graph of and the -axis between the first two non-negative -intercepts of the curve, that is interval . The shaded region is rotated about the -axis to form a solid of revolution.

Find the volume, of the solid formed. (3 marks)

Show Answers Only

$³$

Show Worked Solution






		$³$

Calculus, EXT1 C3 2020 HSC 13b

The region is bounded by the -axis, the graph of and the graph of , as shown in the diagram.

Find the volume of the solid of revolution formed when the region is rotated about the -axis. (4 marks)

--- 8 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution

Mean mark 53%.








	$³$

Calculus, EXT1 C3 SM-Bank 3

Find the volume of the solid of revolution formed when the graph of is rotated about the -axis over the interval . (3 marks)

Show Answers Only

$³$

Show Worked Solution






	$³$

Calculus, EXT1* C3 2019 HSC 13d

The diagram shows the region bounded by the curve , and the -axis between and . The region is rotated about the -axis to form a solid.

Find the exact value of the volume of the solid formed. (3 marks)

Show Answers Only

$³$

Show Worked Solution





	$³$

Calculus, EXT1 C3 SM-Bank 1

The region enclosed by the semicircle and the -axis is to be divided into two pieces by the line , when .

The two pieces are rotated about the -axis to form solids of revolution. The value of is chosen so that the volumes of the solids are in the ratio .

Show that satisfies the equation . (3 marks)

--- 8 WORK AREA LINES (style=lined) ---

Show Answers Only

Show Worked Solution

(i)

Calculus, EXT1* C3 2017 HSC 12b

The diagram shows the region bounded by and the -axis.

The region is rotated about the -axis to form a solid.

Find the exact volume of the solid formed. (3 marks)

Show Answers Only

$³$

Show Worked Solution







	$³$

Calculus, EXT1* C3 2016 HSC 15a

The diagram shows two curves and The curve is the semicircle The curve has equation

An egg is modelled by rotating the curves about the -axis to form a solid of revolution.

Find the exact value of the volume of the solid of revolution. (4 marks)

--- 10 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution





	$³$




	$³$



	$³$

Calculus, EXT1* C3 2004 HSC 4c

In the diagram, the shaded region is bounded by the curve , the coordinate axes and the line . The shaded region is rotated about the -axis.

Calculate the exact volume of the solid of revolution formed. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution







	$³$

Calculus, EXT1* C3 2007 HSC 3a

Find the volume of the solid of revolution formed when the region bounded by the curve , the -axis, the -axis and the line , is rotated about the -axis. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution







	$³$

Calculus, EXT1 C3 2005 HSC 5a

Find the exact value of the volume of the solid of revolution formed when the region bounded by the curve , the -axis and the line is rotated about the -axis. (3 marks)

--- 8 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution

COMMENT: Michael Wells (1st in state Ext2) would derive this formula in his working from the

identity in ~5 seconds every time he used it in an exam.








	$³$

Calculus, EXT1* C3 2007 HSC 9a

The shaded region in the diagram is bounded by the curve , the -axis, and the lines and

Find the volume of the solid of revolution formed when the shaded region is rotated about the -axis. (3 marks)

Show Answers Only

$³$

Show Worked Solution







	$³$

Calculus, EXT1* C3 2008 HSC 6c

The graph of is shown below.

The shaded region in the diagram is bounded by the curve , the -axis and the lines and .

Find the volume of the solid of revolution formed when the shaded region is rotated about the -axis. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution








	$³$

Calculus, EXT1 C3 2014 HSC 12b

The region bounded by and the -axis, between and , is rotated about the -axis to form a solid.

Find the volume of the solid. (3 marks)

Show Answers Only

$³$

Show Worked Solution

COMMENT: The identities

are tested every year – know them.






	$³$

Calculus, EXT1* C3 2014 HSC 14c

The region bounded by the curve and the -axis between and is rotated about the -axis to form a solid.

Find the volume of the solid. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution







	$³$

Calculus, EXT1 C3 2013 HSC 12b

The region bounded by the graph and the -axis between and is rotated about the -axis to form a solid.

Find the exact volume of the solid. (3 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution





	$³$

Calculus, EXT1* C3 2009 HSC 6a

The diagram shows the region bounded by the curve , the lines and , and the -axis.

The region is rotated about the -axis. Find the volume of the solid of revolution formed. (3 marks)

Show Answers Only

$³$

Show Worked Solution






	$³$

Calculus, EXT1* C3 2010 HSC 10b

The circle has radius and centre . The circle meets the positive -axis at . The point is on the interval . A vertical line through meets the circle at . Let .

The shaded region bounded by the arc and the intervals and is rotated about the -axis. Show that the volume, , formed is given by
(3 marks)

--- 8 WORK AREA LINES (style=lined) ---
A container is in the shape of a hemisphere of radius metres. The container is initially horizontal and full of water. The container is then tilted at an angle of to the horizontal so that some water spills out.

(1) Find so that the depth of water remaining is one half of the original depth. (1 mark)

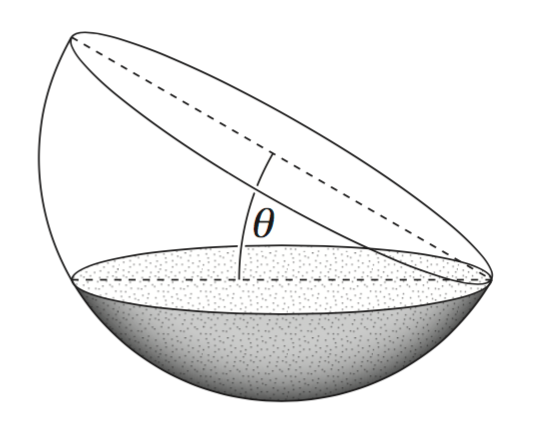
--- 4 WORK AREA LINES (style=lined) ---
(2) What fraction of the original volume is left in the container? (2 marks)

--- 6 WORK AREA LINES (style=lined) ---

Show Answers Only

(1)
(2)

Show Worked Solution

♦♦♦ Mean mark (i) 16%.
MARKER’S COMMENT: A common error was to integrate

instead of

(note that

is a constant).

ii. (1)

♦♦♦ Part (ii) mean marks 3% and 2% for (ii)(1) and (ii)(2) respectively.

MARKER’S COMMENT: Previous parts of a question should always be at the front and centre of a student’s mind and direct their strategy.

ii. (2)

Calculus, EXT1* C3 2012 HSC 14b

The diagram shows the region bounded by , the -axis, the -axis, and the line .

The region is rotated about the -axis to form a solid.

Find the volume of the solid. (3 marks)

--- 8 WORK AREA LINES (style=lined) ---

Show Answers Only

$³$

Show Worked Solution

MARKER’S COMMENT: Most students omitted the

when stating the definite integral in this question!









	$³$

$³$ .