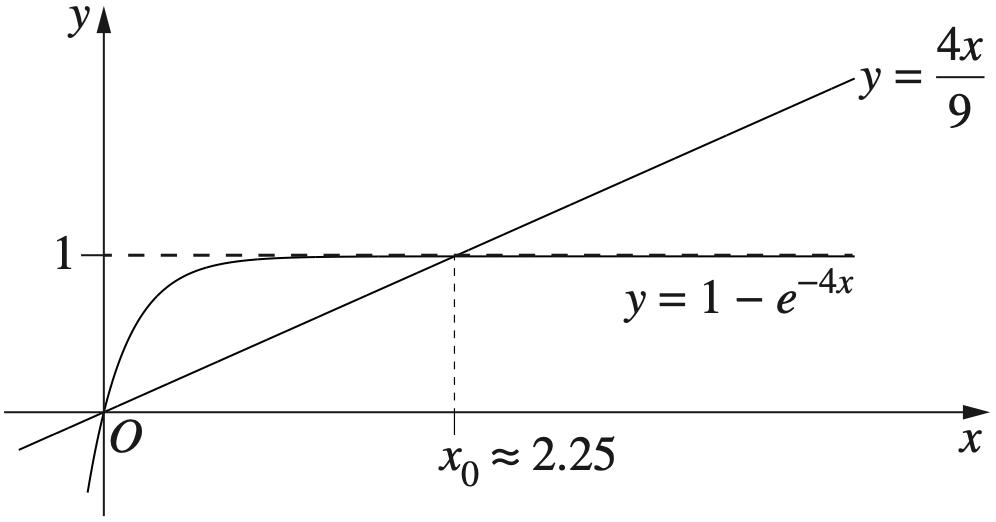
Two particles, \(A\) and \(B\), each have mass 1 kg and are in a medium that exerts a resistance to motion equal to \(k v\), where \(k>0\) and \(v\) is the velocity of any particle. Both particles maintain vertical trajectories. The acceleration due to gravity is \(g\) ms\(^{-2}\), where \(g>0\). The two particles are simultaneously projected towards each other with the same speed, \(v_0\) ms\(^{-1}\), where \(0<v_0<\dfrac{g}{k}\). The particle \(A\) is initially \(d\) metres directly above particle \(B\), where \(d<\dfrac{2 v_0}{k}\). Find the time taken for the particles to meet. (4 marks) --- 16 WORK AREA LINES (style=lined) ---
\(\text{Particle A:}\) \(\ddot{x}=\dfrac{dv_{\small{A}}}{dt}=-g-k v_{\small{A}}\) \(\dfrac{dt}{dv_{\small{A}}}=\dfrac{1}{-g-kv_{\small{A}}}\) \(t=-\displaystyle \int \dfrac{1}{g+k v_{\small{A}}} \, dv_{\small{A}}=-\dfrac{1}{k} \ln \left(g+k v_A\right)+c\) \(\text{At} \ \ t=0, \ v_{\small{A}}=-v_0 \ \Rightarrow \ c=\dfrac{1}{k} \ln \left(g-k v_0\right)\) \(t=\dfrac{1}{k} \ln \left(g-k v_0\right)-\dfrac{1}{k} \ln \left(g+kv_{\small{A}}\right)=\dfrac{1}{k} \ln \left(\dfrac{g-kv_0}{g+kv_{\small{A}}}\right)\) \(\text{Find} \ v_{\small{A}}:\) \(x_{\small{A}}=d-\left(\dfrac{g}{k^2}-\dfrac{v_0}{k}\right) e^{-kt}-gt+\dfrac{g}{k^2}-\dfrac{v_0}{k}\) \(\text{Particle B:}\) \(\ddot{x}=-g-k v_{\small{B}}\) \(t=-\dfrac{1}{k} \ln \left(g+k v_{\small{B}}\right)+c\) \(\text{When} \ \ t=0, v_B=v_0 \ \Rightarrow \ c=\dfrac{1}{k} \ln \left(g+kv_0\right)\) \(\text{When} \ \ t=0, x=0 \ \Rightarrow \ c=\dfrac{g}{k^2}+\dfrac{v_0}{k}\) \(x_{\small{B}}=-\left(\dfrac{g}{k^2}+\dfrac{v_0}{k}\right) e^{-kt}-gt+\dfrac{g}{k^2}+\dfrac{v_0}{k}\) \(\text{Find \(t\) when \(\ x_{\small{A}}=x_{\small{B}}\):}\) \(d-\left(\dfrac{g}{k^2}-\dfrac{v_0}{k}\right) e^{-k t}-g t+\dfrac{g}{k^2}-\dfrac{v_0}{k}=-\left(\dfrac{g}{k^2}+\dfrac{v_0}{k}\right) e^{-k t}-g t+\dfrac{g}{k^2}+\dfrac{v_0}{k}\)
\(\dfrac{g-k v_0}{g+k v_{\small{A}}}\)
\(=e^{kt}\)
\(g-kv_0\)
\(=e^{kt} \cdot g+e^{kt} \cdot kv_{\small{A}}\)
\(kv_{\small{A}}\)
\(=e^{-kt}\left(g-kv_0\right)-g\)
\(v_{\small{A}}\)
\(=\dfrac{1}{k}\left[e^{-kt}\left(g-kv_0\right)-g\right]\)
\(x\)
\(=\displaystyle \frac{1}{k} \int e^{-kt} \cdot g-e^{-kt} \cdot v_0-g \, dt\)
\(=\dfrac{1}{k}\left[-\dfrac{g}{k}e^{-kt}+v_0 e^{-kt}-gt\right]+c\)
\(=-\left(\dfrac{g}{k^2}-\dfrac{v_0}{k}\right) e^{-kt}-gt+c\)
\(\text{When} \ \ t=0, x=0 \ \Rightarrow \ c=\dfrac{g}{k^2}-\dfrac{v_0}{k}\)
\(v_{\small{B}}\)
\(=\dfrac{1}{k}\left[e^{-kt}\left(g+kv_0\right)-g\right]\)
\(x_{\small{B}}\)
\(=-\left(\dfrac{g}{k^2}+\dfrac{v_0}{k}\right) e^{-kt}-gt+c\)
\(\dfrac{2 v_0}{k} \cdot e^{-k t}\)
\(=\dfrac{2 v_0}{k}-d\)
\(e^{-kt}\)
\(=\left(\dfrac{2 v_0-d k}{k}\right) \cdot \dfrac{k}{v_0}\)
\(-kt\)
\(=\ln \left(\dfrac{2 v_0-d k}{v_0}\right)\)
\(t\)
\(=-\dfrac{1}{k} \ln \left(\dfrac{2v_0-dk}{v_0}\right)\)
Mechanics, EXT2 M1 2023 HSC 13c
A particle of mass 1 kg is projected from the origin with speed 40 m s\( ^{-1}\) at an angle 30° to the horizontal plane. --- 6 WORK AREA LINES (style=lined) --- The forces acting on the particle are gravity and air resistance. The air resistance is proportional to the velocity vector with a constant of proportionality 4 . Let the acceleration due to gravity be 10 m s \( ^{-2}\). The position vector of the particle, at time \(t\) seconds after the particle is projected, is \(\mathbf{r}(t)\) and the velocity vector is \(\mathbf{v}(t)\). --- 12 WORK AREA LINES (style=lined) --- --- 10 WORK AREA LINES (style=lined) --- --- 7 WORK AREA LINES (style=lined) ---
i. \(\underset{\sim}{v}(0)={\displaystyle\left(\begin{array}{cc} 40 \cos\ 30° \\ 40 \sin\ 30°\end{array}\right)} = {\displaystyle\left(\begin{array}{cc} 40 \times \frac{\sqrt3}{2} \\ 40 \times \frac{1}{2}\end{array}\right)} = {\displaystyle\left(\begin{array}{cc}20 \sqrt{3} \\ 20\end{array}\right)} \) ii. \(\text{Air resistance:} \) \(\underset{\sim}{F} = -4\underset{\sim}{v} = {\displaystyle\left(\begin{array}{cc} -4\dot{x} \\ -4\dot{y} \end{array}\right)} \) \(\text{Horizontally:}\) iv. \(\text{Range}\ \Rightarrow\ \text{Find}\ \ t\ \ \text{when}\ \ y=0: \) \(\Rightarrow \text{Solution when}\ \ t\approx 2.25\)
Show Worked Solution
\(1 \times \ddot{x} \)
\(=-4 \dot{x} \)
\(\dfrac{d\dot{x}}{dt}\)
\(=-4\dot{x}\)
\(\dfrac{dt}{d\dot{x}}\)
\(= -\dfrac{1}{4\dot{x}} \)
\(t\)
\(=-\dfrac{1}{4} \displaystyle \int \dfrac{1}{\dot{x}} \ d\dot{x} \)
\(-4t\)
\(=\ln |\dot{x}|+c \)
\(\text{When}\ \ t=0, \ \dot{x}=20\sqrt3 \ \ \Rightarrow\ \ c=-\ln{20\sqrt3} \)
\(-4t\)
\(=\ln|\dot{x}|-\ln 20\sqrt3 \)
\(-4t\)
\(=\ln\Bigg{|}\dfrac{\dot{x}}{20\sqrt{3}} \Bigg{|} \)
\(\dfrac{\dot{x}}{20\sqrt{3}} \)
\(=e^{-4t} \)
\(\dot{x}\)
\(=20\sqrt{3}e^{-4t}\)
\(\text{Vertically:} \)
\(1 \times \ddot{y} \)
\(=-1 \times 10-4 \dot{y} \)
\(\dfrac{d\dot{y}}{dt}\)
\(=-(10+4\dot{y})\)
\(\dfrac{dt}{d\dot{y}}\)
\(= -\dfrac{1}{10+4\dot{y}} \)
\(t\)
\(=- \displaystyle \int \dfrac{1}{10+4\dot{y}} \ d\dot{y} \)
\(-4t\)
\(=- \displaystyle \int \dfrac{4}{10+4\dot{y}} \ d\dot{y} \)
\(-4t\)
\(=\ln |10+4\dot{y}|+c \)
\(\text{When}\ \ t=0, \ \dot{y}=20 \ \ \Rightarrow\ \ c=-\ln{90} \)
\(-4t\)
\(=\ln|10+4\dot{y}|-\ln 90 \)
\(-4t\)
\(=\ln\Bigg{|}\dfrac{10+4\dot{y}}{\ln{90}} \Bigg{|} \)
\(\dfrac{10+4\dot{y}}{90} \)
\(=e^{-4t} \)
\(4\dot{y}\)
\(=90e^{-4t}-10\)
\(\dot{y}\)
\(=\dfrac{45}{2} e^{-4t}-\dfrac{5}{2} \)
\(\therefore \underset{\sim}v={\displaystyle \left(\begin{array}{cc}20 \sqrt{3} e^{-4 t} \\ \dfrac{45}{2} e^{-4 t}-\dfrac{5}{2}\end{array}\right)}\)
iii. \(\text{Horizontally:}\)
\(x\)
\(= \displaystyle \int \dot{x}\ dx\)
\(= \displaystyle \int 20\sqrt3 e^{-4t}\ dt \)
\(=-5\sqrt3 e^{-4t}+c \)
\(\text{When}\ \ t=0, \ x=0\ \ \Rightarrow\ \ c=5\sqrt3 \)
\(x\)
\(=5\sqrt3-5\sqrt3 e^{-4t} \)
\(=5\sqrt3(1-e^{-4t}) \)
\(\text{Vertically:}\)
\(y\)
\(= \displaystyle \int \dot{y}\ dx\)
\(= \displaystyle \int \dfrac{45}{2} e^{-4t}-\dfrac{5}{2}\ dt \)
\(=-\dfrac{45}{8}e^{-4t}-\dfrac{5}{2}t+c \)
\(\text{When}\ \ t=0, \ y=0\ \ \Rightarrow\ \ c= \dfrac{45}{8} \)
\(y\)
\(=\dfrac{45}{8}-\dfrac{45}{8} e^{-4t}-\dfrac{5}{2}t \)
\(=\dfrac{45}{8}(1-e^{-4t})-\dfrac{5}{2} \)
\(\therefore \underset{\sim}{r}=\left(\begin{array}{c}5 \sqrt{3}\left(1-e^{-4 t}\right) \\ \dfrac{45}{8}\left(1-e^{-4 t}\right)-\dfrac{5}{2} t\end{array}\right)\)
\(\dfrac{45}{8}(1-e^{-4t})-\dfrac{5}{2}t \)
\(=0\)
\(\dfrac{45}{8}(1-e^{-4t}) \)
\(=\dfrac{5}{2}t \)
\(1-e^{-4t}\)
\(=\dfrac{4}{9}t \)
\(\text{Graph shows intersection of these two graphs.}\)
\(\therefore\ \text{Range}\)
\(=5\sqrt3(1-e^{(-4 \times 2.25)}) \)
\(=8.659…\)
\(=8.7\ \text{metres (to 1 d.p.)}\)
♦ Mean mark (iv) 43%.
Mechanics, EXT2 M1 2022 HSC 10 MC
A particle is moving vertically in a resistive medium under the influence of gravity. The resistive force is proportional to the velocity of the particle.
The initial speed of the particle is NOT zero.
Which of the following statements about the motion of the particle is always true?
- If the particle is initially moving downwards, then its speed will increase.
- If the particle is initially moving downwards, then its speed will decrease.
- If the particle is initially moving upwards, then its speed will eventually approach a terminal speed.
- If the particle is initially moving upwards, then its speed will not eventually approach a terminal speed.
Mechanics, EXT2 M1 2022 HSC 16b
A projectile of mass `M` kg is launched vertically upwards from a horizontal plane with initial speed `v_0\ text{m s}^(-1)` which is less than 100`\ text{m s}^(-1)`. The projectile experiences a resistive force which has magnitude `0.1 M v` newtons, where `v\ text{m s}^(-1)` is the speed of the projectile. The acceleration due to gravity is 10`\ text{m s}^(-2)`.
The projectile lands on the horizontal plane 7 seconds after launch.
Find the value of `v_0`, correct to 1 decimal place. (4 marks)
Mechanics, EXT2 M1 2019 HSC 14b
A parachutist jumps from a plane, falls freely for a short time and then opens the parachute. Let t be the time in seconds after the parachute opens, `x(t)` be the distance in metres travelled after the parachute opens, and `v(t)` be the velocity of the parachutist in `text(ms)^(-1)`.
The acceleration of the parachutist after the parachute opens is given by
`ddot x = g - kv,`
where `g\ text(ms)^(-2)` is the acceleration due to gravity and `k` is a positive constant.
- With an open parachute the parachutist has a terminal velocity of `w\ text(ms)^(-1)`.
Show that `w = g/k`. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
At the time the parachute opens, the speed of descent is `1.6 w\ text(ms)^(-1)`.
- Show that it takes `1/k log_e 6` seconds to slow down to a speed of `1.1w\ text(ms)^(-1)`. (4 marks)
--- 10 WORK AREA LINES (style=lined) ---
- Let `D` be the distance the parachutist travels between opening the parachute and reaching the speed `1.1w\ text(ms)^(-1)`.
Show that `D = g/k^2 (1/2 + log_e 6)`. (3 marks)
--- 8 WORK AREA LINES (style=lined) ---
Mechanics, EXT2 M1 2009 HSC 7a
A bungee jumper of height 2 m falls from a bridge which is 125 m above the surface of the water, as shown in the diagram. The jumper’s feet are tied to an elastic cord of length `L` m. The displacement of the jumper’s feet, measured downwards from the bridge, is `x` m.
The jumper’s fall can be examined in two stages. In the first stage of the fall, where `0 <= x <= L`, the jumper falls a distance of `L` m subject to air resistance, and the cord does not provide resistance to the motion. In the second stage of the fall, where `x > L`, the cord stretches and provides additional resistance to the downward motion.
- The equation of motion for the jumper in the first stage of the fall is
`ddot x = g - rv`
where `g` is the acceleration due to gravity, `r` is a positive constant, and `v` is the velocity of the jumper.
(1) Given that `x = 0` and `v = 0` initially, show that
`qquad x = g/r^2 ln (g/(g - rv)) - v/r.` (3 marks)
--- 8 WORK AREA LINES (style=lined) ---
(2) Given that `g = 9.8\ text(ms)^-2` and `r = 0.2\ text(s)^-1`, find the length, `L`, of the cord such that the jumper’s velocity is `30\ text(ms)^-1` when `x = L`. Give your answer to two significant figures. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- In the second stage of the fall, where `x > L`, the displacement `x` is given by
`x = e^(-t/10)(29 sin t - 10 cos t) + 92`
where `t` is the time in seconds after the jumper’s feet pass `x = L`.
Determine whether or not the jumper’s head stays out of the water. (4 marks)
--- 8 WORK AREA LINES (style=lined) ---