smc-2830-50-SP problems

Calculus, MET1 2024 VCAA 7

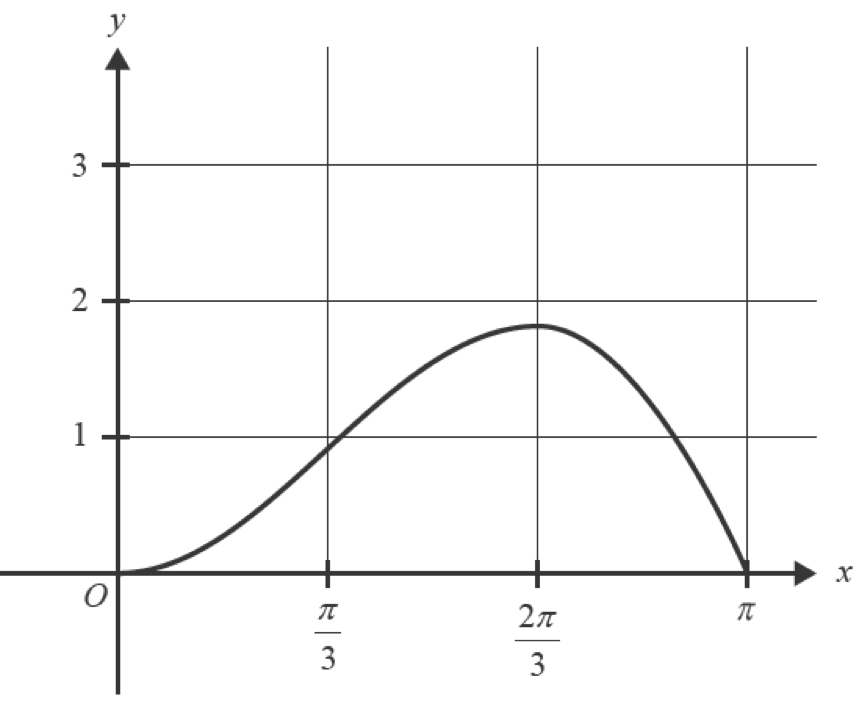
Part of the graph of \(f:[-\pi, \pi] \rightarrow R, f(x)=x \sin (x)\) is shown below.

Use the trapezium rule with a step size of \(\frac{\pi}{3}\) to determine an approximation of the total area between the graph of \(y=f(x)\) and the \(x\)-axis over the interval \(x \in[0, \pi]\). (3 marks)

--- 6 WORK AREA LINES (style=lined) ---
i. Find \(f^{\prime}(x)\). (1 mark)

--- 3 WORK AREA LINES (style=lined) ---
ii. Determine the range of \(f^{\prime}(x)\) over the interval \(\left[\dfrac{\pi}{2}, \dfrac{2 \pi}{3}\right]\). (1 mark)

--- 3 WORK AREA LINES (style=lined) ---
iii. Hence, verify that \(f(x)\) has a stationary point for \(x \in\left[\dfrac{\pi}{2}, \frac{2 \pi}{3}\right]\). (1 mark)

--- 3 WORK AREA LINES (style=lined) ---
On the set of axes below, sketch the graph of \(y=f^{\prime}(x)\) on the domain \([-\pi, \pi]\), labelling the endpoints with their coordinates.
You may use the fact that the graph of \(y=f^{\prime}(x)\) has a local minimum at approximately \((-1.1,-1.4)\) and a local maximum at approximately \((1.1,1.4)\). (3 marks)

--- 0 WORK AREA LINES (style=lined) ---

Show Answers Only

a. \(\dfrac{\sqrt{3}\pi^2}{6}\)

bi. \(x\cos(x)+\sin(x)\)

bii. \(\left[-\dfrac{\pi}{3}+\dfrac{\sqrt{3}}{2},\ 1\right]\)

biii. \(\text{In the interval }\left[\dfrac{\pi}{2}, \dfrac{2\pi}{3}\right],\ f^{\prime}(x)\ \text{changes from positive to negative.}\)

\(\therefore\ f^{\prime}(x)=0\ \text{at some point in the interval, and hence a stationary point exists.}\)

Show Worked Solution

a.	\(A\)	\(=\dfrac{\pi}{3}\times\dfrac{1}{2}\left(f(0)+2f\left(\dfrac{\pi}{3}\right)+2f\left(\dfrac{2\pi}{3}\right)+f(\pi)\right)\)
		\(=\dfrac{\pi}{3}\left(0+2.\dfrac{\pi}{3}\sin\left(\dfrac{\pi}{3}\right)+2.\dfrac{2\pi}{3}\sin\left(\dfrac{2\pi}{3}\right)+\pi\sin({\pi})\right)\)
		\(=\dfrac{\pi}{6}\left(2\times \dfrac{\pi}{3}\times\dfrac{\sqrt{3}}{2}+2\times\dfrac{2\pi}{3}\times \dfrac{\sqrt{3}}{2}+0\right)\)
		\(=\dfrac{\pi}{6}\left(\dfrac{2\pi\sqrt{3}}{6}+\dfrac{4\pi\sqrt{3}}{6}\right)\)
		\(=\dfrac{\pi}{6}\times \dfrac{6\pi\sqrt{3}}{6}\)
		\(=\dfrac{\sqrt{3}\pi^2}{6}\)

bi.	\(f(x)\)	\(=x\sin(x)\)
	\(f^{\prime}(x)\)	\(=x\cos(x)+\sin(x)\)

bii.	\(f^{\prime}\left(\dfrac{\pi}{2}\right)\)	\(=1\)
	\(f^{\prime}\left(\dfrac{2\pi}{3}\right)\)	\(=\dfrac{2\pi}{3}\left(\dfrac{-1}{2}+\dfrac{\sqrt{3}}{2}\right)\)
		\(=-\dfrac{\pi}{3}+\dfrac{\sqrt{3}}{2}\)

\(\therefore\ \text{Range of }\ f^{\prime}(x)\ \text{is}\quad\left[-\dfrac{\pi}{3}+\dfrac{\sqrt{3}}{2},\ 1\right]\)

biii. \(\text{In the interval }\left[\dfrac{\pi}{2}, \dfrac{2\pi}{3}\right],\ f^{\prime}(x)\ \text{changes from positive to negative.}\)

\(\therefore\ f^{\prime}(x)=0\ \text{at some point in the interval, and hence a stationary point exists.}\)

c. \(f^{\prime}(\pi)=\pi\cos(\pi)+\sin(\pi)=-\pi\)

\(f^{\prime}(-\pi)=-\pi\cos(-\pi)+\sin(-\pi)=\pi\)

\(\therefore\ \text{Endpoints are }\ (-\pi,\ \pi)\ \text{and}\ (\pi,\ -\pi).\)

Calculus, MET2 2024 VCAA 15 MC

The points of inflection of the graph of \(y=2-\tan \left(\pi\left(x-\dfrac{1}{4}\right)\right)\) are

\(\left(k+\dfrac{1}{4}, 2\right), k \in Z\)
\(\left(k-\dfrac{1}{4}, 2\right), k \in Z\)
\(\left(k+\dfrac{1}{4},-2\right), k \in Z\)
\(\left(k-\dfrac{3}{4},-2\right), k \in Z\)

Show Answers Only

\(A\)

Show Worked Solution

\(\text{The graph of}\ \ y=-\tan(\pi x)\ \text{is translated 2 units upwards and then}\)

\(\text{translated }\dfrac{1}{4}\ \text{units to the right to become the graph shown below.}\)

\(\text{Hence all points of inflection lie of the line}\ y=2\)

\(\rightarrow\ \text{Eliminate Options C and D}\)

\(\text{Consider Option A:}\)

\(\text{When}\ \ k=0, x=0+\dfrac{1}{4}=\dfrac{1}{4}\)

\(\text{then}\ \ y=2-\tan \left(\pi\left(\dfrac{1}{4}-\dfrac{1}{4}\right)\right)=2\)

\(\text{Similarly for any}\ k \in Z\ \text{a point of inflection will be found at }\ \left(k+\dfrac{1}{4}, 2\right)\)

\(\Rightarrow A\)

Calculus, MET2 2023 VCE SM-Bank 2

Jac and Jill have built a ramp for their toy car. They will release the car at the top of the ramp and the car will jump off the end of the ramp.

The cross-section of the ramp is modelled by the function \(f\), where

\(f(x)= \begin{cases}\displaystyle \ 40 & 0 \leq x<5 \\ \dfrac{1}{800}\left(x^3-75 x^2+675 x+30\ 375\right) & 5 \leq x \leq 55\end{cases}\)

\(f(x)\) is both smooth and continuous at \(x=5\).

The graph of \(y=f(x)\) is shown below, where \(x\) is the horizontal distance from the start of the ramp and \(y\) is the height of the ramp. All lengths are in centimetres.

Find \(f^{\prime}(x)\) for \(0<x<55\). (2 marks)

--- 2 WORK AREA LINES (style=lined) ---
i. Find the coordinates of the point of inflection of \(f\). (1 mark)

--- 1 WORK AREA LINES (style=lined) ---
ii. Find the interval of \(x\) for which the gradient function of the ramp is strictly increasing. (1 mark)

--- 1 WORK AREA LINES (style=lined) ---
iii. Find the interval of \(x\) for which the gradient function of the ramp is strictly decreasing. (1 mark)

--- 1 WORK AREA LINES (style=lined) ---

Jac and Jill decide to use two trapezoidal supports, each of width \(10 cm\). The first support has its left edge placed at \(x=5\) and the second support has its left edge placed at \(x=15\). Their cross-sections are shown in the graph below.

Determine the value of the ratio of the area of the trapezoidal cross-sections to the exact area contained between \(f(x)\) and the \(x\)-axis between \(x=5\) and \(x=25\). Give your answer as a percentage, correct to one decimal place. (3 marks)

--- 5 WORK AREA LINES (style=lined) ---
Referring to the gradient of the curve, explain why a trapezium rule approximation would be greater than the actual cross-sectional area for any interval \(x \in[p, q]\), where \(p \geq 25\). (1 mark)

--- 4 WORK AREA LINES (style=lined) ---
Jac and Jill roll the toy car down the ramp and the car jumps off the end of the ramp. The path of the car is modelled by the function \(P\), where
1. 1. \(P(x)=\begin{cases}f(x) & 0 \leq x \leq 55 \\ g(x) & 55<x \leq a\end{cases}\)
\(P\) is continuous and differentiable at \(x=55, g(x)=-\frac{1}{16} x^2+b x+c\), and \(x=a\) is where the car lands on the ground after the jump, such that \(P(a)=0\).

1. Find the values of \(b\) and \(c\). (2 marks)
  
  --- 6 WORK AREA LINES (style=lined) ---
2. Determine the horizontal distance from the end of the ramp to where the car lands. Give your answer in centimetres, correct to two decimal places. (1 mark)
  
  --- 3 WORK AREA LINES (style=lined) ---

Show Answers Only

a. \(f^{\prime}(x)= \begin{cases}\displaystyle \ 0 & 0 < x<5 \\ \dfrac{1}{800}\left(3x^2-150 x+675 \right) & 5 \leq x < 55\end{cases}\)

b.i \((25, 20)\)

b.ii \([25, 55]\)

b.iii \([5, 25]\)

c. \(98.1\%\)

d. \(\text{In the interval }x\in [25, q]\text{ the curve is concave up.}\)

\(\therefore\ \text{The sloped edges of the trapeziums would be above }f(x)\)

\(\text{making the trapezium rule approximation greater than the}\)

\(\text{actual area.}\)

e.i \(b=8.75, c=-283.4375\)

e.ii \(34.10\ \text{cm}\)

Show Worked Solution

a. \(\text{Using CAS: Define f(x) then}\)

\(\text{OR}\quad f^{\prime}(x)= \begin{cases}\displaystyle \ 0 & 0 < x<5 \\ \dfrac{1}{800}\left(3x^2-150 x+675 \right) & 5 \leq x < 55\end{cases}\)

b.i \(\text{Using CAS: Find }f^”(x)\ \text{then solve }=0\)

\(\therefore\ \text{Point of inflection at }(25, 20)\)

b.ii \(\text{Gradient function strictly increasing for }x\in [25, 55]\)

b.iii \(\text{Gradient function strictly decreasing for }x\in [5, 25]\)

c. \(\text{Using CAS:}\)

\(\text{Area}\)	\(=\dfrac{h}{2}\Big(f(5)+2f(15)+f(25)\Big)\)
	\(=\dfrac{10}{2}\Big(40+2\times 33.75+20\Big)=637.5\)

\(\therefore\ \text{Estimate:Exact}\)	\(=637.5:650\)
	\(=\dfrac{637.5}{650}\times 100\)
	\(=98.0769\%\approx 98.1\%\)

d. \(\text{In the interval }x\in [25, q]\text{ the curve is concave up.}\)

\(\therefore\ \text{The sloped edges of the trapeziums would be above }f(x)\)

\(\text{making the trapezium rule approximation greater than the}\)

\(\text{actual area.}\)

e.i \(f(55)=\dfrac{35}{4}\ \text{(Using CAS)}\)

\(\text{and }f(55)=g(55)\rightarrow g(55)=\dfrac{35}{4}\)

\(\therefore -\dfrac{1}{16}\times 55^2+55b+c\)	\(=\dfrac{35}{4}\)
\(c\)	\(=\dfrac{35}{4}+\dfrac{3025}{16}-55b\)
\(c\)	\(=\dfrac{3165}{16}-55b\quad (1)\)

\(g'(x)=-\dfrac{x}{8}+b\)

\(\text{and }g'(55)=f'(55)\)

\(\rightarrow\ f'(55)=\dfrac{1}{800}(3\times 55^2-150\times 55+675)=\dfrac{15}{8}\)

\(\rightarrow\ -\dfrac{55}{8}+b=\dfrac{15}{8}\)

\(\therefore b=\dfrac{35}{4}\quad (2)\)

\(\text{Sub (2) into (1)}\)

\(c=\dfrac{3165}{16}-55\times\dfrac{35}{4}\)

\(c=-\dfrac{4535}{16}\)

\(\therefore\ b=\dfrac{35}{4}\ \text{or}\ 8.75 , c=-\dfrac{4535}{16}\ \text{or}\ -283.4375\)

e.ii \(\text{Using CAS: Solve }g(x)=0|x>55\)

\(\text{Horizontal distance}=\sqrt{365}+70-55=34.104\dots\approx 34.10\ \text{cm (2 d.p.)}\)

Calculus, MET2 2022 VCAA 16 MC

The function `f(x)=\frac{1}{3} x^3+m x^2+n x+p`, for `m, n, p \in R`, has turning points at `x=-3` and `x=1` and passes through the point (3, 4).

The values of `m, n` and `p` respectively are

`m=0, \quad n=-\frac{7}{3}, p=2`
`m=1, n=-3, \quad p=-5`
`m=-1, n=-3, \quad p=13`
`m=\frac{5}{4}, \quad n=\frac{3}{2}, \quad p=-\frac{83}{4}`
`m=\frac{5}{2}, \quad n=6, \quad p=-\frac{91}{2}`

Show Answers Only

`B`

Show Worked Solution

For turning point `f^{\prime}(x) = 0`

`f(x)`	`=\frac{1}{3} x^3 + mx^2 + nx + p`
`:.\ f^{\prime}(x)`	`= x^2-2mx+n`
` f^{\prime}(- 3)`	`= 9 – 6m + n` …. (1)
` f^{\prime}( 1)`	`= 1 + 2m + n` ….(2)

Solving (1) and (2)	`9 – 6m + n`	`= 0`
	`1 + 2m + n`	`= 0`

`m = 1` and `n = – 3`

`:. \ f(x)=\frac{1}{3} x^3 + x^2- 3x + p`

The point `(3 , 4)` lies on the line

`4`	`= \frac{1}{3} xx 3^3 +3^2- 3 xx 3 + p`
`:. \ p`	`= – 5 `

`:. \ f(x)=\frac{1}{3} x^3 + x^2- 3x – 5`

→ `m=1, n=-3, \quad p=-5`

`=>B`

Calculus, MET2 2023 VCAA 5 MC

Which one of the following functions has a horizontal tangent at \((0, 0)\)?

\(y=x^{-\frac{1}{3}}\)
\(y=x^{\frac{1}{3}}\)
\(y=x^{\frac{2}{3}}\)
\(y=x^{\frac{4}{3}}\)
\(y=x^{\frac{3}{4}}\)

Show Answers Only

\(D\)

Show Worked Solution

\(\text{Index must be greater than 1 otherwise the gradient function }\)

\(\text{will not be defined at }x=0.\)

\(y=x^{\frac{4}{3}}\ \ \Rightarrow \ \dfrac{dy}{dx}=\frac{4}{3}x^{\frac{1}{3}}\ \ (\text{only option defined at}\ x=0)\)

\(\Rightarrow D\)

Calculus, MET2-NHT 2019 VCAA 13 MC

The graph of `f(x) = x^3 - 6(b - 2)x^2 + 18x + 6` has exactly two stationary points for

`1 < b < 2`
`b = 1`
`b = (4 ± sqrt6)/(2)`
`(4 - sqrt6)/(2) ≤ b ≤ (4 + sqrt6)/(2)`
`b < (4 - sqrt6)/(2) \ or \ b > (4 + sqrt6)/(2)`

Show Answers Only

`E`

Show Worked Solution

`f(x)`	`= x^3 – 6(b – 2) x^2 + 18x + 6`
`f′(x)`	`= 3x^3 – 12(b – 2) x + 18`
`Δ`	`= [-12(b – 2)]^2 – 4 . 3 . 18`
	`= 144(b – 2)^2 – 216`

`text(If 2 solutions) \ => \ Δ > 0`

`text(S) text(olve for) \ b \ text{(by CAS):}`

`b < (4 – sqrt6)/(2) \ or \ b > (4 + sqrt6)/(2)`

`=> E`

Calculus, MET2 2018 VCAA 17 MC

The turning point of the parabola `y = x^2 - 2bx + 1` is closest to the origin when

`b = 0`
`b = -1 or b = 1`
`b = -1/sqrt 2 or b = 1/sqrt 2`
`b = 1/2 or b = -1/2`
`b = 1/4 or b = -1/4`

Show Answers Only

`C`

Show Worked Solution

`y = x^2 – 2bx + 1`

♦ Mean mark 45%.

`(dy)/(dx) = 2x – 2b`

`text(T.P. when)\ \ (dy)/(dx) = 0`

`2x – 2b`	`= 0`
`x`	`= b`

`text(T.P. at)\ \ P (b, 1 – b^2)`

`text(Find)\ \ D_p = text(distance of)\ P\ text(from origin:)`

`D_P`	`= sqrt(b^2 + (1 – b^2)^2)`
	`= sqrt(b^4 – b^2 + 1)`

`text(When)\ \ (dD_P)/(db) = 0,\ \ b = +- 1/sqrt 2`

`=> C`

Calculus, MET2 2018 VCAA 5 MC

Consider `f(x) = x^2 + p/x,\ x != 0,\ p in R`.

There is a stationary point on the graph of `f` when `x = -2`.

The value of `p` is

−16
–8
2
8
16

Show Answers Only

`A`

Show Worked Solution

`f(x) = x^2 + p/x`

`f^{′}(x) = 2x-p/x^2`

`text(S.P. occurs when)\ \ f^{′}(x) = 0 and x = -2\ text{(given)}`

`0`	`= 2(-2)-p/(-2)^2`
`:. p`	`= -16`

`=> A`

Calculus, MET2 2017 VCAA 11 MC

The function `f : R → R, \ f (x) = x^3 + ax^2 + bx` has a local maximum at `x = –1` and a local minimum at `x = 3`.

The values of `a` and `b` are respectively

`–2\ text(and)\ \ –3`
`text(2 and 1)`
`text(3 and)\ \ –9`
`–3\ text(and)\ \ –9`
`– 6\ text(and)\ \ –15`

Show Answers Only

`D`

Show Worked Solution

`f′(x) = 3x^2 +2ax +b`

`text(Solve:)quadquadf′(−1)`	`=3-2a+b= 0qquadtext(and)`
`f′(3)`	`=27+6a+b = 0qquadtext(for)\ a, b`

`:. a = −3, \ b = − 9`

`=> D`

Calculus, MET2 2007 VCAA 12 MC

Let `f: R -> R` be a differentiable function such that

`f prime(3) = 0`
`f prime(x) < 0` when `x < 3` and when `x > 3`

When `x = 3`, the graph of `f` has a

local minimum
local maximum
stationary point of inflection
point of discontinuity
gradient of 3

Show Answers Only

`C`

Show Worked Solution

`text(Use a gradient table:)`

`:.\ text(Point of inflection at)\ \ x = 3.`

`=> C`

Calculus, MET2 2009 VCAA 21 MC

A cubic function has the rule `y = f (x)`. The graph of the derivative function `f prime` crosses the `x`-axis at `(2, 0)` and `(– 3, 0)`. The maximum value of the derivative function is 10.

The value of `x` for which the graph of `y = f(x)` has a local maximum is

`– 2`
`2`
`– 3`
`3`
`– 1/2`

Show Answers Only

`B`

Show Worked Solution

`text(If)\ \ f(x)\ \ text(is cubic) -> f prime (x)\ \ text(is quadratic)`

`f′(x) >0\ \ text(for)\ \ x in (-3,2), and f′(x) =0\ \ text(at)\ \ x=2.`

`f′(x) <0\ \ text(for)\ \ x>2.`

`:.\ text(Local max occurs when)\ \ x = 2`

`=> B`

Calculus, MET2 2010 VCAA 17 MC

The function `f` is differentiable for all `x in R` and satisfies the following conditions.

`f prime (x) < 0\ \ text(where)\ \ x < 2`
`f prime (x) = 0\ \ text(where)\ \ x = 2`
`f prime (x) = 0\ \ text(where)\ \ x = 4`
`f prime (x) > 0\ \ text(where)\ \ 2 < x < 4`
`f prime (x) > 0\ \ text(where)\ \ x > 4`

Which one of the following is true?

The graph of `f` has a local maximum point where `x = 4.`
The graph of `f` has a stationary point of inflection where `x = 4.`
The graph of `f` has a local maximum point where `x = 2.`
The graph of `f` has a local minimum point where `x = 4.`
The graph of `f` has a stationary point of inflection where `x = 2.`

Show Answers Only

`B`

Show Worked Solution

`text(A table summary of the gradients is:)`

`:.\ text(Point of Inflection at)\ \ x = 4`

`=> B`

Calculus, MET2 2014 VCAA 4 MC

Let `f` be a function with domain `R` such that `f (5) = 0` and `f prime (x) < 0` when `x != 5.`

At `x = 5`, the graph of `f` has a

local minimum.
local maximum
gradient of 5
gradient of – 5
stationary point of inflection.

Show Answers Only

`E`

Show Worked Solution

`:.\ text(S)text(ince concavity doesn’t change,)`

`text(point of inflection at)\ \ x = 5.`

`=> E`

Calculus, MET2 2013 VCAA 19 MC

Part of the graph of a function `f: [0, oo) -> R, \ f(x) = e^(x sqrt 3) sin (x)` is shown below.

The first three turning points are labelled `T_1, T_2` and `T_3.`

The `x`-coordinate of `T_3` is

`(8 pi)/3`
`(16 pi)/3`
`(13 pi)/6`
`(17 pi)/6`
`(29 pi)/6`

Show Answers Only

`D`

Show Worked Solution

`f(x)`	`=e^(x sqrt 3) sin (x)`
`f′(x)`	`=sqrt3 e^(x sqrt 3) sin (x) + e^(x sqrt 3) cos (x)`

`text(Find)\ \ x\ \ text(when) f′(x) = 0:`

♦ Mean mark 50%.

`sqrt3 e^(x sqrt 3) sin (x)`	`= – e^(x sqrt 3) cos (x)`
`sqrt3 tan(x)`	`=-1`
`tan (x)`	`=- 1/sqrt3`

`x = (5pi)/6,(11pi)/6,(17pi)/6quad \ x∈(0,3pi)`

`=> D`

Calculus, MET2 2013 VCAA 21 MC

The cubic function `f: R -> R, f(x) = ax^3-bx^2 + cx`, where `a, b` and `c` are positive constants, has no stationary points when

`c > b^2/(4a)`
`c < b^2/(4a)`
`c < 4b^2a`
`c > b^2/(3a)`
`c < b^2/(3a)`

Show Answers Only

`D`

Show Worked Solution

`text(If no stationary points,)`

♦♦ Mean mark 29%.

`=>\ text(No solution to)\ \ f{′}(x) = 0`

`f^{′}(x) = 3ax^2 -2bx +c`

`text(No solution when,)`

`Delta`	`< 0`
`(−2b)^2-4(3ac)`	`< 0`
`3ac`	`> b^2`
`:. c`	`> (b^2)/(3a)`

`=> D`

Calculus, MET2 2012 VCAA 16 MC

The graph of a cubic function `f` has a local maximum at `(a, text{−3)}` and a local minimum at `(b, text{−8)}.`

The values of `c`, such that the equation `f(x) + c = 0` has exactly one solution, are

`3 < c < 8`
`c > -3 or c < -8`
`-8 < c < -3`
`c < 3 or c > 8`
`c < -8`

Show Answers Only

`D`

Show Worked Solution

`text(Sketch a possible graph:)`

♦♦ Mean mark 34%.

`text(For one solution:)`

`text(Shift graph up less than 3)`

`c < 3,\ \ text(or)`

`text(Shift graph up more than 8)`

`c > 8`

`=> D`