SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

PHYSICS, M5 2016 HSC 21a

Why does orbital decay occur more rapidly for satellites in a low-Earth orbit than for satellites in other orbits?   (2 marks)

Show Answers Only

Satellites in a low-Earth orbit:

→ Encounter more atmospheric drag than satellites in higher orbits due to their lower altitude.

→ This reduces their orbital velocity which decreases orbital radius which in turn causes orbital decay.

Show Worked Solution

Satellites in a low-Earth orbit:

→ Encounter more atmospheric drag than satellites in higher orbits due to their lower altitude.

→ This reduces their orbital velocity which decreases orbital radius which in turn causes orbital decay.


Mean mark 59%.

PHYSICS, M5 2024 HSC 13 MC

The diagram shows two identical satellites, \(A\) and \(B\), orbiting a planet.
 

Which row in the table correctly compares the potential energy, \(U\), and kinetic energy, \(K\), of the satellites?

\begin{align*}
\begin{array}{l}
\ \rule{0pt}{2.5ex}  \textbf{} \rule[-1.5ex]{0pt}{0pt}  & \\
\rule{0pt}{2.5ex}  \textbf{A.} \rule[-1ex]{0pt}{0pt} \\
\rule{0pt}{2.5ex}  \textbf{B.} \rule[-1ex]{0pt}{0pt} \\
\rule{0pt}{2.5ex}  \textbf{C.} \rule[-1ex]{0pt}{0pt} \\
\rule{0pt}{2.5ex}  \textbf{D.} \rule[-1ex]{0pt}{0pt} \\
\end{array}
\begin{array}{|c|c|}
\hline
\rule{0pt}{2.5ex} \textit{Potential energy} \rule[-1ex]{0pt}{0pt} & \textit{Kinetic energy} \\
\hline
\rule{0pt}{2.5ex} U_\text{A} > U_\text{B} \rule[-1ex]{0pt}{0pt} & K_\text{A} < K_\text{B} \\
\hline
\rule{0pt}{2.5ex} U_\text{A} < U_\text{B} \rule[-1ex]{0pt}{0pt} & K_\text{A} > K_\text{B} \\
\hline
\rule{0pt}{2.5ex} U_\text{A} > U_\text{B} \rule[-1ex]{0pt}{0pt} & K_\text{A} > K_\text{B} \\
\hline
\rule{0pt}{2.5ex} U_\text{A} < U_\text{B} \rule[-1ex]{0pt}{0pt} & K_\text{A} < K_\text{B} \\
\hline
\end{array}
\end{align*}

Show Answers Only

\(A\)

Show Worked Solution

→ As shown in the graph below, as the radius of a satellite increases the kinetic energy decreases and the gravitational potential energy increases.
  

→ This can also be concluded using the energy formulas for kinetic energy and gravitational potential energy:

\(K=\dfrac{GMm}{2r}\)  and  \(U=\dfrac{GMm}{r}\)

\(\Rightarrow A\)

PHYSICS, M5 2019 VCE 5*

Navigation in vehicles or on mobile phones uses a network of global positioning system (GPS) satellites. The GPS consists of 31 satellites that orbit Earth.

In December 2018, one satellite of mass 2270 kg, from the GPS Block \(\text{IIIA}\) series, was launched into a circular orbit at an altitude of \(20\ 000\) km above Earth's surface.

  1. Identify the type(s) of force(s) acting on the satellite and the direction(s) in which the force(s) must act to keep the satellite orbiting Earth.   (2 marks)
  1. Calculate the period of the satellite to three significant figures. You may use data from the table below in your calculations. Show your working.   (3 marks)

\begin{array}{|l|l|}
\hline \rule{0pt}{2.5ex}\text{mass of satellite} \rule[-1ex]{0pt}{0pt}& 2.27 \times 10^3 \ \text{kg} \\
\hline \rule{0pt}{2.5ex}\text{altitude of satellite above Earth's surface} \rule[-1ex]{0pt}{0pt}& 2.00 \times 10^7 \ \text{m} \\
\hline
\end{array}

Show Answers Only

a.    → Only force acting on a satellite is \(F_g\), the force due to gravity.

→ This force acts on the satellite directly towards the centre of the Earth.v

b.    \(4.25 \times 10^4\ \text{s}\)

Show Worked Solution


a.    → Only force acting on a satellite is \(F_g\), the force due to gravity.

→ This force acts on the satellite directly towards the centre of the Earth.
 



♦ Mean mark (a) 46%.
COMMENT: Thrust force is not a requirement for orbit. 


b.    \(\dfrac{r^3}{T^2}=\dfrac{GM}{4\pi^2}\ \ \Rightarrow \ \ T= \sqrt{\dfrac{4 \pi^2r^3}{GM}}\)

\(T=\sqrt{\dfrac{4\pi^2 \times (6.371 \times 10^6 + 2 \times 10^7)^3}{6.67 \times 10^{-11} \times 6 \times 10^{24}}}=42\ 533=4.25\times 10^4\ \text{s}\)

PHYSICS, M5 2020 VCE 4*

The Ionospheric Connection Explorer (ICON) space weather satellite, constructed to study Earth's ionosphere, was launched in October 2019. ICON will study the link between space weather and Earth's weather at its orbital altitude of 600 km above Earth's surface. Assume that ICON's orbit is a circular orbit. 

  1. Calculate the orbital radius of the ICON satellite.   (1 mark)

  2. Calculate the orbital period of the ICON satellite correct to three significant figures. Show your working.   (4 marks)

  3. Explain how the ICON satellite maintains a stable circular orbit without the use of propulsion engines.   (2 marks)

Show Answers Only

a.    \(r_{orbit}= 6.971 \times 10^6\ \text{m}\)

b.    \(5780\ \text{s}\)

c.    → The icon satellite is only subject to the gravitational force of the Earth.

→ This force is a centripetal force of constant magnitude and hence the satellite maintains a stable circular orbit. 

Show Worked Solution

a.    Radius of orbit is equal to the altitude plus the radius of the Earth.

\(r_{orbit}=600\ 000\ \text{m} + 6.371 \times 10^6\ \text{m} = 6.971 \times 10^6\ \text{m}\)

 

b.     \(\dfrac{r^3}{T^2}\) \(=\dfrac{GM}{4\pi^2}\)
  \(T\) \(=\sqrt{\dfrac{4\pi^2r^3}{GM}}\)
    \(=\sqrt{\dfrac{4\pi^2 \times (6.971 \times 10^6)^3}{6.67 \times 10^{-11} \times 6 \times 10^{24}}}\)
    \(=5780\ \text{s}\ \ \text{(3 sig.fig.)}\)

 

c.    → The icon satellite is only subject to the gravitational force of the Earth.

→ This force is a centripetal force of constant magnitude and hence the satellite maintains a stable circular orbit. 

♦♦♦ Mean mark (c) 23%.

PHYSICS, M5 2020 VCE 11 MC

The International Space Station (ISS) is travelling around Earth in a stable circular orbit, as shown in the diagram below.
 

Which one of the following statements concerning the momentum and the kinetic energy of the ISS is correct?

  1. Both the momentum and the kinetic energy vary along the orbital path.
  2. Both the momentum and the kinetic energy are constant along the orbital path.
  3. The momentum is constant, but the kinetic energy changes throughout the orbital path.
  4. The momentum changes, but the kinetic energy remains constant throughout the orbital path.
Show Answers Only

\(D\)

Show Worked Solution

→ The magnitude of both the kinetic energy and momentum of the ISS remains constant.

→ However, momentum is a vector and as the direction of the ISS is continually changing so is the direction of the momentum of the ISS.

\(\Rightarrow D\)

♦♦ Mean mark 31%.
COMMENT: Students need to ensure they consider the direction of vector quantities as well as the magnitudes.

PHYSICS, M5 2022 VCE 2

There are over 400 geostationary satellites above Earth in circular orbits. The period of orbit is one day (86 400 seconds). Each geostationary satellite remains stationary in relation to a fixed point on the equator. Figure 2 shows an example of a geostationary satellite that is in orbit relative to a fixed point, \(\text{X}\), on the equator.
 

  1. Explain why geostationary satellites must be vertically above the equator to remain stationary relative to Earth's surface.  (2 marks)
  1.  Using  \(G=6.67 \times 10^{-11}\ \text{N m}^{2}\ \text{kg}^{-2}, M_{\text{E}} = 5.98 \times 10^{24}\ \text{kg}\)  and  \(R_{\text{E}} = 6.37 \times 10^{6}\ \text{m}\), show that the altitude of a geostationary satellite must be equal to \(3.59 \times 10^{7}\ \text{m}\).  (4 marks)
  1. Calculate the speed of an orbiting geostationary satellite.  (3 marks)

Show Answers Only

a.   Reasons the satellite must orbit relative to a fixed point on the equator:

→ So that it is in the same rotational plane/axis of the Earth. 

→ The gravitational force on the satellite must be directly towards the centre of the Earth and it must orbit at a set altitude to the Earth where the speed of the satellite matches the rotational speed of the Earth.

→ As the speed and direction of the satellite matches that of the Earth, it will remain stationary relative to the motion of the Earth.

b.    See worked solutions

c.    3076 ms\(^{-1}\)

Show Worked Solution

a.   Reasons the satellite must orbit relative to a fixed point on the equator:

→ So that it is in the same rotational plane/axis of the Earth. 

→ The gravitational force on the satellite must be directly towards the centre of the Earth and it must orbit at a set altitude to the Earth where the speed of the satellite matches the rotational speed of the Earth.

→ As the speed and direction of the satellite matches that of the Earth, it will remain stationary relative to the motion of the Earth.
 

♦♦♦ Mean mark (a) 19%.
b.     \(\dfrac{r^3}{T^2}\) \(=\dfrac{GM}{4\pi^2}\)
  \(r\) \(=\sqrt[3]{\dfrac{GMT^2}{4\pi^2}}\)
    \(=\sqrt[3]{\dfrac{6.67 \times 10^{-11} \times 5.98 \times 10^{24} \times (86\ 400)^2}{4\pi^2}}\)
    \(=42.250 \times 10^6\ \text{m}\)

 
\(\therefore \ \text{Altitude}\ =42.250 \times 10^6 -6.37 \times 10^6 =3.59 \times 10^7\ \text{m}\)
 

c.     \(v\) \(=\sqrt{\dfrac{GM}{r}}\)
    \(=\sqrt{\dfrac{6.67 \times 10^{-11} \times 5.98 \times 10^{24}}{42.25 \times 10^6}}\)
    \(=3073\ \text{ms}^{-1}\)

PHYSICS, M5 2023 HSC 34

A 400 kg satellite is travelling in a circular orbit of radius 6.700 × 10\(^6\) m around Earth. Its potential energy is –2.389 × 10\(^{10}\ \text{J}\) and its total energy is –1.195 × 10\(^{10}\ \text{J}\).
 

At point \(P\), the satellite's engines are fired, increasing the satellite's velocity in the direction of travel and causing its kinetic energy to increase by 5.232 × 10\(^8\ \text{J}\). Assume that this happens instantaneously and that the engine is then shut down.

The satellite follows the trajectory shown, which passes through \(Q\), 6.850 × 10\(^6\) m from Earth's centre.

  1. Analyse qualitatively the energy changes as the satellite moves from \(P\) to \(Q\).  (2 marks)
  1. Show that the kinetic energy of the satellite at \(Q\) is 1.194 × 10\(^{10}\ \text{J}\).  (4 marks)
  1. Explain the motion of the satellite after it passes through \(Q\).  (3 marks)

Show Answers Only

a.   Energy changes from \(P\) to \(Q\):

→ As the engine is shut down immediately after increasing the kinetic energy, the total energy of the system will remain the same.

→ As a result of the Law of Conservation of Energy, as the distance of the satellite from the Earth increases, the gravitational potential energy of the satellite increases and the kinetic energy of the satellite decreases.

 

b.   Using the Law of Conservation of Energy:

\(E_Q\) \(=U_Q+K_Q\) \(=E_P+K_{\text{engine}}\)
 
\(U_Q\) \(=-\dfrac{GMm}{r}\)  
  \(=-\dfrac{6.67 \times 10^{-11} \times 6 \times 10^{24} \times 400}{6.85 \times 10^6}\)  
  \(=-2.337 \times 10^{10}\) \(\text{J}\)  

 

\(K_Q\) \(=E_P+K_{\text{engine}}-U_Q\)  
  \(=-1.195 \times 10^{10} + 5.232 \times 10^8 -(-2.337 \times 10^{10})\)  
  \(=1.194 \times 10^{10}\) \(\text{J … as required}\)  

 

c.   The orbital velocity at \(Q\) is:

\(v\) \(=\sqrt{\dfrac{GM}{r}}\)  
  \(=\sqrt{\dfrac{6.67 \times 10^{-11} \times 6 \times 10^{24}}{6.85 \times 10^6}}\)  
  \(=7.644 \times 10^3\ \text{ms}^{-1}\)  

 

The velocity of the satellite at \(Q\) is:

\(K_Q\) \(=\dfrac{1}{2}mv_Q^2\)   
\(v_Q\) \(=\sqrt{\dfrac{2K_Q}{m}}\)  
  \(=\sqrt{\dfrac{2 \times 1.194 \times 10^{10}}{400}}\)  
  \(=7.727 \times 10^3\ \text{ms}^{-1}\)  

 

→ As the velocity of the satellite at \(Q\) is greater than the orbital velocity at  \(r\) = 6.85 × 10\(^6\) m, the satellite will continue to move further away from the Earth.

→ Using the Law of Conservation of Energy, the kinetic energy of the satellite will decrease as the gravitational potential energy of the satellite increases. Hence the satellite will increase its distance from the Earth and slow down until the velocity of the satellite is equal to the orbital velocity at its new distance from the Earth.

Show Worked Solution

a.   Energy changes from \(P\) to \(Q\):

→ As the engine is shut down immediately after increasing the kinetic energy, the total energy of the system will remain the same.

→ As a result of the Law of Conservation of Energy, as the distance of the satellite from the Earth increases, the gravitational potential energy of the satellite increases and the kinetic energy of the satellite decreases.
 

♦ Mean mark (a) 47%.

b.   Using the Law of Conservation of Energy:

\(E_Q\) \(=U_Q+K_Q\) \(=E_P+K_{\text{engine}}\)
 
\(U_Q\) \(=-\dfrac{GMm}{r}\)  
  \(=-\dfrac{6.67 \times 10^{-11} \times 6 \times 10^{24} \times 400}{6.85 \times 10^6}\)  
  \(=-2.337 \times 10^{10}\) \(\text{J}\)  

 

\(K_Q\) \(=E_P+K_{\text{engine}}-U_Q\)  
  \(=-1.195 \times 10^{10} + 5.232 \times 10^8 -(-2.337 \times 10^{10})\)  
  \(=1.194 \times 10^{10}\) \(\text{J … as required}\)  
♦ Mean mark (b) 55%.

c.   The orbital velocity at \(Q\) is:

\(v\) \(=\sqrt{\dfrac{GM}{r}}\)  
  \(=\sqrt{\dfrac{6.67 \times 10^{-11} \times 6 \times 10^{24}}{6.85 \times 10^6}}\)  
  \(=7.644 \times 10^3\ \text{ms}^{-1}\)  

 

The velocity of the satellite at \(Q\) is:

\(K_Q\) \(=\dfrac{1}{2}mv_Q^2\)   
\(v_Q\) \(=\sqrt{\dfrac{2K_Q}{m}}\)  
  \(=\sqrt{\dfrac{2 \times 1.194 \times 10^{10}}{400}}\)  
  \(=7.727 \times 10^3\ \text{ms}^{-1}\)  

♦♦ Mean mark (c) 35%.

→ As the velocity of the satellite at \(Q\) is greater than the orbital velocity at  \(r\) = 6.85 × 10\(^6\) m, the satellite will continue to move further away from the Earth.

→ Using the Law of Conservation of Energy, the kinetic energy of the satellite will decrease as the gravitational potential energy of the satellite increases. Hence the satellite will increase its distance from the Earth and slow down until the velocity of the satellite is equal to the orbital velocity at its new distance from the Earth.

PHYSICS, M5 EQ-Bank 9 MC

A satellite is orbiting a planet at a fixed altitude.

Which row of the table correctly identifies the magnitude of the work done by the forces on the satellite and the reason for this being the case?

\begin{align*}
\begin{array}{l}
\rule{0pt}{2.5ex}\textit{}& \textit{} \\
\textit{}\rule[-2ex]{0pt}{0pt}& \textit{} \\
\rule{0pt}{2.5ex}\textbf{A.}\rule[-1ex]{0pt}{0pt}\\
\rule{0pt}{2.5ex}\textbf{B.}\rule[-1ex]{0pt}{0pt}\\
\textbf{}\rule[-1ex]{0pt}{0pt}\\
\rule{0pt}{2.5ex}\textbf{C.}\rule[-1ex]{0pt}{0pt}\\
\textbf{}\rule[-1ex]{0pt}{0pt}\\
\rule{0pt}{2.5ex}\textbf{D.}\rule[-1ex]{0pt}{0pt}\\
\textbf{}\rule[-1ex]{0pt}{0pt}\\
\end{array}
\begin{array}{|c|l|}
\hline
\rule{0pt}{2.5ex}\textit{Magnitude of}&  \quad \quad \quad \quad \quad \quad \quad \quad \quad \quad\textit{Reason} \\
\textit{work done}\rule[-1ex]{0pt}{0pt}& \textit{} \\
\hline
\rule{0pt}{2.5ex}\text{Zero}\rule[-1ex]{0pt}{0pt}&\text{The net force on the satellite is zero.}\\
\hline
\rule{0pt}{2.5ex}\text{Zero}& \text{Gravity acts at 90 degrees to the direction of motion of the}\\
\text{}\rule[-1ex]{0pt}{0pt}& \text{satellite} \\
\hline
\rule{0pt}{2.5ex}\text{Greater than}& \text{The work done equals the kinetic energy of the satellite.} \\
\text{zero}\rule[-1ex]{0pt}{0pt}& \text{} \\
\hline
\rule{0pt}{2.5ex}\text{Greater than}& \text{The work done equals the gravitational force multiplied by} \\
\text{zero}\rule[-1ex]{0pt}{0pt}& \text{the length of the orbital path of the satellite.} \\
\hline
\end{array}
\end{align*}

Show Answers Only

`B`

Show Worked Solution

→ The work done by a force on an object is the product of the displacement of the object and the magnitude of the force in the direction of the displacement.

→ The satellite is orbiting the planet in uniform circular motion, so the force of gravity acts perpendicular to the direction of motion of the satellite.

→ So, zero work is done on the satellite.

`=>B`

PHYSICS, M5 2015 HSC 19 MC

An astronaut working outside a spacecraft in orbit around Earth is not attached to it.

Why does the astronaut NOT drift away from the spacecraft?

  1. The force of gravity acting on the astronaut and spacecraft is negligible.
  2. The spacecraft and the astronaut are in orbit around the Sun with the Earth.
  3. The forces due to gravity acting on both the astronaut and the spacecraft are the same.
  4. The accelerations of the astronaut and the spacecraft are inversely proportional to their respective masses.
Show Answers Only

`D`

Show Worked Solution

By Elimination:

→ Gravity is the force keeping the spacecraft and the astronaut in orbit, so it is not negligible (eliminate A).

→ Earths rotation around the sun is not relevant to the motion of the astronaut and the spacecraft relative to Earth (eliminate B).

→ Using  `F=(GMm)/(r^2)`, the force due to gravity acting on both is proportional to their respective masses. Since the spacecraft is significantly heavier, it will experience a greater force due to gravity (eliminate C).

→ The accelerations, `g=(F)/(m)` of the spacecraft and the astronaut are inversely proportional to their respective masses. As they both experience a force, `F`, due to gravity proportional to their masses, their accelerations are the same. Therefore, the astronaut will not drift from the spacecraft.

`=>D`


♦♦♦ Mean mark 15%.

PHYSICS, M5 2015 HSC 11 MC

Which of the following diagrams correctly represents the force(s) acting on a satellite in a stable circular orbit around Earth?
 

 

Show Answers Only

`D`

Show Worked Solution

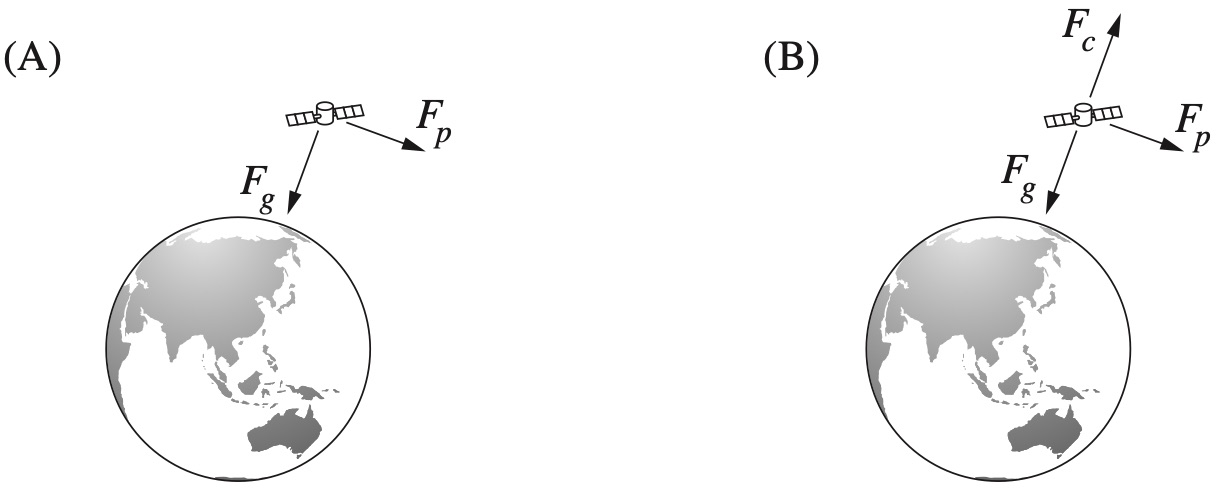
→ In a stable, circular orbit the satellite requires no propulsion force to keep it in orbit. This is due to it undergoing uniform circular motion with gravity acting as its centripetal force.

→ A force of gravity acts on the satellite, with direction towards Earth’s centre of mass. 

→ No ‘reaction force’ acts on the satellite. This is because any ‘reaction’ to the gravitational force exerted on the satellite by the Earth is an equal and opposite force exerted on the Earth by the satellite (Newton’s Third Law).

→ i.e. The reaction force acts on the Earth, not the satellite.

`=>D`


♦♦♦ Mean mark 29%.

PHYSICS, M5 2018 HSC 3 MC

The graph shows the altitude of the International Space Station (ISS) during 2017.
 

The altitude can only be boosted by supply craft visiting the ISS.

Why does the altitude decrease in the times between height boosts?

  1. Momentum of the ISS is being transferred to air molecules.
  2. The moon's gravity changes the net force on the ISS as it orbits Earth.
  3. The decrease in altitude makes it possible for a supply craft to reach the ISS.
  4. The total mass of the ISS changes with the deliveries from each supply craft.
Show Answers Only

`A`

Show Worked Solution

→ Collisions between the ISS and air molecules cause the momentum of the ISS to be transferred to air molecules.

→ This causes the velocity of the ISS to decrease. Using the formula

`v=sqrt((GM)/(r))\ \ =>\ \   r=(GM)/(v^2).`

→ Therefore, the orbital radius (altitude) of the ISS decreases.

`=>A`

PHYSICS, M5 2018 HSC 1 MC

A satellite orbits Earth as shown.

Which diagram correctly shows the direction of the satellite's acceleration?
 

 

Show Answers Only

`A`

Show Worked Solution

The direction of acceleration is the same as the direction of force acting on the satellite, which is towards the centre of mass of the Earth.

`=> A`


Mean mark 64%.
COMMENT: A surprising one third of students answered incorrectly.

PHYSICS M5 2022 HSC 35

A capsule travels around the International Space Station (ISS) in a circular path of radius 200 m as shown.
 


 

Analyse this system to test the hypothesis below.  (5 marks)

The uniform circular motion of the capsule around the ISS can be accounted for in terms of the gravitational force between the capsule and the ISS.

Show Answers Only

Find the gravitational force between the capsule and the ISS:

`F` `=(GMm)/(r^2)`  
  `=(6.67 xx10^-11 xx4.2 xx10^5 xx 1.2 xx10^4)/(200^2)`  
  `=8.4 xx10^(-6)  text{N}`  

 
Find the centripetal force required to keep the capsule in its orbit:

`F_(c)` `=(mv^2)/(r)`  
  `=(1.2 xx10^4 xx 0.233^2)/(200)`  
  `=3.26  text{N}`  

 

→ The gravitational force is not sufficient to provide the necessary centripetal force to keep the capsule in orbit around the ISS.

→ The hypothesis is invalid.

Show Worked Solution

Find the gravitational force between the capsule and the ISS:

`F` `=(GMm)/(r^2)`  
  `=(6.67 xx10^-11 xx4.2 xx10^5 xx 1.2 xx10^4)/(200^2)`  
  `=8.4 xx10^(-6)  text{N}`  

 
Find the centripetal force required to keep the capsule in its orbit:

`F_(c)` `=(mv^2)/(r)`  
  `=(1.2 xx10^4 xx 0.233^2)/(200)`  
  `=3.26  text{N}`  

 

→ The gravitational force is not sufficient to provide the necessary centripetal force to keep the capsule in orbit around the ISS.

→ The hypothesis is invalid.

PHYSICS, M5 2019 HSC 9 MC

Two satellites have the same mass. One (LEO) is in low-Earth orbit and the other (GEO) is in a geostationary orbit.

The total energy of a satellite is half its gravitational potential energy.

Which row of the table correctly identifies the satellite with the greater orbital period and the satellite with the greater total energy?

\begin{align*}
\begin{array}{l}
\rule{0pt}{2.5ex} \ \rule[-1ex]{0pt}{0pt}& \\
\rule{0pt}{2.5ex}\textbf{A.}\rule[-1ex]{0pt}{0pt}\\
\rule{0pt}{2.5ex}\textbf{B.}\rule[-1ex]{0pt}{0pt}\\
\rule{0pt}{2.5ex}\textbf{C.}\rule[-1ex]{0pt}{0pt}\\
\rule{0pt}{2.5ex}\textbf{D.}\rule[-1ex]{0pt}{0pt}\\
\end{array}
\begin{array}{|c|c|}
\hline
\rule{0pt}{2.5ex}\textit{Greater orbital period}\rule[-1ex]{0pt}{0pt}& \textit{Greater total energy} \\
\hline
\rule{0pt}{2.5ex}\text{LEO}\rule[-1ex]{0pt}{0pt}&\text{LEO}\\
\hline
\rule{0pt}{2.5ex}\text{LEO}\rule[-1ex]{0pt}{0pt}& \text{GEO}\\
\hline
\rule{0pt}{2.5ex}\text{GEO}\rule[-1ex]{0pt}{0pt}& \text{LEO} \\
\hline
\rule{0pt}{2.5ex}\text{GEO}\rule[-1ex]{0pt}{0pt}& \text{GEO} \\
\hline
\end{array}
\end{align*}

Show Answers Only

`D`

Show Worked Solution

Orbital velocity decreases as the orbital radius of a satellite increases.

→ the geostationary satellite has the greater orbital period

Total energy increases as orbital radius increases.

→ the geostationary satellite has the greater total energy

`=>D`


♦ Mean mark 43%.