SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Networks, GEN2 2024 VCAA 15

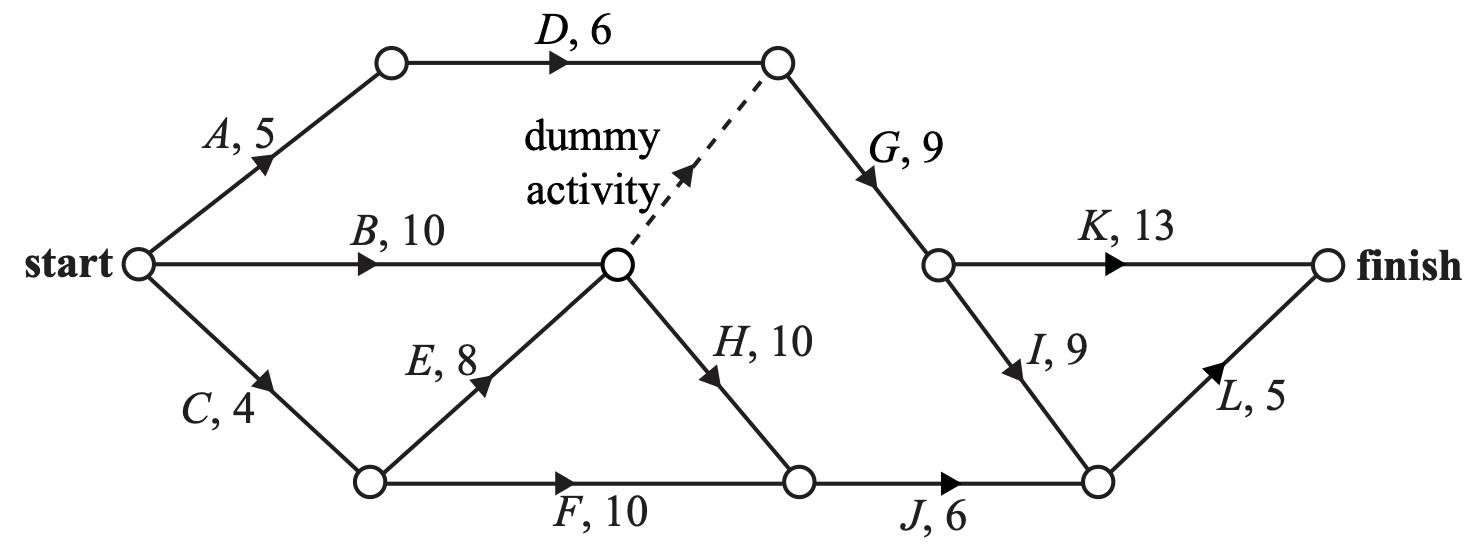
An upgrade to the supermarket requires the completion of 11 activities, to .

The directed network below shows these activities and their completion time, in weeks.

The minimum completion time for the project is 29 weeks.
 

 

  1. Write down the critical path.   (1 mark)

  2. Which activity can be delayed for the longest time without affecting the minimum completion time of the project?   (1 mark)

Use the following information to answer parts c-e.

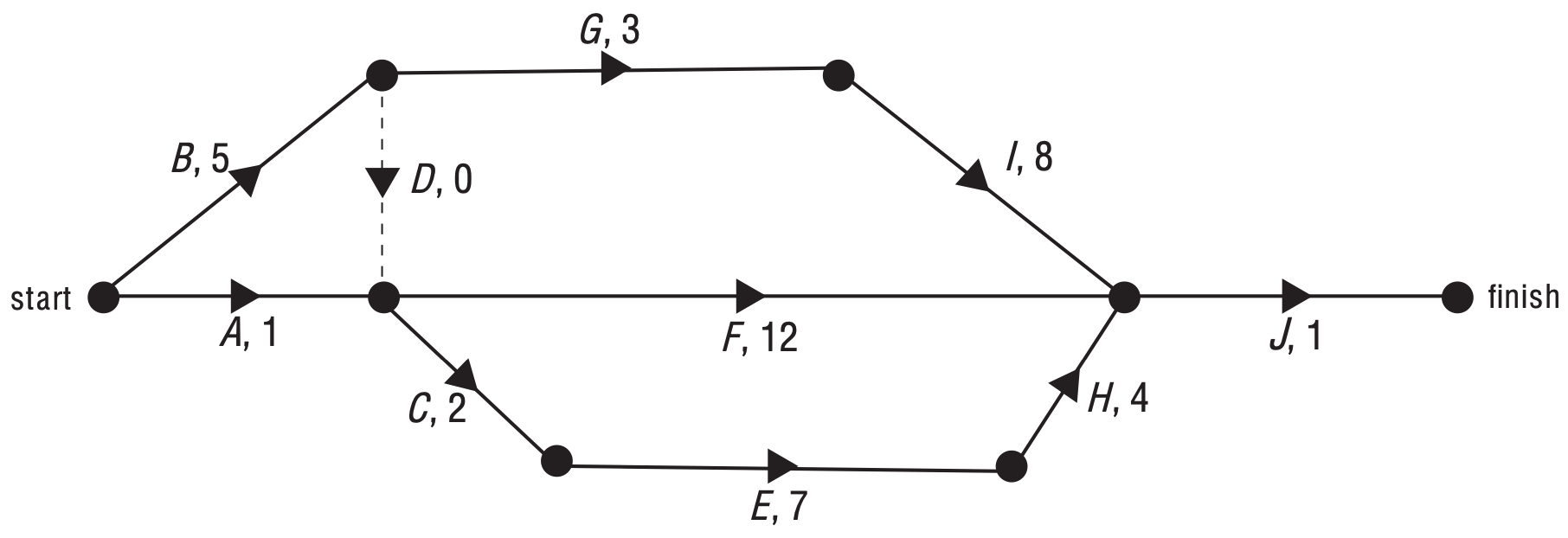
A change is made to the order of activities.

The table below shows the activities and their new latest starting times in weeks.

A dummy activity is now required in the network.

  1. On the directed network below, draw a directed edge to represent the dummy activity. Include a label.  (1 mark)

     

  1. What is the new minimum completion time of the project?  (1 mark)
  2. The owners of the supermarket want the project completed earlier.
  3. They will pay to reduce the time of some of the activities.
  4. A reduction in completion time of an activity will incur an additional cost of $10 000 per week.
  5. Activities can be reduced by a maximum of two weeks.
  6. The minimum number of weeks an activity can be reduced to is seven weeks.
  7. What is the minimum amount the owners of the supermarket will have to pay to reduce the completion time of the project as much as possible?   (1 mark)

Show Answers Only

a.    

b.    

c.    

d.    

e.    

Show Worked Solution

a.    
 

 
b.    


 

♦♦ Mean mark (b) 34%.

c.    

♦♦♦ Mean mark (c) 10%.

d.    
 

♦♦♦ Mean mark (d) 27%.

e.   

♦♦♦ Mean mark (e) 7%.

Networks, GEN2 2023 VCAA 14

One of the landmarks in state requires a renovation project.

This project involves 12 activities, to . The directed network below shows these activities and their completion times, in days.
 

The table below shows the 12 activities that need to be completed for the renovation project.

It also shows the earliest start time (EST), the duration, and the immediate predecessors for the activities.

The immediate predecessor(s) for activity and the EST for activity are missing.

  1. Write down the immediate predecessor(s) for activity .  (1 mark)

  2. What is the earliest start time, in days, for activity ?  (1 mark)

  3. How many activities have a float time of zero?  (1 mark)

The managers of the project are able to reduce the time, in days, of six activities.

These reductions will result in an increase in the cost of completing the activity.

The maximum decrease in time of any activity is two days.

  1. If activities and have their completion time reduced by two days each, the overall completion time of the project will be reduced.
  2. What will be the maximum reduction time, in days?  (1 mark)
  3. The managers of the project have a maximum budget of $15 000 to reduce the time for several activities to produce the maximum reduction in the project's overall completion time.
  4. Complete the table below, showing the reductions in individual activity completion times that would achieve the earliest completion time within the $ 15 000 budget.  (1 mark)

Show Answers Only

a.   

b.   

c.   

d.   

e.    

Show Worked Solution

a.   


 

b.   


 

c.   


 

d.   

♦♦ Mean mark (c) 35%.
♦♦ Mean mark (d) 33%.

e.    

♦♦♦ Mean mark (e) 9%.

NETWORKS, FUR2 2021 VCAA 4

Roadworks planned by the local council require 13 activities to be completed.

The network below shows these 13 activities and their completion times in weeks.
 

  1. What is the earliest start time, in weeks, of activity ?   (1 mark)

  2. How many of these activities have zero float time?   (1 mark)

  3. It is possible to reduce the completion time for activities and .
  4. The reduction in completion time for each of these five activities will incur an additional cost.
  5. The table below shows the five activities that can have their completion time reduced and the associated weekly cost, in dollars.
     
       
  6. The completion time for each these five activities can be reduced by a maximum of two weeks.
  7. The overall completion time for the roadworks can be reduced to 16 weeks.
  8. What is the minimum cost, in dollars, of this change in completion time?   (1 mark)

Show Answers Only
Show Worked Solution

a.    


 

b.    


 

c.   


  


  


 


 

NETWORKS, FUR1 2021 VCAA 6 MC

The directed graph below shows the sequence of activities required to complete a project.

The time taken to complete each activity, in hours, is also shown.
 

The minimum completion time for this project is 18 hours.

The time taken to complete activity is labelled  .

The maximum value of    is

  1. 2
  2. 3
  3. 4
  4. 5
  5. 6
Show Answers Only

Show Worked Solution

♦ Mean mark 46%.


 


 

NETWORKS, FUR1 2020 VCAA 10 MC

The directed network below shows the sequence of activities, to , that is required to complete an office renovation.

The time taken to complete each activity, in weeks, is also shown.
 


 

The project manager would like to complete the office renovation in less time.

The project manager asks all the workers assigned to activity to also work on activity .

This will reduce the completion time of activity to three weeks.

The workers assigned to activity cannot work on both activity and activity at the same time.

No other activity times will be changed.

This change to the network will result in a change to the completion time of the office renovation.

Which one of the following is correct?

  1. The completion time will be reduced by one week if activity is completed before activity is started.
  2. The completion time will be reduced by three weeks if activity is completed before activity is started.
  3. The completion time will be reduced by one week if activity is completed before activity is started.
  4. The completion time will be reduced by three weeks if activity is completed before activity is started.
  5. The completion time will be increased by three weeks if activity is completed before activity is started.
Show Answers Only

Show Worked Solution

♦♦♦ Mean mark 24%.


 


 

NETWORKS, FUR1 2020 VCAA 6 MC

The activity network below shows the sequence of activities required to complete a project.

The number next to each activity in the network is the time it takes to complete that activity, in days.

 

The minimum completion time for this project, in days, is

  1. 18
  2. 19
  3. 20
  4. 21
  5. 22
Show Answers Only

Show Worked Solution


 

 
 

 

NETWORKS, FUR1 2018 VCAA 5 MC

The directed network below shows the sequence of 11 activities that are needed to complete a project.

The time, in weeks, that it takes to complete each activity is also shown.
 


 

How many of these activities could be delayed without affecting the minimum completion time of the project?

  1. 3
  2. 4
  3. 5
  4. 6
  5. 7
Show Answers Only

Show Worked Solution

♦ Mean mark 43%.

NETWORKS, FUR2 2018 VCAA 3

At the Zenith Post Office all computer systems are to be upgraded.

This project involves 10 activities, to .

The directed network below shows these activities and their completion times, in hours.
 

  1. Determine the earliest starting time, in hours, for activity .   (1 mark)

  2. The minimum completion time for the project is 15 hours.

     

    Write down the critical path.   (1 mark)

  3. Two of the activities have a float time of two hours.

     

    Write down these two activities.   (1 mark)

  4. For the next upgrade, the same project will be repeated but one extra activity will be added.
    This activity has a duration of one hour, an earliest starting time of five hours and a latest starting time of 12 hours.

     

    Complete the following sentence by filling in the boxes provided.   (1 mark)

     

    The extra activity could be represented on the network above by a directed edge from the

   end of activity   
 
   to the start of activity   
 
Show Answers Only
  3.  
Show Worked Solution

a.  

 

 
b. 
 

c.  

♦ Mean mark 45%.

 


 


 

d.  

♦♦ Mean mark 25%.
 

NETWORKS, FUR1 2017 VCAA 4-5 MC

The directed graph below shows the sequence of activities required to complete a project.

The time to complete each activity, in hours, is also shown.
 


 

Part 1

The earliest starting time, in hours, for activity is

  1. 3
  2. 10
  3. 11
  4. 12
  5. 13

 

Part 2

To complete the project in minimum time, some activities cannot be delayed.

The number of activities that cannot be delayed is

  1. 2
  2. 3
  3. 4
  4. 5
  5. 6
Show Answers Only

Show Worked Solution

 

 

NETWORKS, FUR2 2016 VCAA 3

A new skateboard park is to be built in Beachton.

This project involves 13 activities, to .

The directed network below shows these activities and their completion times in days.
 


 

  1. Determine the earliest start time for activity .   (1 mark)

  2. The minimum completion time for the skateboard park is 15 days.

     

    Write down the critical path for this project.   (1 mark)

  3. Which activity has a float time of two days?   (1 mark)

  4. The completion times for activities and can each be reduced by one day.

     

    The cost of reducing the completion time by one day for these activities is shown in the table below.
     

     

       

     

    What is the minimum cost to complete the project in the shortest time possible?   (1 mark)

  5. The original skateboard park project from part (a), before the reduction of time in any activity, will be repeated at another town named Campville, but with the addition of one extra activity.

     

    The new activity, , will take six days to complete and has a float time of one day.

     

    Activity will finish at the same time as the project.

     

     i.  Add activity to the network below.   (1 mark) 


      
    ii.  What is the latest start time for activity ?   (1 mark)

Show Answers Only

Show Worked Solution
a.   
   

  
b.  

♦♦ Mean mark part (c) 37%, part (d) 21%.
MARKER’S COMMENT: In part (d), cannot be crashed, therefore shortest duration is 14 days. Activity is cheapest to reduce.
  

c.  
  

d.  


  

e.i.   

♦♦ Mean mark part (e)(i) 21%, (e)(ii) 27%.
MARKER’S COMMENT: In (e)(ii), activity must have an arrow on it.
  

e.ii.   
   
   

NETWORKS, FUR1 2016 VCAA 6-7 MC

The directed graph below shows the sequence of activities required to complete a project.

All times are in hours.
 

 
Part 1

The number of activities that have exactly two immediate predecessors is

  1. 0
  2. 1
  3. 2
  4. 3
  5. 4

 
Part 2

There is one critical path for this project.

Three critical paths would exist if the duration of activity

  1. I were reduced by two hours.
  2. E were reduced by one hour.
  3. G were increased by six hours.
  4. K were increased by two hours.
  5. F were increased by two hours. 
Show Answers Only

Show Worked Solution

 

♦ Mean mark of Part 2: 45%.

NETWORKS, FUR1 2008 VCAA 8-9 MC

The network below shows the activities that are needed to finish a particular project and their completion times (in days).

 

networks-fur1-2008-vcaa-8-mc

Part 1

The earliest start time for Activity , in days, is

A.     7

B.   15

C.   16

D.   19

E.   20

 

Part 2

This project currently has one critical path.

A second critical path, in addition to the first, would be created by

A.   increasing the completion time of by 7 days.

B.   increasing the completion time of by 1 day.

C.   increasing the completion time of by 2 days.

D.   decreasing the completion time of by 1 day.

E.   decreasing the completion time of by 2 days.

Show Answers Only

Show Worked Solution

 

♦♦ Mean mark of Part 2 was 35%.

NETWORKS, FUR1 2006 VCAA 9 MC

The network below shows the activities and their completion times (in hours) that are needed to complete a project.
 


 

The project is to be crashed by reducing the completion time of one activity only.

This will reduce the completion time of the project by a maximum of

A.   1 hour 

B.   2 hours

C.   3 hours

D.   4 hours

E.   5 hours

Show Answers Only

Show Worked Solution

♦♦♦ Mean mark 17%.
MARKER’S COMMENT: When choosing an activity to crash, take care that a new critical path is not created.

 

NETWORKS, FUR1 2007 VCAA 5-6 MC

The following network shows the activities that are needed to complete a project and their completion times (in hours).
 


 

Part 1

Which one of the following statements regarding this project is false?

A.   Activities and all have the same earliest start time.

B.   There is only one critical path for this project.

C.   Activity may start later than activity

D.   The shortest path gives the minimum time for project completion.

E.   Activity must be on the critical path.

 

Part 2

The earliest start time for activity , in hours, is

A.   11

B.   12

C.   14

D.   15

E.   16

Show Answers Only

Show Worked Solution

♦ Mean mark 43%.

 

 

  

NETWORKS, FUR1 2012 VCAA 9 MC

John, Ken and Lisa must work together to complete eight activities, and , in minimum time.

The directed network below shows the activities, their completion times in days, and the order in which they must be completed.

net9

Several activities need special skills. Each of these activities may be completed only by a specified person.

  • Activities and may only be completed by John.
  • Activities and may only be completed by Ken.
  • Activities and may only be completed by Lisa.
  • Activities and may be completed by any one of John, Ken or Lisa.

With these conditions, the minimum number of days required to complete these eight activities is

A.  14

B.  17

C.  20

D.  21

E.  24

Show Answers Only

Show Worked Solution

♦♦♦ Mean mark 18%.

 

NETWORKS, FUR2 2007 VCAA 4

A community centre is to be built on the new housing estate.

Nine activities have been identified for this building project.

The directed network below shows the activities and their completion times in weeks.
 

NETWORKS, FUR2 2007 VCAA 4
 

  1. Determine the minimum time, in weeks, to complete this project.   (1 mark)

  2. Determine the slack time, in weeks, for activity .   (2 marks)

The builders of the community centre are able to speed up the project.

Some of the activities can be reduced in time at an additional cost.

The activities that can be reduced in time are , , , and .

  1. Which of these activities, if reduced in time individually, would not result in an earlier completion of the project?   (1 mark)

The owner of the estate is prepared to pay the additional cost to achieve early completion.

The cost of reducing the time of each activity is $5000 per week.

The maximum reduction in time for each one of the five activities, , , , , , is 2 weeks.

  1. Determine the minimum time, in weeks, for the project to be completed now that certain activities can be reduced in time.   (1 mark)

  2. Determine the minimum additional cost of completing the project in this reduced time.   (1 mark)

Show Answers Only
Show Worked Solution

a.  

♦ Mean mark of all parts (combined) 40%.
 

 

b.   
 
 

  
c.   


  

d.   

 

e.   
   

NETWORKS, FUR1 2014 VCAA 8 MC

Which one of the following statements about critical paths is true?

  1. There can be only one critical path in a project.
  2. A critical path always includes at least two activities.
  3. A critical path will always include the activity that takes the longest time to complete.
  4. Reducing the time of any activity on a critical path for a project will always reduce the minimum completion time for the project.
  5. If there are no other changes, increasing the time of any activity on a critical path will always increase the completion time of a project.
Show Answers Only

Show Worked Solution

 

♦ Mean mark 40%.

 

NETWORKS, FUR2 2009 VCAA 4

A walkway is to be built across the lake.

Eleven activities must be completed for this building project.

The directed network below shows the activities and their completion times in weeks.
 

NETWORKS, FUR2 2009 VCAA 4
 

  1. What is the earliest start time for activity ?   (1 mark)

  2. Write down the critical path for this project.   (1 mark)

  3. The project supervisor correctly writes down the float time for each activity that can be delayed and makes a list of these times.

     

    Determine the longest float time, in weeks, on the supervisor’s list.   (1 mark)

A twelfth activity, , with duration three weeks, is to be added without altering the critical path.

Activity has an earliest start time of four weeks and a latest start time of five weeks.

 

NETWORKS, FUR2 2009 VCAA 4

  1. Draw in activity on the network diagram above.   (1 mark)

  2. Activity starts, but then takes four weeks longer than originally planned.

     

    Determine the total overall time, in weeks, for the completion of this building project.   (1 mark)

Show Answers Only

  4.  
    NETWORKS, FUR2 2009 VCAA 4 Answer
Show Worked Solution

a.  

♦ Mean mark of all parts (combined): 44%.
  

b.  
  

c.   


  

d.    NETWORKS, FUR2 2009 VCAA 4 Answer

  
e.  

NETWORKS, FUR2 2010 VCAA 4

In the final challenge, each of four teams has to complete a construction project that involves activities to .
 

NETWORKS, FUR2 2010 VCAA 4
 

Table 1 shows the earliest start time (EST), latest start time (LST) and duration, in minutes, for each activity. 

The immediate predecessor is also shown. The earliest start time for activity is missing.

  1. What is the least number of activities that must be completed before activity can commence?   (1 mark)

  2. What is the earliest start time for activity ?   (1 mark)

  3. Write down all the activities that must be completed before activity can commence.   (1 mark)

  4. What is the float time, in minutes, for activity ?   (1 mark)

  5. What is the shortest time, in minutes, in which this construction project can be completed?   (1 mark)

  6. Write down the critical path for this network.   (1 mark)

Show Answers Only
Show Worked Solution

a.  
  

b.   
   

  
c.   
  

d.   
   

 

e.  

 

f.  

NETWORKS, FUR2 2011 VCAA 3

A section of the Farnham showgrounds has flooded due to a broken water pipe. The public will be stopped from entering the flooded area until repairs are made and the area has been cleaned up.

The table below shows the nine activities that need to be completed in order to repair the water pipe. Also shown are some of the durations, Earliest Start Times (EST) and the immediate predecessors for the activities.
 

NETWORKS, FUR2 2011 VCAA 3 

  1. What is the duration of activity ?   (1 mark)

  2. What is the Earliest Start Time (EST) for activity ?   (1 mark)

  3. Once the water has been turned off (Activity ), which of the activities to could be delayed without affecting the shortest time to complete all activities?   (1 mark)

It is more complicated to replace the broken water pipe (Activity ) than expected. It will now take four hours to complete instead of two hours.

  1. Determine the shortest time in which activities to can now be completed.   (1 mark)

Turning on the water to the showgrounds (Activity ) will also take more time than originally expected. It will now take five hours to complete instead of one hour.

  1. With the increased duration of Activity and Activity , determine the shortest time in which activities to can be completed.   (1 mark)

Show Answers Only
Show Worked Solution
a.   
   
♦ Mean mark of all parts (combined) was 42%.

  
b.  

  
c.   

MARKER’S COMMENT: Many students incorrectly included in this answer (note that is not on the critical path).

  
d.  


  

e.  

NETWORKS, FUR2 2012 VCAA 2

Thirteen activities must be completed before the produce grown on a farm can be harvested. 

The directed network below shows these activities and their completion times in days.

 

NETWORKS, FUR2 2012 VCAA 2
  

  1. Determine the earliest starting time, in days, for activity .   (1 mark)

  2. A dummy activity starts at the end of activity .

     

    Explain why this dummy activity is used on the network diagram.   (1 mark)

  3. Determine the earliest starting time, in days, for activity .   (1 mark)

  4. In order, list the activities on the critical path.   (1 mark)

  5. Determine the latest starting time, in days, for activity .   (1 mark)

Show Answers Only





Show Worked Solution
a.   
   
♦ Mean mark of all parts (combined) 47%.

 

b.  

 

♦♦ Exact data unavailable but “few students” were able to correctly deal with the dummy activity in this question.
c.   
   

 

d.  

 

e.  

MARKER’S COMMENT: A correct calculation based on an incorrect critical path in part (d) gained a consequential mark here. Show your working!