SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Calculus, MET1 2024 VCAA 8

Let  ,  where    and  .

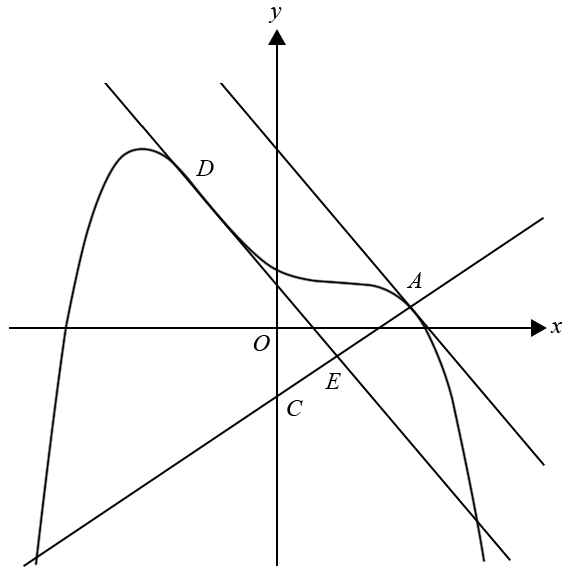
Let the point be the -intercept of the graph of  .

  1. Find the coordinates of , in terms of and .   (1 mark)

  2. Find the gradient of at , in terms of .   (2 marks)

  3. Given that the graph of    passes through the origin, express in terms of .   (1 mark)

  4. Let the point be a point different from the point , such that the gradient of at points and are equal.
  5. Given that the graph of    passes through the origin, find the coordinates of in terms of .   (3 marks)

Show Answers Only

a.    

b.    

c.   

d.   

Show Worked Solution

a.   


 

b.   

 
 
♦ Mean mark (b) 39%.
c.    
      
   
   
♦ Mean mark (c) 47%.
d.    
 
 
 
 
 
 
♦♦♦ Mean mark (d) 15%.

Calculus, MET2 2016 VCAA 2

Consider the function  

  1.  i. Given that  ,
  2.      show that  .   (1 mark)

  3. ii. Find the values of for which the graph of    has a stationary point.   (1 mark)

The diagram below shows part of the graph of  , the tangent to the graph at    and a straight line drawn perpendicular to the tangent to the graph at  . The equation of the tangent at the point with coordinates    is  .

The tangent cuts the -axis at . The line perpendicular to the tangent cuts the -axis at .
 


 

  1.   i. Find the coordinates of .   (1 mark)

  2.  ii. Find the equation of the line that passes through and and, hence, find the coordinates of .   (2 marks)

  3. iii. Find the area of triangle .   (2 marks)

  4. The tangent at is parallel to the tangent at . It intersects the line passing through and at .

     


     
     i. Find the coordinates of .   (2 marks)

  5. ii. Find the length of .   (3 marks)

Show Answers Only
  1.  i.
  2. ii.
  3.   i.
  4. ii.
  5.      
  6. iii. ²
Show Worked Solution
a.i.   
   
   
 

 

  

 

a.ii.   


 

b.i.   


 

b.ii.   

   

   


 

b.iii.  
   
    ²

 

♦ Mean mark part (c)(i) 43%.
MARKER’S COMMENT: Many students gave an incorrect -value here. Be careful!
c.i.   
 
 
   

  

 

c.ii.  

 

♦♦ Mean mark 32%.

 

 

 

Calculus, MET1 2015 VCAA 10

The diagram below shows a point, , on a circle. The circle has radius 2 and centre at the point  with coordinates . The angle is , where  .
  

met1-2015-vcaa-q10
  

The diagram also shows the tangent to the circle at . This tangent is perpendicular to and intersects the -axis at point and the -axis at point .

  1. Find the coordinates of in terms of .   (1 mark)

  2. Find the gradient of the tangent to the circle at in terms of .   (1 mark)

  3. The equation of the tangent to the circle at can be expressed as
  5.  i. Point , with coordinates , is on the line segment .
  6.     Find in terms of .   (1 mark)

  7. ii. Point , with coordinates , is on the line segment .
  8.     Find in terms of .   (1 mark)

  9. Consider the trapezium with parallel sides of length and .
  10. Find the value of for which the area of the trapezium is a minimum. Also find the minimum value of the area.   (3 marks)

Show Answers Only
  3.  i.
  4. ii.
  6. ²
Show Worked Solution
a.   
   
 
♦♦♦ Part (a) mean mark 20%.
MARKER’S COMMENT: Many students did not include the “+2” in the -coordinate.

 

 

♦♦♦ Part (b) mean mark 16%.
b.   
   
   
     
 

 

c.i.  

 

c.ii.   

♦ Part (c)(ii) mean mark 47%.
 

 

♦♦♦ Part (d) mean mark 19%.
d.   
   
   

 

 

 
 

 

²