Let
The shortest distance,
Aussie Maths & Science Teachers: Save your time with SmarterEd
Let
The shortest distance,
Straight line distance is the shortest.
Let
--- 5 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Let
--- 4 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Consider the function
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
The diagram below shows part of the graph of
The tangent cuts the
--- 2 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
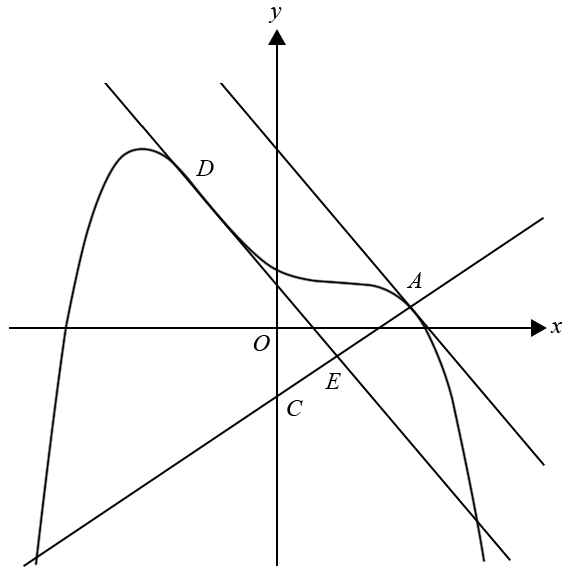
i. Find the coordinates of
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
Consider the graph of the function defined by
The square of the length of the line segment joining the points on the graph for which
| |
|