SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Networks, STD2 N3 2023 HSC 31

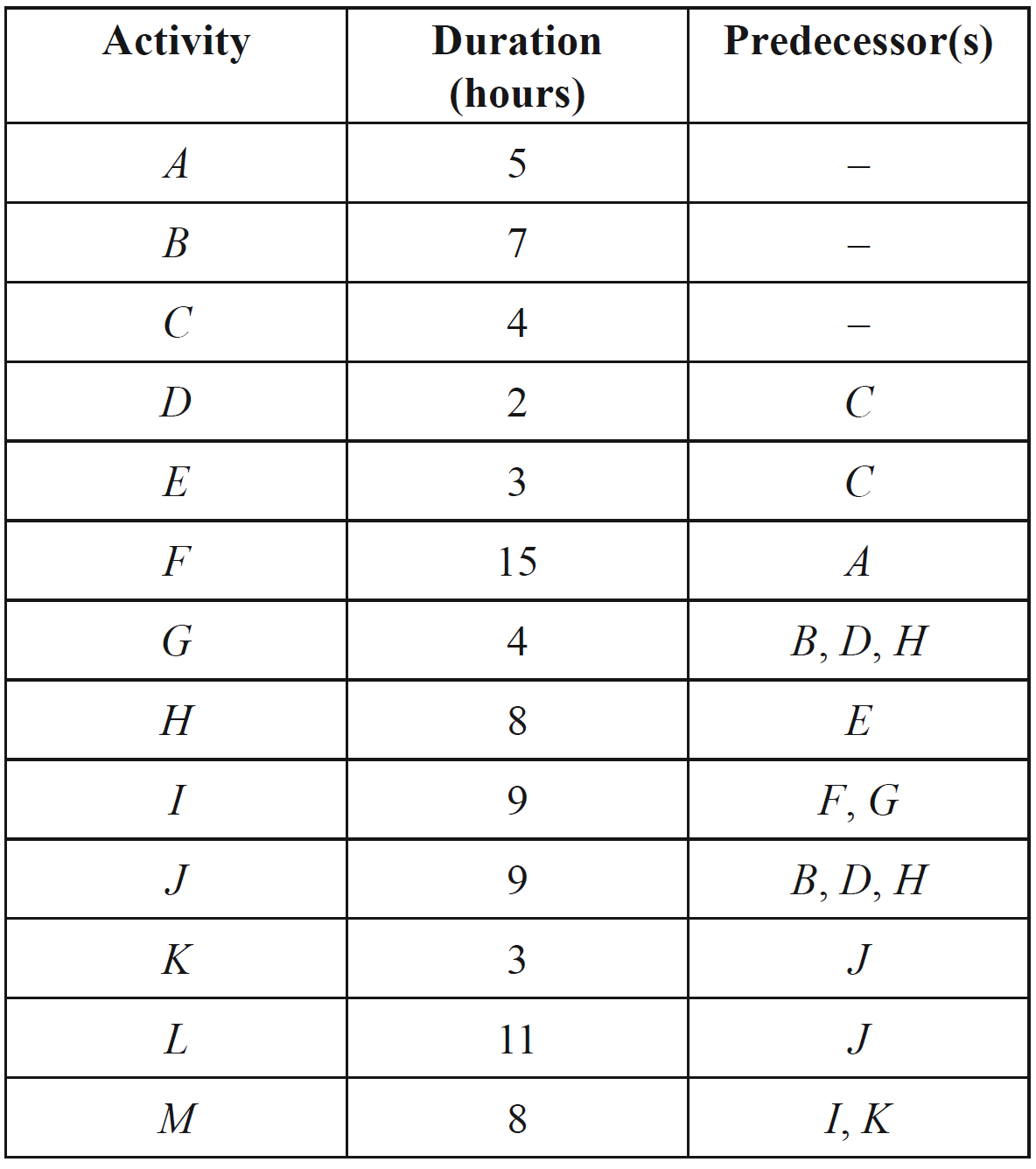
A function centre employs staff so that all necessary tasks can be completed between the end of one function and the beginning of the next function.

The network diagram shows the time taken in hours for the tasks that need to be completed.
 


 

  1. Find the TWO critical paths.  (2 marks)

  2. The function centre wants to decrease the length of each critical path by 3 hours. They can do this by hiring more staff to do ONE of the tasks so it takes less time to complete.
  3. For which task should the centre hire more staff, and how long should that task take to ensure all tasks can be completed in 14 hours?  (2 marks) 

Show Answers Only

a.   

b.   

Show Worked Solution

a.   
 

♦ Mean mark (a) 46%.

 
b.  

Mean mark (b) 52%.

Networks, STD2 N3 SM-Bank 50

Roadworks planned by the local council require 13 activities to be completed.

The network below shows these 13 activities and their completion times in weeks.
 

  1. What is the earliest start time, in weeks, of activity ?  (1 mark)

  2. How many of these activities have zero float time?  (2 marks)

  3. It is possible to reduce the completion time for activities and .
  4. The reduction in completion time for each of these five activities will incur an additional cost.
  5. The table below shows the five activities that can have their completion time reduced and the associated weekly cost, in dollars.
     
       
  6. The completion time for each these five activities can be reduced by a maximum of two weeks.
  7. The overall completion time for the roadworks can be reduced to 16 weeks.
  8. What is the minimum cost, in dollars, of this change in completion time?  (3 marks)

Show Answers Only
Show Worked Solution

a.    


 

b.    


 

c.   


  


  


 


 

Networks, STD2 N3 2021 FUR1 6

The directed graph below shows the sequence of activities required to complete a project.

The time taken to complete each activity, in hours, is also shown.
 

The minimum completion time for this project is 18 hours.

The time taken to complete activity is labelled  .

What is the maximum value of  ?   (2 marks)

Show Answers Only

Show Worked Solution


 

Networks, STD2 N3 2019 FUR2 3

Fencedale High School is planning to renovate its gymnasium.

This project involves 12 activities, to .

The directed network below shows these activities and their completion times, in weeks.
 


 

The minimum completion time for the project is 35 weeks.

  1. Identify the critical path and state how many activities are on it?  (2 marks)

  2. Determine the latest start time of activity .  (1 mark)

  3. Which activity has the longest float time?  (1 mark)

It is possible to reduce the completion time for activities and by employing more workers.

  1.  The completion time for each of these five activities can be reduced by a maximum of two weeks.

      

    What is the minimum time, in weeks, that the renovation project could take?  (1 mark)

Show Answers Only
Show Worked Solution

a. 
 

 

 


 

b. 
 

c.   


 

d.   


 

 

Networks, STD2 N3 2013 FUR2 2

A project will be undertaken in the wildlife park. This project involves the 13 activities shown in the table below. The duration, in hours, and predecessor(s) of each activity are also included in the table.


NETWORKS, FUR2 2013 VCAA 21
 

Activity is missing from the network diagram for this project, which is shown below.

 
NETWORKS, FUR2 2013 VCAA 22
 

  1. Complete the network diagram above by inserting activity .  (1 mark)
  2. Determine the earliest starting time of activity .  (1 mark)

  3. Given that activity  is not on the critical path

     

    1. write down the activities that are on the critical path in the order that they are completed  (1 mark)

    2. find the latest starting time for activity .  (1 mark)

  4. Consider the following statement.

     

    ‘If the time to complete just one of the activities in this project is reduced by one hour, then the minimum time to complete the entire project will be reduced by one hour.’

    Explain the circumstances under which this statement will be true for this project.  (1 mark)

  5. Assume activity  is reduced by two hours.
    What will be the minimum completion time for the project?  (1 mark)

Show Answers Only

a.

networks-fur2-2013-vcaa-2-answer

b. 

c.i.  

c.ii. 

d. 
     

e. 

Show Worked Solution
a.    networks-fur2-2013-vcaa-2-answer

 

b.  


 

c.i.   

♦♦ Mean mark of parts (c)-(e) (combined) was 40%.
 

c.ii.  

 
 

d.  

MARKER’S COMMENT: Most students struggled with part (d).


 

e.  

Networks, STD2 N3 2009 FUR2 4

A walkway is to be built across the lake.

Eleven activities must be completed for this building project.

The directed network below shows the activities and their completion times in weeks.
 

NETWORKS, FUR2 2009 VCAA 4
 

  1. What is the earliest start time for activity E?  (1 mark)

  2. Write down the critical path for this project.  (1 mark)

  3. The project supervisor correctly writes down the float time for each activity that can be delayed and makes a list of these times.

     

    Determine the longest float time, in weeks, on the supervisor’s list.  (1 mark)

A twelfth activity, L, with duration three weeks, is to be added without altering the critical path.

Activity L has an earliest start time of four weeks and a latest start time of five weeks.
 

NETWORKS, FUR2 2009 VCAA 4
 

  1. Draw in activity L on the network diagram above.  (1 mark)
  2. Activity L starts, but then takes four weeks longer than originally planned.

     

    Determine the total overall time, in weeks, for the completion of this building project.  (1 mark)

Show Answers Only

  4.  
    NETWORKS, FUR2 2009 VCAA 4 Answer
Show Worked Solution

a.  

♦ Mean mark of all parts (combined): 44%.

 

b. 
 

 

 

c.   


 

d.    NETWORKS, FUR2 2009 VCAA 4 Answer

 

e.  

Networks, STD2 N3 2014 FUR1 8 MC

Which one of the following statements about critical paths is true?

  1. There can be only one critical path in a project.
  2. A critical path will always include the activity that takes the longest time to complete.
  3. Reducing the time of any activity on a critical path for a project will always reduce the minimum completion time for the project.
  4. If there are no other changes, increasing the time of any activity on a critical path will always increase the completion time of a project.
Show Answers Only

Show Worked Solution

♦ Mean mark 40%.

 

Networks, STD2 N3 SM-Bank 47

The directed graph below shows the sequence of activities required to complete a project.

All times are in hours.
 


 

  1.  Find the number of activities that have exactly two immediate predecessors. (1 mark)

  2. Identify the critical path for this project. (3 marks)
  3. If Activity E is reduced by one hour, identify the two new critical paths. (1 mark)

Show Answers Only
Show Worked Solution

i.    

 

ii.   
 


 

 

iii.   

Networks, STD2 N3 2008 FUR1 8-9 MC

The network below shows the activities that are needed to finish a particular project and their completion times (in days).
 

networks-fur1-2008-vcaa-8-mc

 
Part 1

The earliest start time for Activity K, in days, is

A.     

B.   

C.   

D.   

 

Part 2

This project currently has one critical path.

A second critical path, in addition to the first, would be created by

A.   increasing the completion time of D by 7 days.

B.   increasing the completion time of G by 1 day.

C.   increasing the completion time of I by 2 days.

D.   decreasing the completion time of C by 1 day.

Show Answers Only

Show Worked Solution


 

 

 

♦♦ Mean mark of Part 2 was 35%.