SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Algebra, STD2 A4 2007 HSC 27a

   A rectangular playing surface is to be constructed so that the length is 6 metres more than the width.

  1. Give an example of a length and width that would be possible for this playing surface.   (1 mark)

  2. Write an equation for the area (`A`) of the playing surface in terms of its length (`l`).   (1 mark)

     

    A graph comparing the area of the playing surface to its length is shown.
     
       
     

  3. Why are lengths of 0 metres to 6 metres impossible?   (1 mark)

  4. What would be the dimensions of the playing surface if it had an area of 135 m²?  (2 marks)

     

    Company `A` constructs playing surfaces.

         

  5. Draw a graph to represent the cost of using Company `A` to construct all playing surface sizes up to and including 200 m².

     

    Use the horizontal axis to represent the area and the vertical axis to represent the cost.   (2 marks)

  6. Company `B` charges a rate of $360 per square metre regardless of size. 
  7. Which company would charge less to construct a playing surface with an area of 135 m²

     

    Justify your answer with suitable calculations.   (1 mark)

Show Answers Only
  1. `text(One possibility is a length of 10 m, and a width)`

     

    `text{of 4 m (among many possibilities).}`

  2. `A =  l (l – 6)\ \ text(m²)`
  3. `text(Given the length must be 6 m more than)`

     

    `text(the width, it follows that the length)`

     

    `text(must be greater than 6 m.)`

  4. `text(15 m × 9 m)`
  5.   
       
     
  6. `text(Proof)\ \ text{(See worked solutions)}`
Show Worked Solution

i.   `text(One possibility is a length of 10 m, and a width)`

`text{of 4 m (among many possibilities).}`

 

ii.  `text(Length) = l\ text(m)`

`text(Width) = (l – 6)\ text(m)`

`:.\ A` `= l (l – 6)`

 

iii.  `text(Given the length must be 6m more than the width,)`

 `text(it follows that the length must be greater than 6 m)`

`text(so that the width is positive.)`

 

iv.  `text(From the graph, an area of 135 m² corresponds to)`

`text(a length of 15 m.)`

`:.\ text(The dimensions would be 15 m × 9 m.)`

 

v.   

  

vi.  `text(Company)\ A\ text(cost) = $50\ 000`

`text(Company)\ B\ text(cost)` `= 135 xx 360`
  `= $48\ 600`

 

`:.\ text(Company)\ B\ text(would charge $1400 less)`

`text(than Company)\ A.`

Algebra, STD2 A4 2014 HSC 29a

The cost of hiring an open space for a music festival is  $120 000. The cost will be shared equally by the people attending the festival, so that  `C`  (in dollars) is the cost per person when  `n`  people attend the festival.

  1. Complete the table below by filling in the THREE missing values.   (1 mark)
    \begin{array} {|l|c|c|c|c|c|c|}
    \hline
    \rule{0pt}{2.5ex}\text{Number of people} (n) \rule[-1ex]{0pt}{0pt} & \ 500\ & \ 1000 \ & 1500 \ & 2000 \ & 2500\ & 3000 \ \\
    \hline
    \rule{0pt}{2.5ex}\text{Cost per person} (C)\rule[-1ex]{0pt}{0pt} &  &  &  & 60 & 48\ & 40 \ \\
    \hline
    \end{array}
  2. Using the values from the table, draw the graph showing the relationship between  `n`  and  `C`.   (2 marks)
     
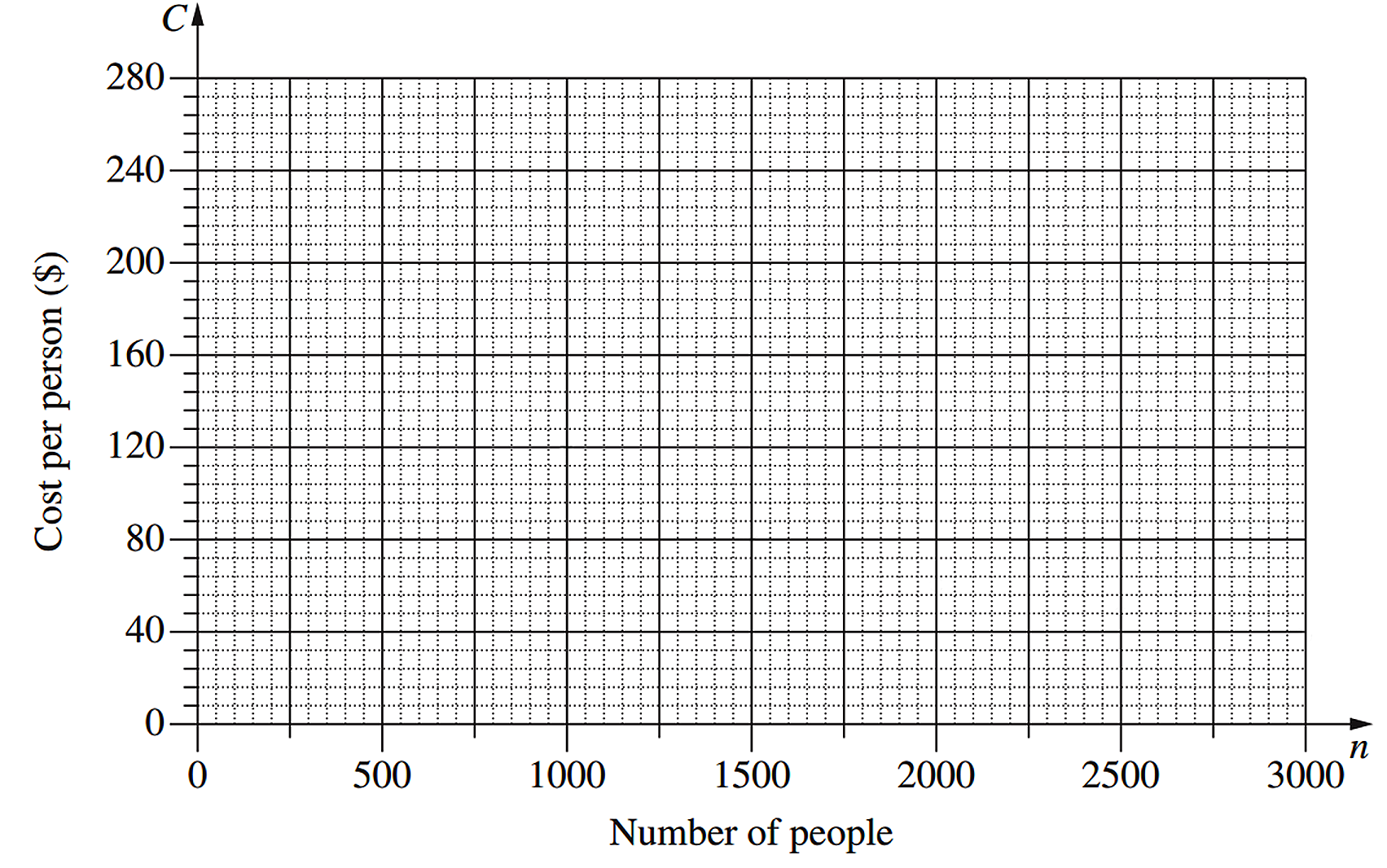
  3. What equation represents the relationship between `n` and `C`?   (1 mark)

  4. Give ONE limitation of this equation in relation to this context.   (1 mark)

  5. Is it possible for the cost per person to be $94? Support your answer with appropriate calculations.   (1 mark)

Show Answers Only

i.   

\begin{array} {|l|c|c|c|c|c|c|}
\hline
\rule{0pt}{2.5ex}\text{Number of people} (n) \rule[-1ex]{0pt}{0pt} & \ 500\ & \ 1000 \ & 1500 \ & 2000 \ & 2500\ & 3000 \ \\
\hline
\rule{0pt}{2.5ex}\text{Cost per person} (C)\rule[-1ex]{0pt}{0pt} & 240 & 120 & 80 & 60 & 48\ & 40 \ \\
\hline
\end{array}
 

ii. 

iii.   `C = (120\ 000)/n`

`n\ text(must be a whole number)`
 

iv.   `text(Limitations can include:)`

  `•\ n\ text(must be a whole number)`

  `•\ C > 0`
 

v.   `text(If)\ C = 94:`

`94` `= (120\ 000)/n`
`94n` `= 120\ 000`
`n` `= (120\ 000)/94`
  `= 1276.595…`

 
`:.\ text(C)text(ost cannot be $94 per person,)`

`text(because)\ n\ text(isn’t a whole number.)`

Show Worked Solution

i.   

\begin{array} {|l|c|c|c|c|c|c|}
\hline
\rule{0pt}{2.5ex}\text{Number of people} (n) \rule[-1ex]{0pt}{0pt} & \ 500\ & \ 1000 \ & 1500 \ & 2000 \ & 2500\ & 3000 \ \\
\hline
\rule{0pt}{2.5ex}\text{Cost per person} (C)\rule[-1ex]{0pt}{0pt} & 240 & 120 & 80 & 60 & 48\ & 40 \ \\
\hline
\end{array}
 

ii. 

 

♦ Mean mark (iii) 48%

iii.   `C = (120\ 000)/n`

 

♦♦♦ Mean mark (iv) 7%
COMMENT: When asked for limitations of an equation, look carefully at potential restrictions with respect to both the domain and range.

iv.   `text(Limitations can include:)`

  `•\ n\ text(must be a whole number)`

  `•\ C > 0`

 

v.   `text(If)\ C = 94`

`=> 94` `= (120\ 000)/n`
`94n` `= 120\ 000`
`n` `= (120\ 000)/94`
  `= 1276.595…`
♦ Mean mark (v) 38%

 

`:.\ text(C)text(ost cannot be $94 per person,)`

`text(because)\ n\ text(isn’t a whole number.)`