SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Statistics, STD2 S5 2024 HSC 35

A random variable is normally distributed with mean 0 and standard deviation 1. The table gives the probability that this random variable is less than \(z\).

\begin{array} {|c|c|c|c|c|c|c|c|c|c|}
\hline
\rule{0pt}{2.5ex} z \rule[-1ex]{0pt}{0pt} & 0.6 & 0.7 & 0.8 & 0.9 & 1.0 & 1.1 & 1.2 & 1.3 & 1.4 \\
\hline
\rule{0pt}{2.5ex} \textit{Probability} \rule[-1ex]{0pt}{0pt} & 0.7257 & 0.7580 & 0.7881 & 0.8159 & 0.8413 & 0.8643 & 0.8849 & 0.9032 & 0.9192 \\
\hline
\end{array}

The probability values given in the table for different values of \(z\) are represented by the shaded area in the following diagram.
 

The scores in a university examination with a large number of candidates are normally distributed with mean 58 and standard deviation 15.

  1. By calculating a \(z\)-score, find the percentage of scores that are between 58 and 70.   (2 marks)
  2. Explain why the percentage of scores between 46 and 70 is twice your answer to part (a).   (1 mark)
  3. By using the values in the table above, find an approximate minimum score that a candidate would need to be placed in the top 10% of the candidates.   (2 marks)
Show Answers Only

a.   \(28.81%\)

b.   \(\text{Normal distribution is symmetrical about the mean (58).}\)

\(\text{Since 70 and 46 are both the same distance (12) from the mean, the percentage}\)

\(\text{of scores in this range will be twice the answer in part (a).}\)

c.   \(\text{Approx minimum score = 78%}\)

Show Worked Solution

a.   \(z\text{-score (58)}\ =\dfrac{x-\mu}{\sigma} = \dfrac{58-58}{15}=0\)

\(z\text{-score (70)}\ = \dfrac{70-58}{15}=0.8\)

\(\text{Using table:}\)

\(\text{% between 58–70}\ =0.7881-0.5=0.2881=28.81%\)
 

b.   \(\text{Normal distribution is symmetrical about the mean (58).}\)

\(\text{Since 70 and 46 are both the same distance (12) from the mean, the percentage}\)

\(\text{of scores in this range will be twice the answer in part (a).}\)
 

c.   \(z\text{-score 1.3 has a table value 0.9032}\)

\(1-0.9032=0.0968\ \Rightarrow\ \text{i.e. 9.68% of students score higher.}\)

\(\text{Find}\ x\ \text{for a}\ z\text{-score of 1.3:}\)

\(1.3\) \(=\dfrac{x-58}{15}\)  
\(x\) \(=1.3 \times 15 +58\)   
  \(=77.5\)  

 
\(\therefore\ \text{Approx minimum score = 78%}\)

Statistics, STD2 S5 2023 HSC 38

A random variable is normally distributed with a mean of 0 and a standard deviation of 1 . The table gives the probability that this random variable lies below `z` for some positive values of `z`.

The probability values given in the table are represented by the shaded area in the following diagram.
 

The weights of adult male koalas form a normal distribution with mean `mu` = 10.40 kg, and standard deviation `sigma` = 1.15 kg.

In a group of 400 adult male koalas, how many would be expected to weigh more than 11.93 kg?  (4 marks)

Show Answers Only

`37\ text{koalas*}`

`text{*36 or 36.72 koalas would also receive full marks}`

Show Worked Solution
`ztext{-score (11.93)}` `=(x-mu)/sigma`  
  `=(11.93-10.4)/1.15`  
  `=1.330`  

 
`Ptext{(Koala weighs > 11.93 kg)}\ = P(z>1.330)`

`text{Using the table:}`

`P(z>1.33)` `=1-0.9082`  
  `=0.0918`  
♦ Mean mark 39%.
`:.\ text{Expected koalas > 11.93 kg}` `=0.0918 xx 400`  
  `=36.72`  
  `=37\ text{koalas*}`  

 
`text{*36 or 36.72 koalas would also receive full marks}`

Statistics, STD2 S5 2022 HSC 18

The marks in a test were normally distributed. The mean mark was 60 and the standard deviation was 15 .

What was the percentage of marks higher than 90?  (2 marks)

Show Answers Only

`2.5text{%}`

Show Worked Solution
`ztext{-score}` `=(x-mu)/sigma`  
  `=(90-60)/15`  
  `=2`  

 

`text{% marks > 90}\ = 2.5text{%}`


Mean mark 52%.
COMMENT: A poor State result that warrants attention.

Statistics, STD2 S5 SM-Bank 7

800 participants auditioned for a stage musical. Each participant was required to complete a series of ability tests for which they received an overall score.

The overall scores were approximately normally distributed with a mean score of 69.5 points and a standard deviation of 6.5 points.

Only the participants who scored at least 76.0 points in the audition were considered successful.

How many of the participants were considered unsuccessful?   (2 marks)

Show Answers Only

`672`

Show Worked Solution

`mu = 69.5 \ , \ sigma= 6.5`

`ztext{-score} \ (76) = {76 – 69.5}/6.5 = 1`

`:.\  text{Unsuccessful}` `=84text(%) xx 800`
  `= 672`

Statistics, STD2 S5 2021 HSC 38

A random variable is normally distributed with mean 0 and standard deviation 1. The table gives the probability that this random variable lies between 0 and `z` for different values of `z`.

 

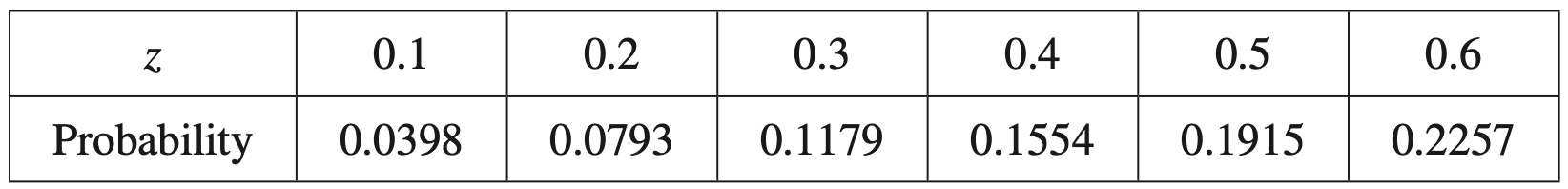
The probability values given in the table for different values of `z` are represented by the shaded area in the following diagram.
 

  1. Using the table, show that the probability that a value from a random variable that is normally distributed with mean 0 and standard deviation 1 is greater than 0.3 is equal to 0.3821.  (1 mark)

  2. Birth weights are normally distributed with a mean of 3300 grams and a standard deviation of 570 grams. By first calculating a `z`-score, find how many babies, out of 1000 born, are expected to have a birth weight greater than 3471 grams.  (3 marks)

Show Answers Only
  1. `text(See Worked Solutions)`
  2. `382`
Show Worked Solution

a.   `P(z>0)=0.5`

♦♦♦ Mean mark part (a) 1%.
COMMENT: Note the Std2 and Advanced questions varied slightly but used the same table and graph.

`P(0<z<0.3)=0.1179`

`P(z>0.3) = 0.5-0.1179=0.3821`
 

b.   `z text{-score (3471)}` `=(x-mu)/sigma`
    `=(3471-3300)/570`
    `=0.3`

 
`P(z>0.3) = 0.3821\ \ text{(see part (a))}`
  

`:.\ text(Number of babies > 3471 grams)`  
`=1000 xx 0.3821`  
`=382\ \ text{(nearest whole)}`  

Statistics, STD2 S5 2021 HSC 8 MC

On a test, Zac's mark corresponded to a `z`-score of 2. The test scores had a mean of 63 and a standard deviation of 8.

What was Zac's actual mark on the test?

  1. 65
  2. 67
  3. 73
  4. 79
Show Answers Only

`D`

Show Worked Solution

`text{Method 1 (quicker)}`

`text(Actual mark)` `= mu + 2 xx sigma`
  `= 63 + 2 xx 8`
  `= 79`

 

`text(Method 2)`

`ztext(-score)` `= (x – mu)/sigma`
`2` `= (x-63)/8`
`16` `=x-63`
`x` `=79`

 
`=>D`

Statistics, STD2 S5 2020 HSC 35

The intelligence Quotient (IQ) scores for adults in City A are normally distributed with a mean of 108 and a standard deviation of 10.

The IQ score for adults in City B are normally distributed with a mean of 112 and a standard deviation of 16.

  1. Yin is an adult who lives in City A and has an IQ score of 128.
    What percentage of the adults in this city have an IQ score higher than Yin's?   (2 marks) 

  2. There are 1 000 000 adults living in City B.
    Calculate the number of adults in City B that would be expected to have an IQ score lower than Yin's.  (2 marks)

  3. Simon, an adult who lives in City A, moves to City B. The  `z` -score corresponding to his IQ score in City A is the same as the `z`-score corresponding to his IQ score in City B.
    By first forming an equation, calculate Simon's IQ score. Give your answer correct to one decimal place.   (3 marks)

Show Answers Only
  1. `text(2.5%)`
  2. `840 \ 000`
  3. `101.3`
Show Worked Solution

a.    `text{In City A:}`

♦ Mean mark 50%.

`z text{-score}\ (128) = frac {x -mu}{sigma} = frac{128 – 108}{10} = 2`
 


 

`therefore\ text{2.5% have a higher IQ in City} \ A.`
 

b.    `text{In City B:}`

♦ Mean mark 44%.

`z text{-score}\ (128) = frac{128 – 112}{16} = 1`
 


 

`therefore \ text{Adults in City} \ B \ text{with an IQ}\ <  128`

`= 84text(%) xx 1 \ 000 \ 000`

`= 840 \ 000`
 

c.    `z text{-score in City A}\ = z text{-score in City B}`

♦♦♦ Mean mark 17%.
`frac{x – 108}{10} ` `= frac{x -112}{16} \ \ text{(multiply b.s.} \ xx 160 text{)}`
`16 (x – 108)` `= 10 (x – 112)`
`16 x – 1728` `= 10 x – 1120`
`6x` `= 608`
`x` `= 101.3`

  
`therefore \ text{Simon’s IQ} = 101.3 \ text{(to 1 d.p.)}`

Statistics, STD2 S5 2019 HSC 38

In a particular country, the birth weight of babies is normally distributed with a mean of 3000 grams. It is known that 95% of these babies have a birth weight between 1600 grams and 4400 grams.

One of these babies has a birth weight of 3497 grams. What is the `z`-score of this baby's birth weight?  (2 marks)

Show Answers Only

`0.71`

Show Worked Solution

`text(95% babies within)\ 1600 – 4400`

♦ Mean mark 44%.

`text(i.e.)\ \ 3000 +- \ 2\ text(σ = 1600 – 4400)`

`2sigma` `= 4400-3000`
  `= 1400`
`sigma` `= 700`
`:. ztext(-score)\ (3497)` `= (x-mu)/σ`
  `= (3497 – 3000)/700`
  `= 0.71`

Statistics, STD2 S5 SM-Bank 5

The diastolic measurement for blood pressure in 30-year-old people is normally distributed, with a mean of 82 and standard deviation of 16.

  1.   A person is considered to have low blood pressure if the diastolic measurement is 66 or less.

     

      What percentage of 30-year-old people have low blood pressure?  (1 mark)

  2.   Calculate the `z`-score for a diastolic measurement of 70.  (1 mark)

Show Answers Only
  1. `16text(%)`
  2. `−0.75`
Show Worked Solution
a.    `ztext(-score)\ (66)` `= (66 – 82)/16`
    `= −1`

 

`:.\ text(Percentage)` `= 100 – (50+34)`
  `= 16text(%)`

 

b.    `ztext(-score)\ (70)` `= (70 – 82)/16`
    `= −0.75`

Statistics, STD2 S5 2018 HSC 27e

Joanna sits a Physics test and a Biology test.

  1. Joanna’s mark in the Physics test is 70. The mean mark for this test is 58 and the standard deviation is 8.

     

    Calculate the `z`-score for Joanna’s mark in this test.  (1 mark)

  2. In the Biology test, the mean mark is 64 and the standard deviation is 10.

     

    Joanna’s `z`-score is the same in both the Physics test and the Biology test.

     

    What is her mark in the Biology test?  (2 marks)

Show Answers Only
  1. `1.5`
  2. `79`
Show Worked Solution

i.   `x = 70, \ mu = 58, \ sigma = 8`

`:. ztext(-score)` `= (x – mu)/sigma`
  `= (70 – 58)/8`
  `= 1.5`

 

ii.    `1.5` `= (x – 64)/10`
  `x – 64` `= 15`
  `:. x` `= 79`

Statistics, STD2 S5 2017 HSC 13 MC

The heights of Year 12 girls are normally distributed with a mean of 165 cm and a standard deviation of 5.5 cm.

What is the `z`-score for a height of 154 cm?

A.     `−2`

B.    `−0.5`

C.     `0.5`

D.     `2`

Show Answers Only

`text(A)`

Show Worked Solution
`ztext(-score)` `= (x – mu)/sigma`
  `= (154 – 165)/5.5`
  `= −2`

 
`=>A`

Statistics, STD2 S5 2016 HSC 13 MC

The speed limit outside a school is 40 km/h. Year 11 students measured the speed of passing vehicles over a period of time. They found the set of data to be normally distributed with a mean speed of 36 km/h and a standard deviation of 2 km/h.

What percentage of the vehicles passed the school at a speed greater than 40 km/h?

  1. `text(2.5%)`
  2. `text(5%)`
  3. `text(47.5%)`
  4. `text(95%)`
Show Answers Only

`=> A`

Show Worked Solution
`z` `= (x – mu)/sigma`
  `= (40 – 36)/2`
  `= 2`

2ug-2016-hsc-13-mc-answer

`=> A`

Statistics, STD2 S5 SM-Bank 1 MC

The head circumference (in cm) of a population of infant boys is normally distributed with a mean of 49.5 cm and a standard deviation of 1.5 cm.

Four hundred of these boys are selected at random and each boy’s head circumference is measured.

The number of these boys with a head circumference of less than 48.0 cm is closest to 

  1. `3`
  2. `10`
  3. `64`
  4. `272`
Show Answers Only

`C`

Show Worked Solution

`mu=49.5,\ \ sigma=1.5`

`z text{-score (49.5)` `=(x-mu)/sigma`
  `=(48.0-49.5)/1.5`
  `=– 1`

 

`:.\ text(Number of boys with a head under 48.0 cm)`

`=16text(%) xx 400`

`=64`

`=>  C`

Statistics, STD2 S5 2015 HSC 28b

The results of two tests are normally distributed. The mean and standard deviation for each test are displayed in the table.
 

2015 28b

 
Kristoff scored 74 in Mathematics and 80 in English. He claims that he has performed better in English.

Is Kristoff correct? Justify your answer using appropriate calculations.  (2 marks)

Show Answers Only

`text(He is correct.)`

Show Worked Solution

`text(In Maths)`

♦ Mean mark 44%.
`ztext{-score(74)}` `= (x − mu)/sigma`
  `= (74 − 70)/6.5`
  `= 0.6153…`

 
`text(In English)`

`ztext{-score(80)}` `= (80 − 75)/8`
  `= 0.625`

 
`=>\ text(Kristoff’s)\ ztext(-score in English is higher than)`

`text(his)\ z text(-score in Maths.)`

`:.\ text(He is correct. He performed better in English.)`

Statistics, STD2 S5 2015 HSC 20 MC

A machine produces cylindrical pipes. The mean of the diameters of the pipes is 8 cm and the standard deviation is 0.04 cm.

Assuming a normal distribution, what percentage of cylindrical pipes produced will have a diameter less than 7.96 cm?

  1. `text(16%)`
  2. `text(32%)`
  3. `text(34%)`
  4. `text(68%)`
Show Answers Only

`A`

Show Worked Solution

`mu = 8\ text(cm)\ \ \ s = 0.04\ text(cm)`

♦ Mean mark 50%.
`ztext{-score(7.96)}` `= (x − mu)/sigma`
  `= (7.96 − 8)/0.04`
  `= −1`

 

2UG 2015 20MC Answer

`:.\ text(% of pipes with a diameter less than 7.96 cm.)`

`=\ text(50%) – text(34%)`

`=\ text(16%)`

`⇒ A`

Statistics, STD2 S5 2005 HSC 26c

The weights of boxes of Brekky Bicks are normally distributed. The mean is 754 grams and the standard deviation is 2 grams.

  1. What is the `z`-score of a box of Brekky Bicks with a weight of 754 g?   (1 mark)

  2. What is the weight of a box that has a `z`-score of  –1?   (1 mark)

  3. Brekky Bicks boxes are labelled as having a weight of 750 g. What percentage of boxes will have a weight less than 750 g?   (2 marks)

Show Answers Only
  1. `0\ text{(mean)}`
  2.  `752\ text(grams)`
  3. `text(2.5%)`
Show Worked Solution

i.    `text{z-score (754 g) = 0}`

`text{(Noting that 754 g is the mean)}`

 

ii.   `ztext(-score)` `= (x − mu)/sigma`
`-1` `= (x − 754)/2`
 `x – 754` `= -2`
`x`  `= 752\ text(grams)`

 

iii. `text{z-score (750)}` `= (750 − 754)/2`
    `= -2`

 

 
  `:.\ text(Graph shows that 2.5% of boxes will weigh)`

`text(less than 750 g.)`

Statistics, STD2 S5 SM-Bank 4 MC

The length of a type of ant is approximately normally distributed with a mean of 4.8 mm and a standard deviation of 1.2 mm.

A standardised ant length of  `z\ text(= −0.5)`  corresponds to an actual ant length of

A.   ` text(2.4 mm)`

B.   `text(3.6 mm)`

C.   `text(4.2 mm)`

D.   `text(5.4 mm)`

Show Answers Only

`C`

Show Worked Solution
`z` `= \ \ (x – mu)/sigma`
`-0.5` `= \ \ (x – 4.8)/1.2`
`-0.6` `= \ \ x – 4.8`
`x` `= \ \ 4.2\ text(mm)`

 
`=>C`

 

Statistics, STD2 S5 SM-Bank 3 MC

The time, in hours, that each student spent sleeping on a school night was recorded for `1550` secondary-school students. The distribution of these times was found to be approximately normal with a mean of 7.4 hours and a standard deviation of 0.7 hours.

How many students would you expect to spend more than 8.1 hours sleeping on a school night?

You may assume for normally distributed data that:

    • `text(68%)` of scores have  `z`-scores between  `–1` and `1`
    • `text(95%)` of scores have  `z`-scores between  `–2` and `2`
    • `text(99.7%)` of scores have  `z`-scores between  `–3` and `3`.

A.  `16`

B.  `248`

C.  `1302`

D.  `1510`

Show Answers Only

`B`

Show Worked Solution

`text (Need to find z-score of 8.1 hours)`

`text(z-score)` `= (x-mu)/sigma` 
  `= (8.1-7.4)/0.7`
  `= 1`

 

`text(68% students with  –1 < z-score < 1)`

`:.\ text(16% have  z-score > 1)`

`text(# Students)` `= 16%×1550`
  `= 248`

`=>B`

Algebra, STD2 A2 2007 HSC 27b

A clubhouse uses four long-life light globes for five hours every night of the year. The purchase price of each light globe is $6.00 and they each cost  `$d`  per hour to run.

  1. Write an equation for the total cost (`$c`) of purchasing and running these four light globes for one year in terms of  `d`.    (2 marks)

  2. Find the value of  `d`  (correct to three decimal places) if the total cost of running these four light globes for one year is $250.   (1 mark)

  3. If the use of the light globes increases to ten hours per night every night of the year, does the total cost double? Justify your answer with appropriate calculations.   (1 mark)

  4. The manufacturer’s specifications state that the expected life of the light globes is normally distributed with a standard deviation of 170 hours.

     

    What is the mean life, in hours, of these light globes if 97.5% will last up to 5000 hours?   (1 mark)

Show Answers Only
  1. `$c = 24 + 7300d`
  2. `0.031\ $ text(/hr)\ text{(3 d.p.)}`
  3. `text(Proof)\ \ text{(See Worked Solutions)}`
  4. `text(4660 hours.)`
Show Worked Solution

i.  `text(Purchase price) = 4 xx 6 = $24`

`text(Running cost)` `= text(# Hours) xx text(Cost per hour)`
  `= 4 xx 5 xx 365 xx d`
  `= 7300d`
 
`:.\ $c = 24 + 7300d`

 

ii.  `text(Given)\ \ $c = $250`

`250` `= 24 + 7300d`
`7300d` `= 226`
`d` `= 226/7300`
  `= 0.03095…`
  `= 0.031\ $ text(/hr)\ text{(3 d.p.)}`

 

iii.  `text(If)\ d\ text(doubles to 0.062)\ \ $text(/hr)`

`$c` `= 24 + 7300 xx 0.062`
  `= $476.60`
   
`text(S) text(ince $476.60 is less than)\ 2 xx $250\ ($500),`
`text(the total cost increases to less than double)`
`text(the original cost.)`

 

iv.  `sigma = 170`

`z\ text(-score of 5000 hours) = 2`

`z` `= (x – mu)/sigma`
`2` `= (5000 – mu)/170`
`340` `= 5000 – mu`
`mu` `= 4660`

 

`:.\ text(The mean life of these globes is 4660 hours.)`

Statistics, STD2 S5 2007 HSC 25d

The results of two class tests are normally distributed. The means and standard deviations of the tests are displayed in the table.
 

 

  1. Stuart scored 63 in Test 1 and 62 in Test 2. He thinks that he has performed better in Test 1. Do you agree? Justify your answer using appropriate calculations.   (2 marks)

  2. If 150 students sat for Test 2, how many students would you expect to have scored less than 64?   (2 marks)

Show Answers Only
  1. `text(Proof)\ \ text{(See Worked Solutions)}`
  2. `126`
Show Worked Solution

i.  `text(In Test 1,)\ \ mu = 60,\ sigma = 6.2`

`z text(-score)\ (63)` `= (x – mu)/sigma`
  `= (63 – 60)/6.2`
  `= 0.483…`

 
`text(In Test 2,)\ \ mu = 58,\ sigma = 6.0`

`z text(-score)\ (62)` `= (62 – 58)/6.0`
  `= 0.666…`
 

`text(S) text(ince Stuart’s)\ z\ text(-score is higher in Test 2,)`

`text(his performance relative to the class is better)`

`text(despite his mark being slightly lower.)`

 

ii.  `text(In Test 2)`

`z text(-score)\ (64)` `= (64 – 58)/6`
  `= 1`

`=> text(84% have)\ z text(-score) < 1`

`:.\ text(# Students expected below 64)`

`= text(84%) xx 150`

`= 126`

Statistics, STD2 S5 2008 HSC 28a

The following graph indicates  `z`-scores of ‘height-for-age’ for girls aged  5 – 19 years.
 

 
 

  1. What is the  `z`-score for a six year old girl of height 120 cm? (1 mark)

  2. Rachel is 10 ½  years of age. 

     

    (1)  If  2.5% of girls of the same age are taller than Rachel, how tall is she?   (1 mark)

     

    (2)  Rachel does not grow any taller. At age 15 ½, what percentage of girls of the same age will be taller than Rachel?   (2 marks)

  3. What is the average height of an 18 year old girl?   (1 mark)

For adults (18 years and older), the Body Mass Index is given by

`B = m/h^2`  where  `m = text(mass)`  in kilograms and  `h = text(height)`  in metres.

The medically accepted healthy range for  `B`  is  `21 <= B <= 25`.

  1. What is the minimum weight for an 18 year old girl of average height to be considered healthy? (2 marks)

  2. The average height, `C`, in centimetres, of a girl between the ages of 6 years and 11 years can be represented by a line with equation
     
            `C = 6A + 79`   where `A` is the age in years. 
     
    (1)  For this line, the gradient is 6. What does this indicate about the heights of girls aged 6 to 11?   (1 mark)

     

    (2)  Give ONE reason why this equation is not suitable for predicting heights of girls older than 12.   (1 mark)

Show Answers Only
  1. `1`
  2. (1) `155\ text(cm)`

     

    (2) `text(84%)`

  3. `163\ text(cm)`
  4. `55.8\ text(kg)`
  5. (1) `text(It indicates that 6-11 year old girls)`

     

          `text(grow, on average, 6cm per year)`

     

    (2) `text(Girls eventually stop growing, and the)`

     

          `text(equation doesn’t factor this in.)`

Show Worked Solution
i.    `z text(-score) = 1`

 

ii. (1)   `text(If 2 ½ % are taller than Rachel)`
    `=> z text(-score of +2)`
    `:.\ text(She is 155 cm)`
     
   (2)   `text(At age)\ 15\ ½,\ 155\ text(cm has a)\ z text(-score of –1)`
    `text(68% between)\ z = 1\ text(and)\ –1`
    `=> text(34% between)\ z = 0\ text(and)\ –1`
    `text(50% have)\ z >= 0`
     
    `:.\ text(% Above)\ z text(-score of –1)`
    `= 50 + 34`
    `= 8text(4%)`

 
`:.\ text(84% of girls would be taller than Rachel at age)\ 15 ½.`

 

iii.   `text(Average height of 18 year old has)\ z text(-score = 0)`
  `:.\ text(Average height) = 163\ text(cm)`

 

iv.   `B = m/h^2`
  `h = 163\ text(cm) = 1.63\ text(m)`

 

`text(Given)\ \ 21 <= B <= 25,\ text(minimum healthy)`

`text(weight occurs when)\ B = 21`

`=> 21` `= m/1.63^2`
`m` `= 21 xx 1.63^2`
  `= 55.794…`
  `= 55.8\ text(kg)\ text{(1 d.p.)}`

 

v. (1)   `text(It indicates that 6-11 year old girls, on average, grow)`
    `text(6 cm per year.)`
  (2) `text(Girls eventually stop growing, and the equation doesn’t)`
    `text(factor this in.)`

Statistics, STD2 S5 2011 HSC 27c

Two brands of light bulbs are being compared. For each brand, the life of the light bulbs is normally distributed.

2011 27c

  1. One of the Brand B light bulbs has a life of 400 hours. 

     

    What is the  `z`-score of the life of this light bulb?  (1 mark)

  2. A light bulb is considered defective if it lasts less than 400 hours. The following claim is made:

     

    ‘Brand A light bulbs are more likely to be defective than Brand B light bulbs.’

     

    Is this claim correct? Justify your answer, with reference to  `z`-scores or standard deviations or the normal distribution.   (2 marks)

Show Answers Only
  1. `-2`
  2. `text(The claim is incorrect.)`
Show Worked Solution

i.    `z text{-score of Brand B bulb (400 hrs)}`

`= (x-mu)/sigma`
`= (400\-500)/50`
`= –2`

 

ii.   `z text{-score of Brand A bulb (400 hours)}`

♦ Mean mark 42%
MARKER’S COMMENT: A number of students found drawing normal curves in their solution advantageous.
`=(400-450)/25`
`=–2`

 
`text(S)text(ince the)\ z text(-score for both brands is –2,)`

`text(they are equally likely to be defective.)`

`:.\ text(The claim is incorrect.)`

Statistics, STD2 S5 2009 HSC 25d

In Broken Hill, the maximum temperature for each day has been recorded. The mean of these maximum temperatures during spring is 25.8°C, and their standard deviation is 4.2° C. 

  1. What temperature has a `z`-score of  –1?    (1 mark)

  2. What percentage of spring days in Broken Hill would have maximum temperatures between 21.6° C and 38.4°C?

     

    You may assume that these maximum temperatures are normally distributed and that

  3.  

    • 68% of maximum temperatures have `z`-scores between –1 and 1
    • 95% of maximum temperatures have `z`-scores between –2 and 2
    • 99.7% of maximum temperatures have `z`-scores between –3 and 3.   (3 marks)

Show Answers Only
  1. `21.6^@`
  2. `text(83.85%)`
Show Worked Solution

i.   `mu = 25.8\ \ \ sigma = 4.2`

`text(Using)\ \ \ \ z` `= (x-mu)/sigma`
`-1` `= (x-25.8)/4.2`
`x` `- 25.8` `= -4.2`
`x` `= 21.6°`

 

`:.\ 21.6^@\ text(has a)\ z text(-score of)  -1` 

 

ii.    `z text(-score of)\ 21.6 = -1`

♦ Mean mark 41%
MARKER’S COMMENT: Many students failed to use the answer to (d)(i), costing them valuable exam time.

`text(Find)\ z text(-score of 38.4)`

`z\ (38.4)` `= (38.4\ – 25.8)/4.2=3`

 

`text(68% of scores are between)\ z= –1\ text(and 1)`

`=>\ text(34%)\ text(are between)\ z=–1\ text(and 0)`

`text(99.7% of scores are between)\ z= –3\ text(and 3)`

`=>\ text(49.85%)\ text(are between)\ z=0\ text(and 3)`

 

`:.\ text(% Temps between 21.6° and 38.4°)`

`=\ text(34% + 49.85%)`
`=\ text(83.85%)`

 

 

Statistics, STD2 S5 2013 HSC 29b

Ali’s class sits two Geography tests. The results of her class on the first Geography test are shown.

`58,\ \ 74,\ \ 65,\ \ 66,\ \ 73,\ \ 71,\ \ 72,\ \ 74,\ \ 62,\ \ 70`

The mean was 68.5 for the first test. 

  1. Calculate the standard deviation for the first test. Give your answer correct to one decimal place.    (1 mark)

  2. On the second Geography test, the mean for the class was 74.4 and the standard deviation was 12.4.

     

    Ali scored 62 on the first test. Calculate the mark that she needed to obtain in the second test to ensure that her performance relative to the class was maintained.   (3 marks)

Show Answers Only
  1. `5.2\ \ \ text{(to 1 d.p.)}`
  2. `text(Ali needs to score 58.9)`
Show Worked Solution
♦ Mean mark 39%
COMMENT: Make sure you are confident with this function on your calculator!
i. `sigma` `=5.2201…`
    `=5.2`  `text{(to 1 d.p.)}`

 

ii. `z =(x-mu)/sigma`
`z text{-score (1st test)}` `= (62-68.5)/5.2`
  `=-1.25`

 
`text(2nd test has)\ z text(-score of)\-1.25 :`

♦♦ Mean mark 21%.
MARKER’S COMMENT: When “performance relative to the class is maintained”, `z text(-scores)` are the same in each test.
`-1.25` `= (x-74.4)/12.4`
`x-74.4` `=-15.5`
`x` `=58.9`

 

`:.\ text(Ali needs to score 58.9)`

Statistics, STD2 S5 2010 HSC 24c

The marks in a class test are normally distributed. The mean is 100 and the standard deviation is 10.

  1. Jason's mark is 115. What is his  `z`-score?  (1 mark)

  2. Mary has a `z`-score of 0. What mark did she achieve in the test?   (1 mark)

  3. What percentage of marks lie between 80 and 110?

     

    You may assume the following:

     

    • 68% of marks have a `z`-score between –1 and 1

     

    • 95% of marks have a `z`-score between  –2 and 2

     

    • 99.7% of marks have a `z`-score between –3 and 3.   (2 marks) 

Show Answers Only
  1. `1.5`
  2. `100`
  3. `81.5%`
Show Worked Solution

i.     ` text(Given) \ \ mu=100,\ \ sigma=10`

MARKER’S COMMENT: Too may students had calculator errors in this question, giving away easy marks. BE CAREFUL!

`text(If mark is 115,`

`ztext(-score)` `=(115-mu)/sigma`
  `=(115-100)/100`
  `=1.5`

 

ii.     `z text(-score = 0 when mark equals the mean)`

`:.\ text(Mary’s score was)\ 100`

 

♦ Mean mark 42%
iii.   `ztext(-score of)\ 110` `=(110-100)/10=1`
`ztext(-score of)\ 80` `=(80-100)/10=–2`

 

`text(68% of marks lie between)\ z= –1 \ text(and)\  1`

 `=>text(34%  lie between)\ z= 0\ text(and)\ 1`

`text(95%  of marks lie between)\ z= –2 \ text(and)\  2`

 `=> text(47.5%  lie between)\ z= –2\ text(and)\ 0`

 

`:.\ text(% marks between 80 and 110`

`=\ text(34% + 47.5%)`

`=\ text(81.5%)`