Students in two classes, Class
The results are shown in the dot plots.
Compare the two datasets by examining the skewness, median and spread of the distributions. (3 marks)
--- 7 WORK AREA LINES (style=lined) ---
Statistics, STD1 S1 2020 HSC 6 MC
When blood pressure is measured, two numbers are recorded: systolic pressure and diastolic pressure. If the measurements recorded are 140 systolic and 90 diastolic, then the blood pressure is written as 140/90 mmHg.
Blood pressure measurements are categorized as shown in the diagram.
Amy has a blood pressure of 97/76 mmHg and Betty has a blood pressure of 125/75 mmHg.
Which row of the table describes the blood pressure for Amy and Betty?
Statistics, STD2 S1 SM-Bank 2 MC
The dot plots show the height of students in Year 9 and Year 12 in a school. They are drawn on the same scale.
Which statement about the change in heights when comparing Y9 to Y12 is correct?
- The mean increased and the standard deviation decreased.
- The mean decreased and the standard deviation decreased.
- The mean increased and the standard deviation increased.
- The mean decreased and the standard deviation increased.
Statistics, STD2 S1 2018 HSC 17 MC
The area chart shows the number of students involved in tennis or cricket at a school over a number of years.
In which year was the number of students involved in tennis equal to the number of students involved in cricket?
- 2013
- 2014
- 2015
- 2016
Statistics, STD2 S1 2017 HSC 26f
The area chart shows the number of goals scored by three hockey teams,
- How many goals were scored by team
in round 1? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- In which round did all three teams score the same number of goals? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
Statistics, STD2 S1 2015 HSC 19 MC
The table shows the life expectancy (expected remaining years of life) for females at selected ages in the given periods of time.
In 1975, a 45‑year‑old female used the information in the table to calculate the age to which she was expected to live. Twenty years later she recalculated the age to which she was expected to live.
What is the difference between the two ages she calculated?
- 2.7 years
- 3.1 years
- 3.7 years
- 5.8 years
Statistics, STD2 S1 2006 HSC 8 MC
Which of these graphs best represents positively skewed data with the smaller standard deviation?
Statistics, STD2 S1 2004 HSC 8 MC
This sector graph shows the distribution of 116 prizes won by three schools: X, Y and Z.
How many prizes were won by School X?
- 26
- 32
- 81
- 99
Statistics, STD2 S1 2008 HSC 23e
In a survey, 450 people were asked about their favourite takeaway food. The results are displayed in the bar graph.
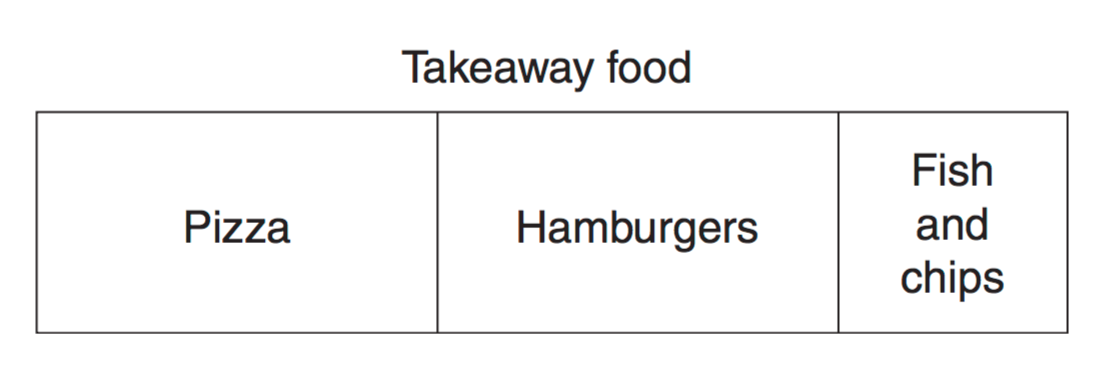
How many people chose pizza as their favourite takeaway food? (2 marks)
Statistics, STD2 S1 2008 HSC 23a
You are organising an outside sporting event at Mathsville and have to decide which month has the best weather for your event. The average temperature must be between 20°C and 30°C, and average rainfall must be less than 80 mm.
The radar chart for Mathsville shows the average temperature for each month, and the table gives the average rainfall for each month.
- If you consider only the temperature data, there are a number of possible months for holding the event. Name ONE of these months. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- If both rainfall and temperature data are considered, which month is the best month for the sporting event? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
Statistics, STD2 S1 2014 HSC 1 MC
The step graph shows the cost of telephone calls to China.
Bella made a telephone call at 9.50 pm to a friend in China and spoke to him until 10.07 pm.
How much did this telephone call cost?
Statistics, STD2 S1 2009 HSC 2 MC
The step graph shows the charges for a carpark.
Maria enters the carpark at 10:10 am and exits at 1:30 pm.
How much will she pay in charges?
Statistics, STD2 S1 2012 HSC 7 MC
The Pi Company has two bakeries. The radar chart displays the monthly sales for the bakeries.
What was the difference in sales in June between the two bakeries?
- $7.50
- $17.50
- $7500
- $17 500
Statistics, STD2 S1 2010 HSC 1 MC
The results of a survey are displayed in the dot plot.
What is the range of this data?
- 7
- 8
- 9
- 10