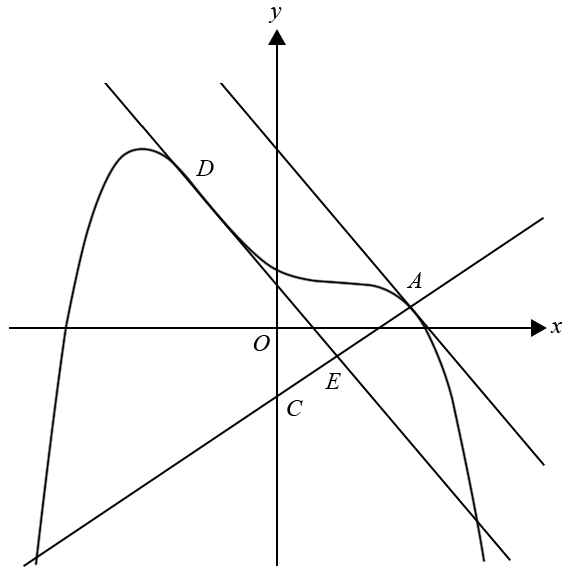
Consider the function
- State, in terms of
where required, the values of for which . (1 mark
--- 2 WORK AREA LINES (style=lined) ---
- Find the values of
for which the graph of has
i. exactly three-intercepts. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
ii. exactly four
-intercepts. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- Let
be the function , which is the function where .
i. Find(1 mark)
--- 2 WORK AREA LINES (style=lined) ---
ii. Find the coordinates of the local maximum of
. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
iii. Find the values of
for which . (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
iv. Consider the two tangent lines to the graph of
at the points where
and . Determine the coordinates of the point of intersection of these two tangent lines. (2 marks) --- 5 WORK AREA LINES (style=lined) ---
- Let
remain as the function , which is the function where . Let
be the function , which is the function where .
i. Using translations only, describe a sequence of transformations of, for which its image would have a local maximum at the same coordinates as that of . (1 mark)
--- 4 WORK AREA LINES (style=lined) ---
ii. Using a dilation and translations, describe a different sequence of transformations of
, for which its image would have both local minimums at the same coordinates as that of . (2 marks)
--- 6 WORK AREA LINES (style=lined) ---