Given the function
- State the domain and range of
. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- i. Find the equation of the tangent to the graph of
at . (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
ii. On the axes below, sketch the graph of the function
, labelling any asymptote with its equation.
Also draw the tangent to the graph of
at . (4 marks)
--- 0 WORK AREA LINES (style=lined) ---


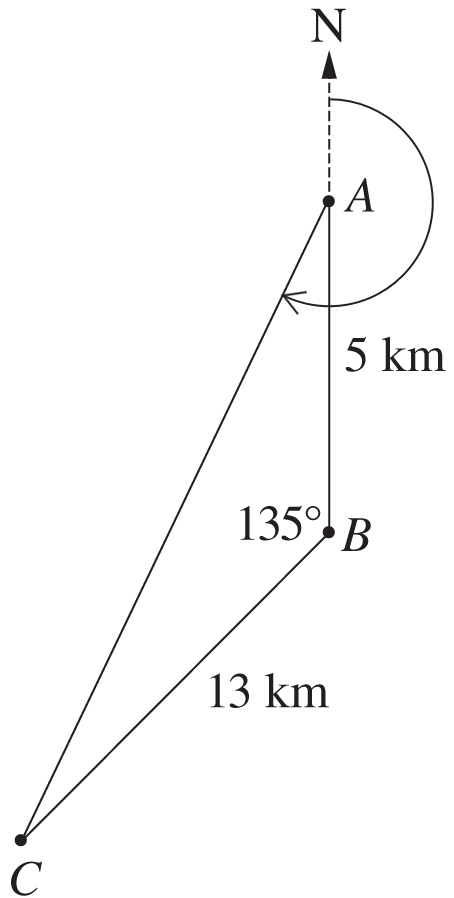




 ).
).