SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Algebra, STD2 A4 2025 HSC 20

The graph of a quadratic function represented by the equation  \(h=t^2-8 t+12\)  is shown.
 

  1. Find the values of \(t\) and \(h\) at the turning point of the graph.   (2 marks)

  2. The graph shows  \(h=12\)  when  \(t=0\).
  3. What is the other value of \(t\) for which  \(h=12\)?   (1 mark)

Show Answers Only

a.   \(\text{Turning point at} \ \ (4,-4)\)

b.   \(t=8\)

Show Worked Solution

a.    \(\text{Axis of quadratic occurs when}\ \ t= \dfrac{2+6}{2} = 4\)

\(\text{At} \ \ t=4:\)

\(h=4^2-8 \times 4+12=-4\)

\(\therefore \ \text{Turning point at} \ \ (4,-4)\)
 

b.    \(\text {When} \ \ h=12:\)

\(t^2-8 t+12\) \(=12\)
\(t(t-8)\) \(=0\)

 
\(\therefore \ \text{Other value:} \ \ t=8\)

v1 Algebra, STD2 A4 2021 HSC 35

A toy store releases a limited edition LEGO set for $20 each. At this price, 3000 LEGO sets are sold each week and the revenue is  `3000 xx 20=$60\ 000`.

The toy store considers increasing the price. For every dollar price increase, 15 fewer LEGO sets will be sold.

If the toy store charges `(20+x)` dollars for each LEGO set, a quadratic model for the revenue raised, `R`, from selling them is

`R=-15x^2+2700x+60\ 000`

 


 

  1. What price should be charged per LEGO set to maximise the revenue?   (2 marks)

  2. How many LEGO sets are sold when the revenue is maximised?   (2 marks)

  3. Find the value of the intercept of the parabola with the vertical axis.   (1 mark) 

Show Answers Only

a.   `$110`

b.    `1650`

c.   `$60\ 000`

Show Worked Solution

a.   `text{Highest revenue}\ (R_text{max})\ text(occurs halfway between)\ \ x= -20 and x=200.`

`text{Midpoint}\ =(-20 + 200)/2 = 90`

`:.\ text(Price of LEGO set for)\ R_text(max)`

`=90 + 20`

`=$110`
 

b.  `text{LEGO sets sold when}\ R_{max}`

`=3000-(90 xx 15)`

`=1650`
 

c.   `ytext(-intercept → find)\ R\ text(when)\ \ x=0:`

`R` `= -15(0)^2 + 2700(0) + 60\ 000`
  `=$60\ 000`

v1 Algebra, STD2 A4 2017 HSC 28e

Sage brings 60 cartons of unpasteurised milk to the market each week. Each carton currently sells for $4 and at this price, all 60 cartons are sold each weekend.

Sage considers increasing the price to see if the total income can be increased.

It is assumed that for each $1 increase in price, 6 fewer cartons will be sold.

A graph showing the relationship between the increase in price per carton and the income is shown below.

 


 

  1. What price per carton should be charged to maximise the income?   (1 mark)

  2. What is the number of cartons sold when the income is maximised?   (1 mark)

  3. The cost of running the market stall is $40 plus $1.50 per carton sold.

    Calculate Sage's profit when the income earned from a day selling at the market is maximised.   (2 marks)

Show Answers Only

a.   `$7`

b.   `42`

c.   `$191`

Show Worked Solution

a.   `text(Graph is highest when increase = $3)`

`:.\ text(Carton price)\ = 4 + 3= $7`
 

b.   `text(Cartons sold)\ =60-(3 xx 6)=42`
  

c.   `text{Cost}\ = 42 xx 1.50 + 40 = $103`

`:.\ text(Profit when income is maximised)`

`= (42 xx 7)-103`

`= $191`

v1 Algebra, STD2 A4 2009 HSC 28c

The brightness of a lamp \((L)\) is measured in lumens and varies directly with the square of the voltage \((V)\) applied, which is measured in volts.

When the lamp runs at 7 volts, it produces 735 lumens.

What voltage is required for the lamp to produce 1820 lumens? Give your answer correct to one decimal place.   (3 marks)

Show Answers Only

 `11.2\ \text(volts)`

Show Worked Solution
♦♦ Mean mark 22%
TIP: Establishing `L=k V^2` is the key part of solving this question.

`L prop V^2\ \ => \ \ L=kV^2`

`text(Find)\ k\ \text{given}\ L = 735\ \text{when}\ V = 7:`

`735` `= k xx 7^2`
`:. k` `= 735/49=15`

 
`text(Find)\ V\ text(when)\ L = 1820:`

`1820` `= 15 xx V^2`
`V^2` `= 1820/15=121.33…`
`V` `= sqrt{121.33} = 11.2\ text(volts)\ \ text{(to 1 d.p.)}`

v1 Algebra, STD2 A4 EO-Bank 1

Taylor discovers that for a Spotted stingray, its mass is directly proportional to the square of its wingspan.

One Spotted stingray has a wingspan of 60 cm and a mass of 5400 grams.

What is the expected wingspan of a Spotted stingray with a mass of 9.6 kg?   (3 marks)

Show Answers Only

`80.0\ text{cm}`

Show Worked Solution

`text(Mass) prop text(wingspan)^2\ \ =>\ \ m = kw^2`

`text(Find)\ k:`

`5400` `= k xx 60^2`
`k` `= 5400/60^2= 1.5`

 
`text(Find)\ w\ text(when)\ \ m = 9600:`

`9600` `= 1.5 xx w^2`
`w^2` `= 9600/1.5=6400`
`:. w` `= 80\ text{cm}`

v1 Algebra, STD2 A4 2023 HSC 22

The stopping distance of a motor bike, in metres, is directly proportional to the square of its speed in km/h, and can be represented by the equation

`text{stopping distance}\ = k xx text{(speed)}^2`

where `k` is the constant of variation.

The stopping distance for a motor bike travelling at 40 km/h is 16 m.

  1. Find the value of `k`.  (2 marks)

  2. What is the stopping distance when the speed of the motor bike is 80 km/h?  (1 mark)

Show Answers Only

a.    `k=0.01`

b.    `64.0\ text{m}`

Show Worked Solution

a.  `text{stopping distance}\ = k xx text{(speed)}^2`

`16` `=k xx 40^2`  
`k` `=16/40^2=0.001`  

 
b.    `text{Find stopping distance}\ (d)\ text{when speed = 80 km/h:}`

`d=0.01 xx 80^2=64.0\ text{m}`

v1 Algebra, STD2 A4 2013 HSC 22 MC

Jevin wants to build a rectangular chicken pen. He has 32 metres of fencing and will use a barn wall as one side of the pen. The width of the pen is \(d\) metres.
 

Which equation gives the area, \(P\), of the chicken pen?

  1. \(P = 16d-d^2\)
  2. \(P = 32d-d^2\)
  3. \(P = 16d-\dfrac{d^2}{2}\)
  4. \(P = 16d-2d^2\) 
Show Answers Only

\(C\)

Show Worked Solution
♦♦♦ Mean mark 24% (lowest mean of any MC question in 2013 exam)

\(\text{Length of pen}\ = \dfrac{1}{2}(32-d)\)

\(\text{Area}\ =d \times \dfrac{1}{2}(32-d)=16d-\dfrac{d^2}{2}\)

 \(\Rightarrow C\)

v1 Algebra, STD2 A4 2022 HSC 9 MC

An object is projected vertically into the air. Its height, \(h\) metres, above the ground after \(t\) seconds is given by  \(h=-5 t^2+80 t\).
 

How far does the object travel in the first 10 seconds?

  1. 300 metres
  2. 320 metres
  3. 340 metres
  4. 480 metres
Show Answers Only

\(C\)

Show Worked Solution

\(\text{By symmetry (or graph), object reaches max height at}\ \ t=8\ \text{seconds.}\)

\(\text{Find}\ h\ \text{when}\ \ t=8:\)

\(h=-5 \times 8^2-10 \times 8= 320 \)

\(\text{When}\ \ t=10\ \ \Rightarrow\ \ h=300\ \text{(from graph)}\)

\(\therefore\ \text{Total distance}\ = 320 + 20=340\ \text{metres}\)

\(\Rightarrow C\)

Algebra, STD2 A4 2024 HSC 26

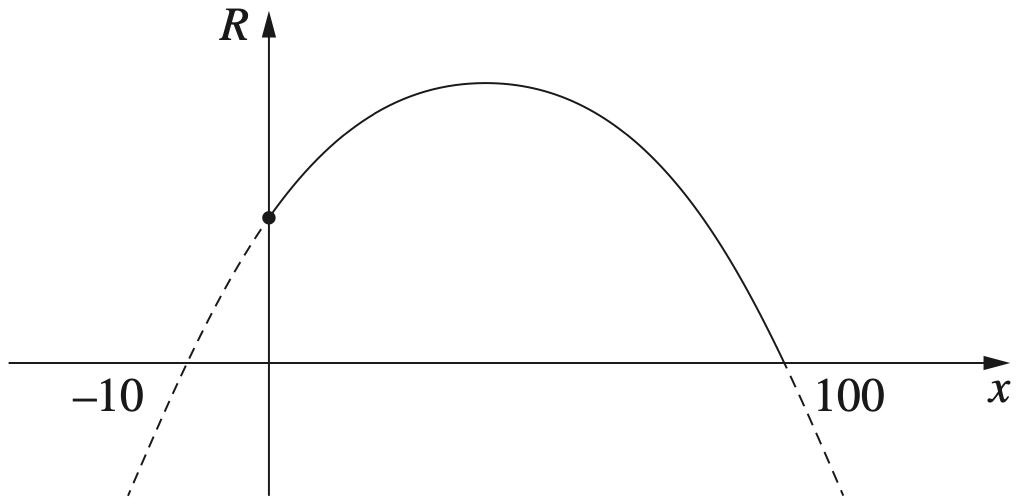
A sheet of metal is folded to make a gutter, as shown. The cross-section of the gutter is a rectangle of width \(w\) cm and height \(h\) cm.
 

 

The area, \(A\) cm\(^{2}\), of the cross-section can be modelled by the quadratic formula

\(A=-0.5w^{2}+20w\)

A graph of this model is shown.
 

Find the width and height of the rectangle which will give the greatest possible area of the cross-section.   (3 marks)

Show Answers Only

\(w=20\ \text{cm}, h=10\ \text{cm}\)

Show Worked Solution

\(\text{Graph cuts}\ w\text{-axis at}\ \ w=0\ \ \text{and}\ \ w=40.\)

\(\text{By symmetry, maximum area occurs at}\ \ w=20.\)

\(A_{\text{max}}=-0.5 \times 20^2 + 20 \times 20 = 200\ \text{cm}^{2}\)

\(A_{\text{max}}\) \(=w \times h\)  
\(200\) \(=20 \times h\)  
\(h\) \(=10\ \text{cm}\)  

 
\(\therefore A_{\text{max}}\ \text{occurs when:}\ w=20\ \text{cm}, \ h=10\ \text{cm}\)

♦ Mean mark 43%.

Algebra, STD2 A4 2023 HSC 22

The braking distance of a car, in metres, is directly proportional to the square of its speed in km/h, and can be represented by the equation

`text{braking distance}\ = k xx text{(speed)}^2`

where `k` is the constant of variation.

The braking distance for a car travelling at 50 km/h is 20 m.

  1. Find the value of `k`.  (2 marks)

  2. What is the braking distance when the speed of the car is 90 km/h?  (1 mark)

Show Answers Only

a.    `k=0.008`

b.    `64.8\ text{m}`

Show Worked Solution

a.  `text{braking distance}\ = k xx text{(speed)}^2`

`20` `=k xx 50^2`  
`k` `=20/50^2`  
  `=0.008`  

 
b.    `text{Find braking distance}\ (d)\ text{when speed = 90 km/h:}`

`d` `=0.008 xx 90^2`  
  `=64.8\ text{m}`  

Algebra, STD2 A4 2023 HSC 20

On another planet, a ball is launched vertically into the air from the ground.

The height above the ground, `h` metres, can be modelled using the function  `h=-6 t^2+24t`, where `t` is measured in seconds. The graph of the function is shown.

  1. Based on the graph, what is the maximum height reached by the ball?  (1 mark)

  2. Based on the graph, at what TWO times is the ball at `3/4` of its maximum height?  (2 marks)

Show Answers Only

a.    `h_max = 24\ text{metres}`

b.    `t=1 and 3\ text{seconds}`

Show Worked Solution

a.    `h_max = 24\ text{metres}`

b.    `3/4 xx h_max = 3/4xx24=18\ text{metres}`

`text{From graph, ball is at at 18 metres when:}`

`t=1 and 3\ text{seconds}`

Algebra, STD2 A4 2022 HSC 9 MC

An object is projected vertically into the air. Its height, `h` metres, above the ground after `t` seconds is given by  `h=-5 t^2+80 t`.
 

For how long is the object at a height of 300 metres or more above the ground?

  1. 4 seconds
  2. 6 seconds
  3. 8 seconds
  4. 10 seconds
Show Answers Only

`A`

Show Worked Solution

`text{Object reaches 300 m when}\ \ t=6\ text{seconds.}`

`text{Object drops back below 300 m when}\ \ t=10\ text{seconds.}`

`text{Time at 300 m or above}\ = 10-6=4\ text{seconds}`

`=>A`

Algebra, STD2 A4 2021 HSC 35

A publisher sells a book for $10. At this price, 5000 copies of the book will be sold and the revenue raised will be  `5000 xx 10=$50\ 000`.

The publisher is considering increasing the price of the book. For every dollar the price of the book is increased, the publisher will sell 50 fewer copies of the book.

If the publisher charges `(10+x)` dollars for each book, a quadratic model for the revenue raised, `R`, from selling books is

`R=-50x^2+4500x + 50\ 000`

 


 

  1. By first finding a suitable value of `x`, find the price the publisher should charge for each book to maximise the revenues raised from sales of the book.  (2 marks)

  2. Find the value of the intercept of the parabola with the vertical axis.  (1 mark) 

Show Answers Only
  1. `$55`
  2. `$50\ 000`
Show Worked Solution

a.   `text{Highest revenue}\ (R)\ text(occurs halfway between)\ \ x= –10 and x=100.`

`text{Midpoint}\ =(-10 + 100)/2 = 45`

♦♦♦ Mean mark part (a) 16%.

`:.\ text(Price of book for)\ R_text(max)`

`=45 + 10`

`=$55`
 

♦♦♦ Mean mark part (b) 21%.

b.   `ytext(-intercept → find)\ \ R\ \ text(when)\ \ x=0:`

`R` `= -50(0)^2 + 4500(0) + 50\ 000`
  `=$50\ 000`

Algebra, STD2 A4 2020 HSC 19

A fence is to be built around the outside of a rectangular paddock. An internal fence is also to be built.

The side lengths of the paddock are `x` metres and `y` metres, as shown in the diagram.
 

 
A total of 900 metres of fencing is to be used. Therefore  `3x + 2y = 900`.
 
The area, `A`, in square metres, of the rectangular paddock is given by  `A =450x - 1.5x^2`.

The graph of this equation is shown.
  

  1. If the area of the paddock is `30 \ 000\ text(m)^2`, what is the largest possible value of `x`?   (1 mark)

  2. Find the values of `x` and `y` so that the area of the paddock is as large as possible.   (2 marks)

  3. Using your value from part (b), find the largest possible area of the paddock.   (1 mark)

Show Answers Only
  1. `200 \ text(m)`
  2. `x = 150 \ text(m and) \ y = 225 \ text(m)`
  3. `33 \ 750 \ text(m)^2`
Show Worked Solution

a.     `text(From the graph, an area of)\ 30\ 000\ text(m)^2`

♦ Mean mark part (a) 39%.

  `text(can have an)\ x text(-value of)\ \ x=100 or 200\ text(m.)`

`:. x_text(max) = 200 text(m)`
 

b.    `A_text(max) \ text(occurs when) \ \ x = 150`

♦♦ Mean mark part (b) 34%.

`text(Substitute)\ \ x=150\ \ text(into)\ \ 3x + 2y = 900:`

`3 xx 150 + 2y` `= 900`
`2y` `= 450`
`y` `= 225`

 
`therefore \ text(Maximum area when) \ \ x = 150 \ text(m  and) \ \ y = 225 \ text(m)`

♦ Mean mark part (c) 40%.
c.    `A_(max)` `= xy`
    `= 150 xx 225`
    `= 33 \ 750 \ text(m)^2`

Algebra, STD2 A4 2019 HSC 31

A rectangle has width `w` centimetres. The area of the rectangle, `A`, in square centimetres, is  `A = 2w^2 + 5w`.

The graph  `A = 2w^2 + 5w`  is shown.
 


 

  1. Explain why, in this context, the model  `A = 2w^2 + 5w`  only makes sense for the bold section of the graph.  (1 mark)

  2. The area of the rectangle is 18 cm². Calculate the perimeter of the rectangle.  (2 marks)

Show Answers Only
  1. `text(The width of a rectangle cannot be negative.)`
  2. `22\ text(cm)`
Show Worked Solution

a.   `text(The width of a rectangle cannot be negative.)`

♦ Mean mark 44%.

 

b.   `text(When)\ A = 18, w = 2`

♦ Mean mark 42%.

`text(Let)\ h =\ text(height of rectangle)`

`18` `= 2 xx h`
`h` `= 9\ text(cm)`

 

`:.\ text(Perimeter)` `= 2 xx (2 + 9)`
  `= 22\ text(cm)`

Algebra, STD2 A4 SM-Bank 2

Moses finds that for a Froghead eel, its mass is directly proportional to the square of its length.

An eel of this species has a length of 72 cm and a mass of 8250 grams.

What is the expected length of a Froghead eel with a mass of 10.2 kg? Give your answer to one decimal place.  (3 marks)

Show Answers Only

`80.1\ text{cm}`

Show Worked Solution

`text(Mass) prop text(length)^2`

`m = kl^2`
 

`text(Find)\ k:`

`8250` `= k xx 72^2`
`k` `= 8250/72^2`
  `= 1.591…`

 
`text(When)\ \ l\ \ text(when)\ \ m = 10\ 200:`

`10\ 200` `= 1.591… xx l^2`
`l^2` `= (10\ 200)/(1.591…)`
`:. l` `= 80.069…`
  `= 80.1\ text{cm  (to 1 d.p.)}`

Algebra, STD2 A4 2017 HSC 28e

A movie theatre has 200 seats. Each ticket currently costs $8.

The theatre owners are currently selling all 200 tickets for each session. They decide to increase the price of tickets to see if they can increase the income earned from each movie session.

It is assumed that for each one dollar increase in ticket price, there will be 10 fewer tickets sold.

A graph showing the relationship between an increase in ticket price and the income is shown below.
 


 

  1. What ticket price should be charged to maximise the income from a movie session?  (1 mark)

  2. What is the number of tickets sold when the income is maximised?  (1 mark)

  3. The cost to the theatre owners of running each session is $500 plus $2 per ticket sold.

     

    Calculate the profit earned by the theatre owners when the income earned from a session is maximised.  (2 marks)

Show Answers Only
  1. `$14`
  2. `140`
  3. `$1180`
Show Worked Solution

i.   `text(Graph is highest when increase = $6)`

♦ Mean mark 50%.

`:.\ text(Ticket price)\ = 8 + 6= $14`
 

ii.   `text(Solution 1)`

♦ Mean mark 45%.

`text(Tickets sold)\ =200-(4 xx 10)=140`
 

`text(Solution 2)`

`text(Tickets)\ = text(max income)/text(ticket price) = 1960/14= 140`
 

iii.  `text{Cost}\ = 140 xx $2 + $500= $780`

`:.\ text(Profit when income is maximised)`

`= 1960-780`

`= $1180`

Algebra, STD2 A4 2015 HSC 29e

A diver springs upwards from a diving board, then plunges into the water. The diver’s height above the water as it varies with time is modelled by a quadratic function. Graphing software is used to produce the graph of this function.
 

Explain how the graph could be used to determine how high above the height of the diving board the diver was when he reached the maximum height.   (2 marks)

Show Answers Only

`1.2\ text(m)`

Show Worked Solution

`text(We can calculate the height of the board by)`

♦♦ Mean mark 19%.

`text(finding the)\ ytext(-value at)\ t = 0,\ text(which is 8 m.)`

`text(The diver’s maximum height occurs at)\ t=0.5,`

`text(which is approximately 9.2 m.)`
 

`:.\ text(Maximum height above the board)`

`= 9.2-8`

`= 1.2\ text(m)`

Algebra, STD2 A4 2006 HSC 28b

A new tunnel is built. When there is no toll to use the tunnel, 6000 vehicles use it each day. For each dollar increase in the toll, 500 fewer vehicles use the tunnel.

  1. Find the lowest toll for which no vehicles will use the tunnel.  (1 mark)

  2. For a toll of $5.00, how many vehicles use the tunnel each day and what is the total daily income from tolls?  (2 marks)

  3. If `d` (dollars) represents the value of the toll, find an equation for the number of vehicles `(v)` using the tunnel each day in terms of `d`.  (2 marks)

  4. Anne says ‘A higher toll always means a higher total daily income’.

     

    Show that Anne is incorrect and find the maximum daily income from tolls. (Use a table of values, or a graph, or suitable calculations.)  (3 marks)

Show Answers Only
  1. `text($12 toll is the lowest for which)`

     

    `text(no vehicles will use the tunnel.)`

  2. `$17\ 500`
  3. `v = 6000 – 500d`
  4. `text{Proof (See Worked Solutions)}`
Show Worked Solution

i.  `text(500 less vehicles per $1 toll)`

`12 xx 500 = 6000`

`:. $12\ text(toll is the lowest for which no)`

`text(vehicles will use the tunnel.)`

 

ii.  `text(If the toll is $5)`

`5 xx 500 = 2500\ text(less vehicles)`

`:.\ text(Vehicles using the tunnel)`

`= 6000 – 2500`

`= 3500`

`:.\ text(Daily toll income)` `= 3500 xx $5`
  `= $17\ 500`

 

iii.    `d` `=\ text(toll)`
`v` `=\ text(Number of vehicles using the tunnel)`
    `:. v` `= 6000 – 500d`

 

iv.  `text(Income from tolls)`

`=\ text(Number of vehicles) xx text(toll)`

`= (6000 – 500d) xx d`

`= 6000d – 500d^2`

`= 500d (12 – d)`
 

 

`text(From the graph, the maximum income from tolls)`

`text(occurs when the toll is $6.)`

`:.\ text(Anne is incorrect.)`

 

`text(Alternate Solution)`

`text{The table of values shows that income (I) increases}`

`text(and peaks when the toll hits $6 before decreasing)`

`text(again as the toll gets more expensive.)`

`:.\ text(Anne is incorrect.)`

Algebra, STD2 A4 2009 HSC 28c

The height above the ground, in metres, of a person’s eyes varies directly with the square of the distance, in kilometres, that the person can see to the horizon.

A person whose eyes are 1.6 m above the ground can see 4.5 km out to sea.

How high above the ground, in metres, would a person’s eyes need to be to see an island that is 15 km out to sea? Give your answer correct to one decimal place.   (3 marks)

Show Answers Only

 `17.8\ text(m)\ \ text{(to 1 d.p.)}`

Show Worked Solution
♦♦ Mean mark 22%
CRITICAL STEP: Reading the first line of the question carefully and establishing the relationship `h=k d^2` is the key part of solving this question.

`h prop d^2`

`h=kd^2`

`text(When)\ h = 1.6,\ d = 4.5`

`1.6` `= k xx 4.5^2`
`:. k` `= 1.6/4.5^2`
  `= 0.07901` `…`

 

`text(Find)\ h\ text(when)\ d = 15`

`h` `= 0.07901… xx 15^2`
  `= 17.777…`
  `= 17.8\ text(m)\ \ \ text{(to 1 d.p.)}`

Algebra, STD2 A4 2009 HSC 28a

Anjali is investigating stopping distances for a car travelling at different speeds. To model this she uses the equation
 

`d = 0.01s^2+ 0.7s`,
 

where `d` is the stopping distance in metres and `s` is the car’s speed in km/h.

The graph of this equation is drawn below.

2009 28a

  1. Anjali knows that only part of this curve applies to her model for stopping distances.

     

    In your writing booklet, using a set of axes, sketch the part of this curve that applies for stopping distances.  (1 mark)

  2. What is the difference between the stopping distances in a school zone when travelling at a speed of 40 km/h and when travelling at a speed of 70 km/h?   (2 marks)

Show Answers Only
  1.  
    2UG-2009-28a-Answer
  2. `54\ text(metres)`
Show Worked Solution
(i)

2UG-2009-28a-Answer

(ii) `text(When)\ \ s = 40`

♦ Mean mark 41%
COMMENT: Students could easily have used the graph for calculating `d` at `text(40 km/h)`, although the formula was required when the speed increased to `text(70 km/h)`.
`d` `= 0.01(40^2)+ 0.7 (40)`
  `= 16+28`
  `= 44\ text(m)`

 

`text(When)\ \ s = 70`

`d` `= 0.01 (70^2) +0.7(70)`
  `= 49 +49`
  `= 98\ text(m)`

 

`:.\ text(Difference)` `= 98\ -44`
  `= 54\ text(metres)`

Algebra, STD2 A4 2012 HSC 30b

A golf ball is hit from point `A` to point `B`, which is on the ground as shown. Point `A` is 30 metres above the ground and the horizontal distance from point `A` to point `B` is  300 m.
 

The path of the golf ball is modelled using the equation 

`h = 30 + 0.2d-0.001d^2` 

where 

`h` is the height of the golf ball above the ground in metres, and 

`d` is the horizontal distance of the golf ball from point `A` in metres.

The graph of this equation is drawn below.

  

  1. What is the maximum height the ball reaches above the ground?    (1 mark)

  2. There are two occasions when the golf ball is at a height of 35 metres.

     

    What horizontal distance does the ball travel in the period between these two occasions?   (1 mark)

  3. What is the height of the ball above the ground when it still has to travel a horizontal distance of 50 metres to hit the ground at point `B`?   (1 mark)

  4. Only part of the graph applies to this model.

     

    Find all values of `d` that are not suitable to use with this model, and explain why these values are not suitable.   (2 marks)

Show Answers Only
  1. `40 text(m)`
  2. `140 text(m)`
  3. `text(17.5 m)`
  4. `d < 0\ text(and)\ d>300`
Show Worked Solution

i.   `text(Max height) = 40 text(m)`

COMMENT: With a mean mark of 92% in (i), a classic example of low hanging fruit in later questions.

 

ii.   `text(From graph)`

`h = 35\ text(when)\ x = 30\ text(and)\ x = 170`

`:.\ text(Horizontal distance)` `= 170-30`
  `= 140\ text(m)`

 

iii.   `text(Ball hits ground at)\ x = 300`

MARKER’S COMMENT: Responses for (iii) in the range  `17<=\ h\ <=18`  were deemed acceptable estimates read off the graph.

`=>text(Need to find)\ y\ text(when)\ x = 250`

`text(From graph,)\ y = 17.5 text(m)\ text(when)\ x = 250`

`:.\ text(Height of ball is 17.5 m at a horizontal)`

`text(distance of 50m before)\ B.`

 

iv.   `text(Values of)\ d\ text(not suitable).`

♦♦♦ Mean mark (iv) 12%
MARKER’S COMMENT: Many students did not refer to the domain `d>300` as unsuitable to the model.

`text(If)\ d < 0 text(, it assumes the ball is hit away)`

`text(from point)\ B text(. This is not the case in our)`

`text(example.)`

`text(If)\ d > 300 text(,)\ h\ text(becomes negative which is)`

`text(not possible given the ball cannot go)`

`text(below ground level.)`

Algebra, STD2 A4 2013 HSC 22 MC

Leanne wants to build a rectangular vegetable garden in her backyard. She has 20 metres of fencing and will use a wall as one side of the garden. The plan for her garden is shown, where `x` metres is the width of her garden.
 

 

Which equation gives the area, `A`, of the vegetable garden?

  1.    `A=10x-x^2`
  2.    `A=10x-2x^2`
  3.    `A=20x-x^2`
  4.    `A=20x-2x^2`
Show Answers Only

`D`

Show Worked Solution
♦♦♦ Mean mark 24% (lowest mean of any MC question in 2013 exam)

`text(Length of garden)=(20-2x)`

`text(Area)` `=x(20-2x)`
  `=20x-2x^2`

 
`=>\ D`