SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Financial Maths, STD2 F1 2019 HSC 7 MC

Julia earns $28 per hour. Her hourly pay rate increases by 2%.

How much will she earn for a 4-hour shift with this increase?

  1. $2.24
  2. $28.56
  3. $112
  4. $114.24
Show Answers Only

Show Worked Solution
 

 

 

Financial Maths, STD2 F1 2019 HSC 6 MC

Mary is 18 years old and has just purchased comprehensive motor vehicle insurance. The following excesses apply to claims for at-fault motor vehicle accidents.

 
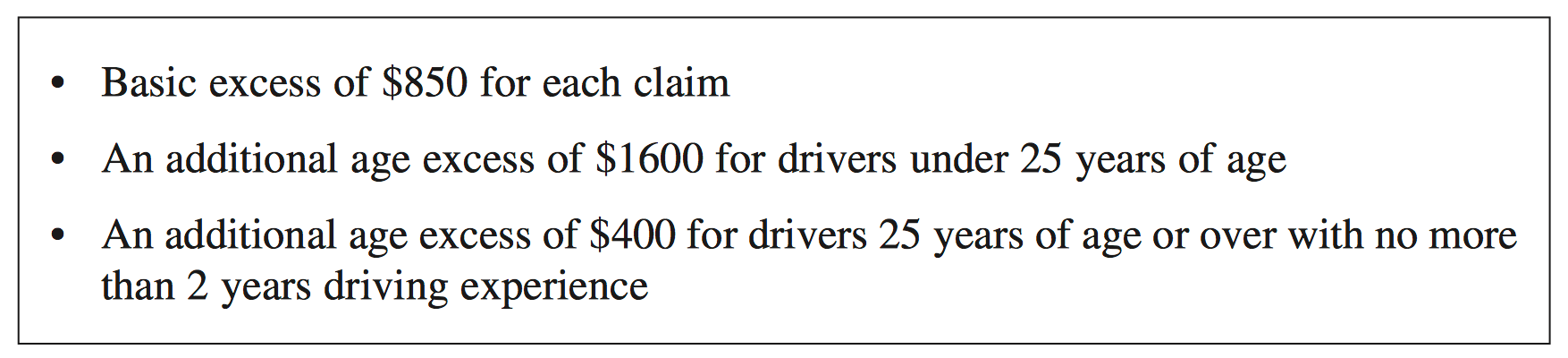
How much would Mary be required to pay as excess if she made a claim as the driver at fault in a car accident?

  1. $1600
  2. $850 + $400
  3. $850 + $1600
  4. $850 + $1600 + $400
Show Answers Only

Show Worked Solution

Statistics, STD2 S4 2019 HSC 23

A set of bivariate data is collected by measuring the height and arm span of seven children. The graph shows a scatterplot of these measurements.
 


 

  1. Calculate Pearson's correlation coefficient for the data, correct to two decimal places.  (1 mark)

  2. Identify the direction and the strength of the linear association between height and arm span.  (1 mark)

  3. The equation of the least-squares regression line is shown.
     
               Height = 0.866 × (arm span) + 23.7
     
    A child has an arm span of 143 cm.

     

    Calculate the predicted height for this child using the equation of the least-squares regression line.  (1 mark)

Show Answers Only

Show Worked Solution

a.   

♦ Mean mark 40%.
COMMENT: Issues here? YouTube has short and excellent help videos – search your calculator model and topic – eg. “fx-82 correlation” .

 

 

b.   

 

c.   
   

Measurement, STD2 M7 2019 HSC 18

Andrew, Brandon and Cosmo are the first three batters in the school cricket team. In a recent match, Andrew scored 30 runs, Brandon scored 25 runs and Cosmo scored 40 runs.

  1. What is the ratio of Andrew's to Brandon's to Cosmo's runs scored, in simplest form?  (2 marks)

  2. In this match, the ratio of the total number of runs scored by Andrew, Brandon and Cosmo to the total number of runs scored by the whole team is .
  3. How many runs were scored by the whole team?  (2 marks)

Show Answers Only
Show Worked Solution
a.   
   

 

b.   
   

 

 

Vectors, EXT1 V1 SM-Bank 9

The diagram shows a projectile fired at an angle    to the horizontal from the origin with initial velocity  .
 

The position vector for the projectile is given by
 

     (DO NOT prove this)
 

where is the acceleration due to gravity.

  1.  Show the horizontal range of the projectile is

      (2 marks)

The projectile is fired so that  .

  1.  State whether the projectile is travelling upwards or downwards when

      (1 mark)

Show Answers Only
Show Worked Solution

i.   

 

 
 

 

ii.   

Vectors, EXT1 V1 SM-Bank 6

A cricketer hits a ball at time    seconds from an origin at ground level across a level playing field.

The position vector  , from , of the ball after seconds is given by
 
  ,
 
where,    is a unit vector in the forward direction,   is a unit vector vertically up and displacement components are measured in metres.

  1. Find the initial velocity of the ball and the initial angle, in degrees, of its trajectory to the horizontal.  (2 marks)

  2. Find the maximum height reached by the ball, giving your answer in metres, correct to two decimal places.  (2 marks)

  3. Find the time of flight of the ball. Give your answer in seconds, correct to three decimal places.  (1 mark)

  4. Find the range of the ball in metres, correct to one decimal place.  (1 mark)

  5. A fielder, more than 40 m from , catches the ball at a height of 2 m above the ground.

     

    How far horizontally from is the fielder when the ball is caught? Give your answer in metres, correct to one decimal place.  (2 marks)

Show Answers Only

  1.  

Show Worked Solution

a.   


 

 
   
 

 

b. 

 

 

 

 

c.   


 

d. 

 
 


e.   

 
 

 

   
     

 


 

 


 

Statistics, EXT1 S1 2012 MET2 3

Steve and Jess are two students who have agreed to take part in a psychology experiment. Each has to answer several sets of multiple-choice questions. Each set has the same number of questions, , where is a number greater than 20. For each question there are four possible options A, B, C or D, of which only one is correct.

  1. Steve decides to guess the answer to every question, so that for each question he chooses A, B, C or D at random.

     

    Let the random variable be the number of questions that Steve answers correctly in a particular set.

    1. What is the probability that Steve will answer the first three questions of this set correctly?  (1 mark)

    2. Use the fact that the variance of is to show that the value of is 25.  (1 mark)

  1. The probability that Jess will answer any question correctly, independently of her answer to any other question, is  . Let the random variable be the number of questions that Jess answers correctly in any set of 25.

    If   , show that the value of  .  (2 marks)

Show Answers Only

a.i. 

a.ii. 

b. 

Show Worked Solution
a.i.   
   

 

a.ii.   
 
 
 

 

b.  

♦♦♦ Mean mark part (c) 19%.

 

Vectors, EXT2 V1 2014 SPEC1 1

Consider the vector  , where    and    are unit vectors in the positive directions of the and axes respectively.

  1.  Find the unit vector in the direction of  .  (1 mark)

  2.  Find the acute angle that    makes with the positive direction of the -axis.  (2 marks)

  3.  The vector  .

     

     Given that    is perpendicular to  find the value of .  (2 marks)

Show Answers Only
Show Worked Solution
i.   
   
 

 

Mean mark part (b) 51%.

ii.   
 
   
 

 

iii.   

 
 

Vectors, EXT2 V1 2013 SPEC1 3

The coordinates of three points are 

  1. Find    (1 mark)

  2. The points and are the vertices of a triangle. 

     

    Prove that the triangle has a right angle at   (2 marks)

  3. Find the length of the hypotenuse of the triangle.  (1 mark)

Show Answers Only
Show Worked Solution
i.  
   

 

ii.   
   

 

 
 

 


 

iii.   
   

 

 
 

Functions, EXT1 F1 SM-Bank 12

Given  , without calculus sketch a separate half page graph of the following functions, showing all asymptotes and intercepts.

  1.     (1 mark)

  2.     (2 marks)

  3.      (2 marks)

Show Answers Only
  1.  
  2.   
  3.   
Show Worked Solution
i.   
   
   

 

 

ii.   

 

 

iii.

Financial Maths, 2ADV M1 SM-Bank 7

Joe buys a tractor under a buy-back scheme. This scheme gives Joe the right to sell the tractor back to the dealer.

The recurrence relation below can be used to calculate the price Joe sells the tractor back to the dealer , after years
 


  

  1. Write the general rule to find the value of    in terms of  .  (1 mark)

  2. After how many years will the dealer offer to buy back Joe's tractor at half of its original value.  (1 mark)

Show Answers Only

i. 

ii. 

Show Worked Solution
i.   
 
   
   
     

 

ii.   

 

Financial Maths, 2ADV M1 SM-Bank 6

Julie deposits some money into a savings account that will pay compound interest every month.

The balance of Julie’s account, in dollars, after months,  , can be modelled by the recurrence relation shown below.
 


 

  1.  Recursion can be used to calculate the balance of the account after one month.

     

    1. Write down a calculation to show that the balance in the account after one month, , is  $12 074.40. (1 mark)

    2. After how many months will the balance of Julie’s account first exceed $12 300  (1 mark)

  2.  A rule of the form    can be used to determine the balance of Julie's account after months.

    1. Complete this rule for Julie’s investment after months by writing the appropriate numbers in the boxes provided below. (1 mark)
       
balance = 
 
  × 
 
  n

 

    1. What would be the value of if Julie wanted to determine the value of her investment after three years?  (1 mark)

Show Answers Only

a.i.   

a.ii. 

b.i 

b.ii. 

Show Worked Solution
a.i.  
   
   

 

a.ii.  
 
 

 

 
b.i.  

 
b.ii. 

Financial Maths, 2ADV M1 SM-Bank 1 MC

On day 1, Vikki spends 90 minutes on a training program.

On each following day, she spends 10 minutes less on the training program than she did the day before.

Let    be the number of minutes that Vikki spends on the training program on day  .

A recursive equation that can be used to model this situation for    is

A.  
B.  
C.  
D.  

 

Show Answers Only

Show Worked Solution

Trigonometry, 2ADV T3 SM-Bank 14

For the function  

  1. Write down the amplitude and period of the function  (2 marks)

  2. Sketch the graph of the function    on the set of axes below. Label axes intercepts with their coordinates.

     

    Label endpoints of the graph with their coordinates.  (3 marks)

VCAA 2006 meth 4b

Show Answers Only

  2.  
Show Worked Solution

a.  

 

b.   

Trigonometry, 2ADV T3 SM-Bank 10

The population of wombats in a particular location varies according to the rule  , where is the number of wombats and is the number of months after 1 March 2018.

  1. Find the period and amplitude of the function .  (2 marks)

  2. Find the maximum and minimum populations of wombats in this location.  (2 marks)

  3. Find  .  (1 mark)

  4. Over the 12 months from 1 March 2018, find the fraction of time when the population of wombats in this location was less than  .  (2 marks)

Show Answers Only
Show Worked Solution

i.   


 

ii.  


 

iii.   
   
   
   

 

iv. 

 
 
 
 

 


 

 

Trigonometry, 2ADV T3 SM-Bank 9

Let     for  .

  1. Solve the equation    for  .  (2 marks)

  2. Sketch the graph of the function    on the axes below. Label the endpoints and local minimum point with their coordinates.  (3 marks)

 

Show Answers Only
  2.  
Show Worked Solution
i.
 

 

 

ii.   

Networks, STD2 N3 SM-Bank 45

An oil pipeline network is drawn below that shows the flow capacity of oil pipelines in kilolitres per hour.
 


 

A cut is shown.

  1. What is the capacity of the cut.  (1 mark)

  2. Calculate the minimum cut of this network?  (2 marks)

  3. Copy the network diagram, showing the maximum flow capacity of the network by labelling the flow of each edge.  (2 marks)

Show Answers Only
  3.  
Show Worked Solution
i.   
   

 

ii. 

♦♦ COMMENT: Be very careful! RS is not included as it goes from sink to source.
 


  

 

 

iii.   

Calculus, SPEC2 2012 VCAA 3

A car accelerates from rest. Its speed after seconds is , where

  1. Write down the limiting speed of the car as  .   (1 mark)

  2. Calculate, correct to the nearest , the acceleration of the car when  .   (1 mark)

  3. Calculate, correct to the nearest second, the time it takes for the car to accelerate from rest to .   (2 marks)

After accelerating to , the car stays at this speed for 120 seconds and then begins to decelerate while braking. The speed of the car    seconds after the brakes are first applied is where

until the car comes to rest.

  1. i.  Find in terms of .   (2 marks)

  2. ii. Find the time, in seconds, taken for the car to come to rest while braking.   (2 marks)

  3. i.  Write down the expressions for the distance travelled by the car during each of the three stages of its motion.   (2 marks)

  4. ii. Find the total distance travelled from when the car starts to accelerate to when it comes to rest.

     

        Give your answer in metres correct to the nearest metre.   (1 mark)

Show Answers Only
  4. i. 
  5. ii.
  6. i. 

     

       

     

       

  7. ii.
Show Worked Solution

a.   

 

 

b. 
 

 

 
c.   

 
 
   

 

d.i.   
 

 


 

d.ii. 

 

e.i.   


  

 

 

 

e.ii.   
 
 

 

 

Complex Numbers, SPEC2 2012 VCAA 2

  1.  Given that  , show that  .   (2 marks)

  2. Express    in polar form.   (1 mark)

  3. i.  Write down    in polar form.   (1 mark)

  4. ii. On the Argand diagram below, shade the region defined by

.   (2 marks)


 

  1.  Find the area of the shaded region in part c.   (2 marks) 
  2. i.  Find the value(s) of such that  , where  .   (3 marks)

  3. ii. Find    for the value(s) of found in part i.   (1 mark)

Show Answers Only
  2. i. 

     

    ii. 

  5. i. 

     

    ii. 

Show Worked Solution
a.   
 
   

 

 

b.i.   
   

 

b.ii.   
   

 

c.   

 

d. 

Mean mark 51%.


  

 


 

♦ Mean mark (e)(i) 34%.

e.i.   
 
 
 
 

 

e.ii. 

♦ Mean mark (e)(ii) 36%.

 
   
   

 

Vectors, SPEC1 2011 VCAA 9

Consider the three vectors

  and  , where

  1. Find the value(s) of for which    (2 marks)

  2. Find the value of such that    is perpendicular to    (1 mark)

  3. i.  Calculate    (1 mark)

  4. ii. Hence find a value of such that    and    are linearly dependent.  (1 mark)

Show Answers Only

  3. i. 
  4. ii.

Show Worked Solution

a.   
   
   
   

 

b.     
   
   
   

 

c.i.   

 

c.ii.   

Vectors, SPEC1 2012 VCAA 9

The position of a particle at time    is given by

  1. Find the velocity of the particle at time     (1 mark)

  2. Find the speed of the particle at time    in the form  , where and are positive integers.   (2 marks)

  3. Show that at time     (2 marks)

  4. Find the angle in terms of , between the vector    and the vector    at time     (2 marks)

Show Answers Only
Show Worked Solution

a. 

 
   
   

 

b.   
   
 
   
   
   
   
   

♦ Mean mark part (c) 41%.

c.   
 
 
   
   
   

 

d.    

♦♦ Mean mark part (d) 32%.