Suppose that a differentiable function
Determine the gradient of the tangent line to the graph of
Aussie Maths & Science Teachers: Save your time with SmarterEd
Suppose that a differentiable function
Determine the gradient of the tangent line to the graph of
The diagram below shows part of the graph of
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
The tangent to
--- 6 WORK AREA LINES (style=lined) ---
The diagram below shows part of the graph of
--- 5 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
a.
b.
c.
di.
dii. Area
e.
a. Axis of symmetry:
| b. | |||
c. At
When
Equation of tangent at
d.i Gradient of tangent
Equation at
d.ii Points of intersection of
So equate
| Area | ||
e.
At
Gradient of tangent
Gradient of normal
Equation of normal at
Points of intersection of normal and parabola (Using CAS)
solve
Calculate area using CAS
Using CAS Solve derivative of
solve
Given
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
Let
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 0 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
a.
b.
ci.
cii.
d.
e.
f.
g.
h.
a.
| b. | ||
| |
||
cii.
| d. | ||
f.
g.
h.
A polynomial has the equation
The number of tangents to this curve that pass through the positive
Let
Let
Let
--- 7 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
The coordinate
Let
--- 8 WORK AREA LINES (style=lined) ---
Let
--- 3 WORK AREA LINES (style=lined) ---
A property of the graphs of
--- 6 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
a.
b.
c.
d.i.
d.ii.
e.
| f. | ||
g.
h.
The graph of the function
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
Two line segments connect the points
--- 3 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
a.
b.
c.
d.i.
d.ii.
e.i.
e.ii.
e.iii.
Let
--- 4 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
Let
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
The diagram below shows parts of the graph of
The line
--- 8 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
Let
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Let
--- 5 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Consider the regions bounded by the graph of
--- 4 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Consider a part of the graph of
--- 5 WORK AREA LINES (style=lined) ---
ii. Given that
Give your answer in simplest form. (1 mark)
--- 4 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
a.i.
a.ii.
| b. | ||
| c. | ||
d.
A tangent to the graph of
This tangent will cross the
A.
B.
C.
D.
E.
Find the equation of the tangent to the curve
--- 7 WORK AREA LINES (style=lined) ---
Let
Find the equation of the tangent to
--- 5 WORK AREA LINES (style=lined) ---
Consider the function
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
The diagram below shows part of the graph of
The tangent cuts the
--- 2 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
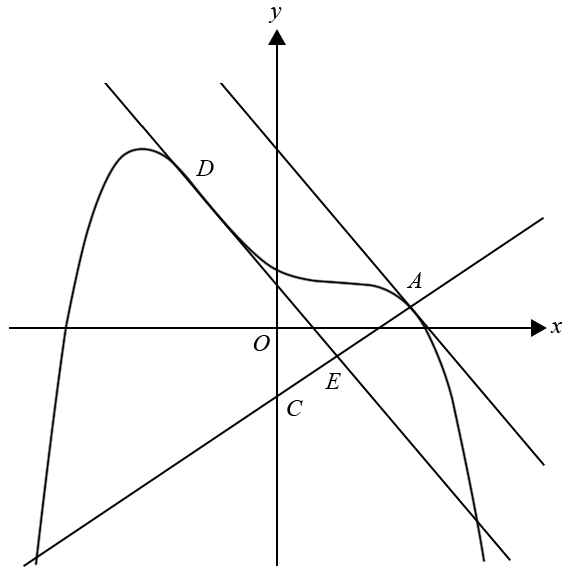
i. Find the coordinates of
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
Let
--- 4 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Find the value of
--- 8 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
a.
b.
c.
d.
e.
f.
Let
--- 10 WORK AREA LINES (style=lined) ---
The line
Find the values of
--- 12 WORK AREA LINES (style=lined) ---
Let
--- 5 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 10 WORK AREA LINES (style=lined) ---
a.i.
| ii. | ||
b.
Let
--- 4 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
a.
| b. | ||
c.i.

c.ii.
d.

e.i.
e.ii.
f.i.
f.ii.
| |
||
| |
||
Let
The tangent at
--- 3 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
ii. Find the coordinates of points
--- 6 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
| a. | ||
b.i.
b.ii.
c.
d.
Part of the graph of a function
--- 5 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
The tangent to the graph of
--- 8 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Trigg the gardener is working in a temperature-controlled greenhouse. During a particular 24-hour time interval, the temperature
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Trigg is designing a garden that is to be built on flat ground. In his initial plans, he draws the graph of
--- 3 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
In further planning for the garden, Trigg uses a transformation of the plane defined as a dilation of factor
--- 8 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Let
--- 0 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
Consider the tangent to the graph of
Which of the following points lies on this tangent?
A.
B.
C.
D.
E.
If the tangent to the graph of
A.
B.
C.
D.
E.