SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Measurement, STD2 M1 2018 HSC 28a

A field is bordered on one side by a straight road and on the other side by a river, as shown. Measurements are taken perpendicular to the road every 7.5 metres along the road.
 

 
Use four applications of the Trapeziodal rule to find an approximation to the area of the field. Answer to the nearest square metre.  (3 marks)

Show Answers Only

²²

Show Worked Solution

 
  ²²

 

  ²

Statistics, STD2 S5 2018 HSC 27e

Joanna sits a Physics test and a Biology test.

  1. Joanna’s mark in the Physics test is 70. The mean mark for this test is 58 and the standard deviation is 8.

     

    Calculate the -score for Joanna’s mark in this test.  (1 mark)

  2. In the Biology test, the mean mark is 64 and the standard deviation is 10.

     

    Joanna’s -score is the same in both the Physics test and the Biology test.

     

    What is her mark in the Biology test?  (2 marks)

Show Answers Only
Show Worked Solution

i.   

 
 

 

ii.   
 
 

Algebra, STD2 A4 2018 HSC 27d

The graph displays the cost () charged by two companies for the hire of a minibus for hours.
 


  

Both companies charge $360 for the hire of a minibus for 3 hours.

  1. What is the hourly rate charged by Company A?  (1 mark)

  2. Company B charges an initial booking fee of $75.

     

    Write a formula, in the form of  , for the cost of hiring a minibus from Company B for hours.  (2 marks)

  3. A minibus is hired for 5 hours from Company B.

     

    Calculate how much cheaper this is than hiring from Company A.  (2 marks)

Show Answers Only
Show Worked Solution
i.   
   

 

ii.   

 

 

iii.   
 

 

Financial Maths, STD2 F4 2018 HSC 26h

A car is purchased for $23 900.

The value of the car is depreciated by 11.5% each year using the declining-balance method.

What is the value of the car after three years?  (2 marks)

Show Answers Only

Show Worked Solution
 
 
 
 

Algebra, STD2 A1 2018 HSC 26b

Clark’s formula, given below, is used to determine the dosage of medicine for children.
 

 
For a particular medicine, the adult dosage is 325 mg and the correct dosage for a specific child is 90 mg.

How much does the child weigh, to the nearest kg?  (2 marks)

Show Answers Only

Show Worked Solution

 
 

Algebra, STD2 A2 2018 HSC 5 MC

The driving distance from Alex's home to his work is 20 km. He drives to and from work five times each week. His car uses fuel at the rate of 8 L/100 km.

How much fuel does he use driving to and from work each week?

  1.  16 L
  2.  20 L
  3.  25 L
  4.  40 L
Show Answers Only

Show Worked Solution


 

Plane Geometry, EXT1 2018 HSC 14c

In triangle is perpendicular to . Side has length , side has length and side has length . A quadrant of a circle of radius , centered at , is constructed. The arc meets side at . It touches the side at , and meets side at . The interval is perpendicular to .
 


 

  1. Show that and are similar.  (1 mark)
  2. Show that
     
    .  (1 mark)
     

  3. From , a line perpendicular to is drawn to meet at , forming the right-angled triangle . A new quadrant is constructed in triangle touching side at . The process is then repeated indefinitely.
     

                
     

  4. Show that the limiting sum of the areas of all the quadrants is
     
      (4 marks)
     

  5. Hence, or otherwise, show that
     
    .  (1 mark)

 

 

Show Answers Only

Show Worked Solution

(i)   


 


 

 

(ii)
   
   
   


(iii) 

♦♦♦ Mean mark part (iii) 19%.

 

 
 
   
 
   
   
 

 

 
 

 

 

(iv) 

♦♦ Mean mark part (iv) 21%.

Mechanics, EXT2* M1 2018 HSC 13c

An object is projected from the origin with an initial velocity of  at an angle    to the horizontal. The equations of motion of the object are

  (Do NOT prove this.)

 

  1. Show that when the object is projected at an angle  , the horizontal range is

     

           (2 marks)

  2. Show that when the object is projected at an angle  , the horizontal range is also 

     

         .  (1 mark)

  3. The object is projected with initial velocity to reach a horizontal distance , which is less than the maximum possible horizontal range. There are two angles at which the object can be projected in order to travel that horizontal distance before landing.

     

    Let these angles be   and  , where 

     

    Let    be the maximum height reached by the object when projected at the angle to the horizontal.

     

    Let    be the maximum height reached by the object when projected at the angle to the horizontal.
     
         
     
    Show that the average of the two heights, , depends only on and .  (3 marks)

Show Answers Only
Show Worked Solution

i.   

 

 
 
 

 

ii. 

 
 

 

iii. 


  

 
 
 

 

♦ Mean mark 44%.
 

 
 
 
 

 

Calculus, EXT1 C2 2018 HSC 12c

Let  .

  1. Show that    (1 mark)

  2. Hence, or otherwise, prove
     
    .  (1 mark)

  3. Hence, sketch
     
    .  (1 mark)

Show Answers Only
Show Worked Solution

i.   

 

ii. 

♦ Mean mark (ii) 37%.

 
   

 

iii. 

♦ Mean mark (iii) 40%.

 

Calculus, EXT1 C1 2018 HSC 12b

A ferris wheel has a radius of 20 metres and is rotating at a rate of 1.5 radians per minute. The top of a carriage is metres above the horizontal diameter of the ferris wheel. The angle of elevation of the top of the carriage from the centre of the ferris wheel is .
 


 

  1. Show that  .  (1 mark)

  2. At what speed is the top of the carriage rising when it is 15 metres higher than the horizontal diameter of the ferris wheel? Give your answer correct to one decimal place.  (2 marks)

Show Answers Only
Show Worked Solution

i.   

 

ii.   

 
 

 

 
   

 

 
 

Functions, EXT1 F2 2018 HSC 11a

Consider the polynomial  .

  1. Show that    is a zero of  .  (1 mark)

  2. Find the other zeros.  (2 marks)

Show Answers Only
Show Worked Solution

i.   

 

ii.   


 

 

 

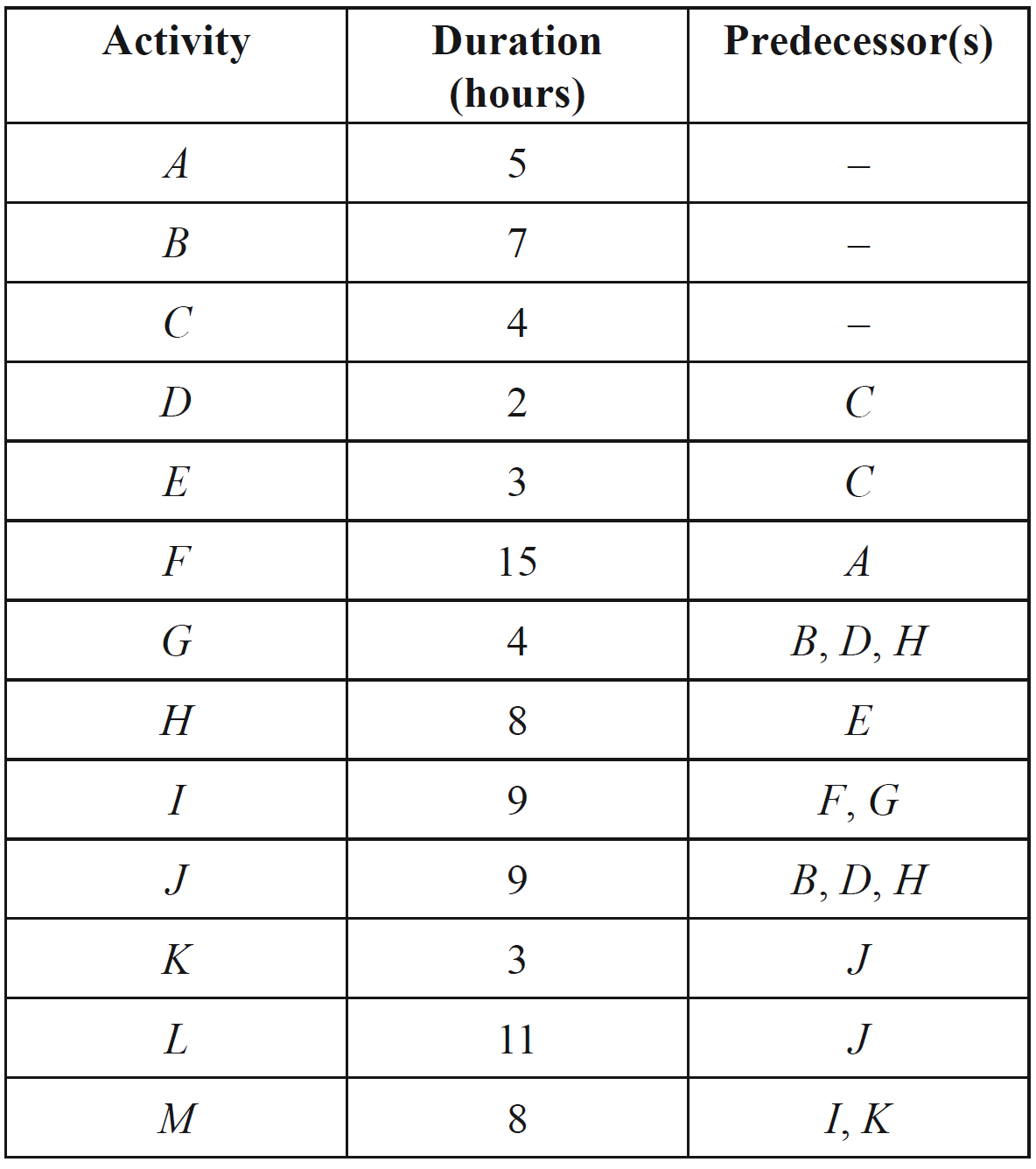
Networks, STD2 N3 2013 FUR2 2

A project will be undertaken in the wildlife park. This project involves the 13 activities shown in the table below. The duration, in hours, and predecessor(s) of each activity are also included in the table.


NETWORKS, FUR2 2013 VCAA 21
 

Activity is missing from the network diagram for this project, which is shown below.

 
NETWORKS, FUR2 2013 VCAA 22
 

  1. Complete the network diagram above by inserting activity .  (1 mark)
  2. Determine the earliest starting time of activity .  (1 mark)

  3. Given that activity  is not on the critical path

     

    1. write down the activities that are on the critical path in the order that they are completed  (1 mark)

    2. find the latest starting time for activity .  (1 mark)

  4. Consider the following statement.

     

    ‘If the time to complete just one of the activities in this project is reduced by one hour, then the minimum time to complete the entire project will be reduced by one hour.’

    Explain the circumstances under which this statement will be true for this project.  (1 mark)

  5. Assume activity  is reduced by two hours.
    What will be the minimum completion time for the project?  (1 mark)

Show Answers Only

a.

networks-fur2-2013-vcaa-2-answer

b. 

c.i.  

c.ii. 

d. 
     

e. 

Show Worked Solution
a.    networks-fur2-2013-vcaa-2-answer

 

b.  


 

c.i.   

♦♦ Mean mark of parts (c)-(e) (combined) was 40%.
 

c.ii.  

 
 

d.  

MARKER’S COMMENT: Most students struggled with part (d).


 

e.  

Networks, STD2 N3 2012 FUR2 2

Thirteen activities must be completed before the produce grown on a farm can be harvested. 

The directed network below shows these activities and their completion times in days.
 


 

  1. Determine the earliest starting time, in days, for activity .  (1 mark)

  2. An activity with zero duration starts at the end of activity .

     

    Explain why this activity is used on the network diagram.  (1 mark)

  3. Determine the earliest starting time, in days, for activity .  (1 mark)

  4. In order, list the activities on the critical path.  (1 mark)

  5. Determine the latest starting time, in days, for activity .  (1 mark)

Show Answers Only




Show Worked Solution
a.   
   
♦ Mean mark of all parts (combined) 47%.

 

b.  


 

♦♦ “Few students” were able to correctly deal with the zero duration activity in part (c).

c.  
 

  

 

d.  
 


 

e.  

MARKER’S COMMENT: A correct calculation based on an incorrect critical path in part (d) gained a consequential mark here. Show your working!.


 

 

Networks, STD2 N3 2006 FUR1 5 MC

For a particular project there are ten activities that must be completed.

These activities and their immediate predecessors are given in the following table.
 

networks-fur1-2006-vcaa-5-mc

 
A directed graph that could represent this project is

 

A.
B.
C.
D.
Show Answers Only

Show Worked Solution


 

Networks, STD2 N2 SM-Bank 13

An estate has large open parklands that contain seven large trees.

The trees are denoted as vertices A to G on the network diagram below.

Walking paths link the trees as shown.

The numbers on the edges represent the lengths of the paths in metres.
 

NETWORKS, FUR2 2007 VCAA 2

 
Jamie is standing at A and Michelle is standing at D.

Write down the shortest route that Jamie can take and the distance travelled to meet Michelle at D.  (1 mark) 

Show Answers Only

Show Worked Solution


 

 

 

Networks, STD2 N2 SM-Bank 2

A school is designing a computer network between five key areas within the school.

The cost of connecting the rooms is shown in the diagram below.
  


 

  1. Complete the spanning tree below that creates the school's network at a minimum cost.  (1 mark)
      


     
     

  2. What is the minimum cost of the network?  (1 mark)

Show Answers Only
  1.  
         
     
Show Worked Solution

i.   


  

 

ii.   
   

Networks, STD2 N2 SM-Bank 1 MC

This diagram shows the possible paths (in km) for laying gas pipes between various locations.
 

 
Gas is to be supplied from one location. Any one of the locations can be the source of the supply.

What is the minimum total length of the pipes required to provide gas to all the locations?

A. 32 km
B. 34 km
C. 36 km
D. 38 km
Show Answers Only

Show Worked Solution


 

 

 


 

Networks, STD2 N2 SM-Bank 14

Water will be pumped from a dam to eight locations on a farm.

The pump and the eight locations (including the house) are shown as vertices in the network diagram below.

The numbers on the edges joining the vertices give the shortest distances, in metres, between locations.
 

NETWORKS, FUR2 2012 VCAA 1

 

  1. How many vertices on the network diagram have an odd degree?  (1 mark)

     

    The total length of all edges in the network is 1180 metres.

     

    The total length of pipe that supplies water from the pump to the eight locations on the farm is a minimum.

     

    This minimum length of pipe is laid along some of the edges in the network.

  2. On the diagram below, draw the minimum length of pipe that is needed to supply water to all locations on the farm.  (2 marks)
     
     
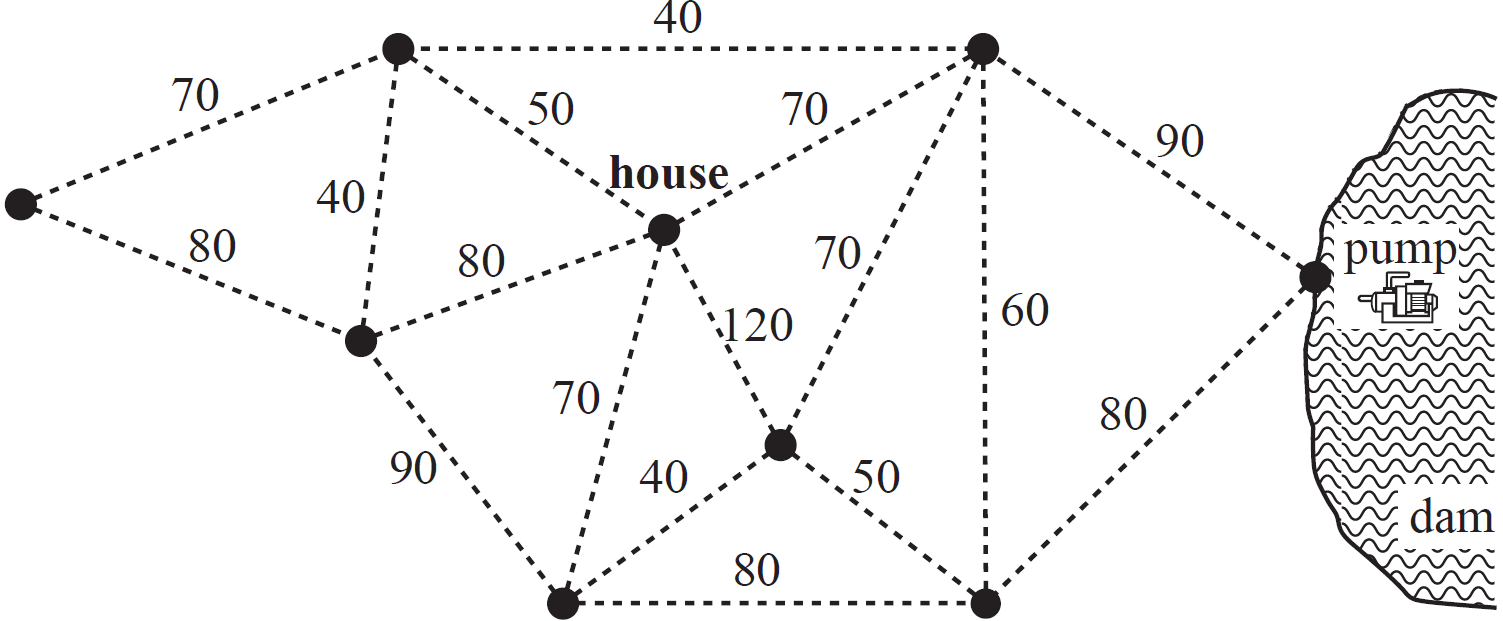
    NETWORKS, FUR2 2012 VCAA 1

  3. What is the mathematical term that is used to describe this minimum length of pipe?  (1 mark)

Show Answers Only

  2.  
    NETWORKS, FUR2 2012 VCAA 1 Answer

Show Worked Solution

i.  

MARKER’S COMMENT: Many students, surprisingly, had problems with part (i).
 

ii. 


 

NETWORKS, FUR2 2012 VCAA 1 Answer


iii.   

Networks, FUR2 2015 VCE 2

The factory supplies groceries to stores in five towns, , , , and , represented by vertices on the graph below.

 

Networks, FUR2 2015 VCAA 2

The edges of the graph represent roads that connect the towns and the factory.

The numbers on the edges indicate the distance, in kilometres, along the roads.

Vehicles may only travel along the road between towns and in the direction of the arrow due to temporary roadworks.

Each day, a van must deliver groceries from the factory to the five towns.

The first delivery must be to town , after which the van will continue on to the other four towns before returning to the factory.

Describe the order in which these deliveries would follow to achieve the shortest possible circuit and the length, in kilometres, of the circuit.  (2 marks)

Show Answers Only

Show Worked Solution

Networks, STD2 N2 2011 FUR2 1

Aden, Bredon, Carrie, Dunlop, Enwin and Farnham are six towns.

The network shows the road connections and distances between these towns in kilometres.
 

  1. In kilometres, what is the shortest distance between Farnham and Carrie?  (1 mark)

  2. How many different ways are there to travel from Farnham to Carrie without passing through any town more than once?  (1 mark)

An engineer plans to inspect all of the roads in this network.

He will start at Dunlop and inspect each road only once.

  1. At which town will the inspection finish?  (1 mark)

Show Answers Only
Show Worked Solution

a.  

 

b.  

 

c.   

Networks, STD2 N2 2010 FUR2 2

The diagram below shows a network of tracks (represented by edges) between checkpoints (represented by vertices) in a short-distance running course. The numbers on the edges indicate the time, in minutes, a team would take to run along each track.

 

Network, FUR2 2011 VCAA 2_1
 

A challenge requires teams to run from checkpoint to checkpoint using these tracks.

What would be the shortest possible time for a team to run from checkpoint to checkpoint ? Mark the shortest route on the diagram below. (2 marks)

 
  Network, FUR2 2011 VCAA 2_2

 
Show Answers Only

Show Worked Solution


 


 

Networks, STD2 N3 2009 FUR2 2

One of the landmarks in a city is a hedge maze. The maze contains eight statues. The statues are labelled to on the following directed graph. Walkers within the maze are only allowed to move in the directions of the arrows.
 

NETWORKS, FUR2 2009 VCAA 2
 

  1. Write down the two statues that a walker could not reach from statue .  (1 mark)

  2. One way that statue can be reached from statue is along path .

     

    List the three other ways that statue can be reached from statue .  (1 mark)

Show Answers Only
Show Worked Solution

a.   

b.  

Networks, STD2 N2 2017 FUR2 1

Bus routes connect six towns.

The towns are Northend (), Opera (), Palmer (), Quigley (), Rosebush () and Seatown ().

The graph below gives the cost, in dollars, of bus travel along these routes.

Bai lives in Northend () and he will travel by bus to take a holiday in Seatown ().
 


 

  1. Bai considers travelling by bus along the route Northend () – Opera () – Seatown ().
    How much would Bai have to pay?  (1 mark)

  2. If Bai takes the cheapest route from Northend () to Seatown (), which other town(s) will he pass through?  (1 mark)

Show Answers Only
Show Worked Solution
a.   
   

 

b.   

 

Networks, STD2 N2 2013 FUR2 1

The vertices in the network diagram below show the entrance to a wildlife park and six picnic areas in the park: , , , , and .

The numbers on the edges represent the lengths, in metres, of the roads joining these locations.

 


 

  1. In this graph, what is the degree of the vertex at the entrance to the wildlife park?  (1 mark)

  2. What is the shortest distance, in metres, from the entrance to picnic area ?  (1 mark)

Show Answers Only
Show Worked Solution

a.  

b.  
 


 

Networks, FUR2 2012 VCE 1

Water will be pumped from a dam to eight locations on a farm.

The pump and the eight locations (including the house) are shown as vertices in the network diagram below.

The numbers on the edges joining the vertices give the shortest distances, in metres, between locations.
 

NETWORKS, FUR2 2012 VCAA 1
 

a.i.  Determine the shortest distance between the house and the pump.  (1 mark)

a.ii. How many vertices on the network diagram have an odd degree?  (1 mark)

The total length of pipe that supplies water from the pump to the eight locations on the farm is a minimum.

This minimum length of pipe is laid along some of the edges in the network.

b.i.  On the diagram below, draw the minimum length of pipe that is needed to supply water to all locations on the farm.  (1 mark)

 

   
  NETWORKS, FUR2 2012 VCAA 1
 

b.ii. What is the mathematical term that is used to describe this minimum length of pipe in part b.i.?  (1 mark)

Show Answers Only

a.i. 

a.ii.

b.i. 

NETWORKS, FUR2 2012 VCAA 1 Answer

b.ii.

Show Worked Solution

a.i.   

MARKER’S COMMENT: Many students, surprisingly, had problems with part (a)(ii).

 

a.ii.  

 

b.i.    NETWORKS, FUR2 2012 VCAA 1 Answer

 

b.ii.   

Networks, STD2 N2 2015 FUR2 1

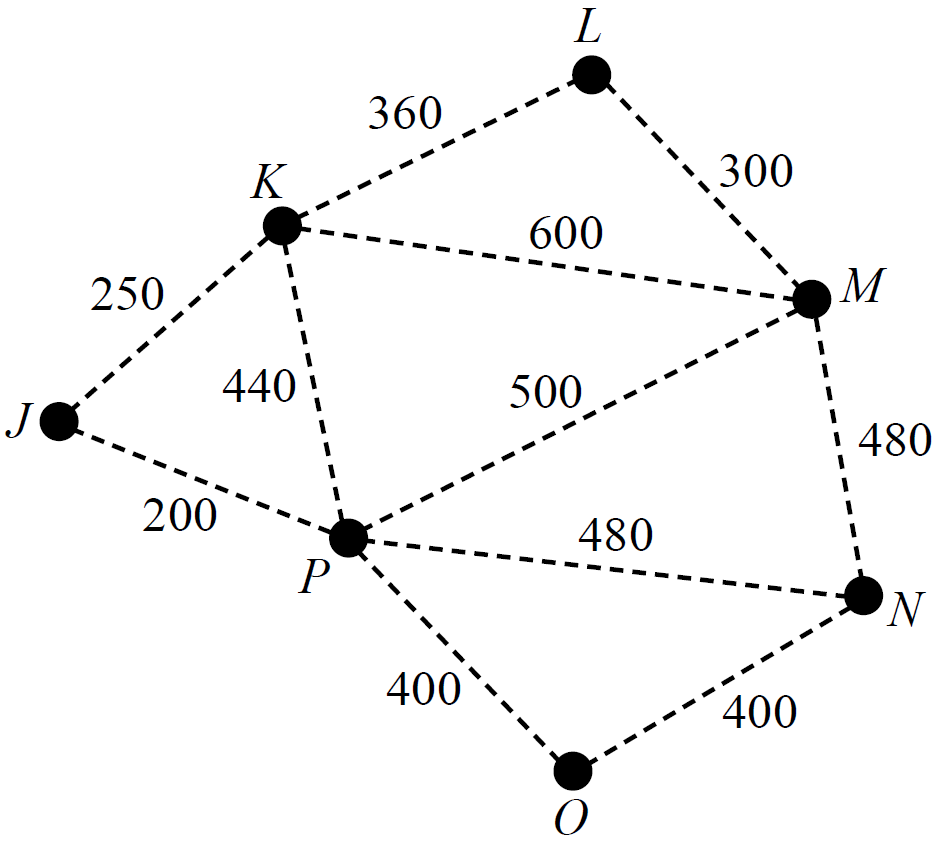
A factory requires seven computer servers to communicate with each other through a connected network of cables.

The servers, , , , , , and , are shown as vertices on the graph below.
 

Networks, FUR2 2015 VCAA 11

 
The edges on the graph represent the cables that could connect adjacent computer servers.

The numbers on the edges show the cost, in dollars, of installing each cable.

  1. What is the cost, in dollars, of installing the cable between server and server ?  (1 mark)

  2. What is the cheapest cost, in dollars, of installing cables between server and server ?  (1 mark)

  3. The computer servers will be able to communicate with all the other servers as long as each server is connected by cable to at least one other server.

    1. The cheapest installation that will join the seven computer servers by cable in a connected network follows a minimum spanning tree.

       

      Draw the minimum spanning tree on the plan below.  (1 mark) 
       

      Networks, FUR2 2015 VCAA 12
       

    2. The factory’s manager has decided that only six connected computer servers will be needed, rather than seven.

       

      How much would be saved in installation costs if the factory removed computer server  from its minimum spanning tree network?

       

      A copy of the graph above is provided below to assist with your working.  (1 mark)

      Networks, FUR2 2015 VCAA 12

Show Answers Only
    1.  
      Networks, FUR2 2015 VCAA 12 Answer
Show Worked Solution

a.   

 

b.  


 

MARKER’S COMMENT: Many students had difficulty finding the minimum spanning tree, often incorrectly excluding or .

c.i.  


 

Networks, FUR2 2015 VCAA 12 Answer


c.ii.   

 

Networks, STD2 N2 2011 FUR2 2

At the Farnham showgrounds, eleven locations require access to water. These locations are represented by vertices on the network diagram shown below. The dashed lines on the network diagram represent possible water pipe connections between adjacent locations. The numbers on the dashed lines show the minimum length of pipe required to connect these locations in metres.
 

NETWORKS, FUR2 2011 VCAA 2 

 
All locations are to be connected using the smallest total length of water pipe possible.

  1. On the diagram, show where these water pipes will be placed.  (1 mark)
  2. Calculate the total length, in metres, of water pipe that is required.  ( 1 mark) 
Show Answers Only
  1.  
    NETWORKS, FUR2 2011 VCAA 2 Answer
Show Worked Solution

a.


 

NETWORKS, FUR2 2011 VCAA 2 Answer


b.  

Networks, STD2 N2 2008 FUR2 1

James, Dante, Tahlia and Chanel are four children playing a game.

In this children’s game, seven posts are placed in the ground.

The network below shows distances, in metres, between the seven posts.

The aim of the game is to connect the posts with ribbon using the shortest length of ribbon.

This will be a minimal spanning tree.

 

NETWORKS, FUR2 2008 VCAA 11

  1. Draw in a minimal spanning tree for this network on the diagram below.  (1 mark)


NETWORKS, FUR2 2008 VCAA 12

  1. Determine the length, in metres, of this minimal spanning tree.  (1 mark)

  2. How many different minimal spanning trees can be drawn for this network?  (1 mark)

Show Answers Only
  1.  


Show Worked Solution

a. 

 
 

 

b.   


 

c.   

Networks, STD2 N2 2013 FUR1 3 MC

 
The vertices of the graph above represent nine computers in a building. The computers are to be connected with optical fibre cables, which are represented by edges. The numbers on the edges show the costs, in hundreds of dollars, of linking these computers with optical fibre cables.

Based on the same set of vertices and edges, which one of the following graphs shows the cable layout (in bold) that would link all the computers with optical fibre cables for the minimum cost?
 

 

vcaa-networks-fur1-2013-3ii

Show Answers Only

Show Worked Solution

Networks, STD2 N2 2017 FUR1 2 MC

Two graphs, labelled Graph 1 and Graph 2, are shown below.
 

 
The sum of the degrees of the vertices of Graph 1 is

  1. two less than the sum of the degrees of the vertices of Graph 2.
  2. one less than the sum of the degrees of the vertices of Graph 2.
  3. equal to the sum of the degrees of the vertices of Graph 2.
  4. two more than the sum of the degrees of the vertices of Graph 2.
Show Answers Only

Show Worked Solution