SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

PATTERNS, FUR1 2012 VCAA 8 MC

The graph above shows consecutive terms of a sequence.

The sequence could be

A.   geometric with common ratio , where  

B.   geometric with common ratio , where  

C.   geometric with common ratio , where  

D.   arithmetic with common difference , where  

E.   arithmetic with common difference , where  

Show Answers Only

Show Worked Solution

♦ Mean mark 50%.

PATTERNS, FUR1 2012 VCAA 7 MC

A dragster is travelling at a speed of 100 km/h.

It increases its speed by

  • 50 km/h in the 1st second
  • 30 km/h in the 2nd second
  • 18 km/h in the 3rd second

and so on in this pattern.

Correct to the nearest whole number, the greatest speed, in km/h, that the dragster will reach is

A.   

B.  

C.   

D.   

E.  

Show Answers Only

Show Worked Solution

   

 

 
 

PATTERNS, FUR1 2012 VCAA 5 MC

On the first day of a fundraising program, three boys had their heads shaved. 

On the second day, each of those three boys shaved the heads of three other boys. 

On the third day, each of the boys who was shaved on the second day shaved the heads of three other boys.

The head-shaving continued in this pattern for seven days. 

The total number of boys who had their heads shaved in this fundraising activity was 

A.   

B.    

C.   

D.   

E.   

Show Answers Only

Show Worked Solution

♦ Mean mark 48%.
   
   
   
 

GEOMETRY, FUR1 2010 VCAA 9 MC

A conical water filter has a diameter of 60 cm and a depth of 24 cm. It is filled to the top with water.

The water filter sits above an empty cylindrical container which has a diameter of 40 cm.

The water is allowed to flow from the water filter into the cylindrical container.

When the water filter is empty, the depth of water in the cylindrical container will be

A.     

B.   

C.   

D.  

E.   

Show Answers Only

Show Worked Solution

♦ Mean mark 48%.

π

π

³

 

π

π
 

GEOMETRY, FUR1 2010 VCAA 8 MC

Dan takes his new aircraft on a test flight.

He starts from his local airport and flies 10 km on a bearing of 045 ° until he reaches his brother’s farm.

From here he flies 18 km on a bearing of 300 ° until he reaches his parents’ farm.

Finally he flies back directly from his parents’ farm to his local airport.

The total distance (in km) that he flies is closest to

A.   

B.   

C.   

D.  

E.   

Show Answers Only

Show Worked Solution

♦ Mean mark 49%.

 

 

GEOMETRY, FUR1 2010 VCAA 7 MC

The diagram below shows a right-triangular prism .

In this prism, , angle and

The size of the angle is closest to

A.   

B.   

C.   

D.  

E.   

Show Answers Only

Show Worked Solution

♦ Mean mark 49%.
 

 

 

PATTERNS, FUR1 2010 VCAA 4 MC

The first four terms of a geometric sequence are 

The sum of the first ten terms of this sequence is

A.   

B.   

C.       

D.     

E.     

Show Answers Only

Show Worked Solution

♦ Mean mark 41%.
 
 

CORE, FUR1 2010 VCAA 7-9 MC

The height (in cm) and foot length (in cm) for each of eight Year 12 students were recorded and displayed in the scatterplot below.
A least squares regression line has been fitted to the data as shown.
 

Part 1

By inspection, the value of the product-moment correlation coefficient for this data is closest to

 

Part 2

The explanatory variable is foot length.

The equation of the least squares regression line is closest to

  1. height = –110 + 0.78 × foot length.
  2. height = 141 + 1.3 × foot length.
  3. height = 167 + 1.3 × foot length.
  4. height = 167 + 0.67 × foot length.
  5. foot length = 167 + 1.3 × height.

 

Part 3

The plot of the residuals against foot length is closest to

CORE, FUR1 2010 VCAA 7-9 MCab

CORE, FUR1 2010 VCAA 7-9 MCcd

CORE, FUR1 2010 VCAA 7-9 MCe

Show Answers Only

Show Worked Solution

 

♦♦ Mean mark 35%.
STRATEGY: An alternate but less efficient strategy could be to find 2 points and calculate the gradient and then use the point gradient formula.

 

CORE, FUR1 2011 VCAA 1-3 MC

The histogram below displays the distribution of the percentage of Internet users in 160 countries in 2007.
 

Part 1

The shape of the histogram is best described as

A.   approximately symmetric.

B.   bell shaped. 

C.   positively skewed.

D.   negatively skewed.

E.   bi-modal.

 

Part 2

The number of countries in which less than 10% of people are Internet users is closest to

A.   

B.   

C.   

D.   

E.   

 

Part 3

From the histogram, the median percentage of Internet users is closest to

A.   

B.   

C.   

D.   

E.   

Show Answers Only

Show Worked Solution

 

 

♦ Mean mark 45%.

Plane Geometry, EXT1 2008 HSC 5c

Inverse Functions, EXT1 2008 HSC 5c

Two circles    and    intersect at    and    as shown in the diagram. The tangent    to    at    meets    at  . The line    meets    at  . The line    meets    at  .

Copy or trace the diagram into your writing booklet.

Prove that    is isosceles.   (3 marks)

Show Answers Only

Show Worked Solution

Inverse Functions, EXT1 2008 HSC 5c Answer

 

 

 

 

 

CORE, FUR1 2014 VCAA 6 MC

The dot plot below shows the distribution of the time, in minutes, that 50 people spent waiting to get help from a call centre.
 

     

Which one of the following boxplots best represents the data?

Show Answers Only

Show Worked Solution

♦ Mean mark 37%.
MARKER’S COMMENT: A majority of students failed to calculate the outer fence where outliers begin, a step required to get the correct answer.

 

 

CORE, FUR1 2012 VCAA 13 MC

A trend line was fitted to a deseasonalised set of quarterly sales data for 2012.

The seasonal indices for quarters 1, 2 and 3 are given in the table below. The seasonal index for quarter 4 is not shown.

 The equation of the trend line is

Using this trend line, the actual sales for quarter 4 in 2012 are predicted to be closest to

A.   

B.   

C.   

D.   

E.   

Show Answers Only

Show Worked Solution

♦ Mean mark 41%.

 

 

CORE, FUR1 2012 VCAA 9 MC

The time series plot below shows the number of days that it rained in a town each month during 2011.
 


 

 Using five-median smoothing, the smoothed time series plot will look most like

Show Answers Only

Show Worked Solution

♦ Mean mark 42%.
MARKERS’ COMMENT: Many students incorrectly gave a “five mean smoothing”.

 

CORE, FUR1 2010 VCAA 1-3 MC

To test the temperature control on an oven, the control is set to 180°C and the oven is heated for 15 minutes.
The temperature of the oven is then measured. Three hundred ovens were tested in this way. Their temperatures were recorded and are displayed below using both a histogram and a boxplot.
 

CORE, FUR1 2010 VCAA 1-3 MC

Part 1

A total of 300 ovens were tested and their temperatures were recorded.

The number of these temperatures that lie between 179°C and 181°C is closest to

A.      

B.      

C.     

D.  

E.   

 

Part 2

The interquartile range for temperature is closest to 

A.     

B.     

C.     

D.    

E.     

 

Part 3

Using the 68–95–99.7%  rule, the standard deviation for temperature is closest to

A.     

B.     

C.     

D.    

E.     

 

Show Answers Only

Show Worked Solution


 

             
 
 

 

 

 
 

 

 

♦ Mean mark 43%.


 

CORE, FUR1 2009 VCAA 9-10 MC

The table below lists the average life span (in years) and average sleeping time (in hours/day) of 12 animal species.
 


 

Part 1

Using sleeping time as the independent variable, a least squares regression line is fitted to the data.

The equation of the least squares regression line is closest to

A.   life span = 38.9 – 2.36 × sleeping time.

B.   life span = 11.7 – 0.185 × sleeping time.

C.   life span = – 0.185 – 11.7 × sleeping time.

D.   sleeping time = 11.7 – 0.185 × life span.

E.   sleeping time = 38.9 – 2.36 × life span.

 

Part 2

The value of Pearson’s product-moment correlation coefficient for life span and sleeping time is closest to

A. 

B. 

C.  

D.   

E.    

Show Answers Only

Show Worked Solution

♦ Mean mark 49%.
MARKERS’ COMMENT: Almost a quarter of students incorrectly assumed the independent variable was in the first column!

 

CORE, FUR1 2008 VCAA 11-13 MC

The time series plot below shows the number of users each month of an online help service over a twelve-month period.
 

2008 11-13

Part 1

The time series plot has

A.   no trend. 

B.   no variability.  

C.   seasonality only.

D.   an increasing trend with seasonality.

E.   an increasing trend only.

 

Part 2

The data values used to construct the time series plot are given below.

2008 12

A four-point moving mean with centring is used to smooth timeline series.
The smoothed value of the number of users in month number 5 is closest to

 

A.   

B.   

C.   

D.   

E.   

 

Part 3

A least squares regression line is fitted to the time series plot.
The equation of this least squares regression line is

number of users = 346 + 2.77 × month number

Let month number 1 = January 2007, month number 2 = February 2007, and so on.

Using the above information, the regression line predicts that the number of users in December 2009 will be closest to

A.   

B.   

C.   

D.   

E.   

Show Answers Only

Show Worked Solution

♦ Mean mark 39%.
MARKERS’ COMMENT: 50% of students incorrectly read the three large random fluctuations in monthly sales as seasonality, which can’t be determined over only 12 months.

 

 

 

 

PATTERNS, FUR1 2013 VCAA 6 MC

There are 3000 tickets available for a concert.

On the first day of ticket sales, 200 tickets are sold.

On the second day, 250 tickets are sold.

On the third day, 300 tickets are sold.

This pattern of ticket sales continues until all 3000 tickets are sold.

How many days does it take for all of the tickets to be sold?

A.  

B.  

C.  

D.  

D.  

Show Answers Only

Show Worked Solution

 

 

 

 
 

CORE, FUR1 2013 VCAA 9 MC

The following data was recorded in an investigation of the relationship between age and reaction time. In this investigation, age is the explanatory variable.
 

CORE, FUR1 2013 VCAA 9 MC

Several statistics were calculated for this data.

When the data was checked, a recording error was found; the age of a 69-year-old had been incorrectly entered as 96. The recording error was corrected and the statistics were calculated. 

The statistics that will remain unchanged when recalculated is the 

A.  slope of the three median line.

B.  intercept of the least squares regression line.

C.  correlation coefficient, .

D.  range of age.

E.  standard deviation of age.

Show Answers Only

Show Worked Solution

♦ Mean mark 47%.

CORE, FUR1 2013 VCAA 3-4 MC

The heights of 82 mothers and their eldest daughters are classified as 'short', 'medium' or 'tall'. The results are displayed in the frequency table below.
 

CORE, FUR1 2013 VCAA 3-4 MC

 
 Part 1

The number of mothers whose height is classified as 'medium' is

 A.    

B.   

C.  

D.  

E.  

 

Part 2

Of the mothers whose height is classified as 'tall', the percentage who have eldest daughters whose height is classified as 'short' is closest to

A.    

B.    

C.  

D.  

E.  

Show Answers Only

Show Worked Solution

       

 

 

♦ Mean mark 45%.
MARKER’S COMMENT: Many students obtained the wrong base of 82 for this percentage calculation.
 
 

 

Quadratic, EXT1 2008 HSC 4c

2008 4c

The points  ,    lie on the parabola  . The tangents to the parabola at    and    intersect at  . The chord    produced meets    at  , and    is a right angle.

  1. Find the gradient of  , and hence show that  .   (2 marks)
  2. The chord    produced meets    at  . Show that    is a right angle.   (1 mark)
  3. Let    be the midpoint of the chord  . By considering the quadrilateral  , or otherwise, show that  .   (2 marks)

 

Show Answers Only
Show Worked Solution

(i) 

 
 

 

 

(ii) 

 
 
 

 

 

(iii)  

 

Combinatorics, EXT1 A1 2008 HSC 4b

Barbara and John and six other people go through a doorway one at a time.

  1. In how many ways can the eight people go through the doorway if John goes through the doorway after Barbara with no-one in between?  (1 mark)

  2. Find the number of ways in which the eight people can go through the doorway if John goes through the doorway after Barbara.   (1 mark)

Show Answers Only
Show Worked Solution

i. 

 

 

ii. 


 

 


 


 


 

Calculus, EXT1 C1 2008 HSC 3c

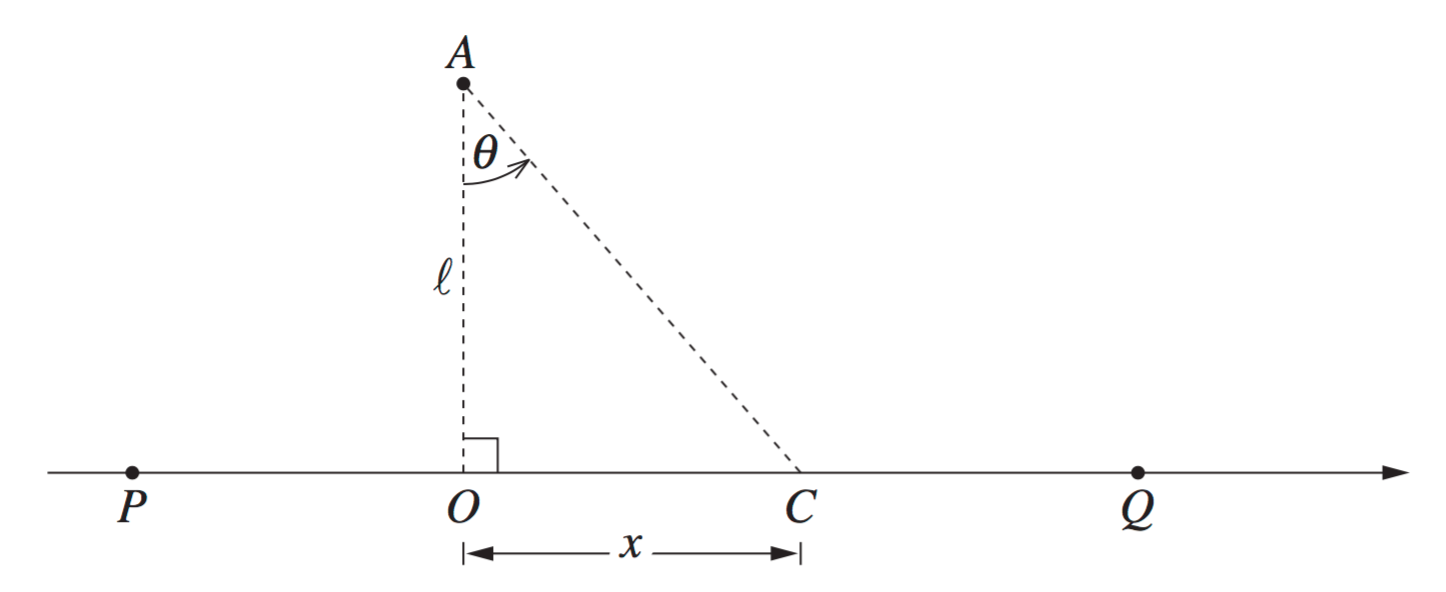
2008 3c
  
 A race car is travelling on the  -axis from    to    at a constant velocity,  .

A spectator is at    which is directly opposite  , and    metres. When the car is at  , its displacement from    is    metres and  , with  .

  1. Show that  .   (2 marks)

  2. Let    be the maximum value of  .  

     

    Find the value of    in terms of    and  .   (1 mark)

  3. There are two values of    for which  .

     

    Find these two values of  .   (2 marks)

Show Answers Only
Show Worked Solution

i. 

 

MARKER’S COMMENT: The most successful students used   and the chain rule to find .
 
 

 

ii. 

 

 

iii. 

MARKER’S COMMENT: Many students lost marks by not giving their answer in the specified range. Be careful!

 

 

Financial Maths, STD2 F5 SM-Bank 3

Camilla buys a car for $21 000 and repays it over 4 years through equal monthly instalments.

She pays a 10% deposit and interest is charged at 9% p.a. on the reducing balance loan.

Using the Table of present value interest factors below, where represents the monthly interest and represents the number of repayments
 

2UG FM5 S-2 

  1. Calculate the monthly repayment,  , that Camilla must pay to complete the loan after 4 years  (to the nearest $).   (3 marks)

  2. Calculate the total interest paid over the life of the loan.    (1 mark)

Show Answers Only
Show Worked Solution

i. 

 

 

 
 

 

 

ii. 


 

Polynomials, EXT1 2008 HSC 2d

The function    has a zero near  .

Use one application of Newton’s method to obtain another approximation to this zero. Give your answer correct to two decimal places.   (3 marks)

Show Answers Only

Show Worked Solution

 

 
 

 

 
 
 

Statistics, STD2 S5 2008 HSC 28a

The following graph indicates  -scores of ‘height-for-age’ for girls aged  5 – 19 years.
 

 
 

  1. What is the  -score for a six year old girl of height 120 cm? (1 mark)

  2. Rachel is 10 ½  years of age. 

     

    (1)  If  2.5% of girls of the same age are taller than Rachel, how tall is she?   (1 mark)

     

    (2)  Rachel does not grow any taller. At age 15 ½, what percentage of girls of the same age will be taller than Rachel?   (2 marks)

  3. What is the average height of an 18 year old girl?   (1 mark)

For adults (18 years and older), the Body Mass Index is given by

 where    in kilograms and    in metres.

The medically accepted healthy range for    is  .

  1. What is the minimum weight for an 18 year old girl of average height to be considered healthy? (2 marks)

  2. The average height, , in centimetres, of a girl between the ages of 6 years and 11 years can be represented by a line with equation
     
               where is the age in years. 
     
    (1)  For this line, the gradient is 6. What does this indicate about the heights of girls aged 6 to 11?   (1 mark)

     

    (2)  Give ONE reason why this equation is not suitable for predicting heights of girls older than 12.   (1 mark)

Show Answers Only
  2. (1)

     

    (2)

  5. (1)

     

         

     

    (2)

     

         

Show Worked Solution
i.   

 

ii. (1)   ½
   
   
     
   (2)   ½
   
   
   
     
   
   
   

 
½

 

iii.  
 

 

iv.  
 

 

 
 

 

v. (1)  
   
  (2)
   

Statistics, STD2 S1 2008 HSC 26d

The graph shows the predicted population age distribution in Australia in 2008.
 

 

  1. How many females are in the 0–4 age group?  (1 mark)

  2. What is the modal age group?   (1 mark)

  3. How many people are in the 15–19 age group?   (2 marks)

  4. Give ONE reason why there are more people in the 80+ age group than in the 75–79 age group.   (1 mark)

Show Answers Only

  5.  

Show Worked Solution
i.   
   

 

ii.   

 

iii.  
   

 

 

 

 

 

iv.  
 

Probability, 2UG 2008 HSC 26c

Joel is designing a game with four possible outcomes. He has decided on three of these outcomes.
 

 VCAA 2008 26c
 

What must be the value of the loss in Outcome 4 in order for the financial expectation of this game to be  $0?   (2 marks)

Show Answers Only

Show Worked Solution


 

 

 

Probability, STD2 S2 2008 HSC 26b

The retirement ages of two million people are displayed in a table.
 

 
 

  1. What is the relative frequency of the 51–55 year retirement age?  (1 mark)

  2. Describe the distribution.   (1 mark)

Show Answers Only

  2.  


     

Show Worked Solution

i. 

 

ii. 

Measurement, STD2 M6 2008 HSC 25c

Pieces of cheese are cut from cylindrical blocks with dimensions as shown.

 

Twelve pieces are packed in a rectangular box. There are three rows with four pieces of cheese in each row. The curved surface is face down with the pieces touching as shown.
  

  1. What are the dimensions of the rectangular box?  (4 marks)

     

    To save packing space, the curved section is removed.
     
             
     

  2. What is the volume of the remaining triangular prism of cheese? Answer to the nearest cubic centimetre.    (2 marks)

Show Answers Only
  2. ³
Show Worked Solution

i. 

♦ Mean mark 45%.

 

 
 

 

 

 

 

ii. 

♦♦♦ Mean mark 22%.

 
 

 

 
  ³

Probability, STD2 S2 2008 HSC 25b

In a drawer there are 30 ribbons. Twelve are blue and eighteen are red.

Two ribbons are selected at random.

  1. Copy and complete the probability tree diagram.  (1 mark)
     

     
  2. What is the probability of selecting a pair of ribbons which are the same colour?  (2 marks)

Show Answers Only
  1.  
Show Worked Solution

i. 

ii. 

 

Probability, STD2 S2 2008 HSC 24b

Three-digit numbers are formed from five cards labelled  1,  2,  3,  4  and  5.

  1. How many different three-digit numbers can be formed?  (1 mark)

  2. If one of these numbers is selected at random, what is the probability that it is odd?   (1 mark)

  3. How many of these three-digit numbers are even?   (1 mark)

  4. What is the probability of randomly selecting a three-digit number less than 500 with its digits arranged in descending order?   (2 marks)

Show Answers Only
Show Worked Solution

i. 

♦ Mean mark 45%.

 

ii. 

 

iii. 

♦ Mean mark 48%.

 

iv. 

♦♦♦ Mean mark 10%.

Statistics, STD2 S1 2008 HSC 23f

Christina has completed three Mathematics tests. Her mean mark is 72%.

What mark (out of 100) does she have to get in her next test to increase her mean mark to 73%?   (2 marks)

Show Answers Only

Show Worked Solution

 

 

 

Probability, STD2 S2 2008 HSC 22 MC

A die has faces numbered 1 to 6. The die is biased so that the number 6 will appear more often than each of the other numbers. The numbers 1 to 5 are equally likely to occur.

The die was rolled 1200 times and it was noted that the 6 appeared 450 times.

Which statement is correct?

  1. The probability of rolling the number 5 is expected to be  .
  2. The number 6 is expected to appear 2 times as often as any other number.
  3. The number 6 is expected to appear 3 times as often as any other number.
  4. The probability of rolling an even number is expected to be equal to the probability of rolling an odd number.
Show Answers Only

Show Worked Solution


 

 


 

 
 

Probability, STD2 S2 2008 HSC 18 MC

New car registration plates contain two letters followed by two numerals followed by two more letters eg  AC 12 DC. Letters and numerals may be repeated.

Which of the following expressions gives the correct number of car registration plates that begin with the letter M?

  1.     
  2.    
  3.    
  4.    
Show Answers Only

Show Worked Solution

Calculus, 2ADV C4 2008 HSC 9c

A beam is supported at    and    as shown in the diagram.
 

2008 9c

 
It is known that the shape formed by the beam has equation  , where    satisfies

  ( is a positive constant) 
and        .

 

  1. Show that  .   (2 marks)

  2. How far is the beam below the  -axis at  ?   (2 marks)

Show Answers Only
Show Worked Solution
i.   
 
 

 

 

 

ii.  
   
   

 

 
 

 

Probability, 2ADV S1 2008 HSC 9a

It is estimated that 85% of students in Australia own a mobile phone.

  1. Two students are selected at random. What is the probability that neither of them owns a mobile phone?  (2 marks)

  2. Based on a recent survey, 20% of the students who own a mobile phone have used their mobile phone during class time. A student is selected at random. What is the probability that the student owns a mobile phone and has used it during class time?   (1 mark)

Show Answers Only
Show Worked Solution

i.     

COMMENT: is syllabus notation for the complement of event .

 
 

 

ii. 

Plane Geometry, 2UA 2008 HSC 8b

In the diagram,    is a parallelogram and    and    are both squares.

Copy or trace the diagram into your writing booklet.

  1. Prove that  .  (1 mark)
  2. Prove that  .   (3 marks)
Show Answers Only
Show Worked Solution

(i) 

 

(ii)

   
 
 

 

Calculus, 2ADV C3 2008 HSC 8a

Let  .

  1. Find the coordinates of the points where the graph of    crosses the axes.  (2 marks)

  2. Show that    is an even function.   (1 mark)

  3. Find the coordinates of the stationary points of    and determine their nature.   (4 marks)

  4. Sketch the graph of  .   (1 mark)

Show Answers Only
  4.  
  5. 2UA HSC 2008 8ai
Show Worked Solution
i.   

 

 

ii.   
 
   
   

 

 

iii.  
 
 

 
   
 

 

   
 

 

iv. 

Probability, 2ADV S1 2008 HSC 7c

Xena and Gabrielle compete in a series of games. The series finishes when one player has won two games. In any game, the probability that Xena wins is    and the probability that Gabrielle wins is  .

Part of the tree diagram for this series of games is shown.
 

 
 

  1. Complete the tree diagram showing the possible outcomes.  (1 mark)
  2. What is the probability that Gabrielle wins the series?   (2 marks)

  3. What is the probability that three games are played in the series?   (2 marks)

Show Answers Only
  1.    
Show Worked Solution
i. 2UA HSC 2008 7ci

 

MARKER’S COMMENT: A tree diagram with 8 outcomes is incorrect (i.e. no third game is played if 1 player wins the first 2 games). If outcomes cannot occur, do not draw them on a tree diagram.

 

ii. 

 

iii. 

 

Trigonometry, 2ADV T1 2008 HSC 7b

2008 7b

The diagram shows a sector with radius    and angle    where  .

The arc length is  . 

  1.  Show that  .   (2 marks)

  2.  Calculate the area of the sector when  .    (2 marks)

Show Answers Only
  2. ²
Show Worked Solution
i.   

 

 

 

ii.  
   
    ²

Trigonometry, 2ADV T2 2008 HSC 6a

Solve    for  .   (3 marks)

Show Answers Only

Show Worked Solution
♦♦ Although exact data not available, markers specifically mentioned this question was poorly answered.

 

MARKER’S COMMENT: Many students had problems adjusting their answer to the given domain, especially when dealing with negative angles.

 

 

 

Quadratic, 2UA 2008 HSC 4c

Consider the parabola  .

  1. Write down the coordinates of the vertex.  (1 mark)
  2. Find the coordinates of the focus.   (1 mark)
  3. Sketch the parabola.   (1 mark)
  4. Calculate the area bounded by the parabola and the line  .   (3 marks)
Show Answers Only

(i)  

(ii)  

(iii)  

(iv)  ²

Show Worked Solution
(i)   

 

(ii)  
 
 

 

(iii) 2UA HSC 2008 4c 

 

(iv)  

 

 
 
 
 
 
  ²

Mechanics, EXT2* M1 2014 HSC 14a

The take-off point on a ski jump is located at the top of a downslope. The angle between the downslope and the horizontal is  .  A skier takes off from with velocity m s−1 at an angle to the horizontal, where  .  The skier lands on the downslope at some point , a distance metres from .
 

2014 14a
 

The flight path of the skier is given by

,      (Do NOT prove this.)

where    is the time in seconds after take-off.

  1. Show that the cartesian equation of the flight path of the skier is given by
     
         .   (2 marks)

  2. Show that  
     
         .   (3 marks)

  3. Show that  
     
         .   (2 marks)

  4. Show that has a maximum value and find the value of for which this occurs.   (3 marks)

Show Answers Only
Show Worked Solution

i. 

 
 

 

ii. 

♦♦ Mean mark 28%
MARKER’S COMMENT: The key to finding and solving for is to realise you need the intersection of the Cartesian equation in (i) and the line .

 

 

 

 

iii. 

 
 

 

iv. 

♦ Mean mark 47%
IMPORTANT: Note that students can attempt every part of this question, even if they couldn’t successfully prove earlier parts.
 

 

 
 

 

Plane Geometry, EXT1 2014 HSC 13d

In the diagram,    is a diameter of a circle with centre  . The point    is chosen such that    is acute-angled. The circle intersects    and    at    and    respectively.

Copy or trace the diagram into your writing booklet.

  1. Why is   ?   (1 mark)
  2. Show that the line    is a tangent to the circle through  ,    and  .    (2 marks)
Show Answers Only
Show Worked Solution
(i)   

 

(ii)  

♦ Mean mark 37%
COMMENT: Labelling angles and etc.. can often make a proof clearer and less messy.

 

Polynomials, EXT1 2014 HSC 12e

The diagram shows the graph of a function  .

The equation    has a root at  . The value , as shown in the diagram, is chosen as a first approximation of .
 

 
 

A second approximation, , of is obtained by applying Newton’s method once, using    as the first approximation.

Using a diagram, or otherwise, explain why is a closer approximation of than .   (1 mark) 

Show Answers Only

Show Worked Solution

♦ Mean mark 47%
COMMENT: A Newton approximation can actually be worse in certain circumstances.

 

Plane Geometry, EXT1 2009 HSC 7c

Consider the billboard below. There is a unique circle that passes through the top and bottom of the billboard (points    and    respectively) and is tangent to the street at  .

Let    be the angle subtended by the billboard at  , the point where    intersects the circle.

Copy the diagram into your writing booklet. 

  1. Show that    when    and    are different points, and hence show that    is a maximum when    and    are the same point.   (3 marks)
  2. Using circle properties, find the distance of    from the building.     (1 mark)
Show Answers Only
Show Worked Solution
(i) 
♦♦ Both parts proved extremely challenging for students.
MARKER’S COMMENT: Very few students recognised that is the external angle of .

 

 

(ii)  

 

Geometry and Calculus, EXT1 2009 HSC 7b

A billboard of height    metres is mounted on the side of a building, with its bottom edge    metres above street level. The billboard subtends an angle    at the point  ,    metres from the building.
 

 
 

  1. Use the identity    to show that
  2.  
    1. .   (2 marks)
    2.  
  4. The maximum value of    occurs when    and    is positive.
  5.  
  6. Find the value of    for which    is a maximum.     (3 marks)

 

Show Answers Only
Show Worked Solution

(i)   

♦♦ Mean mark data not available for parts of questions although Q7 as a whole scored <30%.
MARKER’S COMMENT: Answers that included a diagram and clearly labelled angles were generally successful.
 
 

 

 

(ii)  

 

 

 
 
 

 

Binomial, EXT1 2009 HSC 6b

  1. Sum the geometric series  
  3.  
  4. and hence show that
     
    1. .   (3 marks)
    2.  
  6. Consider a square grid with    rows and    columns of equally spaced points.
  7.  
    1. 2009 6b
  8. The diagram illustrates such a grid. Several intervals of gradient  , whose endpoints are a pair of points in the grid, are shown. 
  9.  
  10. (1)   Explain why the number of such intervals on the line    is equal to  .   (1 mark)
  11. (2)   Explain why the total number,  , of such intervals in the grid is given by
  12.  
      1. .   (1 mark)
    2.  
  14. Using the result in part (i), show that 
    1. .   (3 marks)

 

 

Show Answers Only
  2. (1)
  3. (2)
  4.  
Show Worked Solution

(i)   

♦♦ Exact data unavailable although mean marks for all Q6 was < 25%.
MARKER’S COMMENT: A common mistake was to assume the number of terms in the series was  .

 

 
 
 

 

 

 

 

 

 

(ii)(1)  
 
 
   
     (2)  
 
 
 
 
 

 

 
 

 

♦♦♦ Exact data unavailable although very few completed part (iii) correctly. 
MARKER’S COMMENT: Note that even if part (i) wasn’t solved, the proof as stated in the question can be used to solve part (iii).
(iii)
   
 
 
 
 
 

Calculus, EXT1 C1 2009 HSC 5b

The cross-section of a 10 metre long tank is an isosceles triangle, as shown in the diagram. The top of the tank is horizontal.
 

 
 

When the tank is full, the depth of water is 3 m. The depth of water at time days is metres.   

  1. Find the volume, , of water in the tank when the depth of water is metres.     (1 mark)

  2. Show that the area, , of the top surface of the water is given by  .   (1 mark)

  3. The rate of evaporation of the water is given by  , where is a positive constant. 

     

    Find the rate at which the depth of water is changing at time .   (2 marks)

  4. It takes 100 days for the depth to fall from 3 m to 2 m. Find the time taken for the depth to fall from 2 m to 1 m.   (1 mark)

Show Answers Only
  1. ³
Show Worked Solution
MARKER’S COMMENT: Students who drew a diagram and included their working calculations on it were the most successful.
(i) 
 
 
  ²

 

 
  ³

 

(ii)   
 
  ²

 

(iii)   
   

 

MARKER’S COMMENT: Half marks awarded for stating an appropriate chain rule, even if the following calculations were incorrect. Show your working!
 
 

 

 

♦♦♦ Exact data for part (iv) not available.
COMMENT: Interpreting a constant rate of change was very poorly understood!
(iv)   
 

 

Mechanics, EXT2* M1 2009 HSC 5a

The equation of motion for a particle moving in simple harmonic motion is given by

where    is a positive constant,    is the displacement of the particle and    is time.  

  1. Show that the square of the velocity of the particle is given by
     
         

     

    where    and    is the amplitude of the motion.   (3 marks)

  2. Find the maximum speed of the particle.     (1 mark)

  3. Find the maximum acceleration of the particle.    (1 mark)

  4. The particle is initially at the origin. Write down a formula for    as a function of  , and hence find the first time that the particle’s speed is half its maximum speed.   (2 marks)

Show Answers Only
Show Worked Solution
i.   

 

 
 

 

ii.   
 

 

iii.  
 

 

iv.  
 

 

Geometry and Calculus, EXT1 2009 HSC 4b

Consider the function  . 

  1. Show that    is an even function.    (1 mark)
  2. What is the equation of the horizontal asymptote to the graph  ?    (1 mark)
  3. Find the  -coordinates of all stationary points for the graph  .   (3 marks)
  4. Sketch the graph  . You are not required to find any points of inflection.    (2 marks)
Show Answers Only
  5.  
Show Worked Solution
(i)   
 
   
   

 

 

(ii)   
   
 

 

 

(iii)  
 
 
 
 

 

MARKER’S COMMENT: Many students did not realise the denominator of   could be ignored when equating  .

 

 

 

(iv)   
 

 

EXT1 2009 4b

Statistics, EXT1 S1 2009 HSC 4a

A test consists of five multiple-choice questions. Each question has four alternative answers. For each question only one of the alternative answers is correct.

Huong randomly selects an answer to each of the five questions. 

  1. What is the probability that Huong selects three correct and two incorrect answers?   (2 marks)

  2. What is the probability that Huong selects three or more correct answers?    (2 marks)

  3. What is the probability that Huong selects at least one incorrect answer?  (1 mark)

Show Answers Only
Show Worked Solution

i.   


 

ii. 


 

iii. 

TIP: The use of “at least” should flag a good chance of applying to solve.