SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Calculus, 2ADV C4 2018* HSC 15c

The shaded region is enclosed by the curve    and the line  , as shown in the diagram. The line    meets the curve    at    and  . Do NOT prove this.
 


 

  1.  Use integration to find the area of the shaded region.  (2 marks)

  2. Use the Trapezoidal rule and four function values to approximate the area of the shaded region.  (2 marks)

The point is chosen on the curve    so that the tangent at is parallel to the line    and the -coordinate of is positive

  1.  Show that the coordinates of are  .  (2 marks)

  2.  Using the perpendicular distance formula  ,  find the area of  .  (2 marks)

Show Answers Only
  1. ²
  2. ²
  4. ²
Show Worked Solution
i.  
   
   
   
   
    ²

 

ii. 

 
  ²

 

iii.   

 

 
 

 

 

iv.  

 

 
 

 

 
   
  ²

Calculus, 2ADV C4 2017* HSC 14b

  1. Find the exact value of  .  (1 mark)

  2. Using the Trapezoidal rule with three function values, find an approximation to the integral  leaving your answer in terms of and .  (2 marks)

  3. Using parts (i) and (ii), show that  .  (1 mark)

Show Answers Only
Show Worked Solution
i.  
   
   

 

ii.  

 
 

 

♦ Mean mark part (iii) 49%.

(iii)   
 
   

Calculus, 2ADV C4 2011* HSC 5c

The table gives the speed of a jogger at time in minutes over a  20-minute period. The speed is measured in metres per minute, in intervals of 5 minutes.

The distance covered by the jogger over the 20-minute period is given by  .

Use the Trapezoidal rule and the speed at each of the five time values to find the approximate distance the jogger covers in the 20-minute period.   (3 marks)

Show Answers Only

 

Show Worked Solution
 
 

Calculus, 2ADV C4 SM-Bank 07

The diagram shows a block of land and its dimensions, in metres. The block of land is bounded on one side by a river. Measurements are taken perpendicular to the line  , from    to the river, at equal intervals of 50 m.
 

Integration, 2UA 2009 HSC 3d

 
Use the Trapezoidal rule with six subintervals to find an approximation to the area of the block of land.   (3 marks)

Show Answers Only

 ²

Show Worked Solution

 

 
  ²

 
²

Trigonometry, 2ADV’ T1 2004 HSC 3d

Trig Ratios, EXT1 2004 HSC 3d
 

The length of each edge of the cube    is 2 metres. A circle is drawn on the face    so that it touches all four edges of the face. The centre of the circle is    and the diagonal    meets the circle at    and  .

  1. Explain why  .  (1 mark)

  2. Show that   metres.  (1 mark)

  3. Calculate the size of    to the nearest degree.  (1 mark)

Show Answers Only
Show Worked Solution
i.   

Trig Ratios, EXT1 2004 HSC 3d Answer

 

ii.   

Trig Ratios, EXT1 2004 HSC 3d Answer2

 
 
 

 

 

 

iii.

Trig Ratios, EXT1 2004 HSC 3d Answer3

 

 
 

Trigonometry, 2ADV’ T1 2010 HSC 5a

A boat is sailing due north from a point    towards a point    on the shore line.

The shore line runs from west to east.

In the diagram,    represents a tree on a cliff vertically above  , and    represents a landmark on the shore. The distance    is 1 km.

From    the point    is on a bearing of 020°, and the angle of elevation to    is 3°.

After sailing for some time the boat reaches a point  , from which the angle of elevation to    is 30°.
 

5a
 

  1. Show that  .   (3 marks) 

  2. Find the distance . Give your answer to 1 decimal place.   (1 mark)

Show Answers Only
Show Worked Solution

i.    

 

 

 

 

 

ii.   
 
 
 
 

Trigonometry, 2ADV’ T1 2008 HSC 6a

From a point    due south of a tower, the angle of elevation of the top of the tower  , is 23°. From another point  , on a bearing of 120° from the tower, the angle of elevation of    is 32°. The distance    is 200 metres.
 

Trig Ratios, EXT1 2008 HSC 6a 
 

  1. Copy or trace the diagram into your writing booklet, adding the given information to your diagram.  (1 mark)

  2. Hence find the height of the tower. Give your answer to the nearest metre.  (3 marks)

Show Answers Only
  1.  
    Trig Ratios, EXT1 2008 HSC 6a Answer

Show Worked Solution

i.

Trig Ratios, EXT1 2008 HSC 6a Answer 

 

ii. 

 

 


 

 
 
 
 

Trigonometry, 2ADV’ T1 2015 HSC 12c

A person walks 2000 metres due north along a road from point to point . The point is due east of a mountain , where is the top of the mountain. The point is directly below point and is on the same horizontal plane as the road. The height of the mountain above point is metres.

From point , the angle of elevation to the top of the mountain is 15°.

From point , the angle of elevation to the top of the mountain is 13°.
 

Trig Ratios, EXT1 2015 HSC 12c
 

  1. Show that  .  (1 mark)

  2. Hence, find the value of  .  (2 marks)

Show Answers Only
Show Worked Solution

i.    

Trig Ratios, EXT1 2015 HSC 12c Answer1

 

 

ii.   

 

 

Trig Ratios, EXT1 2015 HSC 12c Answer2 
 
 
 

Measurement, STD2 M6 2011 HSC 24c

A ship sails 6 km from to on a bearing of 121°. It then sails 9 km to .  The
size of angle is 114°.
 

  1. What is the bearing of from ?   (1 mark)

  2. Find the distance . Give your answer correct to the nearest kilometre.   (2 marks)

  3. What is the bearing of from ? Give your answer correct to the nearest degree.   (3 marks)

Show Answers Only
  1.  
  2.  
  3.  
Show Worked Solution
STRATEGY: Important: Draw North-South parallel lines through major points to make the angle calculations easier!
i.     2011 HSC 24c

 

   

 

ii.   

 
 
 

 

iii.   

MARKER’S COMMENT: The best responses clearly showed what steps were taken with working on the diagram. Note that all North/South lines are parallel.
 
 

 

 
 
 

Trigonometry, 2ADV* T1 2009 HSC 27b

A yacht race follows the triangular course shown in the diagram. The course from    to    is 1.8 km on a true bearing of 058°.

At    the course changes direction. The course from    to    is 2.7 km and  .
 

 2009-2UG-27b
 

  1. What is the bearing of    from  ?   (1 mark)

  2. What is the distance from    to  ?     (2 marks)

  3. The area inside this triangular course is set as a ‘no-go’ zone for other boats while the race is on.

     

    What is the area of this ‘no-go’ zone?   (1 mark)

Show Answers Only
  3. ²
Show Worked Solution
i.    2UG-2009-27b-Answer

TIP: Draw North-South parallel lines through relevant points to help calculate angles as shown in the Worked Solutions.

 

(ii)   

 +  
   +  
 
 
 
 

 

(iii)   

 
  ²

 

²

Trigonometry, 2ADV* T1 2014 HSC 23 MC

The following information is given about the locations of three towns , and : 
 

• is due east of 

• is on a bearing of    from   

• is on a bearing of    from  . 

 
Which diagram best represents this information?
 

HSC 2014 23mci

Show Answers Only

Show Worked Solution
COMMENT: Drawing a parallel North/South line through makes this question much simpler to solve.


 

 


 

Measurement, STD2 M1 2007 HSC 28c*

A piece of plaster has a uniform cross-section, which has been shaded, and has dimensions as shown.
 

 
 

  1. Use the Trapezoidal rule to approximate the area of the cross-section.    (3 marks)

  2. The total surface area of the piece of plaster is 7480.8 cm²
  3. Calculate the area of the curved surface as shown on the diagram. Give your answer to the nearest square centimetre   (2 marks)

Show Answers Only
  1. ²
  2. ²
Show Worked Solution
i.   
 
  ²

 

ii.  ²

  ²
  ²
  ²

 

²²

Measurement, STD2 M1 2017 HSC 29a*

A new 200-metre long dam is to be built.

The plan for the new dam shows evenly spaced cross-sectional areas.
 
 


 

  1. Using the Trapezoidal rule, show that the volume of the dam is approximately 44 500 m³.  (2 marks)

  2. It is known that the catchment area for this dam is 2 km².

     

    Assuming no wastage, calculate how much rainfall is needed, to the nearest mm, to fill the dam.  (2 marks)

Show Answers Only
Show Worked Solution
i.   
 
  ³

 

ii.   ²²

♦♦♦ Mean mark 11%.
²
  ²

 

 
 
 

Measurement, STD2 M1 2008 HSC 28b*

A tunnel is excavated with a cross-section as shown.

 

  1. Find an expression for the area of the cross-section using the Trapezoidal rule.  (2 marks)

  2. The area of the cross-section must be 600 m2. The tunnel is 80 m wide. 

     

    If the value of increases by 2 metres, by how much will change?   (2 marks)

Show Answers Only
Show Worked Solution
i.   
 
 

 

ii.   ²

 

 

Measurement, STD2 M1 2014 HSC 28d*

An aerial diagram of a swimming pool is shown. 

The swimming pool is a standard length of 50 metres but is not in the shape of a rectangle.

In the diagram of the swimming pool, the five widths are measured to be: 
 

 
 

  1. Use four applications of the Trapezoidal Rule to calculate the surface area of the pool.  (2 marks)

  2.  The average depth of the pool is 1.2 m

     

    Calculate the approximate volume of the swimming pool, in litres.  (1 mark)

Show Answers Only
  1. ²
Show Worked Solution
i.   

²
 

♦ Mean mark 50%. Be careful not to give away easy marks! 
ii.   
   
    ³
   

Measurement, STD2 M1 2009 HSC 25c*

There is a lake inside the rectangular grass picnic area  , as shown in the diagram.
 

2UG-2009-25c
  

  1. Use Trapezoidal’s Rule to find the approximate area of the lake’s surface.   (3 marks)

The lake is 60 cm deep. Bozo the clown thinks he can empty the lake using a four-litre bucket.

  1. How many times would he have to fill his bucket from the lake in order to empty the lake? (Note that 1 m³ = 1000 L).    (2 marks)

Show Answers Only
  1. 426 m²
  2. 63 900 times
Show Worked Solution
i.    
  ²

 

²

 

  ²

 

♦ Mean mark 44%
STRATEGY: Most students who did calculations in cm² and cm³ made errors. Keeping calculations in metres is much easier here.
ii.   
   
    ³
    ³

 

 

Measurement, STD2 M1 SM-Bank 14

Cassius is a boxer and skips to keep fit.

The table below shows the average energy used, in kilojuoles per kilogram of body mass, by a person skipping for 10 minutes at different speeds.

   

Cassius eats a hamburger that contains 550 kilocalories.

If Cassuis weighs 72 kilograms, how long must he skip at 150 skips per minute to burn off the energy contained in the hamburger? (1 kilocalorie = 4.184 kJ) Give your answer to 1 decimal place.  (3 marks)

Show Answers Only

Show Worked Solution


 

Measurement, STD2 M1 SM-Bank 13 MC

The table shows the average energy used, in kilojoules per kilogram of body mass, by a person walking for 30 minutes at different speeds.

Rob, who weighs 90 kg, drinks a large cappuccino made with full cream milk. It contains 146 kilocalories.

For approximately how long must Rob walk at 3 km/hr to burn off the energy contained in the cappuccino? (1 kilocalorie = 4.184 kilojoules)

  1. 28 minutes
  2. 37 minutes
  3. 83 minutes
  4. 110 minutes
Show Answers Only

Show Worked Solution


 


 

 

Measurement, STD2 M1 SM-Bank 12

The scale diagram shows the aerial view of a block of land bounded on one side by a road. The length of the block, , is known to be 90 metres.
 


 

Calculate the approximate area of the block of land, using three applications of the Trapezoidal rule.  (3 marks)

Show Answers Only

5175 m²

Show Worked Solution


 

 
  ²

 

  ²

Proof, EXT2 P1 2018 HSC 15c

Let    be a positive integer and let    be a positive real number.

  1.  Show that  .  (1 mark)

  2.  Hence, show that  .  (2 marks)

  3.  Deduce that for positive real numbers and ,
     
            (2 marks)

Show Answers Only
Show Worked Solution
i.   
   
   
   

 

ii.   

♦♦♦ Mean mark 11%.


 


 


 

 

iii.   

♦♦ Mean mark 23%.

Complex Numbers, EXT2 N2 2018 HSC 15b

  1. Use De Moivre's theorem and the expansion of to show that
     

     
                          (2 marks)

  2. Hence, show that
     
    .  (3 marks)

Show Answers Only
Show Worked Solution

i.   


 

 


 

ii.   
   

 

Harder Ext1 Topics, EXT2 2018 HSC 15a

The point  , where  , lies on the ellipse  , where . The point    lies vertically above on the auxiliary circle  . The point lies on the auxiliary circle such that    and the point lies on the ellipse vertically below , as shown.
 

  1. Show that has coordinates  .  (2 marks)
     

The line meets the ellipse again at  .   (Do NOT prove this.)

  1. Show that the minimum size of    is  .  (3 marks)
Show Answers Only
Show Worked Solution

i.   


 

 

 

 

 

 

ii.   

♦ Mean mark 38%.

 


 

 
 
 
 
 
 

 


 

Mechanics, EXT2 2018 HSC 13c

A particle of mass is attached to a light inextensible string of length . The string makes an angle to the vertical.

The particle is moving in a circle of radius on a smooth horizontal surface. The particle is moving with uniform angular velocity ω. The forces on the particle are the tension in the string; the normal reaction to the horizontal surface; and the gravitational force  .
 

 
The particle remains in contact with the horizontal surface.

By resolving the forces on the particle in the horizontal and vertical directions, show that

ω.   (3 marks)

Show Answers Only

Show Worked Solution

ω

 

ω


 

ω
ω
ω

 

ω
ω
ω
ω

Volumes, EXT2 2018 HSC 13a

The graph  is shown.
 

 
Use the method of cylindrical shells to find the volume of the solid formed when the shaded region is rotated about the line  .  (3 marks)

Show Answers Only

³

Show Worked Solution

 

δ δ
  δ
  δ

 

 
 
 
 
 
  ³

Volumes, EXT2 2018 HSC 12a

The base of a solid is the region enclosed by the parabola    and the -axis. Each cross-section perpendicular to the -axis is an equilateral triangle, as shown in the diagram.
 

 
Find the volume of the solid.  (3 marks)

Show Answers Only

³

Show Worked Solution

 

δ δ
  δ

 

 
 
 
 
  ³

Complex Numbers, EXT2 N2 2018 HSC 11d

The points , and on the Argand diagram represent the complex numbers , and respectively.

The points , , and form a square as shown on the diagram.
 

 
It is given that  .

  1.  Find  .  (1 mark)

  2.  Find  .  (1 mark)

  3.  Find  .  (1 mark)

Show Answers Only
Show Worked Solution
i.   
   
   

 

ii.   
   
   

 

iii.   
   

Harder Ext1 Topics, EXT2 2018 HSC 10 MC

Consider the functions    and  .

The -coordinate of each stationary point of    is very close to the -coordinate of a stationary point of .

Suppose    has a stationary point at    and the stationary point of    with -coordinate closest to    is at  .

Which statement is always true?

Show Answers Only

Show Worked Solution

Mean mark 57%. A challenging question well answered. 

 


 

Integration, 2UA 2018 HSC 15c

The shaded region is enclosed by the curve    and the line  , as shown in the diagram. The line    meets the curve    at    and  . Do NOT prove this.
 


 

  1. Use integration to find the area of the shaded region.  (2 marks)
  2. Verify that one application of Simpson’s rule gives the exact area of the shaded region.  (2 marks)
     
  3. The point is chosen on the curve    so that the tangent at is parallel to the line    and the -coordinate of is positive.
     
  4. Show that the coordinates of are  .  (2 marks)
  5. Find the area of  .  (2 marks)
Show Answers Only
  1. ²

  4. ²
Show Worked Solution
(i)  
   
   
   
   
    ²

 

(ii) 

♦ Mean mark 45%.


 

 
 
 

 

(iii)   

 

 
 

 

 

(iv)  

 

 
 

 

♦♦ Mean mark 27%.

 
   
  ²

Calculus, 2ADV C3 2018 HSC 16a

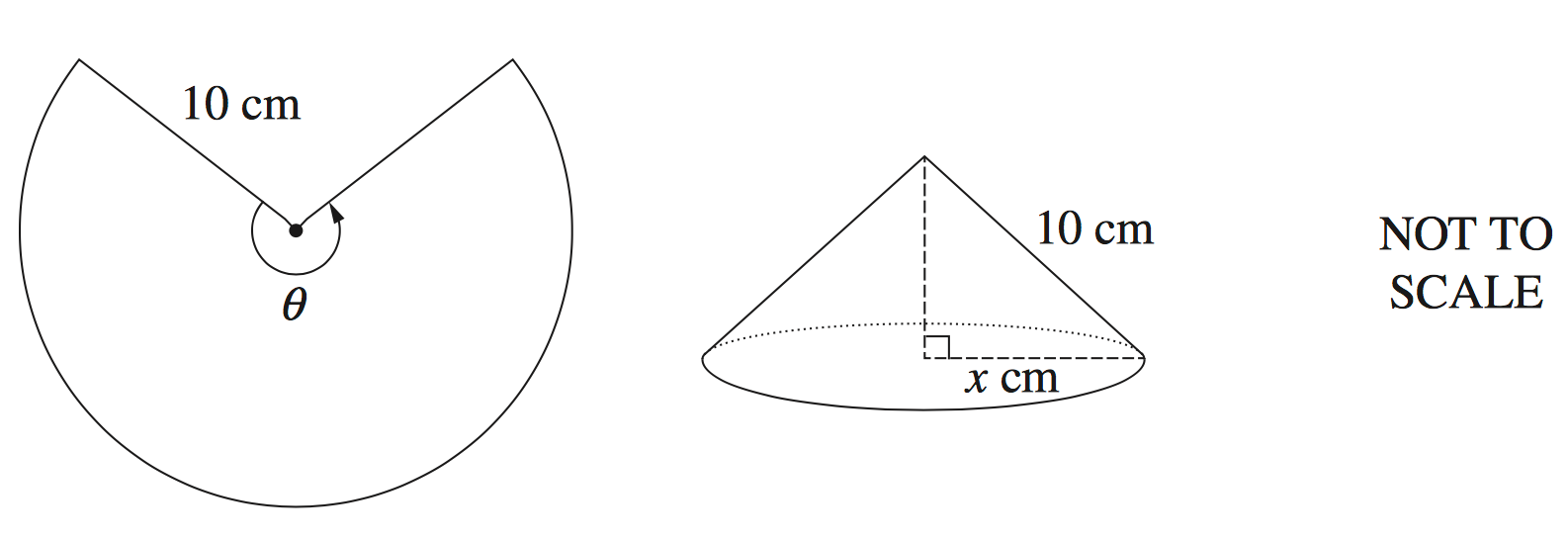
A sector with radius 10 cm and angle    is used to form the curved surface of a cone with base radius cm, as shown in the diagram.
 


 

The volume of a cone of radius and height is given by  .

  1. Show that the volume, cm³, of the cone described above is given by
     
          .   (1 mark)

  2. Show that  .   (2 marks)

  3. Find the exact value of    for which is a maximum.   (3 marks)

Show Answers Only
Show Worked Solution

i.   


 

♦ Mean mark (ii) 45%.

ii.  
 
   
   
   

 

iii. 

♦♦ Mean mark (iii) 23%.

 


 

 

Calculus, 2ADV C4 2018 HSC 15b

The diagram shows the region bounded by the curve    and the lines  ,    and  . The region is divided into two parts of equal area by the line  , where is a positive integer. 
 

 
What is the value of the integer , given that the two parts have equal areas?  (3 marks)

Show Answers Only

Show Worked Solution
 
 
 

 

 

Trigonometry, 2ADV T3 2018 HSC 15a

The length of daylight, , is defined as the number of hours from sunrise to sunset, and can be modelled by the equation

,

where is the number of days after 21 December 2015, for  .

  1. Find the length of daylight on 21 December 2015.   (1 mark)

  2. What is the shortest length of daylight?  (1 mark)

  3. What are the two values of    for which the length of daylight is 11?  (2 marks)

Show Answers Only

Show Worked Solution

i.   

 

 

ii.   

♦ Mean mark 43%.


 

 

 

iii.   


 

   
 
     

 

Probability, 2ADV S1 2018 HSC 14e

Two machines, and , produce pens. It is known that 10% of the pens produced by machine are faulty and that 5% of the pens produced by machine are faulty.

  1. One pen is chosen at random from each machine.

     

    What is the probability that at least one of the pens is faulty?  (1 mark)

  2. A coin is tossed to select one of the two machines. Two pens are chosen at random from the selected machine.

     

    What is the probability that neither pen is faulty?  (2 marks)

Show Answers Only

Show Worked Solution
i.  
   
   
   

 

ii.   

♦ Mean mark 48%.

Calculus, EXT1* C3 2018 HSC 14b

The shaded region shown in the diagram is bounded by the curve  , the -axis and the line  .
  


 

Find the volume of the solid of revolution formed when the shaded region is rotated about the -axis.  (3 marks)

Show Answers Only

²

Show Worked Solution


 

 
 
 
 
  ²

Trigonometry, 2ADV T1 2018 HSC 14a

In    has length 3, has length 6 and is 60°. The point is chosen on side    so that    bisects . The length    is .
 


 

  1. Find the exact value of the area of  .  (1 mark)

  2. Hence, or otherwise, find the exact value of .  (2 marks)

Show Answers Only

Show Worked Solution

i.   

  ²

 

ii. 

♦ Mean mark 37%.

 

Calculus, 2ADV C3 2018 HSC 13a

Consider the curve  .

  1. Find the stationary points and determine their nature.  (3 marks)

  2. Given that the point  (2,16)  lies on the curve, show that it is a point of inflection.  (2 marks)

  3. Sketch the curve, showing the stationary points, the point of inflection and the and intercepts.  (2 marks)

Show Answers Only
Show Worked Solution

i.   


 


 


 

 

ii. 

 

 

 

iii.  

Plane Geometry, 2UA 2018 HSC 12c

The diagram shows the square . The point is chosen on and the point is chosen on so that  .
 

  1. Prove that is congruent to .   (2 marks)

  2. The side length of the square is 14 cm and has length 4 cm. Find the area of  .   (2 marks)

Show Answers Only
Show Worked Solution

i.   

 

ii.  
   

 

Probability, 2UG 2018 HSC 30d

A game consists of two tokens being drawn at random from a barrel containing 20 tokens. There are 17 tokens labelled 10 cents and 3 tokens labelled $2. The player wins the total value of the two tokens drawn.

  1. Complete the probability tree by writing the missing probabilities in the boxes.  (2 marks)
     

     
  2. Considering that the game costs $1 to play, calculate the financial expectation of the game.  (3 marks)
Show Answers Only
  1.  
Show Worked Solution
i.   

 

ii.   

♦♦ Mean mark part (ii) 27%.
COMMENT: Financial expectation included here (one example only in database) as it is possible to argue that it is an application of expectation.

Financial Maths, STD2 F1 2018 HSC 30b

Last year, Luke’s taxable income was and the tax payable on this income was . This year, Luke’s taxable income has increased by .

  1. Use the table to calculate the tax payable by Luke this year.  (2 marks)
     

  2. How much extra money will Luke have this year, after paying tax, as a result of the increase in his taxable income? Ignore the Medicare levy.  (2 marks)

Show Answers Only
Show Worked Solution

i.   

 

 

ii.