SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

ENGINEERING, AE 2022 HSC 27b

The top and front views of a transition piece in an aircraft air conditioning duct are shown.

Complete a half-pattern development of the transition piece, starting from the line -1 given below.   (6 marks)
 

     

Show Answers Only

 

Notes on this drawing:

→ Ensure you find the true length of lines

→ Ensure fold lines are lighter than the outline

→ Since the half development cuts through a side, ensure you use a break line

→ Do not dimension

→ Only complete a half development and not a full development

Show Worked Solution

 

Notes on this drawing:

→ Ensure you find the true length of lines

→ Ensure fold lines are lighter than the outline

→ Since the half development cuts through a side, ensure you use a break line

→ Do not dimension

→ Only complete a half development and not a full development


♦ Mean mark 40%.

ENGINEERING, CS 2017 HSC 27b

An engineering team has been contracted to design a multi-function lifting device for a coastal container wharf.

The table shows some of the engineering design elements for this lifting device.
 

Explain how the lifting device can be tested and evaluated to determine if the criteria for the listed engineering elements are met.   (6 marks)

Show Answers Only

Show Worked Solution


♦ Mean mark 46%.

ENGINEERING, CS 2017 HSC 25a

A pin-jointed truss designed to support a roadside sign is shown.
 

  1. Determine the magnitude and direction of the reactions at and .   (4 marks)
  1. Determine the magnitude and nature of the force in member .   (2 marks)
  1. Explain why concrete would be a suitable material to support the roadside sign around point .   (2 marks)
Show Answers Only

i.   


 

ii.   
 

iii.   Suitability of concrete

→ Easily poured and formed around the sign.

→ Relatively quick to cure.

→ Weather resistant.

→ Hardens and sets with high strength and hardness.

Show Worked Solution

 
 
   

 

Mean mark 56%.


  

 
   
   

 

 
   
   
 

 

 
 
 

 


♦♦ Mean mark (ii) 38%.

iii.   Suitability of concrete

→ Easily poured and formed around the sign.

→ Relatively quick to cure.

→ Weather resistant.

→ Hardens and sets with high strength and hardness.


♦ Mean mark (iii) 52%.

ENGINEERING, PPT 2017 HSC 24b

A child and sled with a combined mass of 23 kg are being pulled along a horizontal snow-covered surface using a rope.
 

The coefficient of static friction between the sled and the snow is 0.14.

  1. Draw a free-body diagram that indicates the forces acting on the sled. Label the diagram.   (2 marks)
  1. Calculate the tension in the rope immediately before the point at which the sled and child begin to move.   (3 marks)
Show Answers Only

i.
       

 

ii.   

Show Worked Solution
i.
       

Mean mark (i) 57%.

ii.        

 
 
   

  


♦ Mean mark (ii) 40%.

ENGINEERING, PPT 2017 HSC 24a

Sleds are used to slide loads over soft surfaces such as grass, sand and snow. They can be made using polypropylene.

  1. Explain how the properties of polypropylene make it a suitable material for this application.   (3 marks)
  1. Describe a suitable manufacturing method to make a sled from polypropylene.   (3 marks)
Show Answers Only

i.   Polypropylene properties

→ Low coefficient of friction, lightweight and durable with excellent flexural strength.

→ In the manufacturing process, polypropylene is a good material for injection moulding which makes it easy to produce.
 

ii.  Manufacturing method:

→ Polymer pellets are introduced into an injection moulding machine.

→ A heating unit surrounding the exterior of the machine and internal friction heat the pellets.

→ They are forced into a steel mould under pressure.

→ After solidifying, the mould halves are separated and removed using injector pins.

→ Cooling, removal of surplus flash and polishing complete the process.

Show Worked Solution

i.   Polypropylene properties

→ Low coefficient of friction, lightweight and durable with excellent flexural strength.

→ In the manufacturing process, polypropylene is a good material for injection moulding which makes it easy to produce.
 

ii.  Manufacturing method:

→ Polymer pellets are introduced into an injection moulding machine.

→ A heating unit surrounding the exterior of the machine and internal friction heat the pellets.

→ They are forced into a steel mould under pressure.

→ After solidifying, the mould halves are separated and removed using injector pins.

→ Cooling, removal of surplus flash and polishing complete the process.


♦ Mean mark (ii) 53%.

ENGINEERING, PPT 2019 HSC 24b

Normalised high-tensile steel has been chosen for the manufacture of a wing support beam.

  1. Draw and label the microstructures of a normalised high-tensile steel and an annealed high-tensile steel.   (2 marks)
     

  1. Explain how the microstructure produced by normalising high-tensile steel improves the steel's suitability for this application.   (2 marks)
Show Answers Only

i.    Microstructures

 

ii.    → The strength of the steel is markedly increased.

→ Normalising produces finer and more uniform grains.

Show Worked Solution

i.    Microstructures


♦♦♦ Mean mark (i) 26%.

ii.    → The strength of the steel is markedly increased.

→ Normalising produces finer and more uniform grains.


♦ Mean mark (ii) 41%.

BIOLOGY, M8 2019 HSC 33b

Alzheimer's disease causes destruction of brain tissue, dementia and eventually death.

The gene with the greatest known effect on the risk of developing late-onset Alzheimer's disease is called APOE. It is found on chromosome 19.

The APOE gene has multiple alleles, including e2, e3 and e4 .

  1.  What are multiple alleles?   (2 marks)

  2. The table shows the risk of developing Alzheimer's disease for various APOE genotypes compared to average risk in the population.
     

   

  1. Analyse the data to assess the risk of developing Alzheimer's disease associated with the e2, e3 and e4 alleles.   (4 marks)

Show Answers Only

i.     → Alleles are the different variations of the same gene.

→ While most genes only have two alleles, dominant and recessive, some genes have 3 or more versions of itself. This phenomena is referred to as the gene having “multiple alleles”.
  

ii.  Analysis of data 

→ The table indicates that the alleles follow a hierarchy and have influence over the risk of Alzheimer’s, with certain combinations masking effects of others or amplifying them.

→ The e2 allele in both a homozygous genotype and coupled with e3 reduces the risk of Alzheimer’s by 40%. This suggests that the e2 allele is the one which reduces the risk of Alzheimer’s and can mask the effect of e3.

→ However, when coupled with e4, the risk of developing Alzheimer’s is 2.6 times more likely. This suggests that e4 is the more dominant allele.

→ The e4/e3 genotype also makes Alzheimer’s 3.2 times more likely in those individuals, and the e4/e4 genotypes makes it 14.9 times more likely. We can then make the conclusion that the e4 allele makes Alzheimer’s much more common in any genotype where it is present.

→ The e3 allele seems to be completely neutral and almost completely masked by both e2 and e4. In its homozygous genotype, it has no effect on the risk of developing Alzheimer’s, and when heterozygous with either e2 or e4, has little to no effect on the risk in comparison the e2 and e4’s homozygous genotypes.

→ It would then be accurate to conclude that the allele hierarchy is e3<e2<e4, with e3 being neutral and having no known effect, e2 reducing the risk of Alzheimer’s and e4 greatly increasing the risk of developing Alzheimer’s.

Show Worked Solution

i.     → Alleles are the different variations of the same gene.

→ While most genes only have two alleles, dominant and recessive, some genes have 3 or more versions of itself. This phenomena is referred to as the gene having “multiple alleles”.
 


♦♦♦ Mean mark (i) 24%.

ii.  Analysis of data 

→ The table indicates that the alleles follow a hierarchy and have influence over the risk of Alzheimer’s, with certain combinations masking effects of others or amplifying them.

→ The e2 allele in both a homozygous genotype and coupled with e3 reduces the risk of Alzheimer’s by 40%. This suggests that the e2 allele is the one which reduces the risk of Alzheimer’s and can mask the effect of e3.

→ However, when coupled with e4, the risk of developing Alzheimer’s is 2.6 times more likely. This suggests that e4 is the more dominant allele.

→ The e4/e3 genotype also makes Alzheimer’s 3.2 times more likely in those individuals, and the e4/e4 genotypes makes it 14.9 times more likely. We can then make the conclusion that the e4 allele makes Alzheimer’s much more common in any genotype where it is present.

→ The e3 allele seems to be completely neutral and almost completely masked by both e2 and e4. In its homozygous genotype, it has no effect on the risk of developing Alzheimer’s, and when heterozygous with either e2 or e4, has little to no effect on the risk in comparison the e2 and e4’s homozygous genotypes.

→ It would then be accurate to conclude that the allele hierarchy is e3<e2<e4, with e3 being neutral and having no known effect, e2 reducing the risk of Alzheimer’s and e4 greatly increasing the risk of developing Alzheimer’s.


♦♦ Mean mark (ii) 40%.

BIOLOGY, M5 2019 HSC 33a

Alzheimer's disease causes destruction of brain tissue, dementia and eventually death.

The diagram shows the effect of Alzheimer's disease on the brain.

Amyloid beta protein is produced in the human brain throughout life. In people with Alzheimer's disease, it accumulates in excessive amounts.

Outline the main steps that brain cells use to make proteins such as amyloid beta.   (3 marks)

Show Answers Only
  1. A complementary mRNA strand is transcribed from a section of DNA by RNA polymerase in the nucleus.
  2. mRNA moves to the cytoplasm where it binds to ribosomes.
  3. Each 3 nucleic bases (a codon) has a corresponding anticodon of tRNA with an amino acid attached.
  4. The amino acids are ‘stitched’ together in a chain by the ribosomes, forming a polypeptide chain.
  5. The single polypeptide chain or multiple polypeptide chains are folded to form a protein.
Show Worked Solution

     Steps that brain cells use to make proteins such as amyloid beta

  1. A complementary mRNA strand is transcribed from a section of DNA by RNA polymerase in the nucleus.
  2. mRNA moves to the cytoplasm where it binds to ribosomes.
  3. Each 3 nucleic bases (a codon) has a corresponding anticodon of tRNA with an amino acid attached.
  4. The amino acids are ‘stitched’ together in a chain by the ribosomes, forming a polypeptide chain.
  5. The single polypeptide chain or multiple polypeptide chains are folded to form a protein.

♦ Mean mark 41%.

ENGINEERING, TE 2019 HSC 26b

A simple circuit diagram of the AM radio receiver is shown.
 

  1. Complete the table giving the function of each of the circuit components listed.   (5 marks)
     

  1. Complete the table by drawing the waveform at positions B and C.   (2 marks)
     

  1. This AM radio receiver produced a demodulated electrical signal with a small current. The speakers in the earphones converted this signal into sound.
  2. Explain why the speakers in the earphones required a high impedance (resistance) in order to produce sound of sufficient volume to be heard.   (2 marks)
Show Answers Only

“

Show Worked Solution

i.  


♦ Mean mark (i) 47%.

ii.  


♦♦♦ Mean mark (ii) 22%.

iii.   → An external power source is not used to power this radio.

→ The signal picked up by the aerial powers the speakers.

→ If the impedance (resistance) is high an audible signal can be generated by the speaker at a low current.

→ Because the earphone impedance is high, the sensitivity of the coil improves, resulting in a more accurately tuned signal.

→ The sound would be inaudible if the impedance is low, as the power to the earpiece would also be low.


♦♦♦ Mean mark (iii) 15%.

BIOLOGY, M5 2020 HSC 32b

The rabies virus is a single-stranded RNA virus. It contains and codes for only five proteins. The diagrams show the structure and reproduction of the virus.
 

 

  1. Use the information provided in Diagram 1 to explain why the rabies virus cannot be classified as a cellular pathogen.   (3 marks)

  2. After infection the virus reproduces in muscle cells near the bite site and in the central nervous system. This requires the single-stranded rabies RNA to be transcribed, translated and replicated in the cytoplasm of host cells. These processes are shown in Diagram 2.
  3. Use the information provided in Diagrams 1 and 2 to explain the role of viral RNA polymerase in the reproduction of the virus.   (5 marks)

Show Answers Only

i.   Rabies virus cannot be a singular pathogen:

→ This pathogen contains only a single strand of RNA which only codes for five proteins.

→ Cellular pathogens such as bacteria contain a much larger genome in the form of DNA that allows the pathogen to perform complex processes without relying on a host.
 

ii.   The Role of Viral RNA 

→ The viral RNA polymerase (which is made from L and P proteins) is responsible for the production of the viral proteins and RNA, components of the rabies viruses.

→ RNA polymerase is responsible for transcription of viral RNA into mRNA, which is then used by the host’s ribosomes to produce respective viral proteins.

→ RNA polymerase is also responsible for the replication of the viral RNA. In this process, a complementary RNA strand is produced from the original RNA strand. This strand is then used as a template for RNA polymerase to rapidly produce more RNA, complementary to the template. The new RNA will therefore be identical to the original.

→ In this way, RNA polymerase is essential in producing viral proteins and new RNA strands which form new rabies virus particles.

Show Worked Solution

i.   Rabies virus cannot be a singular pathogen:

→ This pathogen contains only a single strand of RNA which only codes for five proteins.

→ Cellular pathogens such as bacteria contain a much larger genome in the form of DNA that allows the pathogen to perform complex processes without relying on a host.


♦♦ Mean mark (i) 33%.

ii.   The Role of Viral RNA 

→ The viral RNA polymerase (which is made from L and P proteins) is responsible for the production of the viral proteins and RNA which are components of the rabies viruses.

→ RNA polymerase is responsible for transcription of viral RNA into mRNA, which is then used by the host’s ribosomes to produce viral proteins.

→ RNA polymerase is also responsible for the replication of the viral RNA. In this process, a complementary RNA strand is produced from the original RNA strand. This strand is then used as a template for RNA polymerase to rapidly produce more RNA, complementary to the template. The new RNA will therefore be identical to the original.

→ In this way, RNA polymerase is essential in producing viral proteins and new RNA strands which form new rabies virus particles.


♦♦♦ Mean mark (ii) 29%.

BIOLOGY, M7 2020 HSC 32a

Rabies is a disease that can affect all mammals and is caused by the rabies virus. It is transmitted by the bite of an infected animal. Without treatment it almost always results in death.


Use the information provided to identify TWO features of the rabies infection that facilitate transmission of the pathogen to a new host.   (2 marks) 

Show Answers Only

Successful answers should include two of the following:

→ The rabies virus can travel through the nervous system which allows it to reach the salivary glands.

→ The virus can then be directly transmitted to another host with a bite from the infected host.

→ The virus can replicate in muscle after an infected bite.

Show Worked Solution

Successful answers should include two of the following:

→ The rabies virus can travel through the nervous system which allows it to reach the salivary glands.

→ The virus can then be directly transmitted to another host with a bite from the infected host.

→ The virus can replicate in muscle after an infected bite.


Mean mark 51%.

BIOLOGY, M6 2021 HSC 33a

Genetically engineered Atlantic salmon have been produced and approved for aquaculture in the US. These salmon have a transgene that includes a protein-coding sequence from a Chinook salmon's growth hormone gene and the promoter region of an Ocean Pout's antifreeze protein gene. The following diagram provides an overview of the production of the transgenic salmon.
 

Explain the processes shown in steps 1-4.   (3 marks)

Show Answers Only

Step 1–2: The transgene is inserted into a plasmid by using enzymes.

Step 2–3: The hybrid plasmid is then placed into a bacterial host.

Step 3–4: As the bacteria replicates via binary fission, multiple copies of the hybrid plasmid are obtained.

Show Worked Solution

Step 1–2: The transgene is inserted into a plasmid by using enzymes.

Step 2–3: The hybrid plasmid is then placed into a bacterial host.

Step 3–4: As the bacteria replicates via binary fission, multiple copies of the hybrid plasmid are obtained.


Mean mark 54%.

ENGINEERING, AE 2019 HSC 24c

A drawing of an aircraft in flight is shown. This aircraft maintains a constant velocity when in level flight.
 

On the following page, draw a labelled shear force diagram AND a labelled bending moment diagram for this situation. Include a labelled free body diagram to support your answer.

Assume the centre of lift on each wing acts as a point load located 7.5 m from the centre line of the plane.

Calculations can be completed in the space below.   (6 marks)

 

Show Answers Only

Show Worked Solution


♦ Mean mark 50%.

ENGINEERING, CS 2019 HSC 23c

The weight of a 650 N rider and the mass of the scooter are evenly distributed between the front and rear pneumatic tyres. The area of contact between each of the two tyres and the ground is 1200 mm². The pressure inside each tyre is 300 kPa.

What is the mass of the scooter?   (3 marks) 

Show Answers Only

Show Worked Solution

 
 
   
   
   

 

 
 
   
   

♦ Mean mark 48%.

ENGINEERING, CS 2019 HSC 23d

A scooter deck, made from aluminium alloy, and its cross-section are shown.

The scooter's manufacturer is concerned that there is too much deflection along the length of the deck when a rider stands on it.

Describe ONE suitable design modification to give the deck greater rigidity without adding extra mass. Use a labelled sketch to support your answer.   (3 marks)

Show Answers Only

Design modifications

→ Add longitudinal ribbing to the deck, ensuring not to create additional mass.

→ This will create an increase in the distance of the neutral axis from the bottom of the deck and a thinner wall structure.

→ The outcome will be a decrease in deflection

Show Worked Solution

Design modifications

→ Add longitudinal ribbing to the deck, ensuring not to create additional mass.

→ This will create an increase in the distance of the neutral axis from the bottom of the deck and a thinner wall structure.

→ The outcome will be a decrease in deflection


 
♦ Mean mark 41%.

ENGINEERING, PPT 2019 HSC 23b

An electric scooter is powered by a 12 volt rechargeable battery with a capacity of 18 Ah.

Calculate the energy stored in the battery. Use 1 Wh = 3600 J.   (2 marks)

Show Answers Only

Show Worked Solution

Power = volts × current

12 V × 18 A × 1 h = 216 W in 1 h

Power stored = 216 Wh

  
1 W = 1 J/s

1 Wh = 3600 J  (1 h = 60 × 60 seconds)

Energy stored  = 216 Wh × 3600 J  
   = 777 600 J  
   = 777.6 kJ  
♦ Mean mark 46%.

ENGINEERING, CS 2019 HSC 22c

The diagram shows some dimensions and forces associated with a telecommunications tower.
 

By considering any necessary reaction, calculate the magnitude of the forces in members and . State the nature of each force. Ignore the weight of the tower.   (6 marks)

Show Answers Only

“

Show Worked Solution

Forces at Joint

Horizontal forces

To calculate vertical force at → use moments.


♦♦ Mean mark 36%.
 
 
 
 
   

    
Forces in Member → method of joints at

→ No horizontal forces

→ Member redundant and carrying no load

→

Member in compression (the force acting down on joint from member is 14.33 kN)

Force in N = 14.33 kN (compression)

  
Using Method of Sections → take moments about Joint

Find the perpendicular distance

 
 
º  
º  
 

 

  

 

ENGINEERING, CS 2019 HSC 21a

A bus shelter is shown.

The design of this bus shelter includes the use of toughened glass panels.

Outline advantages of using toughened glass for this bus shelter.   (3 marks)

Show Answers Only

→ Flexural strength is high.

→ The risk of serious injury is reduced as toughened glass breaks into smaller pieces when broken by applied load.

→ Withstands chipping during transportation and insulation due to strong edge strength.

Show Worked Solution

→ Flexural strength is high.

→ The risk of serious injury is reduced as toughened glass breaks into smaller pieces when broken by applied load.

→ Withstands chipping during transportation and insulation due to strong edge strength.


Mean mark 55%.

ENGINEERING, CS 2019 HSC 20 MC

An annealed copper tensile test specimen has an original cross-sectional area (CSA) of 100 mm².

During testing, an engineering stress of 150 MPa is induced within the specimen before necking occurs. At this strain the CSA reduces uniformly by 10% to 90 mm².

This is illustrated in the diagrams below.
 

What is the value of the true stress induced at this strain?

  1. 135.0 MPa
  2. 136.4 MPa
  3. 150.0 MPa
  4. 166.7 MPa
Show Answers Only

Show Worked Solution
 
 
 

 

 
   

 


♦ Mean mark 49%.

ENGINEERING, PPT 2019 HSC 17 MC

Which row of the table correctly identifies the rolling process used to manufacture each of the components listed at room temperature?

Show Answers Only

Show Worked Solution

→ Steel body panels are formed by cold rolling to make them hard and rigid.

→ Lead alloy battery plates are formed by hot rolling to make them less brittle and to create an equiaxed, uniform grain structure.


♦♦ Mean mark 37%.

ENGINEERING, CS 2019 HSC 16 MC

The image shows part of a large anchor recovered from waters off Western Australia. The anchor's approximate date of manufacture was 1790 .
 

This anchor is most likely made from

  1. cast iron.
  2. cast steel.
  3. wrought iron.
  4. laminated silicon steel.
Show Answers Only

Show Worked Solution

By Elimination:

→ In 1790 mild steel and silicon steel did not exist (eliminate and ).

→ Since it is less brittle, wrought iron would have been used as anchors are subjected to sudden impacts against rocks (eliminate ).


Mean mark 52%.

ENGINEERING, TE 2019 HSC 14 MC

Which row of the table correctly identifies key features of analogue and digital signals used in the transmission of television in Australia?
 

Show Answers Only

Show Worked Solution

By Elimination:

→ VOIP is used to transmit audio, not video (not or )

→ FM signals are better for transmitting audio as they offer a higher fidelity than AM, creating a more accurate reproduction of the original sound (not )


♦ Mean mark 42%.

ENGINEERING, PPT 2019 HSC 13 MC

The diagram shows a seesaw being used by two children, one of mass 25 kg and the other of mass 20 kg. It has a corroded pivot mechanism that reduces its efficiency by 25%.
 

What is the mechanical advantage of the seesaw?

  1. 0.25
  2. 0.75
  3. 0.80
  4. 1.00
Show Answers Only

Show Worked Solution

→ If the arms are the same length, then the MA and VR should be 1. 

→ However, the MA is reduced by 25% due to the decrease in efficiency, as losses in efficiency only change the MA, not the VR.


♦ Mean mark 50%.

ENGINEERING, AE 2019 HSC 10 MC

The diagram shows the airflow in a venturi.
 

What does this diagram illustrate?

  1. Coriolis effect
  2. Pascal's principle
  3. Dynamic pressure
  4. Bernoulli's principle
Show Answers Only

Show Worked Solution

→ Bernoulli’s principle states that the higher the pressure is, the lower the velocity will be.

→ This is illustrated in the diagram as it shows that there is a decreased pressure in the thinner, higher velocity section.


♦ Mean mark 55%.

ENGINEERING, CS 2022 HSC 26a

  1.  The diagram shows a tower crane being used in the construction of a building.
     

  1. Determine the number of 100 kg concrete blocks required to place the boom arm in equilibrium.   (2 marks)
  1. Under a different set of conditions, a wind force is applied, as shown in the diagram.
     

  1. Determine the magnitude and nature of the internal reaction in member A.   (6 marks)
Show Answers Only

i.   

ii. 

Show Worked Solution

i.   Let the total weight of the concrete blocks

 
 
 
   
   
 

 
∴ 12 × 100 kg concrete blocks are needed for the counterweight.


♦ Mean mark (i) 46%.

ii.   Magnitude and nature of internal reaction

 
 
 

  
Taking the horizontal section shown:


  

 
 
 

  
∴


♦ Mean mark (ii) 43%.

ENGINEERING, PPT 2022 HSC 25b

A pictorial view of a machine part is shown.
 

Use the front view and side view provided to complete a sketch of an offset section of the part in the top view.   (3 marks)

 

Show Answers Only

Notes on this drawing:

→ Do not dimension if not asked

→ Ensure section is offset, both larger holes and the smaller hole should be visible as the section is not one line, it cuts through all of them.

→ No hidden detail

→ Section lines correct width apart and angle (approx. 3mm and 45°)

Show Worked Solution

Notes on this drawing:

→ Do not dimension if not asked

→ Ensure section is offset, both larger holes and the smaller hole should be visible as the section is not one line, it cuts through all of them.

→ No hidden detail

→ Section lines correct width apart and angle (approx. 3mm and 45°)


♦ Mean mark 44%.

ENGINEERING, TE 2022 HSC 25a

Describe how an insulation test is performed on electrical cabling.   (3 marks)

Show Answers Only

→ The primary method of testing insulation is the use of a megohmmeter.

→ The megohmmeter is connected across the material to be tested and applies a test voltage for a sample period of around 60 seconds.

→ This allows the device to detect the amount of current leakage, measured in ohms.

Show Worked Solution

→ The primary method of testing insulation is the use of a megohmmeter.

→ The megohmmeter is connected across the material to be tested and applies a test voltage for a sample period of around 60 seconds.

→ This allows the device to detect the amount of current leakage, measured in ohms.


♦♦ Mean mark 39%.

ENGINEERING, AE 2022 HSC 24c

An image of a glider is shown.

The glider is currently on a descent at an angle of 19 degrees. The total lift force is 6250 N.

  1. Draw a free-body diagram, indicating all forces acting on the glider.   (1 mark)   
  1. If the mass of the pilot is 95 kg, calculate the mass of the glider.   (3 marks)
  1. Calculate the lift-to-drag ratio.   (2 marks)
Show Answers Only

i.   Free-body diagram

ii.  Mass of the glider

 
 
   

 


 

iii.   
   

 

Show Worked Solution

i.   Free-body diagram


♦ Mean mark (i) 49%.

ii.  Mass of the glider

 
 
   

 


♦ Mean mark (ii) 46%.
iii.   
   

 


♦♦ Mean mark (iii) 37%.

ENGINEERING, AE 2022 HSC 24b

The image shows corroded screws on the body of an aircraft.

Identify this type of corrosion and explain how it can occur.   (3 marks)

Show Answers Only

→ The corrosion shown in the image is galvanic corrosion

→ It has occurred since the metal used for the body of the plane is not the same as the metal used for the bolts. 

→ This causes galvanic corrosion as the bolts become an anode to protect the cathodic fuselage from rusting. This can also be referred to as dissimilar metal corrosion.
 

Pit and crevice corrosion

→ Although less likely, the image could also show pit and crevice corrosion (concentration cell corrosion), as it is occurring around the cracks between sheets of the fuselage.

→ This occurs when a metal is placed in an electrolyte of varying concentration. In this case there is a lower concentration of oxygen in the crack, causing the tip of the crack to become the anode and corrode.

Show Worked Solution

→ The corrosion shown in the image is galvanic corrosion

→ It has occurred since the metal used for the body of the plane is not the same as the metal used for the bolts. 

→ This causes galvanic corrosion as the bolts become an anode to protect the cathodic fuselage from rusting. This can also be referred to as dissimilar metal corrosion.
 

Pit and crevice corrosion

→ Although less likely, the image could also show pit and crevice corrosion (concentration cell corrosion), as it is occurring around the cracks between sheets of the fuselage.

→ This occurs when a metal is placed in an electrolyte of varying concentration. In this case there is a lower concentration of oxygen in the crack, causing the tip of the crack to become the anode and corrode.


Mean mark 54%.

BIOLOGY, M5 2021 HSC 17 MC

The images show the sequence of changes in the chromosomes (stained black) during mitosis in plant cells.

Which statement is true for mitosis?

  1. Crossing over occurs during prophase.
  2. Sister chromatids separate during anaphase.
  3. Two daughter cells are produced during telophase.
  4. Homologous pairs of chromosomes line up in metaphase.
Show Answers Only

Show Worked Solution

→ Anaphase is the process in which the sister chromatids move away from one another to opposite poles of the spindle and thus separate in mitosis.

♦ Mean mark 45%.

CHEMISTRY, M6 2021 HSC 36

The of sulfurous acid in the following reaction is 1.82.

The of hydrogen sulfite in the following reaction is 7.17.

Calculate the equilibrium constant for the following reaction:    (5 marks)

Show Answers Only

Show Worked Solution

can be derived by multiplying with

 
Therefore


♦ Mean mark 50%.

PHYSICS, M8 2015 HSC 34e

Assess the impact of THREE advances in knowledge about particles and forces on the understanding of the atomic nucleus.   (6 marks)

Show Answers Only

Three of many possible developments are included below.

Advance One:

→ The discovery of the neutron allowed scientists to understand the masses of the nuclei.

→ This discovery enabled scientists to better identify trends in both the periodic table.

Advance Two:

→ Knowledge of the strong nuclear force helps us to explain the interaction between protons and neutrons in the nucleus, and how this force can overcome the electrostatic  force of repulsion.

→ This discovery helps to explain why certain isotopes are unstable.

Advance Three:

→ Knowledge that protons and neutrons are made from different combinations of two types of quarks.

→ This helped to unify our understanding of subatomic particles, informing our base knowledge of quantum physics through the development of the Standard Model.

Show Worked Solution

Three of many possible developments are included below.

Advance One:

→ The discovery of the neutron allowed scientists to understand the masses of the nuclei.

→ This discovery enabled scientists to better identify trends in both the periodic table.

Advance Two:

→ Knowledge of the strong nuclear force helps us to explain the interaction between protons and neutrons in the nucleus, and how this force can overcome the electrostatic  force of repulsion.

→ This discovery helps to explain why certain isotopes are unstable.

Advance Three:

→ Knowledge that protons and neutrons are made from different combinations of two types of quarks.

→ This helped to unify our understanding of subatomic particles, informing our base knowledge of quantum physics through the development of the Standard Model.


♦♦♦ Mean mark 32%.

PHYSICS, M8 2015 HSC 34d

In 1927, Davisson and Germer reported the results of an experiment in which they fired electrons at a crystal of nickel and observed how the electrons were scattered.

  1. State their conclusion, with reference to the results they obtained.   (2 marks)
  1. Explain the significance of this experiment to the Rutherford-Bohr model of the atom.   (3 marks)
Show Answers Only

i.    Experiment Results:

→ Davisson and Germer found that the electrons were not scattered in a random pattern, but formed an interference pattern after passage through the crystal.

→ This pattern is formed by constructive and destructive wave interference.

Conclusion:

→ Since interference is a phenomenon only observed with waves they concluded that electrons were also waves.
 

ii.   The Rutherford-Bohr model:

→ Postulated that electrons existed in fixed orbits. The model however, was unable to explain why only these orbits were stable.

→ The knowledge that electrons are waves provides a plausible explanation for this stability.

→ If electrons act as waves, as indicated by de Broglie, the electron can only exist in orbits where it experiences constructive interference.

Show Worked Solution

i.    Experiment Results:

→ Davisson and Germer found that the electrons were not scattered in a random pattern, but formed an interference pattern after passage through the crystal.

→ This pattern is formed by constructive and destructive wave interference.

Conclusion:

→ Since interference is a phenomenon only observed with waves they concluded that electrons were also waves.
 

ii.   The Rutherford-Bohr model:

→ Postulated that electrons existed in fixed orbits. The model however, was unable to explain why only these orbits were stable.

→ The knowledge that electrons are waves provides a plausible explanation for this stability.

→ If electrons act as waves, as indicated by de Broglie and experimentally shown by Davisson and Germer, the electron can only exist in orbits where it experiences constructive interference.


Mean mark (ii) 54%.

PHYSICS, M8 2016 HSC 34d

Explain how evidence from experiments involving particle accelerators and detectors has provided support for the standard model of matter.   (4 marks)

Show Answers Only

→ High energy collisions in particle accelerators between heavier particles such as protons and materials such as lead have produced many different types of subatomic particles that had never been observed in experiments previously.

→ Properties of these particles, such as momentum and charge, could be deduced from measurements made using a range of sensitive detectors such as calorimeters.

→ The standard model provides an important framework by which physicists can understanding these new particles and their behaviour in collisions.

→ The standard model has predicted the existence of certain particles which were subsequently detected in experiments. This provides further  important validation of the model.

Show Worked Solution

→ High energy collisions in particle accelerators between heavier particles such as protons and materials such as lead have produced many different types of subatomic particles that had never been observed in experiments previously.

→ Properties of these particles, such as momentum and charge, could be deduced from measurements made using a range of sensitive detectors such as calorimeters.

→ The standard model provides an important framework by which physicists can understanding these new particles and their behaviour in collisions.

→ The standard model has predicted the existence of certain particles which were subsequently detected in experiments. This provides further  important validation of the model.


♦♦ Mean mark 38%.

PHYSICS, M8 2016 HSC 33e

Describe how the distribution of stars on a Hertzsprung-Russell diagram relates to the processes that occur during their evolution.   (6 marks)

Show Answers Only

→ An H-R diagram distributes stars into different groupings that relate to the processes that occurred during their evolution.

→ Hydrogen fusion is the primary source of energy of stars on the main sequence.

→ Hydrogen fusion is replaced by helium fusion as the main source of energy in the star’s next evolutionary phase. This grouping of stars is also known as red giants.

→ After helium fusion, the next evolutionary stage for most stars involves gravitational collapse. At this stage, the surface of the star recedes and gravitational potential energy is converted to radiant energy. This grouping is known as white dwarfs.

→ A star’s transition between evolutionary phases occurs quickly relative to time spent in each group. 

→ This transition speed results in fewer stars being distributed in areas outside of these groups on the H-R diagram.
 

Other possible answers could include:

→ Reference to other groups such as protostars, supergiants

→ Sketch of H-R diagram

→ Reference to properties of stars related to their distribution within particular groups

→ Globular and open clusters.

Show Worked Solution

→ An H-R diagram distributes stars into different groupings that relate to the processes that occurred during their evolution.

→ Hydrogen fusion is the primary source of energy of stars on the main sequence.

→ Hydrogen fusion is replaced by helium fusion as the main source of energy in the star’s next evolutionary phase. This grouping of stars is also known as red giants.

→ After helium fusion, the next evolutionary stage for most stars involves gravitational collapse. At this stage, the surface of the star recedes and gravitational potential energy is converted to radiant energy. This grouping is known as white dwarfs.

→ A star’s transition between evolutionary phases occurs quickly relative to time spent in each group. 

→ This transition speed results in fewer stars being distributed in areas outside of these groups on the H-R diagram.
 

Other possible answers could include:

→ Reference to other groups such as protostars, supergiants

→ Sketch of H-R diagram

→ Reference to properties of stars related to their distribution within particular groups

→ Globular and open clusters.


♦♦ Mean mark 44%.

PHYSICS, M8 2016 HSC 33c

Describe how the spectrum of a star can be used to determine its chemical composition and surface temperature.   (4 marks)

Show Answers Only

→ Compounds in the atmosphere of stars absorb specific frequencies of light allowing the elements and molecules to be identified.

→ The resulting absorption spectrum is unique and can be used to accurately describe the chemical composition and each chemical’s relative proportion in the star’s atmosphere.

→ The presence of molecules, neutral atoms or ionised atoms in a star are determined by its temperature.

→ These can also be detected in the absorption spectrum and after adjusting for any Doppler effect, can allow the star’s temperature to be calculated.

Show Worked Solution

→ Compounds in the atmosphere of stars absorb specific frequencies of light allowing the elements and molecules to be identified.

→ The resulting absorption spectrum is unique and can be used to accurately describe the chemical composition and each chemical’s relative proportion in the star’s atmosphere.

→ The presence of molecules, neutral atoms or ionised atoms in a star are determined by its temperature.

→ These can also be detected in the absorption spectrum and after adjusting for any Doppler effect, can allow the star’s temperature to be calculated.


♦ Mean mark 49%.

PHYSICS, M8 2017 HSC 34dii

How did de Broglie, and Davisson and Germer contribute to the modification of the Bohr model of the atom?   (3 marks)

Show Answers Only

→ Bohr’s model posed serious challenges for classical physicists who could not explain the stable electron orbits which were integral to this model.

→ de Broglie modified Bohr’s model by postulating that electrons exist as standing waves about an atom’s nucleus with each of Bohr’s stationary states (energy levels) being associated with various wavelengths.

→ An important feature of de Broglie’s model was that only integer multiples of the fundamental wavelength were possible.

→ This idea was then tested experimentally by Davisson and Germer, whose electron scattering experiments provided evidence that electrons were waves.

Show Worked Solution

→ Bohr’s model posed serious challenges for classical physicists who could not explain the stable electron orbits which were integral to this model.

→ de Broglie modified Bohr’s model by postulating that electrons exist as standing waves about an atom’s nucleus with each of Bohr’s stationary states (energy levels) being associated with various wavelengths.

→ An important feature of de Broglie’s model was that only integer multiples of the fundamental wavelength were possible.

→ This idea was then tested experimentally by Davisson and Germer, whose electron scattering experiments provided evidence that electrons were waves.


Mean mark 55%.

PHYSICS, M8 2017 HSC 34aii

Outline features of the strong nuclear force.   (3 marks)

Show Answers Only

→ The strong nuclear force is an attractive force between nucleons (both protons and neutrons).

→ This force is much stronger than the coulomb repulsion but only acts over very small distances, and is responsible for the stability of the nucleus.

→ The strong nuclear force is extremely attractive between nucleons about 1 fm apart but decreases rapidly at distances greater than about 2.5 fm, effectively limiting the size of a nucleus.

→ This force becomes repulsive at distances less than 0.7 fm.

Show Worked Solution

→ The strong nuclear force is an attractive force between nucleons (both protons and neutrons).

→ This force is much stronger than the coulomb repulsion but only acts over very small distances, and is responsible for the stability of the nucleus.

→ The strong nuclear force is extremely attractive between nucleons about 1 fm apart but decreases rapidly at distances greater than about 2.5 fm, effectively limiting the size of a nucleus.

→ This force becomes repulsive at distances less than 0.7 fm.


Mean mark 53%.

PHYSICS, M8 2017 HSC 34ai

State the composition of the He-3 nucleus in terms of fundamental particles.   (2 marks)

Show Answers Only

He-3 nucleus is made up of the following:

→ 1 neutron

→ 2 protons

→ Each neutron and proton is itself made up of three quarks.

Show Worked Solution

He-3 nucleus is made up of the following:

→ 1 neutron

→ 2 protons

→ Each neutron and proton is itself made up of three quarks.


Mean mark 52%.

PHYSICS, M8 2017 HSC 33bii

Describe a process which can be used to obtain the spectrum of an individual star.   (3 marks)

Show Answers Only

→ Place a diffraction grating over the opening of a telescope.

→ The photographic image produced will show multiple spectra.

→ Each spectrum will represent an individual star in the field of view.
 

Other possible answers may include:

→ Optic fibres are attached to a platen. This should target where starlight falls within a defined field of view.

→ The starlight should then be passed through a spectroscope via the optic fibre for individual stars.

Show Worked Solution

→ Place a diffraction grating over the opening of a telescope.

→ The photographic image produced will show multiple spectra.

→ Each spectrum will represent an individual star in the field of view.
 

Other possible answers may include:

→ Optic fibres are attached to a platen. This should target where starlight falls within a defined field of view.

→ The starlight should then be passed through a spectroscope via the optic fibre for individual stars.


♦♦ Mean mark 38%.

PHYSICS, M8 2017 HSC 33bi

The diagram shows the positions of stars and on a H-R diagram.
 

     

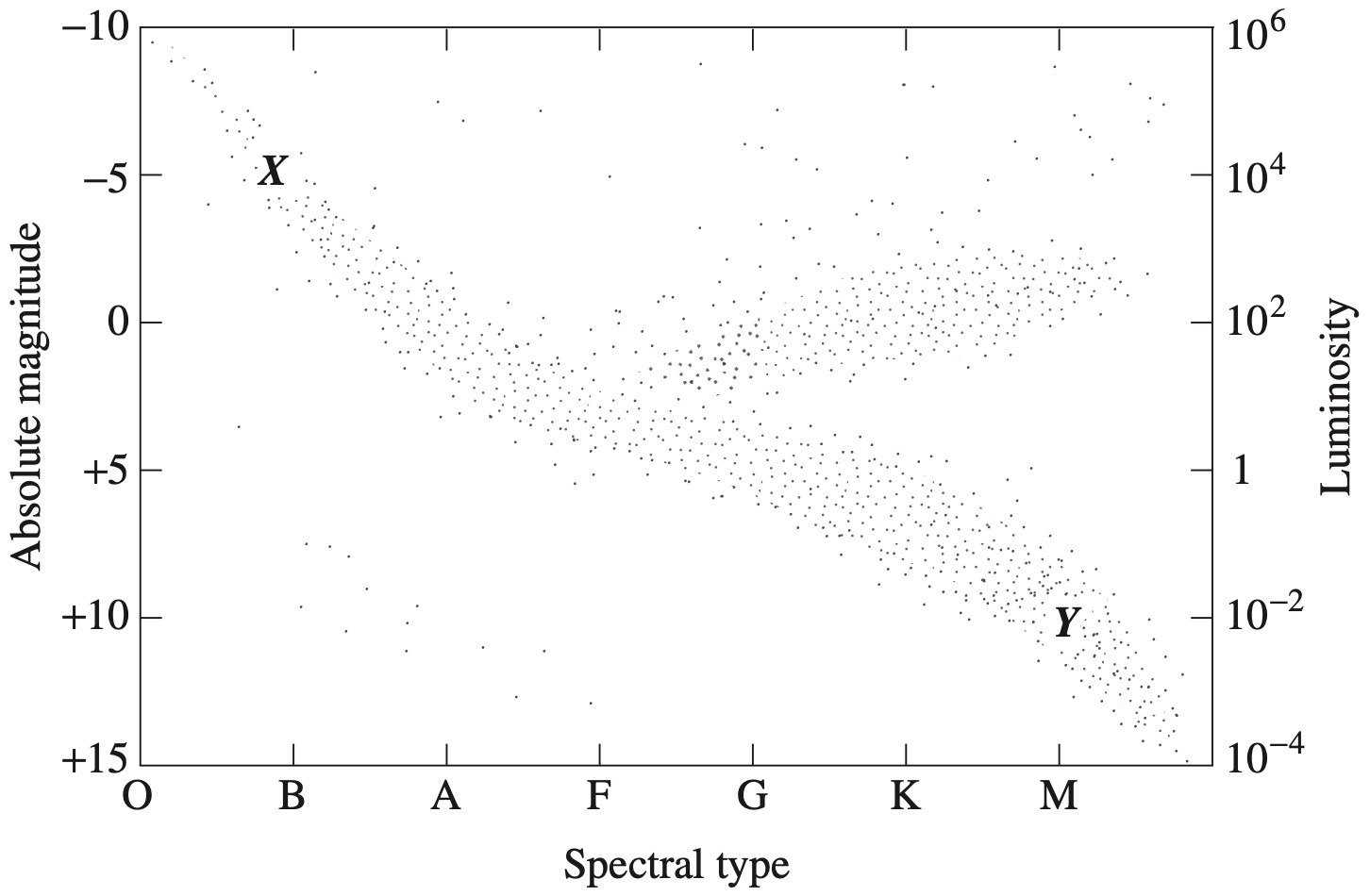
Outline the differences in the spectra of stars and .   (2 marks)

Show Answers Only

→ Star : the H-R diagram exhibits the highest intensity in the blue part of the spectrum (short wavelength) with ionised lines and strong UV component.

→ Star : its highest intensity appears in the red part of the spectrum (long wavelength) with dominant molecular lines and strong neutral metal lines.

Show Worked Solution

→ Star : the H-R diagram exhibits the highest intensity in the blue part of the spectrum (short wavelength) with ionised lines and strong UV component.

→ Star : its highest intensity appears in the red part of the spectrum (long wavelength) with dominant molecular lines and strong neutral metal lines.


♦♦ Mean mark 36%.

BIOLOGY, M8 2018 HSC 31d

A person with normal vision and a person with myopia are both looking at an object in the distance.

Construct THREE labelled diagrams of an eye to show the light path through the eye of the person with:   (5 marks)

    • normal vision
    • myopia
    • myopia corrected with a suitable lens.
Show Answers Only

Show Worked Solution


Mean mark 51%.

BIOLOGY, M8 2018 HSC 31a

  1. Identify TWO structures that refract light as it passes through the eye.   (2 marks)

  2. The diagram shows the human eye. The two lines labelled and represent two beams of light passing through the eye and stimulating different areas of the retina.   

 

  1. The visual perception will be different at the two stimulated areas.
  2. Identify TWO of these differences.  (2 marks)

Show Answers Only

“

Show Worked Solution

i.    Cornea and lens

ii.   Differences in visual perception at and .

→ At the fovea (where strikes), there is a high concentration of cones, so more detail will be detected and better colour perceived than at the periphery.

→ At the periphery (where strikes) there is low visual acuity (detail) and less colour perception, however, there is good distinction between black and white due to the high proportion of rods.


♦♦ Mean mark (ii) 32%.

BIOLOGY, M8 2018 HSC 30

The graph shows the expected life span (the age to which people are expected to live in years) for people of different ages during the 20th century in one country.
 

There have been many biological developments that have contributed to our understanding of the identification, treatment and prevention of disease.

Evaluate the impact of these developments on the expected life span. In your answer, include reference to trends in the data provided.  (8 marks)

Show Answers Only

→ For all ages listed in the graph, life expectancy increased during the 20th century.

→ The lifespan from birth has increased more significantly than other ages ~ 48 to 74 years.

→ The smallest increase being for 60 year olds at ~ 5 years.

→ The ability to understand pathogens and the causes of infectious disease (Koch and Pasteur) has led to early identification and treatment of childhood illnesses such as rubella, polio and whooping cough.

→ Koch and Pasteur established germ theory, culture techniques and a set of postulates to follow in order to create the link between a particular pathogen and disease.

→ Vaccines to combat childhood illnesses were developed through a knowledge of germ theory.

→ The infant/childhood mortality rate has improved significantly, and hence life expectancy, due to the immunity provided by vaccines.

→ An understanding of inherited disorders has also improved lifespans with early diagnosis and prenatal genetic screening for genetic disorders and illnesses.

→ Antibiotic remedies were developed to combat bacterial diseases such as Staphylococcus aureus, due to an understanding of the difference between prokaryotic and eukaryotic cells.

→ With the use of antibiotics many diseases were then no longer life threatening, leading to improved mortality rates across all ages.

→ However, bacterial resistance has resulted with the overuse of antibiotics, so some diseases are now unresponsive to antibiotic treatment.

→ Epidemiology studies involving intricate planning and design, control groups and large scale analysis of data have lead to improvements in the treatment of non-infectious diseases such as cancer.

→ For example the discovery of links between smoking and lung cancer, sun exposure and melanoma, obesity and type II diabetes, has lead to widespread public health campaigns to inform people of the health risks and lowered the associated mortality rates.

→ Improved hygiene, food storage and preservation, and water filtration also occurred in the 20th century leading to fewer preventable diseases and hence increased life spans for all age groups.

→ Improved quarantine requirements have helped prevent the spread of plant, animal and human diseases via international travel.

→In conclusion, developments in biology have lead to increased life expectancy across all age groups, with the biggest improvements for babies and children.

→ These benefits are not necessarily a worldwide phenomenon as poor living conditions and access to medical treatment is not available in many poor socioeconomic communities.

Show Worked Solution

→ For all ages listed in the graph, life expectancy increased during the 20th century.

→ The lifespan from birth has increased more significantly than other ages ~ 48 to 74 years.

→ The smallest increase being for 60 year olds at ~ 5 years.

→ The ability to understand pathogens and the causes of infectious disease (Koch and Pasteur) has led to early identification and treatment of childhood illnesses such as rubella, polio and whooping cough.

→ Koch and Pasteur established germ theory, culture techniques and a set of postulates to follow in order to create the link between a particular pathogen and disease.

→ Vaccines to combat childhood illnesses were developed through a knowledge of germ theory.

→ The infant/childhood mortality rate has improved significantly, and hence life expectancy, due to the immunity provided by vaccines.

→ An understanding of inherited disorders has also improved lifespans with early diagnosis and prenatal genetic screening for genetic disorders and illnesses.

→ Antibiotic remedies were developed to combat bacterial diseases such as Staphylococcus aureus, due to an understanding of the difference between prokaryotic and eukaryotic cells.

→ With the use of antibiotics many diseases were then no longer life threatening, leading to improved mortality rates across all ages.

→ However, bacterial resistance has resulted with the overuse of antibiotics, so some diseases are now unresponsive to antibiotic treatment.

→ Epidemiology studies involving intricate planning and design, control groups and large scale analysis of data have lead to improvements in the treatment of non-infectious diseases such as cancer.

→ For example the discovery of links between smoking and lung cancer, sun exposure and melanoma, obesity and type II diabetes, has lead to widespread public health campaigns to inform people of the health risks and lowered the associated mortality rates.

→ Improved hygiene, food storage and preservation, and water filtration also occurred in the 20th century leading to fewer preventable diseases and hence increased life spans for all age groups.

→ Improved quarantine requirements have helped prevent the spread of plant, animal and human diseases via international travel.

→In conclusion, developments in biology have lead to increased life expectancy across all age groups, with the biggest improvements for babies and children.

→ These benefits are not necessarily a worldwide phenomenon as poor living conditions and access to medical treatment is not available in many poor socioeconomic communities.


♦♦ Mean mark 35%.

BIOLOGY, M5 2018 HSC 29

The diagram models the process of meiosis.
 

  1. Describe the process that accounts for the changes shown in the model during interphase.   (2 marks)

  2. Explain the structure and behaviour of chromosomes in the first division of meiosis. Include detailed reference to the model.   (5 marks)

Show Answers Only

a.   Interphase:

→ In preparation for cell division, DNA is replicated during interphase with the cell.

→ During replication the bonds between the hydrogen bases are broken and the DNA strands (double helix) are unwound into separate strands.

→ Polymerase enzymes add complementary nucleotides to each strand until the strands are identical DNA copies.

→ Half of the original strand is contained in each copy.
 

b.   The first division of meiosis:

→ Homologous chromosomes are represented in the model as the same size.

→ Different shading is used to distinguish between paternal or maternal origins.

→ Individual chromosomes form homologous pairs during the first division of meiosis.

→ These pairs have the same genes but the alleles are not identical.

→ Pairing results in crossing over and an exchange in genetic material between non-sister chromatids takes place.

→ The shading in the diagram illustrates these new combinations.

→ Half the number of chromosomes are possessed by the two resultant daughter cells (diploid cells ⇒ haploid cells).

→ These are randomly assigned, one from each homologous pair.

→ Therefore, when they return to the diploid number, new combinations of chromosomes and greater genetic variation results.

Show Worked Solution

a.   Interphase:

→ In preparation for cell division, DNA is replicated during interphase with the cell.

→ During replication the bonds between the hydrogen bases are broken and the DNA strands (double helix) are unwound into separate strands.

→ Polymerase enzymes add complementary nucleotides to each strand until the strands are identical DNA copies.

→ Half of the original strand is contained in each copy.
 


♦♦ Mean mark (a) 23%.

b.   The first division of meiosis:

→ Homologous chromosomes are represented in the model as the same size.

→ Different shading is used to distinguish between paternal or maternal origins.

→ Individual chromosomes form homologous pairs during the first division of meiosis.

→ These pairs have the same genes but the alleles are not identical.

→ Pairing results in crossing over and an exchange in genetic material between non-sister chromatids takes place.

→ The shading in the diagram illustrates these new combinations.

→ Half the number of chromosomes are possessed by the two resultant daughter cells (diploid cells ⇒ haploid cells).

→ These are randomly assigned, one from each homologous pair.

→ Therefore, when they return to the diploid number, new combinations of chromosomes and greater genetic variation results.


♦ Mean mark (b) 45%.

BIOLOGY, M5 2018 HSC 28

  1. Define the terms genotype and phenotype.  (2 marks)

  2. A population of mammals living in a very cold climate have long, thick fur which assists their survival. At times, a widespread infestation of mites causes their fur to become thin, and bald patches appear in their coats. In this population some individuals are resistant to the mites. This is due to the presence of a co-dominant allele . Individuals that are homozygous for this allele are infertile.
  3. Explain how both genotype and phenotype influence the inheritance of genes and natural selection in this population.  (4 marks)

Show Answers Only

a.   Definitions:

→ Genotype refers to the genetic makeup or combination of genes for a specific trait in an organism.

→ Phenotype refers to the physical manifestation of the genotype or how it appears, develops or behaves in the organism.
  

b.   Genotype and Phenotype influences:

→ The selection pressure for the mammals is long, thick fur to enable them to survive the cold climate.

→ Therefore the allele for long, thick hair is carried by most of the population.

→ The phenotype of these mammals has changed because of the mite infestation.

→ Even though they still have genes for long fur their survival fitness has been compromised as they develop bald patches.

→ The survival of the mammals should be improved during infestations because they possess the mite resistant gene.

→ However, they become infertile when they carry the two alleles – homozygous .

→ Even though they are genetically better suited to the conditions, having long fur and mite resistance, they cannot pass the genes on as they are sterile.

→ Heterozygotes will have some mite resistance as the allele for mite resistance is co-dominant, so their survival rates will be better than those without the allele.

→ The allele can be kept in the population as it can be passed by heterozygotes to future generations.

Show Worked Solution

a.   Definitions:

→ Genotype refers to the genetic makeup or combination of genes for a specific trait in an organism.

→ Phenotype refers to the physical manifestation of the genotype or how it appears, develops or behaves in the organism.
  

b.   Genotype and Phenotype influences:

→ The selection pressure for the mammals is long, thick fur to enable them to survive the cold climate.

→ Therefore the allele for long, thick hair is carried by most of the population.

→ The phenotype of these mammals has changed because of the mite infestation.

→ Even though they still have genes for long fur their survival fitness has been compromised as they develop bald patches.

→ The survival of the mammals should be improved during infestations because they possess the mite resistant gene.

→ However, they become infertile when they carry the two alleles – homozygous .

→ Even though they are genetically better suited to the conditions, having long fur and mite resistance, they cannot pass the genes on as they are sterile.

→ Heterozygotes will have some mite resistance as the allele for mite resistance is co-dominant, so their survival rates will be better than those without the allele.

→ The allele can be kept in the population as it can be passed by heterozygotes to future generations.


♦ Mean mark (b) 44%.

BIOLOGY, M5 2018 HSC 26b

Antidiuretic hormone (ADH) is a protein produced by cells in the hypothalamus. The AVP gene codes for the production of ADH.

  1. Outline the steps to show how a mutation in the AVP gene could result in changes in the ADH protein.    (3 marks)

  2. Identify ONE possible effect of the AVP mutation on kidney function.   (1 mark)

Show Answers Only

i.   Steps: AVP mutation → Change in ADH protein

  1. AVP gene base sequence is changed.
  2. During transcription an error would occur in the mRNA strand produced.
  3. The wrong amino acids would be transported to the ribosome by tRNA at translation.
  4. The polypeptide chain produced is incorrect, dysfunctional or different.
  5. Altered ADH protein results.
     

ii.  Malfunction of ADH will result in less re-absorption of water.

Show Worked Solution

i.   Steps: AVP mutation → Change in ADH protein

  1. AVP gene base sequence is changed.
  2. During transcription an error would occur in the mRNA strand produced.
  3. The wrong amino acids would be transported to the ribosome by tRNA at translation.
  4. The polypeptide chain produced is incorrect, dysfunctional or different.
  5. Altered ADH protein results.
     

ii.  Malfunction of ADH will result in less re-absorption of water.


♦ Mean mark (i) 41%.
Mean mark (ii) 51%

BIOLOGY, M7 2018 HSC 24

A new flu vaccine is prepared each year to protect the population against the current strains of influenza virus. The effectiveness of flu vaccines varies from year to year and can be measured using the overall vaccination effectiveness (VE) index. A VE of 60% means that a vaccinated individual's chance of getting the flu is reduced by 60%.

The following data show the VE over a 10-year period.

  1. Draw an appropriate graph to represent the data on the following grid.   (3 marks)
     
     

     
  2. Provide a possible explanation for the vaccination effectiveness (VE) index in 2014-2015.   (3 marks)

Show Answers Only

a.

   

b.   → The vaccine was less effective between 2014-15 (at 19%).

→ When vaccines are administered the production of antibodies specific to a particular antigen is triggered.

→ If the pathogen mutates after the production of the vaccine the vaccine will not be effective.

→ Therefore, mutation of the virus may be responsible for the vaccine being less effective in 2014-15 and hence the lower VE %.

Show Worked Solution

a.

   

b.   → The vaccine was less effective between 2014-15 (at 19%).

→ When vaccines are administered the production of antibodies specific to a particular antigen is triggered.

→ If the pathogen mutates after the production of the vaccine the vaccine will not be effective.

→ Therefore, mutation of the virus may be responsible for the vaccine being less effective in 2014-15 and hence the lower VE %.


♦ Mean mark (b) 40%.

BIOLOGY, M5 2018 HSC 19 MC

Colour blindness in humans is determined by a sex-linked gene. Two family trees are shown.
 

Which row of the table shows the probability of colour-blind offspring of each sex if individuals 1 and 2 were to have children together?

Show Answers Only

Show Worked Solution

→ A female carrier and a colour-blind male will have a 50% chance of producing either male or female offspring with colour blindness.


Mean mark 55%.

BIOLOGY, M7 2018 HSC 16 MC

How do helper T cells assist in raising a specific immune response to a pathogen?

  1. They mass produce specific antibodies.
  2. They stimulate the cloning of specific T cells.
  3. They are cloned and differentiate to become specific cytotoxic T cells.
  4. They produce cytokines that stimulate the cloning of specific phagocytes.
Show Answers Only

Show Worked Solution

Helper T cells do not:

→ produce antibodies,

→ differentiate into other types of cells.

Further, phagocytes are not part of the immune response.


♦ Mean mark 42%.

BIOLOGY, M5 2017 HSC 24

  1. Three genes are arranged along a homologous pair of chromosomes as shown.
     

   

    1. What is the individual's genotype before crossing over occurs?   (1 mark)

    2. Label, on the diagram below, the alleles after crossing over has occurred.   (1 mark)
       

   

  1. Explain the effect of independent assortment of chromosomes on the genotype of the offspring.   (2 marks)

 

Show Answers Only

a.i   

a.ii


 

b.  Independent Assortment

→ A random alignment of homologous chromosomes takes place during meiosis.

→ The possible number of chromosome combinations is consequently increased.

→ Therefore, the genetic variation of offspring increases.

Show Worked Solution

a.i   

a.ii


♦♦♦ Mean mark (a)(i) 11% 
♦♦ Mean mark (a)(ii) 35%.

b.  Independent Assortment

→ A random alignment of homologous chromosomes takes place during meiosis.

→ The possible number of chromosome combinations is consequently increased.

→ Therefore, the genetic variation of offspring increases.


♦ Mean mark (b) 48%.

BIOLOGY, M7 2017 HSC 14 MC

Which statement correctly describes fungi and protozoans?

  1. Fungi and protozoans are unicellular.
  2. Fungi and protozoans have chloroplasts.
  3. Fungi have a cell wall and protozoans do not.
  4. Fungi are procaryotic and protozoans are eucaryotic.
Show Answers Only

Show Worked Solution

→ Protozoans are unicellular organisms with no cell walls.

→ Fungi cells have cell walls.


♦ Mean mark 43%.

CHEMISTRY, M8 2018 HSC 20 MC

The Winkler method is used to determine the amount of dissolved oxygen in a water sample. The procedure involves the following sequence of reactions.

Step 1. 

Step 2. 

Step 3. 

When a 5.00 L sample of water was analysed using the Winkler method, a total of mol of thiosulfate was required in Step 3.

What concentration of oxygen was present in the original sample?

Show Answers Only

Show Worked Solution

Consider Step 3:

 

Consider Step 2:


 

Consider Step 1:


♦ Mean mark 45%.

CHEMISTRY, M8 2018 HSC 16 MC

An investigation was carried out to determine the calcium ion concentration of a 2.0 L sample of tap water. Excess was added to the sample. The precipitate was filtered, dried and weighed. The mass of the dried precipitate was 400 mg.

What was the concentration of calcium ions in the sample of tap water?

Show Answers Only

Show Worked Solution


♦ Mean mark 41%.

CHEMISTRY, M6 2018 HSC 15 MC

A solution containing potassium dihydrogen phosphate and potassium hydrogen phosphate is a common laboratory buffer with a close to 7.

Which row of the table correctly identifies the chemistry of this buffer?
 

Show Answers Only

Show Worked Solution

By Elimination:

Dihydrogen phosphate molecule is and hydrogen phosphate is (eliminate A and B)

When acid is added, by Le Chatelier’s principle, the equation will shift to the left to reduce the


Mean mark 55%.