A billboard of height
- Use the identity
to show that -
. (2 marks)
- The maximum value of
occurs when and is positive. - Find the value of
for which is a maximum. (3 marks)
Aussie Maths & Science Teachers: Save your time with SmarterEd
A billboard of height
(i)
| (ii)(1) | |
| (2) | |
| (iii) |
| |
|
The cross-section of a 10 metre long tank is an isosceles triangle, as shown in the diagram. The top of the tank is horizontal.
When the tank is full, the depth of water is 3 m. The depth of water at time
--- 6 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
Find the rate at which the depth of water is changing at time
--- 6 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
| (i) |  |
| (ii) | |
| (iii) | ||
| (iv) | |
In
The area of
(i)
(ii)
(iii)
(iv)
How many solutions of the equation
The scatterplot shows the relationship between expenditure per primary school student, as a percentage of a country’s Gross Domestic Product (GDP), and the life expectancy in years for 15 countries.
--- 1 WORK AREA LINES (style=lined) ---
What is the interquartile range? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
Would this country be an outlier for this set of data? Justify your answer with calculations. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
The cost of hiring an open space for a music festival is $120 000. The cost will be shared equally by the people attending the festival, so that
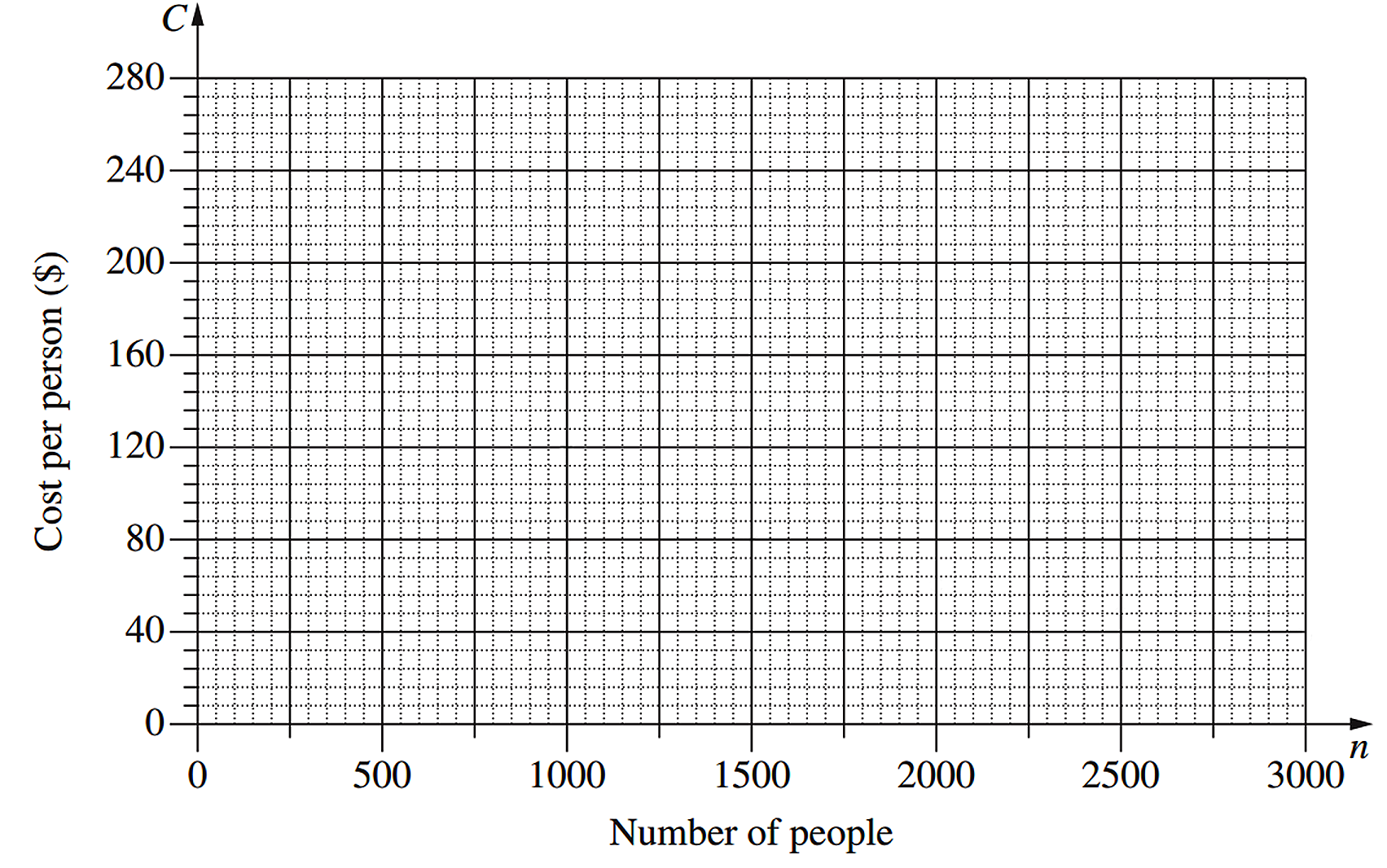
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
Alex is buying a used car which has a sale price of $13 380. In addition to the sale price there are the following costs:
| (i) |
| (ii) | ||
| (iii) |
| (iv) | |
The times taken for 160 music downloads were recorded, grouped into classes and then displayed using the cumulative frequency histogram shown.
On the diagram, draw the lines that are needed to find the median download time. (2 marks)
--- 0 WORK AREA LINES (style=lined) ---
In Mathsville, there are on average eight rainy days in October.
Which expression could be used to find a value for the probability that it will rain on two consecutive days in October in Mathsville?
How many kilobytes are there in 2 gigabytes?
(A)
(B)
(C)
(D)
The point
The point
(i)
| (ii) | ||
| (iii) | |
The binomial theorem states that
(i)
(ii)
(iii)
| |
The equation
| (i) | ||
| (ii) | |||
(i)
(ii)
(iii)
| |
Explain why the number of possible colour combinations is
--- 4 WORK AREA LINES (style=lined) ---
Explain why the number of different selections is
--- 4 WORK AREA LINES (style=lined) ---
Using the identity,
--- 6 WORK AREA LINES (style=lined) ---
| i. | ||
| ii. |
iii.
| |
|
In the diagram,
The points
Copy or trace the diagram into your writing booklet
| (i) |  |
| (ii) | ||
(iii)
The diagram shows two identical circular cones with a common vertical axis. Each cone has height
The lower cone is completely filled with water. The upper cone is lowered vertically into the water as shown in the diagram. The rate at which it is lowered is given by
where
As the upper cone is lowered, water spills from the lower cone. The volume of water remaining in the lower cone at time
--- 4 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
i.
ii.
iii.
A game is played by throwing darts at a target. A player can choose to throw two or three darts.
Darcy plays two games. In Game 1, he chooses to throw two darts, and wins if he hits the target at least once. In Game 2, he chooses to throw three darts, and wins if he hits the target at least twice.
The probability that Darcy hits the target on any throw is
--- 4 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
i.
ii.
| |
|
iii.
iv.
In the diagram,
In the diagram, the vertices of
Copy or trace the diagram into your writing booklet.
The equation of motion for a particle undergoing simple harmonic motion is
where
--- 5 WORK AREA LINES (style=lined) ---
Find the values of
--- 6 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
| i. | ||
ii.
iii.
iv.
A plane
Let
--- 10 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
i.
ii.
--- 5 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
i.
ii.
--- 4 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
ii. Let
Use the result in part c.i. to show that
--- 5 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
Each week Van and Marie take part in a raffle at their respective workplaces.
The probability that Van wins a prize in his raffle is
What is the probability that, during the next three weeks, at least one of them wins a prize? (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
The diagram shows the graph of a function
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 7 WORK AREA LINES (style=lined) ---
Between 5 am and 5 pm on 3 March 2009, the height,
where
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Find all times between 5 am and 5 pm on 3 March 2009 during which the ship was able to enter the harbour. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
The diagram illustrates the design for part of a roller-coaster track. The section
In order that the ride is smooth, the straight line sections must be tangent to the parabola at
--- 6 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
| i. | ||
| ii. | ||
In the diagram,
Copy or trace the diagram into your writing booklet.
The circle
--- 8 WORK AREA LINES (style=lined) ---
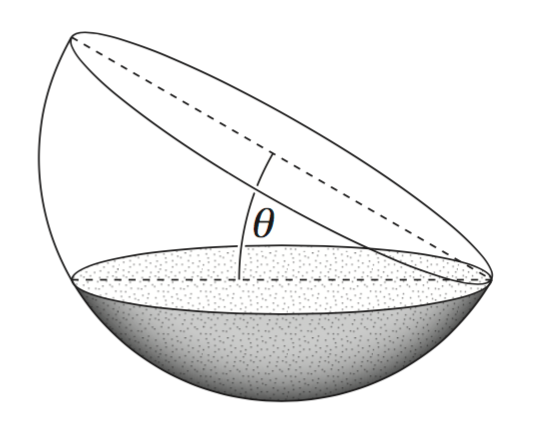
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
i.
ii. (1)
| ii. (2) | ||
In the diagram
| (i) |  |
(ii)
(iii)
(iv)
Let
The diagram shows the graph of the derivative of
The shaded region
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
| i. | |
| ii. | |
| iii. | ||
| iv. |  |
--- 4 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
i.
ii.
A tap releases liquid
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
i.
ii.
The diagram shows
A function is given by
--- 6 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
The diagram shows the region enclosed by the parabola
The point
--- 5 WORK AREA LINES (style=lined) ---
What is the ratio of the volume of the paraboloid to the volume of the cylinder? (1 mark)
--- 8 WORK AREA LINES (style=lined) ---
| i. | ||
ii.
The diagram shows triangles
--- 6 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
i.
| ii. |
|
The diagram shows the front of a tent supported by three vertical poles. The poles are 1.2 m apart. The height of each outer pole is 1.5 m, and the height of the middle pole is 1.8 m. The roof hangs between the poles.
The front of the tent has area
| (i) | ||
| (ii) | ||
| (iii) | |
 |
The diagram shows the graph
What is the value of
--- 3 WORK AREA LINES (style=lined) ---
| |
|
A particle is moving along the
At a certain time,
Which statement describes the motion of the particle at that time?
Norman and Pat each bought the same type of tractor for $60 000 at the same time. The value of their tractors depreciated over time.
The salvage value
Norman drew a graph to represent the salvage value of his tractor.
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Pat used the declining balance formula for calculating the salvage value of her tractor. The depreciation rate that she used was 20% per annum.
--- 4 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
| i. | ||
ii.
| iii. | |
iv.
| v. |
| vi. | |
A study on the mobile phone usage of NSW high school students is to be conducted.
Data is to be gathered using a questionnaire.
The questionnaire begins with the three questions shown.
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
Describe a method that could be used to obtain a representative stratified sample. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
i.
ii.
iii.
iv.
The graph shows tax payable against taxable income, in thousands of dollars.

--- 1 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
| i. |  |
ii.
iii.
iv.
A new shopping centre has opened near a primary school. A survey is conducted to determine the number of motor vehicles that pass the school each afternoon between 2.30 pm and 4.00 pm.
The results for 60 days have been recorded in the table and are displayed in the cumulative frequency histogram.
--- 1 WORK AREA LINES (style=lined) ---
What problem could arise from the change in the median number of motor vehicles passing the school before and after the opening of the new shopping centre?
Briefly recommend a solution to this problem. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
| i. | ||
| ii. |  |
iii.
| iv. | |
Joel mixes petrol and oil in the ratio 40 : 1 to make fuel for his leaf blower.
How much oil should he add to the petrol to ensure that the fuel is in the correct ratio? (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
He wishes to use this fuel in his lawnmower. However, his lawnmower requires the petrol and oil to be mixed in the ratio 25 : 1.
How much oil should he add to the container so that the fuel is in the correct ratio for his lawnmower? (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
| i. | |
ii.
A ship sails 6 km from
size of angle
Copy the diagram into your writing booklet and show all the information on it.
| (i) |  |
(ii)
(iii)
In an experiment, two unbiased dice, with faces numbered 1, 2, 3, 4, 5, 6 are rolled 18 times.
The difference between the numbers on their uppermost faces is recorded each time. Juan performs this experiment twice and his results are shown in the tables.
Juan states that Experiment 2 has given results that are closer to what he expected than the results given by Experiment 1.
Is he correct? Explain your answer by finding the sample space for the dice differences and using theoretical probability. (4 marks)
--- 8 WORK AREA LINES (style=lined) ---
The height and mass of a child are measured and recorded over its first two years.
This information is displayed in a scatter graph.
--- 2 WORK AREA LINES (style=lined) ---
Find the equation of this line. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
i.
ii.
A yacht race follows the triangular course shown in the diagram. The course from
At
--- 1 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
What is the area of this ‘no-go’ zone? (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
In a school, boys and girls were surveyed about the time they usually spend on the internet over a weekend. These results were displayed in box-and-whisker plots, as shown below.
--- 1 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
Under what circumstances would this statement be true? (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
| i. | ||
| ii. | |
| iii. | |
The mass of a sample of microbes is 50 mg. There are approximately
In standard form, what is the approximate mass in grams of one microbe? (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
Adhele has 2000 shares. The current share price is $1.50 per share. Adhele is paid a dividend of $0.30 per share.
| i. | |
| ii. | ||
A retailer has collected data on the number of televisions that he sold each week in 2012.
He grouped the data into classes and displayed the data using a cumulative frequency histogram and polygon (ogive).
--- 4 WORK AREA LINES (style=lined) ---
Is he correct? Give a reason for your answer. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
i.
ii.
The probability that Michael will score more than 100 points in a game of bowling is
Is the commentator correct? Give a reason for your answer. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
i.
| ii. | ||
In July, Ms Alott received a statement for her credit card account. The account has no interest free period. Compound interest is calculated daily and charged to her account on the statement date.
What is the minimum payment due on this account?
The area graph shows the cost and profits for a business over a period of time.
The information in the area graph is then displayed as a line graph.
Which of the following line graphs best displays the data from the area graph?
A restaurant serves three scoops of different flavoured ice-cream in a bowl. There are five flavours to choose from.
How many different combinations of ice-cream could be chosen?
(A)
(B)
(C)
(D)