SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

EXAMCOPY Functions, 2ADV F1 2009 HSC 1a

Sketch the graph of  `y-2x = 3`, showing the intercepts on both axes.   (2 marks)

Show Answers Only

 

Show Worked Solution

`y-2x=3\ \ =>\ \ y=2x+3`

`ytext{-intercept}\ = 3`

`text{Find}\ x\ text{when}\ y=0:`

`0-2x=3\ \ =>\ \ x=-3/2`
 

Trigonometry, 2ADV T1 2023 HSC 16

The diagram shows a shape `APQBCD`. The shape consists of a rectangle `ABCD` with an arc `PQ` on side `AB` and with side lengths `BC` = 3.6 m and `CD` = 8.0 m.

The arc `PQ` is an arc of a circle with centre `O` and radius 2.1 m and `∠POQ=110°`.

 

What is the perimeter of the shape `APQBCD`? Give your answer correct to one decimal place.  (4 marks)

Show Answers Only

`23.8\ text{m}`

Show Worked Solution
`text{Arc}\ PQ` `=110/360 xx pi xx 2 xx 2.1`  
  `=4.03171… \ text{m}`  

 
`text{Consider}\ ΔOPQ:`
 
 

`sin 55^@` `=x/2.1`  
`x` `=2.1 xx sin 55^@`  
  `=1.7202…`  

 
`PQ=2x=3.440\ text{m}`

`:.\ text{Perimeter}` `=8+(2xx3.6)+4.031+(8-3.440)`  
  `=23.79…\ text{m}`  
  `=23.8\ text{m  (to 1 d.p.)}`  

Measurement, STD2 M6 2023 HSC 33

The diagram shows a shape `APQBCD`. The shape consists of a rectangle `ABCD` with an arc `PQ` on side `AB` and with side lengths `BC` = 3.6 m and `CD` = 8.0 m.

The arc `PQ` is an arc of a circle with centre `O` and radius 2.1 m and `∠POQ=110°`.

 

What is the perimeter of the shape `APQBCD`? Give your answer correct to one decimal place.  (4 marks)

Show Answers Only

`23.8\ text{m}`

Show Worked Solution
`text{Arc}\ PQ` `=110/360 xx pi xx 2.1^2`  
  `=4.03171… \ text{m}`  

 
`text{Consider}\ ΔOPQ:`
 

♦ Mean mark 42%.
`sin 55^@` `=x/2.1`  
`x` `=2.1 xx sin 55^@`  
  `=1.7202…`  

 
`PQ=2x=3.440\ text{m}`

`:.\ text{Perimeter}` `=8+(2xx3.6)+4.031+(8-3.440)`  
  `=23.79…`  
  `=23.8\ text{m  (to 1 d.p.)}`  

Statistics, STD2 S4 2023 HSC 3 MC

The number of bees leaving a hive was observed and recorded over 14 days at different times of the day.
 

Which Pearson's correlation coefficient best describes the observations?

  1. `-0.8`
  2. `-0.2`
  3. `0.2`
  4. `0.8`
Show Answers Only

`D`

Show Worked Solution

`text{Correlation is positive and strong.}`

`text{Best option:}\ r=0.8`

`=>D`

NOTE: Inputting all data points into a calculator is unnecessary and time consuming here.

Functions, 2ADV F1 2022 HSC 12

A student believes that the time it takes for an ice cube to melt (`M` minutes) varies inversely with the room temperature `(T^@ text{C})`. The student observes that at a room temperature of `15^@text{C}` it takes 12 minutes for an ice cube to melt.

  1. Find the equation relating `M` and `T`.    (2 marks)

  2. By first completing this table of values, graph the relationship between temperature and time from `T=5^@C` to `T=30^@ text{C}`.   (2 marks)
     

\begin{array} {|c|c|c|c|}
\hline  \ \ T\ \  & \ \ 5\ \  & \ 15\  & \ 30\  \\
\hline M &  &  &  \\
\hline \end{array}

 
                   

Show Answers Only

a.    `M=180/T`

 b.    

\begin{array} {|c|c|c|c|}
\hline  \ \ T\ \  & \ \ 5\ \  & \ 15\  & \ 30\  \\
\hline M & 36 & 12 & 6 \\
\hline \end{array}       

 

Show Worked Solution
a.    `M` `prop 1/T`
  `M` `=k/T`
  `12` `=k/15`
  `k` `=15 xx 12`
    `=180`

 
`:.M=180/T`
 


♦ Mean mark (a) 49%.

b.   

\begin{array} {|c|c|c|c|}
\hline  \ \ T\ \  & \ \ 5\ \  & \ 15\  & \ 30\  \\
\hline M & 36 & 12 & 6 \\
\hline \end{array}

Statistics, 2ADV S3 2022 HSC 26

The life span of batteries from a particular factory is normally distributed with a mean of 840 hours and a standard deviation of 80 hours.

It is known from statistical tables that for this distribution approximately 60% of the batteries have a life span of less than 860 hours.

What is the approximate percentage of batteries with a life span between 820 and 920 hours?  (3 marks)

Show Answers Only

`44text{%}`

Show Worked Solution

`mu=840, \ sigma=80`

`ztext{-score (860)}\ = (x-mu)/sigma=(860-840)/80=0.25` 

`ztext{-score (820)}\ =(820-840)/80=-0.25` 

`ztext{-score (920)}\ =(920-840)/80=1`
 

`text{50% of batteries have a life span below 840 hours (by definintion)}`

`=>\ text{10% lie between 840 and 860 hours}`

`=>\ text{By symmetry, 10% lie between 820 and 840 hours}`

`=> P(-0.25<=z<=0)=10text{%}`
 

`:.\ text{Percentage between 820 and 920}`

`=P(-0.25<=z<=1)`

`=P(-0.25<=z<=0) + P(0<=z<=1)`

`=10+34`

`=44text{%}`

Statistics, 2ADV S2 2022 HSC 24

Jo is researching the relationship between the ages of teenage characters in television series and the ages of actors playing these characters.

After collecting the data, Jo finds that the correlation coefficient is 0.4564.

A scatterplot showing the data is drawn. The line of best fit with equation  `y=-7.51+1.85 x`, is also drawn.
 


 

Describe and interpret the data and other information provided, with reference to the context given.  (4 marks)

Show Answers Only

`text{Correlation coefficient}\ (r) = 0.4564`

    • `text{Moderate and positive correlation}`

`text{Gradient of LOBF}\ = 1.85`

    • `text{On average, each extra year of a character’s age results}`
      `text{in the actor being 1.85 years older.}`

`text{Mode of data set = 15 years}`
  

`text{Limitations}`

    • `text{Data set is very restricted with just a 4 year range of}`
      `text{character ages.}`
    • `text{LOBF not useful when extrapolated to the left as it drops}`
      `text{below zero (on y-axis).}`
    • `text{Relationship describes correlation only, not causation.}`
Show Worked Solution

`text{Correlation coefficient}\ (r) = 0.4564`

    • `text{Moderate and positive correlation}`

`text{Gradient of LOBF}\ = 1.85`

    • `text{On average, each extra year of a character’s age results}`
      `text{in the actor being 1.85 years older.}`

`text{Mode of data set = 15 years}`
  

`text{Limitations}`

    • `text{Data set is very restricted with just a 4 year range of}`
      `text{character ages.}`
    • `text{LOBF not useful when extrapolated to the left as it drops}`
      `text{below zero (on y-axis).}`
    • `text{Relationship describes correlation only, not causation.}`

Financial Maths, 2ADV M1 2022 HSC 21

Eli is choosing between two investment options.

A table of future value interest factors for an annuity of $1 is shown.

  1. What is the value of Eli's investment after 10 years using Option 1 ?  (2 marks)

  2. What is the difference between the future values after 10 years using Option 1 and Option 2?  (2 marks)

Show Answers Only
  1. `$45\ 097.17`
  2. `$40.87`
Show Worked Solution

a.   `text{Monthly r/i}\ = 1.2/12=0.1text{%}\ \ =>\ \ r= 0.001`

`text{Compounding periods}\ (n)=12xx10=120`

`FV` `=PV(1+r)^n`  
  `=40\ 000(1+0.001)^120`  
  `=$45\ 097.17`  

 

b.   `text{Quarterly r/i}\ = 2.4/4=0.6text{%}\ \ =>\ \ r= 0.006`

`text{Compounding periods}\ (N) =4xx10=40`

`text{Annuity factor (from table) = 45.05630}`

`FV` `=1000xx45.05630`  
  `=45\ 056.30`  

 

`text{Difference}` `=45\ 097.17-45\ 056.30`  
  `=$40.87`  

Statistics, STD2 S5 2022 HSC 37

The life span of batteries from a particular factory is normally distributed with a mean of 840 hours and a standard deviation of 80 hours.

It is known from statistical tables that for this distribution approximately 60% of the batteries have a life span of less than 860 hours.

What is the approximate percentage of batteries with a life span between 820 and 920 hours?  (3 marks)

Show Answers Only

`44text{%}`

Show Worked Solution

`mu=840, \ sigma=80`

`ztext{-score (860)}\ = (x-mu)/sigma=(860-840)/80=0.25` 

`ztext{-score (820)}\ =(820-840)/80=-0.25` 

`ztext{-score (920)}\ =(920-840)/80=1`
 

`text{50% of batteries have a life span below 840 hours (by definition)}`

`=>\ text{10% lie between 840 and 860 hours}`

`=>\ text{By symmetry, 10% lie between 820 and 840 hours}`

`=> P(-0.25<=z<=0)=10text{%}`
 

`:.\ text{Percentage between 820 and 920}`

`=P(-0.25<=z<=1)`

`=P(-0.25<=z<=0) + P(0<=z<=1)`

`=10+34`

`=44text{%}`


♦♦ Mean mark 28%.

Statistics, STD2 S4 2022 HSC 35

Jo is researching the relationship between the ages of teenage characters in television series and the ages of actors playing these characters.

After collecting the data, Jo finds that the correlation coefficient is 0.4564.

A scatterplot showing the data is drawn. The line of best fit with equation  `y=-7.51+1.85 x`, is also drawn.
 


 

Describe and interpret the data and other information provided, with reference to the context given.  (4 marks)

Show Answers Only

`text{Correlation coefficient}\ (r) = 0.4564`

    • `text{Moderate and positive correlation}`

`text{Gradient of LOBF}\ = 1.85`

    • `text{On average, each extra year of a character’s age results}`
      `text{in the actor being 1.85 years older.}`

`text{Mode of data set = 15 years}`
  

`text{Limitations}`

    • `text{Data set is very restricted with just a 4 year range of}`
      `text{character ages.}`
    • `text{LOBF not useful when extrapolated to the left as it drops}`
      `text{below zero (on y-axis).}`
    • `text{Relationship describes correlation only, not causation.}`
Show Worked Solution

`text{Correlation coefficient}\ (r) = 0.4564`

    • `text{Moderate and positive correlation}`

`text{Gradient of LOBF}\ = 1.85`

    • `text{On average, each extra year of a character’s age results}`
      `text{in the actor being 1.85 years older.}`

`text{Mode of data set = 15 years}`
  

`text{Limitations}`

    • `text{Data set is very restricted with just a 4 year range of}`
      `text{character ages.}`
    • `text{LOBF not useful when extrapolated to the left as it drops}`
      `text{below zero (on y-axis).}`
    • `text{Relationship describes correlation only, not causation.}`

♦♦ Mean mark 30%.

Statistics, STD2 S4 2022 HSC 23

A teacher surveyed the students in her Year 8 class to investigate the relationship between the average number of hours of phone use per day and the average number of hours of sleep per day.

The results are shown on the scatterplot below.
 

  1. The data for two new students, Alinta and Birrani, are shown in the table below. Plot their results on the scatterplot.  (2 marks)

                               
  2. By first fitting the line of best fit by eye on the scatterplot, estimate the average number of hours of sleep per day for a student who uses the phone for an average of 2 hours per day.  (2 marks)

Show Answers Only
  2. `text{9 hours (see LOBF in diagram above)}`
Show Worked Solution

a.   

b.   `text{9 hours (see LOBF in diagram above)}`

Financial Maths, STD2 F5 2022 HSC 30

Eli is choosing between two investment options.

A table of future value interest factors for an annuity of $1 is shown.

  1. What is the value of Eli's investment after 10 years using Option 1 ?  (2 marks)

  2. What is the difference between the future values after 10 years using Option 1 and Option 2?  (2 marks)

Show Answers Only
  1. `$45\ 097.17`
  2. `$40.87`
Show Worked Solution

a.   `text{Monthly r/i}\ = 1.2/12=0.1text{%}\ \ =>\ \ r= 0.001`

`text{Compounding periods}\ (n)=12xx10=120`

`FV` `=PV(1+r)^n`  
  `=40\ 000(1+0.001)^120`  
  `=$45\ 097.17`  

 


♦ Mean mark 48%.

b.   `text{Quarterly r/i}\ = 2.4/4=0.6text{%}\ \ =>\ \ r= 0.006`

`text{Compounding periods}\ (N) =4xx10=40`

`text{Annuity factor (from table) = 45.05630}`

`FV` `=1000xx45.05630`  
  `=45\ 056.30`  

 

`text{Difference}` `=45\ 097.17-45\ 056.30`  
  `=$40.87`  

♦ Mean mark 43%.

Financial Maths, STD2 F5 2022 HSC 25

The table shows the future value of an annuity of $1.
 
     

Zal is saving for a trip and estimates he will need $15 000. He opens an account earning 3% per annum, compounded annually.

  1. How much does Zal need to deposit every year if he wishes to have enough money for the trip in 4 years time?  (2 marks)

  2. How much interest will Zal earn on his investment over the 4 years? Give your answer to the nearest dollar.  (2 marks)

Show Answers Only
  1. `$3589.09`
  2. `$660`
Show Worked Solution

a.   `text{Using the table:}\ r=3text{%},\ \ n=4`

`text{Annuity factor}\ = 4.184`

`text{Let}\ \ A=\ text{amount invested each year}`

`FV` `=A xx 4.184`  
`15\ 000` `=A xx 4.184`  
`:.A` `=(15\ 000)/4.184`  
  `=$3585.09`  

 

b.   `text{Total payments}\ = 4 xx 3585.09=$14\ 340.36`

`text{Interest earned}` `=FV-\ text{total payments}`  
  `=15\ 000-14\ 340.36`  
  `=659.64`  
  `=$660\ \ text{(nearest $)}`  

♦♦ Mean mark 33%.

Statistics, STD2 S1 2022 HSC 19

The table shows the types of customer complaints received by an online business in a month.
 

  1. What are the values of `A` and `B`?  (2 marks)

  2. The data from the table are shown in the following Pareto chart.
     
     
  3. The manager will address 80% of the complaints.
  4. Which types of complaints will the manager address?  (1 mark)

Show Answers Only
  1. `A=160, \ B=96`
  2. `text{Stock shortages and delivery fees.}`
Show Worked Solution

a.   `A=98+62=160`

`text{% Damaged items}\ = 8/200 xx 100 = 4text{%}`

`text{Cumulative % after damaged items = 96%}`

`B = 92+4=96`
 

b.   `text{The right hand side cumulative frequency percentage}`

`text{shows that 80% of all complaints received concern}`

`text{stock shortages and delivery fees.}`

`:.\ text{The manager will address stock shortages and delivery fees.}`


♦ Mean mark part (b) 41%.

Statistics, STD2 S5 2022 HSC 18

The marks in a test were normally distributed. The mean mark was 60 and the standard deviation was 15 .

What was the percentage of marks higher than 90?  (2 marks)

Show Answers Only

`2.5text{%}`

Show Worked Solution
`ztext{-score}` `=(x-mu)/sigma`  
  `=(90-60)/15`  
  `=2`  

 

`text{% marks > 90}\ = 2.5text{%}`


Mean mark 52%.
COMMENT: A poor State result that warrants attention.

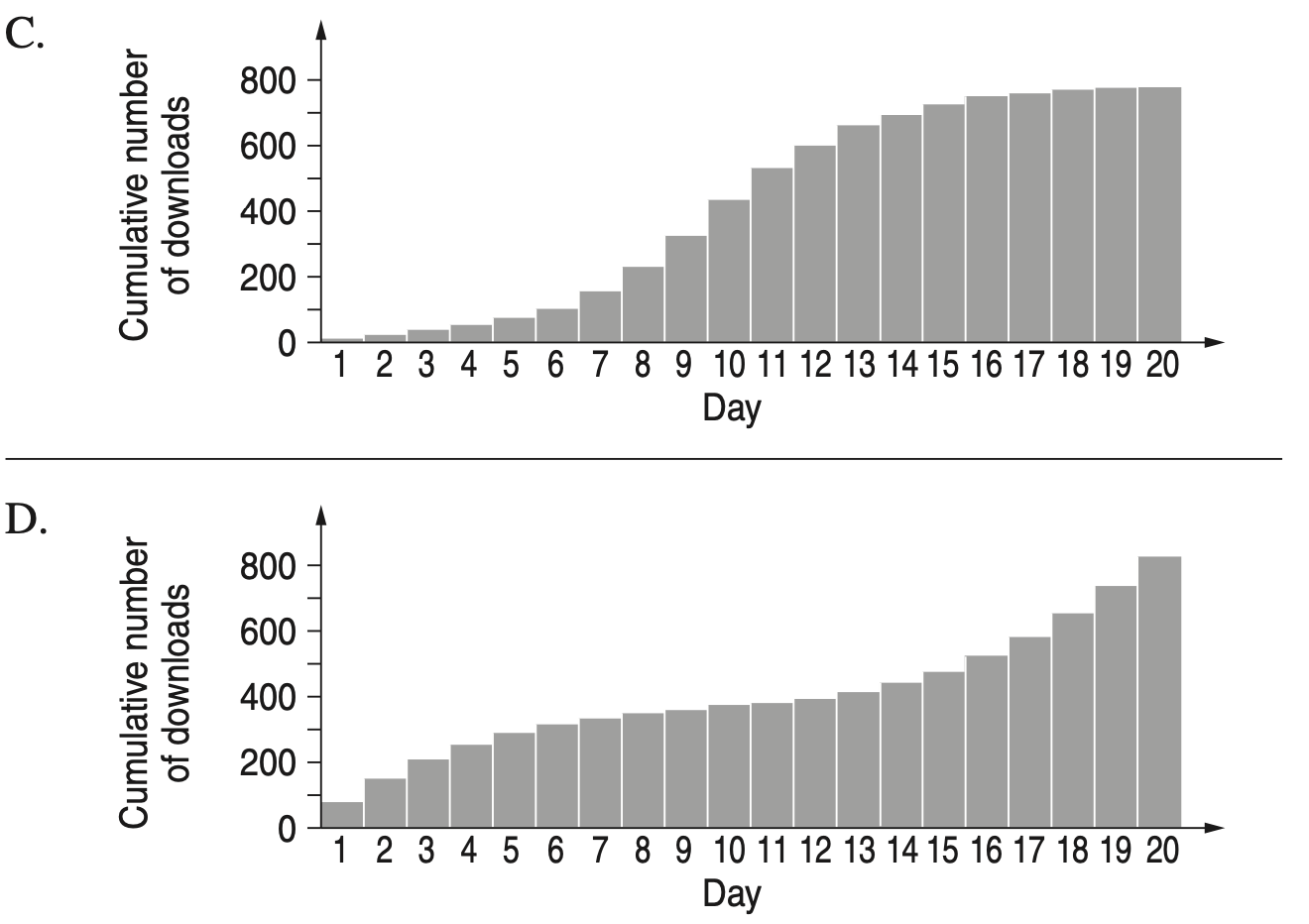
Statistics, STD2 S1 2022 HSC 15 MC

The cumulative frequency graph shows the distribution of the number of movie downloads made by 100 people in one month.
 

Which box-plot best represents the same data as displayed in the cumulative frequency graph?
 

Show Answers Only

`C`

Show Worked Solution

`text{1st quartile}\ ~~ 3`

`text{Median}\ ~~ 6`

`text{3rd quartile}\ ~~ 7`

`=>C`


♦♦♦ Mean mark 30%.

Statistics, STD2 S4 2022 HSC 12 MC

For a particular course, the recorded data show a relationship between the number of hours of study per week and the marks achieved out of 100 .

A least-squares regression line is fitted to this dataset. The equation of this line is given by

`M=20+3 H,`

where `M` is the predicted mark and `H` is the number of hours of study per week.

Based on this regression equation, which of the following is correct regarding the predicted mark of a student?

  1. It will be 3 for zero hours of study per week.
  2. It will be 20 for zero hours of study per week.
  3. It will increase by 20 for every additional hour of study per week.
  4. It will increase by 1 for every 3 additional hours of study per week.
Show Answers Only

`B`

Show Worked Solution

`text{Consider Option}\ B:`

`text{If zero hours of study are done per week}\ \ → \ \ H=0`

`:. M=20+(3 xx 0) = 20`

`=>B`

Financial Maths, STD2 F4 2022 HSC 11 MC

In ten years, the future value of an investment will be $150 000. The interest rate is 4% per annum, compounded half-yearly.

Which equation will give the present value `(PV)` of the investment?

  1. `PV=(150\ 000)/((1+0.04)^(10))`
  2. `PV=(150\ 000)/((1+0.04)^(20))`
  3. `PV=(150\ 000)/((1+0.02)^(10))`
  4. `PV=(150\ 000)/((1+0.02)^(20))`
Show Answers Only

`D`

Show Worked Solution

`text{Compounding periods}\ = 10 xx 2 = 20`

`text{Compounding rate}\ = (4text{%})/2 = 2text{%} = 0.02`

`PV=(150\ 000)/((1+0.02)^(20))`

`=>D`

Statistics, STD2 S5 SM-Bank 7

800 participants auditioned for a stage musical. Each participant was required to complete a series of ability tests for which they received an overall score.

The overall scores were approximately normally distributed with a mean score of 69.5 points and a standard deviation of 6.5 points.

Only the participants who scored at least 76.0 points in the audition were considered successful.

How many of the participants were considered unsuccessful?   (2 marks)

Show Answers Only

`672`

Show Worked Solution

`mu = 69.5 \ , \ sigma= 6.5`

`ztext{-score} \ (76) = {76 – 69.5}/6.5 = 1`

`:.\  text{Unsuccessful}` `=84text(%) xx 800`
  `= 672`

Statistics, 2ADV S2 SM-Bank 5 MC

 The stem plot below shows the height, in centimetres, of 20 players in a junior football team.
 

A player with a height of 179 cm is considered an outlier because 179 cm is greater than

  1. 162 cm
  2. 169 cm
  3. 173 cm
  4. 175.5 cm
Show Answers Only

`D`

Show Worked Solution

`Q_1 = (148 + 148)/2 = 148`

`Q_3 = (158 + 160)/2 = 159`

`IQR = 159 – 148 = 11`

`text{Upper fence}` `= Q_3 + 1.5 xx IQR`
  `= 159 + 1.5 xx 11`
  `= 175.5`

 
`=> D`

Statistics, STD2 S1 SM-Bank 5 MC

 The stem plot below shows the height, in centimetres, of 20 players in a junior football team.
 

A player with a height of 179 cm is considered an outlier because 179 cm is greater than

  1. 162 cm
  2. 169 cm
  3. 173 cm
  4. 175.5 cm
Show Answers Only

`D`

Show Worked Solution

`Q_1 = (148 + 148)/2 = 148`

`Q_3 = (158 + 160)/2 = 159`

`IQR = 159 – 148 = 11`

`text{Upper fence}` `= Q_3 + 1.5 xx IQR`
  `= 159 + 1.5 xx 11`
  `= 175.5`

 
`=> D`

Statistics, STD2 S5 2021 HSC 41

In a particular city, the heights of adult females and the heights of adult males are each normally distributed.

Information relating to two females from that city is given in Table 1.
 

The means and standard deviations of adult females and males, in centimetres, are given in Table 2.
 


 

A selected male is taller than 84% of the population of adult males in this city.

By first labelling the normal distribution curve below with the heights of the two females given in Table 1, calculate the height of the selected male, in centimetres, correct to two decimal places.  (4 marks)

 

Show Answers Only

`178.95 \ text{cm}`

Show Worked Solution

 

`z text{-score (175 cm, female)} = 2`

♦♦♦ Mean mark 16%.

`z text{-score (160.6 cm, female)} = -1`
 

`text{Find} \ mu \ text{of female heights:}`

`mu – sigma` `= 160.6`  
`mu + 2sigma` `= 175`  
`3 sigma` `= 175 – 160.6`  
`sigma` `= 14.4/3`  
  `= 4.8 \ text{cm}`  
`:. \ mu` `= 165.4 \ text{cm}`  

 

`text{Selected male’s height has} \ z text{-score} = 1`

`mu text{(male)} = 1.05 times 165.4 = 173.67`

`sigma \ text{(male)} = 1.1 times 4.8 = 5.28`

 

`:. \ text{Actual male height}` `= 173.67 + 5.28`  
  `= 178.95 \ text{cm}`  

Statistics, 2ADV S2 2021 HSC 17

For a sample of 17 inland towns in Australia, the height above sea level, `x` (metres), and the average maximum daily temperature, `y` (°C), were recorded.

The graph shows the data as well as a regression line.
 

     
 

The equation of the regression line is  `y = 29.2 − 0.011x`.

The correlation coefficient is  `r = –0.494`.

  1. i.  By using the equation of the regression line, predict the average maximum daily temperature, in degrees Celsius, for a town that is 540 m above sea level. Give your answer correct to one decimal place.  (1 mark)

  2. ii. The gradient of the regression line is −0.011. Interpret the value of this gradient in the given context.  (2 marks)

  3. The graph below shows the relationship between the latitude, `x` (degrees south), and the average maximum daily temperature, `y` (°C), for the same 17 towns, as well as a regression line.
     
     
         
     
    The equation of the regression line is  `y = 45.6 − 0.683x`.
  4. The correlation coefficient is  `r = − 0.897`.
  5. Another inland town in Australia is 540 m above sea level. Its latitude is 28 degrees south.
  6. Which measurement, height above sea level or latitude, would be better to use to predict this town’s average maximum daily temperature? Give a reason for your answer.  (1 mark)

Show Answers Only
  1. i.  `23.3°text(C)`
  2. ii. `text(See Worked Solutions)`
  3. `text(Latitude. Correlation coefficient shows a stronger relationship.)`
Show Worked Solution
a.i.    `y` `=29.2 – 0.011(540)`
    `=23.26`
    `=23.3°text{C  (1 d.p.)`
 

a.ii.  `text(On average, the average maximum daily temperature of)`

`text(inland towns drops by 0.011 of a degree for every metre)`

`text(above sea level the town is situated.)`
 

b.  `text(The correlation co-efficient of the regression line using)`

`text(latitude is significantly stronger than the equivalent)`

`text(co-efficient for the regression line using height above sea)`

`text(level.)`

`:.\ text(The equation using latitude is preferred.)`

Statistics, STD2 S4 2021 HSC 33

For a sample of 17 inland towns in Australia, the height above sea level, `x` (metres), and the average maximum daily temperature, `y` (°C), were recorded.

The graph shows the data as well as a regression line.
 

     
 

The equation of the regression line is  `y = 29.2 − 0.011x`.

The correlation coefficient is  `r = –0.494`.

  1. i.  By using the equation of the regression line, predict the average maximum daily temperature, in degrees Celsius, for a town that is 540 m above sea level. Give your answer correct to one decimal place.  (1 mark)

  2. ii. The gradient of the regression line is −0.011. Interpret the value of this gradient in the given context.  (2 marks)

  3. The graph below shows the relationship between the latitude, `x` (degrees south), and the average maximum daily temperature, `y` (°C), for the same 17 towns, as well as a regression line.
     
     
         
     
    The equation of the regression line is  `y = 45.6 − 0.683x`.
  4. The correlation coefficient is  `r = − 0.897`.
  5. Another inland town in Australia is 540 m above sea level. Its latitude is 28 degrees south.
  6. Which measurement, height above sea level or latitude, would be better to use to predict this town’s average maximum daily temperature? Give a reason for your answer.  (1 mark)

Show Answers Only
  1. i.  `23.3°text(C)`
  2. ii. `text(See Worked Solutions)`
  3. `text(Latitude. Correlation coefficient shows a stronger relationship.)`
Show Worked Solution
a.i.    `y` `=29.2 – 0.011(540)`
    `=23.26`
    `=23.3°text{C  (1 d.p.)`
♦♦ Mean mark part a.ii. 28%.

 
a.ii.  `text(On average, the average maximum daily temperature of)`

`text(inland towns drops by 0.011 of a degree for every metre)`

`text(above sea level the town is situated.)`
 

♦♦♦ Mean mark part b 18%.

b.  `text(The correlation co-efficient of the regression line using)`

`text(latitude is significantly stronger than the equivalent)`

`text(co-efficient for the regression line using height above sea)`

`text(level.)`

`:.\ text(The equation using latitude is preferred.)`

Financial Maths, STD2 F5 2021 HSC 31

Present value interest factors for an annuity of $1 for various interest rates (`r`) and numbers of periods (`N`) are given in the table.
 

   
 

A bank lends Martina $500 000 to purchase a home, with interest charged at 1.5% per annum compounding monthly. She agrees to repay the loan by making equal monthly repayments over a 30-year period.

How much should the monthly payment be in order to pay off the loan in 30 years?

Give your answer correct to the nearest cent.  (2 marks)

Show Answers Only

`$ 1725.60`

Show Worked Solution

`text{Monthly interest rate}\ (r) = 1.5/12 = 0.125text(%) = 0.00125`

♦ Mean mark 43%.

`N = 30 xx 12 = 360`

`=>\ text(PV annuity factor = 289.75411)`

`:.\ text{Monthly payment}` `= (500\ 000)/289.75411`  
  `= $1725.60`  

Trigonometry, 2ADV T1 2021 HSC 18

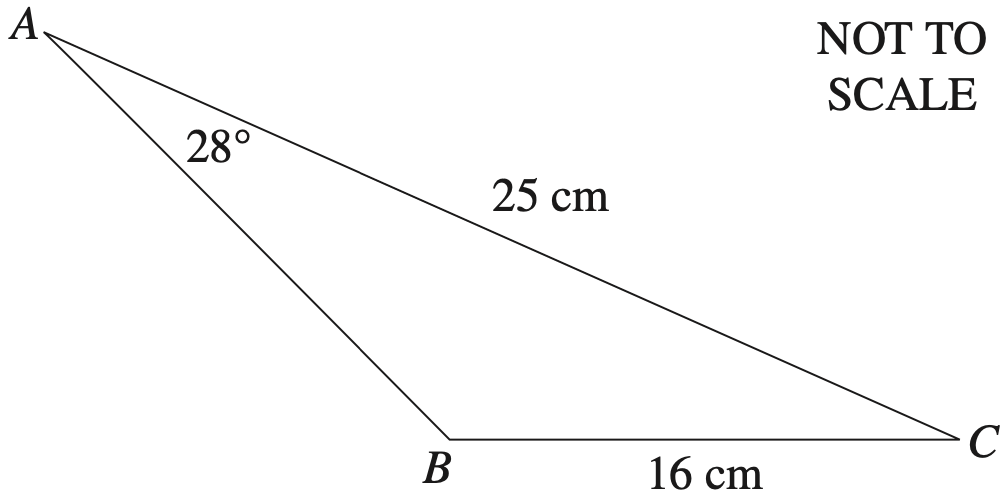
The diagram shows a triangle `ABC` where `AC` = 25 cm, `BC` = 16 cm, `angle BAC` = 28° and angle `ABC` is obtuse.
 


 

Find the size of the obtuse angle `ABC` correct to the nearest degree.  (3 marks)

Show Answers Only

`133°`

Show Worked Solution

`text(Using the sine rule:)`

`sin theta/25` `= (sin 28°)/16`
`sin theta` `= (25 xx sin 28°)/16`
`sin theta` `= 0.73355`
`theta` `= 47°`
 
`:. angleABC` `= 180-47`
  `= 133°`

Financial Maths, 2ADV M1 2021 HSC 25

A table of future value interest factors for an annuity of $1 is shown.
 

   

Simone deposits $1000 into a savings account at the end of each year for 8 years. The interest rate for these 8 years is 0.75% per annum, compounded annually.

After the 8th deposit, Simone stops making deposits but leaves the money in the savings account. The money in her savings account then earns interest at 1.25% per annum, compounded annually, for a further two years.

Find the amount of money in Simone's savings account at the end of ten years.  (3 marks)

Show Answers Only

`$8419.81`

Show Worked Solution

`text(In 1st 8 years:)`

Mean mark 52%.

`text(Future value factor = 8.2132)`

`text(Value of annuity)` `= 8.2132 xx 1000`
  `= $8213.20`
 
`text(After 10 years:)` 
`text(Value of investment)` `= 8213.2 xx (1.0125)^2`
  `= $$8419.81`

Financial Maths, STD2 F5 2021 HSC 40

A table of future value interest factors for an annuity of $1 is shown.
 

   

Simone deposits $1000 into a savings account at the end of each year for 8 years. The interest rate for these 8 years is 0.75% per annum, compounded annually.

After the 8th deposit, Simone stops making deposits but leaves the money in the savings account. The money in her savings account then earns interest at 1.25% per annum, compounded annually, for a further two years.

Find the amount of money in Simone's savings account at the end of ten years.  (3 marks)

Show Answers Only

`$8419.81`

Show Worked Solution

`text(In 1st 8 years:)`

♦ Mean mark 35%.

`text(Future value factor = 8.2132)`

`text(Value of annuity)` `= 8.2132 xx 1000`
  `= $8213.20`
 
`text(After 10 years:)` 
`text(Value of investment)` `= 8213.2 xx (1.0125)^2`
  `= $8419.81`

Statistics, STD2 S5 2021 HSC 38

A random variable is normally distributed with mean 0 and standard deviation 1. The table gives the probability that this random variable lies between 0 and `z` for different values of `z`.

 

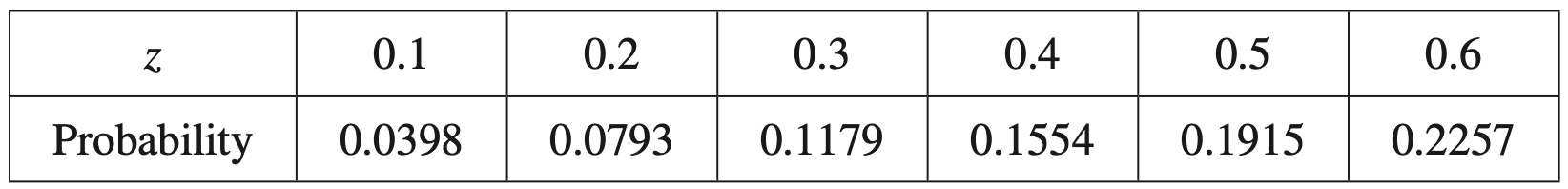
The probability values given in the table for different values of `z` are represented by the shaded area in the following diagram.
 

  1. Using the table, show that the probability that a value from a random variable that is normally distributed with mean 0 and standard deviation 1 is greater than 0.3 is equal to 0.3821.  (1 mark)

  2. Birth weights are normally distributed with a mean of 3300 grams and a standard deviation of 570 grams. By first calculating a `z`-score, find how many babies, out of 1000 born, are expected to have a birth weight greater than 3471 grams.  (3 marks)

Show Answers Only
  1. `text(See Worked Solutions)`
  2. `382`
Show Worked Solution

a.   `P(z>0)=0.5`

♦♦♦ Mean mark part (a) 1%.
COMMENT: Note the Std2 and Advanced questions varied slightly but used the same table and graph.

`P(0<z<0.3)=0.1179`

`P(z>0.3) = 0.5-0.1179=0.3821`
 

b.   `z text{-score (3471)}` `=(x-mu)/sigma`
    `=(3471-3300)/570`
    `=0.3`

 
`P(z>0.3) = 0.3821\ \ text{(see part (a))}`
  

`:.\ text(Number of babies > 3471 grams)`  
`=1000 xx 0.3821`  
`=382\ \ text{(nearest whole)}`  

Measurement, STD2 M6 2021 HSC 37

The diagram shows a triangle `ABC` where `AC` = 25 cm, `BC` = 16 cm, `angle BAC` = 28° and angle `ABC` is obtuse.
 


 

Find the size of the obtuse angle `ABC` correct to the nearest degree.  (3 marks)

Show Answers Only

`133°`

Show Worked Solution

`text(Using the sine rule:)`

♦♦ Mean mark 31%.
`sin theta/25` `= (sin 28°)/16`
`sin theta` `= (25 xx sin 28°)/16`
`sin theta` `= 0.73355`
`theta` `= 47°`
 
`:. angleABC` `= 180-47`
  `= 133°`

Statistics, 2ADV S3 2021 HSC 22

A random variable is normally distributed with mean 0 and standard deviation 1. The table gives the probability that this random variable lies between 0 and `z` for different values of `z`.
 

   

The probability values given in the table for different values of `z` are represented by the shaded area in the following diagram.
 

  1. Using the table, find the probability that a value from a random variable that is normally distributed with a mean of 0 and standard deviation 1 lies between 0.1 and 0.5.  (1 mark)

  2. Birth weights are normally distributed with a mean of 3300 grams and a standard deviation of 570 grams. By first calculating a `z`-score, find how many babies, out of 1000 born, are expected to have a birth weight greater than 3528 grams.  (3 marks)

Show Answers Only
  1. `0.1517`
  2. `345\ text(babies)`
Show Worked Solution

♦♦ Mean mark part (a) 29%.
COMMENT: Note the Advanced and Std2 questions varied slightly but used the same table and graph.
a.    `P(0.1 < x < 0.5)` `= 0.1915 – 0.0398`
    `= 0.1517`
 

b.   `mu = 330, sigma = 570`

♦ Mean mark part (b) 48%.
`ztext(-score)\ (3528)` `= (x – mu)/sigma`
  `= (3528 – 3300)/570`
  `= 0.4`

 

`P(ztext(-score) > 0.4)`  `= 0.5 – 0.1554`
  `= 0.3446`

 
`:.\ text(Expected babies > 3528 grams)`

`= 1000 xx 0.3446`

`= 344.6`

`~~ 345\ text(babies)`

Statistics, STD2 S4 2021 HSC 28

A salesperson is interested in the relationship between the number of bottles of lemonade sold per day and the number of hours of sunshine on the day.

The diagram shows the dataset used in the investigation and the least-squares regression line.


 

  1. Find the equation of the least-squares regression line relating to the dataset.  (2 marks)

  2. Suppose a sixth data point was collected on a day which had 10 hours of sunshine. On that day 45 bottles of lemonade were sold.
  3. What would happen to the gradient found in part (a)?  (1 mark)

Show Answers Only
  1. `y=3.2x+2`
  2. `text{Gradient would increase (steepen).}`
Show Worked Solution

a.   `text(Method 1)`

♦ Mean mark part (a) 37%.

`text{Input data points (in Stats Mode “Ax + B”):}`

`(2,8), (3, 11), (5, 19), (6, 22), (9, 30)`

`=>\ y=3.2x + 2`
 

`text(Method 2)`
 

`text{Find gradient using (0, 2) and (5, 18)}:`

`m=(18-2)/(5-0) = 3.2,\ \ ytext(-intercept)\ = 2`

`:.\ text(Equation:)\ y=3.2x + 2`

Mean mark part (b) 53%.

 
 b.   `text(Method 1)`

`text{Add (10, 45) to the data set in Stats Mode above:}`

`text(Gradient increases to 4.1.)`
 

`text(Method 2)`

`text{Data point (10, 45) lies above the regression line.`

`:.\ text{Gradient would increase (steepen).}`

Financial Maths, STD2 F5 2021 HSC 21

Julie invests $12 500 in a savings account. Interest is paid at a fixed monthly rate. At the end of each month, after the monthly interest is added, Julie makes a deposit of $500.

Julie has created a spreadsheet to show the activity in her savings account. The details for the first 6 months are shown.
 

   

By finding the monthly rate of interest, complete the final row above for the 7th month.  (3 marks)

Show Answers Only

`15\ 624.20, 23.44, 16\ 147.64`

Show Worked Solution

`\text{Monthly interest rate} = \frac{18.75}{12\ 500} = 0.0015 =\ text{0.15%}`

♦ Mean mark 43%.
 

`\text{Row 7 calculations:}`

`\text{Beginning balance}` `= 15\ 624.20`
`\text{Monthly interest}` `= 15\ 624.20 \times 0.0015`
  `= 23.44`

 

`\text{End of month balance}` `= 15\ 624.20 + 23.44 + 500`
  `= 16\ 147.64`

Probability, 2ADV S1 2021 HSC 6 MC

There are 8 chocolates in a box. Three have peppermint centres (P) and five have caramel centres (C).

Kim randomly chooses a chocolate from the box and eats it. Sam then randomly chooses and eats one of the remaining chocolates.

A partially completed probability tree is shown.
 

What is the probability that Kim and Sam choose chocolates with different centres?

  1. `\frac{15}{64}`
  2. `\frac{15}{56}`
  3. `\frac{15}{32}`
  4. `\frac{15}{28}`
Show Answers Only

`D`

Show Worked Solution

 

`Ptext{(different centres)}` `= P text{(PC)} + P text{(CP)}`
  `=\frac{3}{8} · \frac{5}{7} + \frac{5}{8} · \frac{3}{7}`
  `= \frac{15}{56} + \frac{15}{56}`
  `= \frac{15}{28}`

 
`=> D`

Probability, STD2 S2 2021 HSC 11 MC

There are 8 chocolates in a box. Three have peppermint centres (P) and five have caramel centres (C).

Kim randomly chooses a chocolate from the box and eats it. Sam then randomly chooses and eats one of the remaining chocolates.

A partially completed probability tree is shown.
 

What is the probability that Kim and Sam choose chocolates with different centres?

  1. `\frac{15}{64}`
  2. `\frac{15}{56}`
  3. `\frac{15}{32}`
  4. `\frac{15}{28}`
Show Answers Only

`D`

Show Worked Solution

♦♦ Mean mark 35%.

 

`Ptext{(different centres)}` `= P text{(PC)} + P text{(CP)}`
  `=\frac{3}{8} · \frac{5}{7} + \frac{5}{8} · \frac{3}{7}`
  `= \frac{15}{56} + \frac{15}{56}`
  `= \frac{15}{28}`

 
`=> D`

Functions, 2ADV F2 2021 HSC 5 MC

Which of the following best represents the graph of  `y = 10 (0.8)^x`?
 

Show Answers Only

`A`

Show Worked Solution

`\text{By elimination:}`

`\text{When} \ x = 0 \ , \ y = 10(0.8) ^0 = 10`

`-> \ text{Eliminate B and D}`

`text(As)\ \ x→oo, \ y→0`

`-> \ text{Eliminate C}`

`=> A`

Algebra, STD2 A4 2021 HSC 10 MC

Which of the following best represents the graph of  `y = 10 (0.8)^x`?
 

Show Answers Only

`A`

Show Worked Solution

`\text{By elimination:}`

♦ Mean mark 41%.

`\text{When} \ x = 0 \ , \ y = 10(0.8) ^0 = 10`

`-> \ text{Eliminate B and D}`

`text(As)\ \ x→oo, \ y→0`

`-> \ text{Eliminate C}`

`=> A`

Statistics, 2ADV S2 2021 HSC 4 MC

The number of downloads of a song on each of twenty consecutive days is shown in the following graph.
 

Which of the following graphs best shows the cumulative number of downloads up to and including each day?

Show Answers Only

`C`

Show Worked Solution

`text{The gradient of the cumulative frequency histogram}`

`text{will increase gradually, be steepest at day 10 then}`

`text{decrease gradually.}`

`=> C`

Statistics, STD2 S1 2021 HSC 7 MC

The number of downloads of a song on each of twenty consecutive days is shown in the following graph.
 

Which of the following graphs best shows the cumulative number of downloads up to and including each day?

Show Answers Only

`C`

Show Worked Solution

`text{The gradient of the cumulative frequency histogram}`

♦ Mean mark 50%.

`text{will increase gradually, be steepest at day 10 then}`

`text{decrease gradually.}`

`=> C`

Statistics, STD2 S1 2021 HSC 3 MC

The stem-and-leaf plot shows the number of goals scored by a team in each of ten netball games.
  

What is the mode of this dataset?

  1.  5
  2.  18
  3.  25
  4.  29
Show Answers Only

`C`

Show Worked Solution

`\text{Mode}  -> \text{data point with highest frequency}`

`\text{Mode}  = 25`

`=> C`

Statistics, STD2 S5 2021 HSC 8 MC

On a test, Zac's mark corresponded to a `z`-score of 2. The test scores had a mean of 63 and a standard deviation of 8.

What was Zac's actual mark on the test?

  1. 65
  2. 67
  3. 73
  4. 79
Show Answers Only

`D`

Show Worked Solution

`text{Method 1 (quicker)}`

`text(Actual mark)` `= mu + 2 xx sigma`
  `= 63 + 2 xx 8`
  `= 79`

 

`text(Method 2)`

`ztext(-score)` `= (x – mu)/sigma`
`2` `= (x-63)/8`
`16` `=x-63`
`x` `=79`

 
`=>D`

Financial Maths, STD2 F2 2021 HSC 5 MC

Peter currently earns $21.50 per hour. His hourly wage will increase by 2.1% compounded each year for the next four years.

What will his hourly wage be after four years?

  1. `21.50(1.21)^4`
  2. `21.50(1.021)^4`
  3. `21.50 + 21.50 xx 0.21 xx 4`
  4. `21.50 + 21.50 xx 0.021 xx 4`
Show Answers Only

`B`

Show Worked Solution

`text(Wage after 1 year) = 21.50 xx 1.021`

`text(Wage after 2 years) = 21.50 xx 1.021 xx 1.021 = 21.50(1.021)^2`

`vdots`

`text(Wage after 4 years) = 21.50(1.021)^4`

`=>  B`

Financial Maths, STD2 F5 2020 HSC 37

Wilma deposited a lump sum into a new bank account which earns 2% per annum compound interest.

Present value interest factors for an annuity of $1 for various interest rates (`r`) and numbers of periods (`N`) are given in the table.
 


 

Wilma was able to make the following withdrawals from this account.

  • $1000 at the end of each year for twenty years (starting one year after the account is opened)
  • $3000 each year for ten years starting 21 years after the account is opened.

Calculate the minimum lump sum Wilma must have deposited when she opened the new account.   (3 marks)

Show Answers Only

`$ 34 \ 486`

Show Worked Solution

`text{Annuity 1:}\ PV\ text{of $1000 annuity for 20 years at} \ \ r = 0.02`

♦♦♦ Mean mark 23%.

`PV\ text{factor} = 16.351`

`therefore \ PV\ text{Annuity 1}` `= 16.351 xx 1000`
  `= $16 \ 351`

 
`text{Annuity 2:}\ PV\ text{of $3000 annuity for years 21-30 at} \ \ r = 0.02`

`PV\ text{Annuity 2}` `= PVtext{(30 years)} – PVtext{(20 years)}`
  `= 3000 xx 22.396 – 3000 xx 16.351`
  `= $ 18 \ 135`

 

`:.\ text{Lump sum required}` `= 16 \ 351 + 18\ 135`
  `= $ 34 \ 486`

Statistics, STD2 S5 2020 HSC 35

The intelligence Quotient (IQ) scores for adults in City A are normally distributed with a mean of 108 and a standard deviation of 10.

The IQ score for adults in City B are normally distributed with a mean of 112 and a standard deviation of 16.

  1. Yin is an adult who lives in City A and has an IQ score of 128.
    What percentage of the adults in this city have an IQ score higher than Yin's?   (2 marks) 

  2. There are 1 000 000 adults living in City B.
    Calculate the number of adults in City B that would be expected to have an IQ score lower than Yin's.  (2 marks)

  3. Simon, an adult who lives in City A, moves to City B. The  `z` -score corresponding to his IQ score in City A is the same as the `z`-score corresponding to his IQ score in City B.
    By first forming an equation, calculate Simon's IQ score. Give your answer correct to one decimal place.   (3 marks)

Show Answers Only
  1. `text(2.5%)`
  2. `840 \ 000`
  3. `101.3`
Show Worked Solution

a.    `text{In City A:}`

♦ Mean mark 50%.

`z text{-score}\ (128) = frac {x -mu}{sigma} = frac{128 – 108}{10} = 2`
 


 

`therefore\ text{2.5% have a higher IQ in City} \ A.`
 

b.    `text{In City B:}`

♦ Mean mark 44%.

`z text{-score}\ (128) = frac{128 – 112}{16} = 1`
 


 

`therefore \ text{Adults in City} \ B \ text{with an IQ}\ <  128`

`= 84text(%) xx 1 \ 000 \ 000`

`= 840 \ 000`
 

c.    `z text{-score in City A}\ = z text{-score in City B}`

♦♦♦ Mean mark 17%.
`frac{x – 108}{10} ` `= frac{x -112}{16} \ \ text{(multiply b.s.} \ xx 160 text{)}`
`16 (x – 108)` `= 10 (x – 112)`
`16 x – 1728` `= 10 x – 1120`
`6x` `= 608`
`x` `= 101.3`

  
`therefore \ text{Simon’s IQ} = 101.3 \ text{(to 1 d.p.)}`

Statistics, STD2 S4 2020 HSC 36

A cricket is an insect. The male cricket produces a chirping sound.

A scientist wants to explore the relationship between the temperature in degrees Celsius and the number of cricket chirps heard in a 15-second time interval.

Once a day for 20 days, the scientist collects data. Based on the 20 data points, the scientist provides the information below.

  • A box-plot of the temperature data is shown.
     
       
  • The mean temperature in the dataset is 0.525°C below the median temperature in the dataset.
  • A total of 684 chirps was counted when collecting the 20 data points.

The scientist fits a least-squares regression line using the data `(x, y)`, where `x` is the temperature in degrees Celsius and `y` is the number of chirps heard in a 15-second time interval. The equation of this line is

`y = −10.6063 + bx`,

where `b` is the slope of the regression line.

The least-squares regression line passes through the point  `(barx, bary)`, where  `barx`  is the sample mean of the temperature data and  `bary`  is the sample mean of the chirp data.

Calculate the number of chirps expected in a 15-second interval when the temperature is 19° Celsius. Give your answer correct to the nearest whole number.  (5 marks)

Show Answers Only

`29\ text(chirps)`

Show Worked Solution

`y = −10.6063 + bx`

♦♦♦ Mean mark 18%.

`text(Find)\ b:`

`text(Line passes through)\ \ (barx, bary)`

`barx` `= 22 – 0.525`
  `= 21.475`
`bary` `= text(total chirps)/text(number of data points)`
  `= 684/20`
  `= 34.2`

 

`34.2` `= −10.6063 + b(21.475)`
`:.b` `= 44.8063/21.475`
  `~~ 2.0864`

 
`text(If)\ \ x = 19,`

`y` `= −10.6063 + 2.0864 xx 19`
  `= 29.03`
  `= 29\ text(chirps)`

Statistics, STD2 S2 2020 HSC 28

Consider the following dataset.

`1        5        9         10        15`

Suppose a new value, `x`, is added to this dataset, giving the following.

      `1        5        9         10        15        x`

It is known that  `x`  is greater than 15. It is also known that the difference between the means of the two datasets is equal to ten times the difference between the medians of the two datasets.

Calculate the value of `x`.    (4 marks)

Show Answers Only

`38`

Show Worked Solution

`text{Dataset 1 : Median} = 9`

Mean mark 53%.

`text{Dataset 2 : Median} = frac{9 + 10}{2} = 9.5`

`:.\ text{Difference between means}`

`= 10 xx (9.5 – 9)`

`= 5`
 

`overset_ x_1 = frac{1 + 5 + 9 + 10 + 15}{5} = 8`
  
`therefore overset_ x_2 = 8 + 5 = 13`
 

`text{Sum of all data points in Dataset 2}`

`= 6 xx 13`

`= 78`
 

`78` `= 1 + 5 + 9 + 10 + 15 + x`
`therefore \ x` `= 78 – 40`
  `= 38`

Functions, 2ADV F1 2020 HSC 11

There are two tanks on a property, Tank `A` and Tank `B`. Initially, Tank `A` holds 1000 litres of water and Tank B is empty.

  1.  Tank `A` begins to lose water at a constant rate of 20 litres per minute. The volume of water in Tank `A` is modelled by  `V = 1000 - 20t`  where  `V`  is the volume in litres and  `t`  is the time in minutes from when the tank begins to lose water.   (1 mark)
     
    On the grid below, draw the graph of this model and label it as Tank `A`.
     
       

  2. Tank `B` remains empty until  `t=15`  when water is added to it at a constant rate of 30 litres per minute.

     

    By drawing a line on the grid (above), or otherwise, find the value of  `t`  when the two tanks contain the same volume of water.  (2 marks)

  3. Using the graphs drawn, or otherwise, find the value of  `t`  (where  `t > 0`) when the total volume of water in the two tanks is 1000 litres.  (1 mark)

Show Answers Only
  1.  `text{T} text{ank} \ A \ text{will pass trough (0, 1000) and (50, 0)}` 
      
  2. `29 \ text{minutes}`
  3. `45 \ text{minutes}`
Show Worked Solution

a.     `text{T} text{ank} \ A \ text{will pass trough (0, 1000) and (50, 0)}` 
 


 

b.   `text{T} text{ank} \ B \ text{will pass through (15, 0) and (45, 900)}`  
 

   

`text{By inspection, the two graphs intersect at} \ \ t = 29 \ text{minutes}`

 
c.   `text{Strategy 1}`

`text{By inspection of the graph, consider} \ \ t = 45`

`text{T} text{ank A} = 100 \ text{L} , \ text{T} text{ank B} =900 \ text{L} `

`:.\ text(Total volume = 1000 L when  t = 45)`
  

`text{Strategy 2}`

`text{Total Volume}` `=text{T} text{ank A} + text{T} text{ank B}`
`1000` `= 1000 – 20t + (t – 15) xx 30`
`1000` `= 1000 – 20t + 30t – 450 `
`10t` `= 450`
`t` `= 45 \ text{minutes}`

Algebra, STD2 A4 2020 HSC 24

There are two tanks on a property, Tank A and Tank B. Initially, Tank A holds 1000 litres of water and Tank B is empty.

  1. Tank A begins to lose water at a constant rate of 20 litres per minute.

     

    The volume of water in Tank A is modelled by  `V = 1000 - 20t`  where `V` is the volume in litres and  `t`  is the time in minutes from when the tank begins to lose water.

     

    On the grid below, draw the graph of this model and label it as Tank A.   (1 mark)
     

     

  2. Tank B remains empty until  `t=15`  when water is added to it at a constant rate of 30 litres per minute.
     By drawing a line on the grid (above), or otherwise, find the value of  `t`  when the two tanks contain the same volume of water.  (2 marks)

  3. Using the graphs drawn, or otherwise, find the value of  `t`  (where  `t > 0`) when the total volume of water in the two tanks is 1000 litres.  (1 mark)

Show Answers Only
  1.  `text{T} text{ank} \ A \ text{will pass trough (0, 1000) and (50, 0)}` 
      
  2. `29 \ text{minutes}`
  3. `45 \ text{minutes}`
Show Worked Solution

a.     `text{T} text{ank} \ A \ text{will pass trough (0, 1000) and (50, 0)}` 
 

 

b.   `text{T} text{ank} \ B \ text{will pass through (15, 0) and (45, 900)}`  
 

   

`text{By inspection, the two graphs intersect at} \ \ t = 29 \ text{minutes}`

 
c.   `text{Strategy 1}`

♦♦ Mean mark part (c) 22%.

`text{By inspection of the graph, consider} \ \ t = 45`

`text{T} text{ank A} = 100 \ text{L} , \ text{T} text{ank B} =900 \ text{L} `

`:.\ text(Total volume = 1000 L when  t = 45)`
  

`text{Strategy 2}`

`text{Total Volume}` `=text{T} text{ank A} + text{T} text{ank B}`
`1000` `= 1000 – 20t + (t – 15) xx 30`
`1000` `= 1000 – 20t + 30t – 450 `
`10t` `= 450`
`t` `= 45 \ text{minutes}`

Statistics, 2ADV S2 2020 HSC 27

A cricket is an insect. The male cricket produces a chirping sound.

A scientist wants to explore the relationship between the temperature in degrees Celsius and the number of cricket chirps heard in a 15-second time interval.

Once a day for 20 days, the scientist collects data. Based on the 20 data points, the scientist provides the information below.

  • A box-plot of the temperature data is shown.
     
       
  • The mean temperature in the dataset is 0.525°C below the median temperature in the dataset.
  • A total of 684 chirps was counted when collecting the 20 data points.

The scientist fits a least-squares regression line using the data `(x, y)`, where `x` is the temperature in degrees Celsius and `y` is the number of chirps heard in a 15-second time interval. The equation of this line is

`y = −10.6063 + bx`,

where `b` is the slope of the regression,

The least-squares regression line passes through the point  `(barx, bary)`, where  `barx`  is the sample mean of the temperature data and  `bary`  is the sample mean of the chirp data.

Calculate the number of chirps expected in a 15-second interval when the temperature is 19° Celsius. Give your answer correct to the nearest whole number.  (5 marks)

Show Answers Only

`29\ text(chirps)`

Show Worked Solution

`y = −10.6063 + bx`

♦ Mean mark 46%.

`text(Find)\ b:`

`text(Line passes through)\ \ (barx, bary)`

`barx` `= 22 – 0.525`
  `= 21.475`

 

`bary` `= text(total chirps)/text(number of data points)`
  `= 684/20`
  `= 34.2`

 

`34.2` `= −10.6063 + b(21.475)`
`:.b` `= 44.8063/21.475`
  `~~ 2.0864`

 

`text(If)\ \ x = 19,`

`y` `= −10.6063 + 2.0864 xx 19`
  `= 29.03`
  `= 29\ text(chirps)`

Financial Maths, STD2 F5 2020 HSC 34

Tina inherits $60 000 and invests it in an account earning interest at a rate of 0.5% per month. Each month, immediately after the interest has been paid, Tina withdraws $800.

The amount in the account immediately after the `n`th withdrawal can be determined using the recurrence relation

`A_n = A_(n - 1)(1.005) - 800`,

where `n = 1, 2, 3, …`  and  `A_0 = 60\ 000`

  1. Use the recurrence relation to find the amount of money in the account immediately after the third withdrawal.  (2 marks)

  2. Calculate the amount of interest earned in the first three months.  (2 marks)

Show Answers Only
  1. `$58\ 492.49`
  2. `$892.49`
Show Worked Solution

♦ Mean mark part (a) 41%.
a.    `A_1` `= 60\ 000(1.005) – 800 = $59\ 500`
  `A_2` `= 59\ 500(1.005) – 800 = $58\ 997.50`
  `A_3` `= 58\ 997.50(1.005) – 800 = $58\ 492.49`

 

b.   `text{Amount (not interest)}`

♦♦ Mean mark part (b) 33%.

`= 60\ 000 – (3 xx 800)`

`= $57\ 600`
 

`:.\ text(Interest earned in 3 months)`

`= A_3 – 57\ 600`

`= 58\ 492.49 – 57\ 600`

`= $892.49`

Trigonometry, 2ADV T1 2020 HSC 15

Mr Ali, Ms Brown and a group of students were camping at the site located at `P`. Mr Ali walked with some of the students on a bearing of 035° for 7 km to location `A`. Ms Brown, with the rest of the students, walked on a bearing of 100° for 9 km to location `B`.
 


 

  1. Show that the angle `APB` is 65°.  (1 mark)

  2. Find the distance `AB`.  (2 marks)

  3. Find the bearing of Ms Brown's group from Mr Ali's group. Give your answer correct to the nearest degree.  (2 marks)

Show Answers Only
  1. `text(See Worked Solutions)`
  2. `8.76\ text{km  (to 2 d.p.)}`
  3. `146^@`
Show Worked Solution
a.    `angle APB` `= 100 – 35`
    `= 65^@`

 

b.   `text(Using cosine rule:)`

`AB^2` `= AP^2 + PB^2 – 2 xx AP xx PB cos 65^@`
  `= 49 + 81 – 2 xx 7 xx 9 cos 65^@`
  `= 76.750…`
`:.AB` `= 8.760…`
  `= 8.76\ text{km  (to 2 d.p.)}`

 
c.

`anglePAC = 35^@\ (text(alternate))`

`text(Using cosine rule, find)\ anglePAB:`

`cos anglePAB` `= (7^2 + 8.76 – 9^2)/(2 xx 7 xx 8.76)`  
  `= 0.3647…`  
`:. angle PAB` `= 68.61…^@`  
  `= 69^@\ \ (text(nearest degree))`  

 

`:. text(Bearing of)\ B\ text(from)\ A\ (theta)` 

`= 180 – (69 – 35)`

`= 146^@`