SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Calculus, MET1 2016 VCAA 3

Let    where  .

  1. Sketch the graph of  . Label the axis intercepts with their coordinates and label any asymptotes with the appropriate equation.   (3 marks)
     

     

  2. Find the area enclosed by the graph of  , the lines    and  , and the -axis.   (2 marks)

Show Answers Only
  1.  
Show Worked Solution
a.   

 

b.   
   
   
    ²

Probability, MET2 2009 VCAA 3

The Bouncy Ball Company (BBC) makes tennis balls whose diameters are normally distributed with mean 67 mm and standard deviation 1 mm. The tennis balls are packed and sold in cylindrical tins that each hold four balls. A tennis ball fits into such a tin if the diameter of the ball is less than 68.5 mm.

  1. What is the probability, correct to four decimal places, that a randomly selected tennis ball produced by BBC fits into a tin?  (2 marks)

BBC management would like each ball produced to have diameter between 65.6 and 68.4 mm.

  1. What is the probability, correct to four decimal places, that the diameter of a randomly selected tennis ball made by BBC is in this range?  (2 marks)

    1. What is the probability, correct to four decimal places, that the diameter of a tennis ball which fits into a tin is between 65.6 and 68.4 mm?  (1 mark)

    2. A tin of four balls is selected at random. What is the probability, correct to four decimal places, that at least one of these balls has diameter outside the desired range of 65.6 to 68.4 mm?  (2 marks)

BBC management wants engineers to change the manufacturing process so that 99% of all balls produced have diameter between 65.6 and 68.4 mm. The mean is to stay at 67 mm but the standard deviation is to be changed.

  1. What should the new standard deviation be (correct to two decimal places)?  (3 marks)

Show Answers Only

a. 

b. 

c.i. 

c.ii. 

d. 

Show Worked Solution

a.  

 

b.  

 

c.i.  

♦ Mean mark 42%.

 

  ii. 

♦♦ Mean mark 30%.

 

d.  

♦♦ Mean mark 35%.

 vcaa-graphs-fur2-2009-3di

 

Calculus, MET2 2009 VCAA 1

Let  

The graph of    is shown below.

VCAA 2009 1a

  1. State the interval for which the graph of is strictly decreasing.   (2 marks)

  2. Points and are the points of intersection of    with the -axis. Point has coordinates  and point has coordinates .

     

    Find the length of such that the area of rectangle is equal to the area of the shaded region.   (2 marks)
     
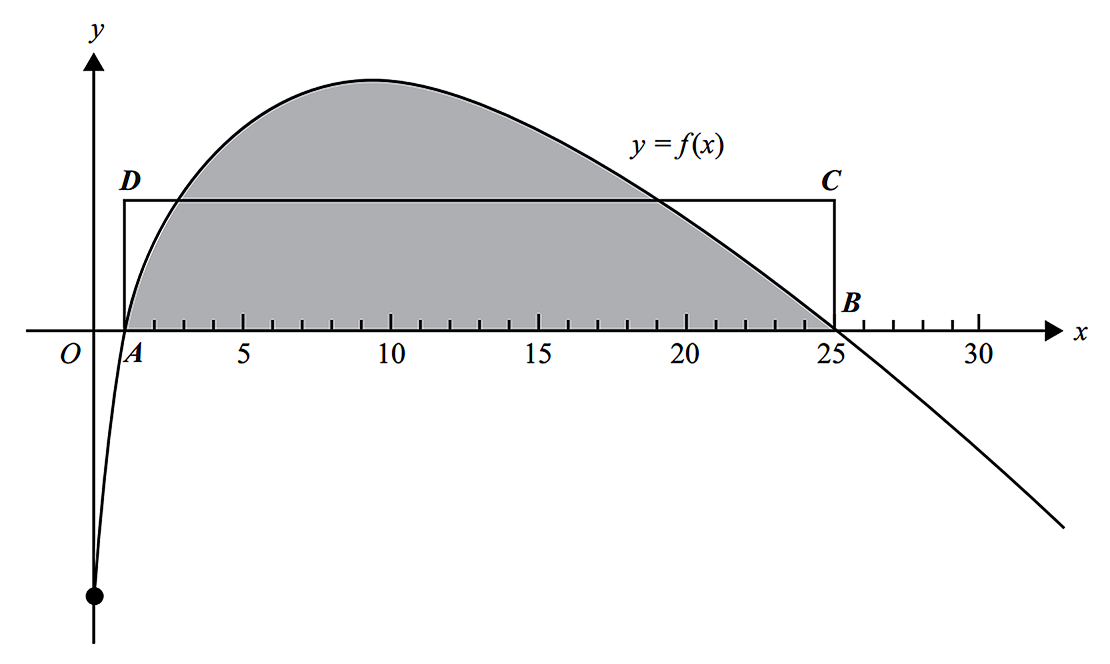
    VCAA 2009 1c

  3. The points and are labelled on the diagram.

      
     

          VCAA 2009 1d

     

    1. Find , the gradient of the chord .   (Exact value to be given.)   (1 mark)

    2. Find    such that  .  (Exact value to be given.)   (2 marks)

Show Answers Only

a. 

b. 

c.i.   

c.ii. 

Show Worked Solution
a.   vcaa-2009-1ai

 

 

b.   vcaa-2009-1ci
 
 

 

c.i.  
 

 

 c.ii.  
 

Calculus, MET2 2011 VCAA 3

  1. Consider the function  .

     

    1. Find     (1 mark)

    2. Explain why   for all .   (1 mark)

  2. The cubic function is defined by  , where , , and are real numbers.

     

    1. If has stationary points, what possible values can have?   (1 mark)

    2. If has an inverse function, what possible values can have?   (1 mark)

  3. The cubic function is defined by  .

     

    1. Write down a expression for  .   (2 marks)

    2. Determine the coordinates of the point(s) of intersection of the graphs of    and  .   (2 marks)

  4. The cubic function is defined by  , where and are real numbers.

     

    1. If has exactly one stationary point, find the value of .   (3 marks)

    2. If this stationary point occurs at a point of intersection of    and  , find the value of .   (3 marks)

Show Answers Only
Show Worked Solution

a.i.  
  

a.ii.  

♦ Mean mark 47%.

 

b.i.  

♦♦♦ Mean mark part (b)(i) 9%, and part (b)(ii) 20%.
MARKER’S COMMENT: Good exam strategy should point students to investigate earlier parts for direction. Here, part (a) clearly sheds light on a solution!

 

b.ii.  

 

c.i.  


  

c.ii. 

   

 


  

♦ Mean mark part (d)(i) 44%.
d.i.   
 
 
 
 

 

d.ii.  

♦♦♦ Mean mark part (d)(ii) 14%.

 

Probability, MET2 2016 VCAA 3*

A school has a class set of 22 new laptops kept in a recharging trolley. Provided each laptop is correctly plugged into the trolley after use, its battery recharges.

On a particular day, a class of 22 students uses the laptops. All laptop batteries are fully charged at the start of the lesson. Each student uses and returns exactly one laptop. The probability that a student does not correctly plug their laptop into the trolley at the end of the lesson is 10%. The correctness of any student’s plugging-in is independent of any other student’s correctness.

  1. Determine the probability that at least one of the laptops is not correctly plugged into the trolley at the end of the lesson. Give your answer correct to four decimal places.   (2 marks)

  2. A teacher observes that at least one of the returned laptops is not correctly plugged into the trolley.
  3. Given this, find the probability that fewer than five laptops are not correctly plugged in. Give your answer correct to four decimal places.   (2 marks)

The time for which a laptop will work without recharging (the battery life) is normally distributed, with a mean of three hours and 10 minutes and standard deviation of six minutes. Suppose that the laptops remain out of the recharging trolley for three hours.

  1. For any one laptop, find the probability that it will stop working by the end of these three hours. Give your answer correct to four decimal places.   (2 marks)

A supplier of laptops decides to take a sample of 100 new laptops from a number of different schools. For samples of size 100 from the population of laptops with a mean battery life of three hours and 10 minutes and standard deviation of six minutes,  is the random variable of the distribution of sample proportions of laptops with a battery life of less than three hours.

  1. Find the probability that . Give your answer correct to three decimal places. Do not use a normal approximation.   (3 marks)

It is known that when laptops have been used regularly in a school for six months, their battery life is still normally distributed but the mean battery life drops to three hours. It is also known that only 12% of such laptops work for more than three hours and 10 minutes.

  1. Find the standard deviation for the normal distribution that applies to the battery life of laptops that have been used regularly in a school for six months, correct to four decimal places.   (2 marks)

The laptop supplier collects a sample of 100 laptops that have been used for six months from a number of different schools and tests their battery life. The laptop supplier wishes to estimate the proportion of such laptops with a battery life of less than three hours.

  1. Suppose the supplier tests the battery life of the laptops one at a time.
  2. Find the probability that the first laptop found to have a battery life of less than three hours is the third one.   (1 mark)

The laptop supplier finds that, in a particular sample of 100 laptops, six of them have a battery life of less than three hours.

  1. Determine the 95% confidence interval for the supplier’s estimate of the proportion of interest. Give values correct to two decimal places.   (1 mark)

  2. The supplier also provides laptops to businesses. The probability density function for battery life, (in minutes), of a laptop after six months of use in a business is
     

     


     

  3. Find the mean battery life, in minutes, of a laptop with six months of business use, correct to two decimal places.   (1 mark)

Show Answers Only

Show Worked Solution

a.  

 

 
 

 

 b.   

MARKER’S COMMENT: Early rounding was a common mistake, producing 0.9312.

 

c.   

MARKER’S COMMENT: Some working must be shown for full marks in questions worth more than 1 mark.

 

d.   

♦ Mean mark part (d) 33%.

 
 
 

 

e.   

 

f.   
   

 

g.   

 

h.   
 

Calculus, MET2 2016 VCAA 2

Consider the function  

  1.  i. Given that  ,
  2.      show that  .   (1 mark)

  3. ii. Find the values of for which the graph of    has a stationary point.   (1 mark)

The diagram below shows part of the graph of  , the tangent to the graph at    and a straight line drawn perpendicular to the tangent to the graph at  . The equation of the tangent at the point with coordinates    is  .

The tangent cuts the -axis at . The line perpendicular to the tangent cuts the -axis at .
 


 

  1.   i. Find the coordinates of .   (1 mark)

  2.  ii. Find the equation of the line that passes through and and, hence, find the coordinates of .   (2 marks)

  3. iii. Find the area of triangle .   (2 marks)

  4. The tangent at is parallel to the tangent at . It intersects the line passing through and at .

     


     
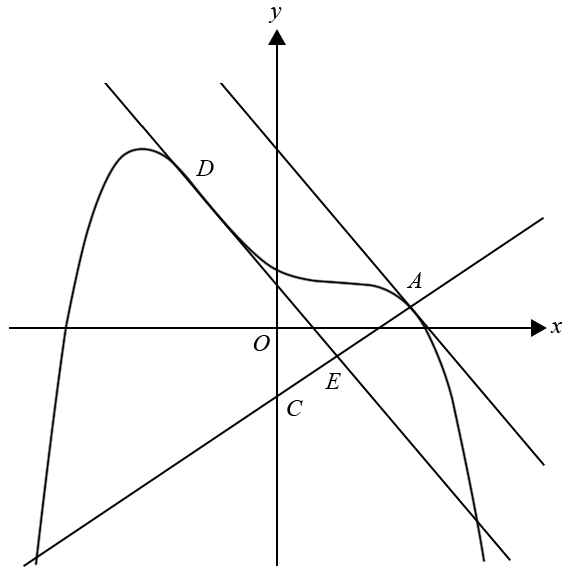
     i. Find the coordinates of .   (2 marks)

  5. ii. Find the length of .   (3 marks)

Show Answers Only
  1.  i.
  2. ii.
  3.   i.
  4. ii.
  5.      
  6. iii. ²
Show Worked Solution
a.i.   
   
   
 

 

  

 

a.ii.   


 

b.i.   


 

b.ii.   

   

   


 

b.iii.  
   
    ²

 

♦ Mean mark part (c)(i) 43%.
MARKER’S COMMENT: Many students gave an incorrect -value here. Be careful!
c.i.   
 
 
   

  

 

c.ii.  

 

♦♦ Mean mark 32%.

 

 

 

Calculus, MET2 2016 VCAA 1

Let  .

  1. Find the period and range of .   (2 marks)

  2. State the rule for the derivative function .   (1 mark)

  3. Find the equation of the tangent to the graph of at  .   (1 mark)

  4. Find the equations of the tangents to the graph of    that have a gradient of 1.   (2 marks)

  5. The rule of   can be obtained from the rule of  under a transformation , such that
      

     

     

    Find the value of and the value of .   (3 marks)

  6. Find the values of  , such that  .   (2 marks)

Show Answers Only
Show Worked Solution

a.   

MARKER’S COMMENT: Including round brackets rather than square ones was a common mistake.


  

b.   
 

c.  


 

d.   

♦ Mean mark part (d) 50%.

 

e.  

♦♦ Mean mark part (e) 27%.
   

 
   

 

f.   

♦ Mean mark part (f) 50%.

Graphs, MET2 2016 VCAA 8 MC

The UV index, , for a summer day in Melbourne is illustrated in the graph below, where is the number of hours after 6 am.
 

     
 

The graph is most likely to be the graph of

A.  

B.  

C.  

D.  

E.  

Show Answers Only

Show Worked Solution

 

CORE, FUR2 SM-Bank VCE 2

Spiro is saving for a car. He has an account with $3500 in it at the start of the year.

At the end of each month, Spiro adds another $180 to the account.

The account pays 3.6% interest per annum, compounded monthly.

    1. What is the interest rate per month?   (1 mark)
    2. Write a recurrence relation that models Spiro's investment, with representing the balance of his account after months.   (1 mark)
  2. What will be the balance of Spiro's account after 3 months?
  3. Write your answer correct to the nearest cent.   (1 mark)

Show Answers Only

Show Worked Solution

a.i.   
   

 

a.ii.   
   
   
     
     

  


  

b.   
 
   

CORE, FUR2 SM-Bank VCE 1

Joe buys a tractor under a buy-back scheme. This scheme gives Joe the right to sell the tractor back to the dealer through either a flat rate depreciation or unit cost depreciation.

  1. The recurrence relation below can be used to calculate the price Joe sells the tractor back to the dealer , based on the flat rate depreciation, after years
     

     

    1. Write the general rule to find the value of in terms of .?   (1 mark)
    2. Hence or otherwise, find the time it will take Joe's tractor to lose half of its value.   (1 mark)
  2. Joe uses the unit cost method to depreciate his tractor, he depreciates $2.75 per kilometre travelled.
    1. How many kilometres does Joe's tractor need to travel for half its value to be depreciated? Round your answer to the nearest kilometre?   (1 mark)
    2. Joe's tractor travels, on average, 2500 kilometres per year. Which method, flat rate depreciation or unit cost depreciation, will result in the greater annual depreciation? Write down the greater depreciation amount correct to the nearest dollar.   (1 mark)

Show Answers Only

  1. i. 
    ii.
  2. i.
    ii.
         

Show Worked Solution

a.i.   
 
   
   
     

 

a.ii.   

 

b.i.   
   
   

  
b.ii.  


 

 

NETWORKS, FUR2 2016 VCAA 2

The suburb of Alooma has a skateboard park with seven ramps.

The ramps are shown as vertices , , , , , and on the graph below.
 

 

The tracks between ramps and and between ramps and are rough, as shown on the graph above.

  1. Nathan begins skating at ramp and follows an Eulerian trail.

     

    At which ramp does Nathan finish?   (1 mark)

  2. Zoe begins skating at ramp and follows a Hamiltonian path.

     

    The path she chooses does not include the two rough tracks.

     

    Write down a path that Zoe could take from start to finish.   (1 mark)

  3. Birra can skate over any of the tracks, including the rough tracks.

     

    He begins skating at ramp and will complete a Hamiltonian cycle.

     

    In how many ways could he do this?   (1 mark) 

Show Answers Only

Show Worked Solution

a.  

“XYZWVZUYTU`


  

b.  


  

c.  

♦♦ Mean mark 31%.

GRAPHS, FUR2 2016 VCAA 2

The bonus money is provided by a company that manufactures and sells hockey balls.

The cost, in dollars, of manufacturing a certain number of balls can be found using the equation

cost = 1200 + 1.5 × number of balls

  1. How many balls would be manufactured if the cost is $1650?  (1 mark)
  2. On the grid below, sketch the graph of the relationship between the manufacturing cost and the number of balls manufactured.  (1 mark)

     

  3. The company will break even on the sale of hockey balls when it manufactures and sells 200 hockey balls.

     

    Find the selling price of one hockey ball.  (1 mark) 

Show Answers Only

Show Worked Solution
a.   
 
   

 

b.   

 

c.  

 

 

GRAPHS, FUR2 2016 VCAA 1

Maria is a hockey player. She is paid a bonus that depends on the number of goals that she scores in a season.

The graph below shows the value of Maria’s bonus against the number of goals that she scores in a season.

  1. What is the value of Maria’s bonus if she scores seven goals in a season?  (1 mark) 
  2. What is the least number of goals that Maria must score in a season to receive a bonus of $2500?  (1 mark)

Another player, Bianca, is paid a bonus of $125 for every goal that she scores in a season.

  1. What is the value of Bianca’s bonus if she scores eight goals in a season?  (1 mark)
  2. At the end of the season, both players have scored the same number of goals and receive the same bonus amount.

     

    How many goals did Maria and Bianca each score in the season?  (1 mark)

Show Answers Only
Show Worked Solution

a.  

 

b.  

 

c.   
   

 

d.  

MATRICES, FUR2 2016 VCAA 2

A travel company has five employees, Amara (), Ben (), Cheng (), Dana () and Elka ().

The company allows each employee to send a direct message to another employee only as shown in the communication matrix below.

The matrix is also shown below.
 


 

The 1 in row , column of matrix indicates that Elka (sender) can send a direct message to Dana (receiver).

The 0 in row , column of matrix indicates that Elka cannot send a direct message to Cheng.

  1. To whom can Dana send a direct message?   (1 mark)

  2. Cheng needs to send a message to Elka, but cannot do this directly.
  3. Write down the names of the employees who can send the message from Cheng directly to Elka.   (1 mark) 

Show Answers Only
Show Worked Solution

a.  
 

b.  

MATRICES, FUR2 2016 VCAA 1

A travel company arranges flight (), hotel (), performance () and tour () bookings.

Matrix contains the number of each type of booking for a month.

  1. Write down the order of matrix .   (1 mark)

A booking fee, per person, is collected by the travel company for each type of booking.

Matrix contains the booking fees, in dollars, per booking.

  1.  i. Calculate the matrix product  .   (1 mark)

  2. ii. What does matrix represent?   (1 mark) 

Show Answers Only

Show Worked Solution

a.  
 

b.i.   

 
b.ii.  

♦ Mean mark 42%.

 

GEOMETRY, FUR2 2016 VCAA 2

Salena practises golf at a driving range by hitting golf balls from point  .

The first ball that Salena hits travels directly north, landing at point  .

The second ball that Salena hits travels 50 m on a bearing of 030°, landing at point  .

The diagram below shows the positions of the two balls after they have landed.
  

  1. How far apart, in metres, are the two golf balls?  (1 mark)
  2. A fence is positioned at the end of the driving range.

     

    The fence is 16.8 m high and is 200 m from the point  .


     
     
    What is the angle of elevation from   to the top of the fence?

     

    Round your answer to the nearest degree.  (1 mark) 

Show Answers Only
Show Worked Solution

a.  

 

 

b.  

 
 

GEOMETRY, FUR2 2016 VCAA 1

A golf ball is spherical in shape and has a radius of 21.4 mm, as shown in the diagram below.

Assume that the surface of the golf ball is smooth.

  1. What is the surface area of the golf ball shown?

     

    Round your answer to the nearest square millimetre.  (1 mark)

  2. Golf balls are sold in a rectangular box that contains five identical golf balls, as shown in the diagram below.

     


     

    What is the minimum length, in millimetres, of the box?  (1 mark)

Show Answers Only
  1. ²
Show Worked Solution
a.   
   
   
    ²

 

b.   
   

CORE, FUR2 2016 VCAA 5

Ken has opened a savings account to save money to buy a new caravan.

The amount of money in the savings account after years, , can be modelled by the recurrence relation shown below.

  1. How much money did Ken initially deposit into the savings account?  (1 mark)

  2. Use recursion to write down calculations that show that the amount of money in Ken’s savings account after two years, , will be $16 224.  (1 mark)

  3. What is the annual percentage compound interest rate for this savings account?  (1 mark)

  4. The amount of money in the account after years, , can also be determined using a rule.
    i.     Complete the rule below by writing the appropriate numbers in the boxes provided.   (1 mark)

     
     
    		 ­
     
  5. ii. How much money will be in Ken’s savings account after 10 years?   (1 mark)

Show Answers Only
  4. i.
    ii.  
Show Worked Solution
a.   
   
b.   
 
   
 
   

MARKER’S COMMENT: (b) Stating is not using recursion as required here and did not gain a mark.
c.   
   

 

d.i.  
d.ii.   
   
   

♦ Mean mark (d)(ii) 47%.
MARKER’S COMMENT: Rounding to $22 203.70 lost a mark!

CORE, FUR2 2016 VCAA 3

The data in the table below shows a sample of actual temperatures and apparent temperatures recorded at a weather station. A scatterplot of the data is also shown.

The data will be used to investigate the association between the variables apparent temperature and actual temperature.
 

  1. Use the scatterplot to describe the association between apparent temperature and actual temperature in terms of strength, direction and form.   (1 mark)

  2.  i. Determine the equation of the least squares line that can be used to predict the apparent temperature from the actual temperature.
  3. Write the values of the intercept and slope of this least squares line in the appropriate boxes provided below.
  4. Round your answers to two significant figures.   (3 marks)
     apparent temperature    
 
  
 
   actual temperature
  1. ii. Interpret the intercept of the least squares line in terms of the variables apparent temperature and actual temperature.   (1 mark)

  2. The coefficient of determination for the association between the variables apparent temperature and actual temperature is 0.97
  3. Interpret the coefficient of determination in terms of these variables.   (1 mark)

  4. The residual plot obtained when the least squares line was fitted to the data is shown below.
     
     
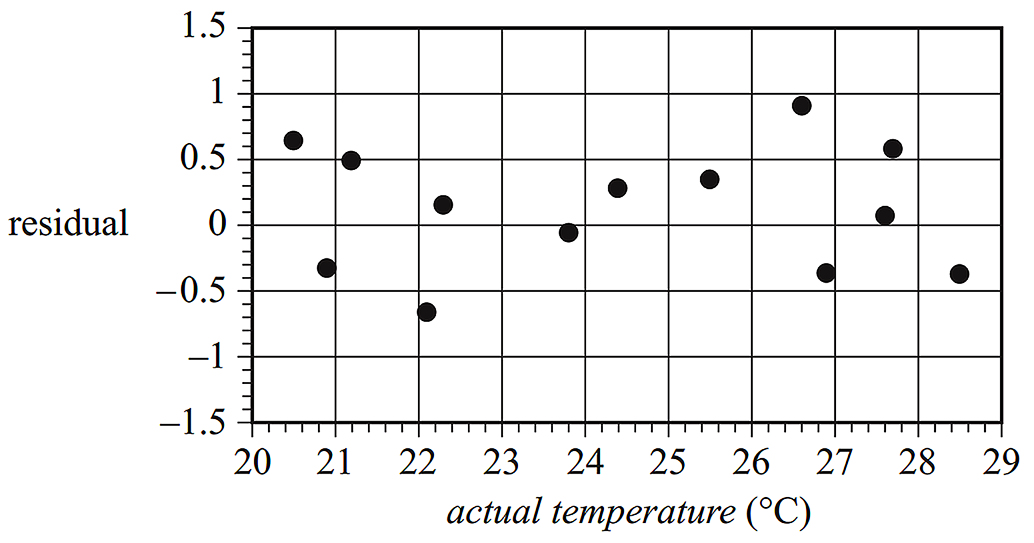
  5.  i. A residual plot can be used to test an assumption about the nature of the association between two numerical variables.
  6.     What is this assumption?   (1 mark)
  7. ii. Does the residual plot above support this assumption? Explain your answer.   (1 mark)
Show Answers Only

a.   

b.i. 

b.ii.  `text(When actual temperature is 0°C, on average,)`

c. 

d.i. 

d.ii. 

Show Worked Solution

a.   
 

b.i.   


 

♦♦ Mean mark of part (b)(ii) – 28%.
MARKER’S COMMENT: “the predicted apparent temp is -1.7°C” also gained a mark.
b.ii.   
 

 

♦ Mean mark 49%.
IMPORTANT: Any mention of causality loses a mark!

c. 


  

d.i. 

♦ Mean mark of both parts of (d) was 46%.

d.ii. 

CORE, FUR2 2016 VCAA 2

A weather station records daily maximum temperatures.

  1. The five-number summary for the distribution of maximum temperatures for the month of February is displayed in the table below.

 

  1. There are no outliers in this distribution.
  2.  i. Use the five-number summary above to construct a boxplot on the grid below.   (1 mark)

 

  1. ii. What percentage of days had a maximum temperature of 21°C, or greater, in this particular February?   (1 mark)

  2. The boxplots below display the distribution of maximum daily temperature for the months of May and July.
     

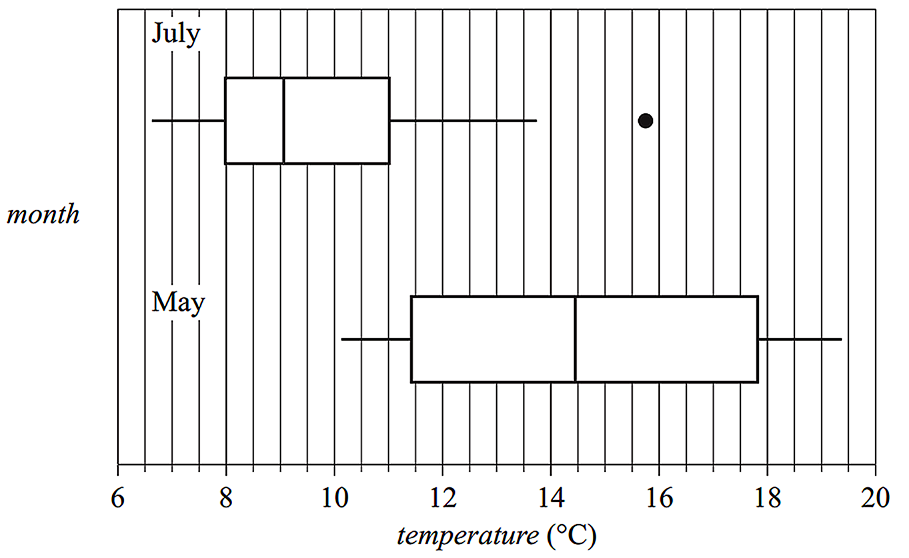
  3.   i. Describe the shapes of the distributions of daily temperature (including outliers) for July and for May.   (1 mark)

  4.  ii. Determine the value of the upper fence for the July boxplot.   (1 mark)

  5. iii. Using the information from the boxplots, explain why the maximum daily temperature is associated with the month of the year. Quote the values of appropriate statistics in your response.   (1 mark)

Show Answers Only
a.i.   

a.ii.  

b.i.   
 

b.ii. 

b.iii.

Show Worked Solution
a.i.   

a.ii.  

MARKER’S COMMENT: Incorrect May descriptors included “evenly or normally distributed”, “bell shaped” and “symmetrically skewed.”
b.i.   
 

 

b.ii.   
   
   
   
♦♦ Mean mark (b)(iii) – 30%.
COMMENT: Refer to the difference in medians. Just quoting the numbers was not enough to gain a mark here.

b.iii.

CORE, FUR2 2016 VCAA 1

The dot plot below shows the distribution of daily rainfall, in millimetres, at a weather station for 30 days in September.
 

 

  1. Write down the
  2.  i. range   (1 mark)

  3. ii. median   (1 mark)

  1. Circle the data point on the dot plot above that corresponds to the third quartile    (1 mark)

  2. Write down the
  3.  i. the number of days on which no rainfall was recorded.   (1 mark)

  4. ii. the percentage of days on which the daily rainfall exceeded 12 mm.   (1 mark)

  1. Use the grid below to construct a histogram that displays the distribution of daily rainfall for the month of September. Use interval widths of two with the first interval starting at 0.   (2 marks) 

  

Show Answers Only

  2.  

Show Worked Solution
a.i.   
   
   
     

a.ii.  

 

 

b.   

 

c.i.   

c.ii.   
   

 

d.   

GRAPHS, FUR1 2016 VCAA 3 MC

The graph below shows a straight line that passes through the points (6, 6) and (8, 9).

The coordinates of the point where the line crosses the -axis are

Show Answers Only

Show Worked Solution
 
 

 

 

 

MATRICES, FUR1 2016 VCAA 6 MC

Families in a country town were asked about their annual holidays.

Every year, these families choose between staying at home (H), travelling (T) and camping (C).

The transition diagram below shows the way families in the town change their holiday preferences from year to year.

 

A transition matrix that provides the same information as the transition diagram is

A. B.
       
C. D.
       
E.    
Show Answers Only

Show Worked Solution

CORE, FUR1 2016 VCAA 14-16 MC

The table below shows the long-term average of the number of meals served each day at a restaurant. Also shown is the daily seasonal index for Monday through to Friday.

 

Part 1

The seasonal index for Wednesday is 0.84

This tells us that, on average, the number of meals served on a Wednesday is

  1. 16% less than the daily average.
  2. 84% less than the daily average.
  3. the same as the daily average.
  4. 16% more than the daily average.
  5. 84% more than the daily average.

 

Part 2

Last Tuesday, 108 meals were served in the restaurant.

The deseasonalised number of meals served last Tuesday was closest to

  1.  

 

Part 3

The seasonal index for Saturday is closest to

Show Answers Only

Show Worked Solution

 

 

 

CORE, FUR1 2016 VCAA 13 MC

Consider the time series plot below.
 


 

The pattern in the time series plot shown above is best described as having

  1. irregular fluctuations only.
  2. an increasing trend with irregular fluctuations.
  3. seasonality with irregular fluctuations.
  4. seasonality with an increasing trend and irregular fluctuations.
  5. seasonality with a decreasing trend and irregular fluctuations.
Show Answers Only

Show Worked Solution

CORE, FUR1 2016 VCAA 1-2 MC

The blood pressure (low, normal, high) and the age (under 50 years, 50 years or over) of 110 adults were recorded. The results are displayed in the two-way frequency table below.
 

     

Part 1

The percentage of adults under 50 years of age who have high blood pressure is closest to

  1.  11%
  2.  19%
  3.  26%
  4.  44%
  5.  58%

Part 2

The variables blood pressure (low, normal, high) and age (under 50 years, 50 years or over) are

  1. both nominal variables.
  2. both ordinal variables.
  3. a nominal variable and an ordinal variable respectively.
  4. an ordinal variable and a nominal variable respectively.
  5. a continuous variable and an ordinal variable respectively.
Show Answers Only

Show Worked Solution

 
 

 

 

♦♦ Mean mark of Part 2: 31%.
MARKER’S COMMENT: Many students incorrectly identified the age (under 50, 50 or over) as nominal.