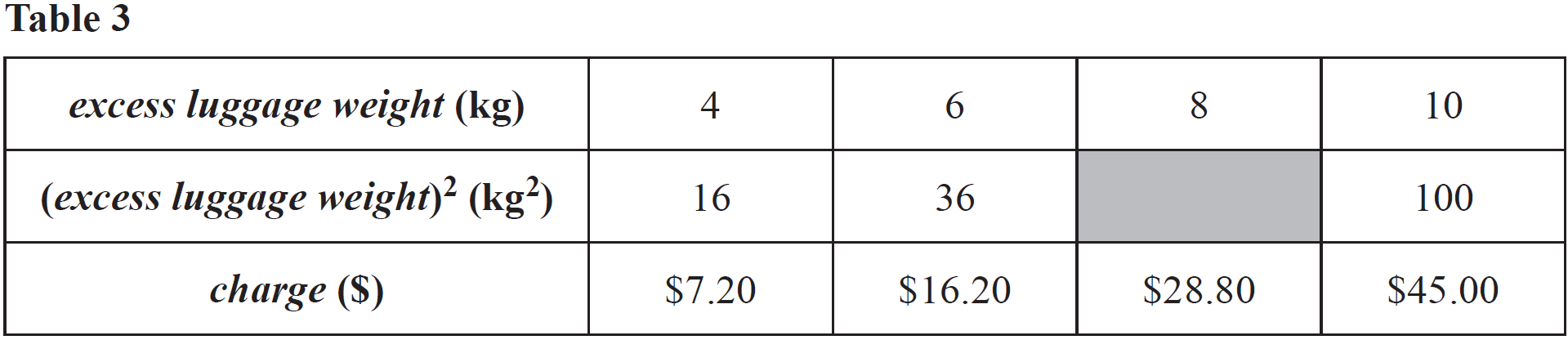SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Probability, MET1 2009 VCAA 5

Four identical balls are numbered 1, 2, 3 and 4 and put into a box. A ball is randomly drawn from the box, and not returned to the box. A second ball is then randomly drawn from the box.

  1. What is the probability that the first ball drawn is numbered 4 and the second ball drawn is numbered 1?  (1 mark)

  2. What is the probability that the sum of the numbers on the two balls is 5?  (1 mark)

  3. Given that the sum of the numbers on the two balls is 5, what is the probability that the second ball drawn is numbered 1?  (2 marks)

Show Answers Only

Show Worked Solution

a.  

 

b.  

 

c.  

♦ Mean mark 46%.

Calculus, MET1 2010 VCAA 9

Part of the graph of    is shown below.

vcaa-2010-meth-9a

  1. Find the derivative of  .   (1 mark)

  2. Use your answer to part a. to find the area of the shaded region in the form    where and are non-zero real constants.   (3 marks)

Show Answers Only
  2. ²
Show Worked Solution

a.  

 

 

b.  

♦♦ Mean mark 33%.
MARKER’S COMMENT: The most common error was not dividing everything through by 2. Be careful!

 

 
 
  ²

Functions, MET1 2011 VCAA 4

If the function has the rule    and the function has the rule  

  1.  find integers and such that    (2 marks)

  2.  state the maximal domain for which  is defined.   (2 marks)

Show Answers Only
Show Worked Solution
a.  
   
   

 
 

 

b.   

♦♦♦ Mean mark 13%.
MARKER’S COMMENT: “Very poorly answered” with a common response of that ignored the information from part (a).


 

 vcaa-2011-meth-4ii

Functions, MET1 2012 VCAA 6

The graphs of  ,  where is a real constant, have a point of intersection at  

  1. Find the value of .  (2 marks)

  2. If  , find the -coordinate of the other point of intersection of the two graphs.  (1 mark)

Show Answers Only
Show Worked Solution

a.  

 

b.  
 
 

Probability, MET1 2012 VCAA 4

On any given day, the number of telephone calls that Daniel receives is a random variable with probability distribution given by

vcaa-2012-meth-4

  1. Find the mean of .   (2 marks)

  2. What is the probability that Daniel receives only one telephone call on each of three consecutive days?   (1 mark)

  3. Daniel receives telephone calls on both Monday and Tuesday.
  4. What is the probability that Daniel receives a total of four calls over these two days?   (3 marks)

Show Answers Only

Show Worked Solution

a.   
   
   

 

b.  

MARKER’S COMMENT: Many students understood that the calculation of 0.2³ was required but were not able evaluate correctly.

 

c.  

♦ Mean mark 36%.

Calculus, MET1 2014 VCAA 5

Consider the function  ,  .

  1. Find the coordinates of the stationary points of the function.   (2 marks)

  2. On the axes  below, sketch the graph of .

     

    Label any end points with their coordinates.   (2 marks)

     

     
        met1-2014-vcaa-q5

     

  3. Find the area enclosed by the graph of the function and the horizontal line given by  .   (3 marks)

Show Answers Only
  2.  
    met1-2014-vcaa-q5-answer3
  3. ²
Show Worked Solution
a.   
   
 


 

 

b.    met1-2014-vcaa-q5-answer3

 

♦ Mean mark (c) 48%.
c.    met1-2014-vcaa-q5-answer4

 
 
 
   
²

 

 
 
  ²

Probability, MET1 2015 VCAA 8

For events  and  from a sample space,  and  .

  1. Calculate  .   (1 mark)

  2. Calculate  , where denotes the complement of .   (1 mark)

  3. If events and are independent, calculate  .   (1 mark)

Show Answers Only

Show Worked Solution

a.  

 

b.    met1-2015-vcaa-q8-answer
 
 

 

c.  

♦♦ Mean mark 28%.
MARKER’S COMMENT: A lack of understanding of independent events was clearly evident.

 

 

Calculus, MET1 2015 VCAA 4

Consider the function  .

  1. Find the coordinates of the stationary points of the function.   (2 marks)

The rule for   can also be expressed as  .

  1. On the axes below, sketch the graph of  , clearly indicating axis intercepts and turning points.

     

    Label the end points with their coordinates.   (2 marks)


      

    met1-2015-vcaa-q4
     

  2. Find the average value of   over the interval    (2 marks)

Show Answers Only
  2.  

     

    met1-2015-vcaa-q4-answer

Show Worked Solution

a.  

 

 

 

b.    met1-2015-vcaa-q4-answer
♦ Part (c) mean mark 50%.
MARKER’S COMMENT: Most students recalled the average value definition but then did not integrate correctly.

 

c.   
   
   
   
   

CORE*, FUR2 2006 VCAA 1

A company purchased a machine for $60 000.

For taxation purposes the machine is depreciated over time.

Two methods of depreciation are considered.

  1. Flat rate depreciation

    The machine is depreciated at a flat rate of 10% of the purchase price each year.

    i.      By how many dollars will the machine depreciate annually?   (1 mark)

    ii.  Calculate the value of the machine after three years.   (1 mark)

  2. iii. After how many years will the machine be $12 000 in value?   (1 mark)

  3. Reducing balance depreciation

    The value, , of the machine after years is given by the formula .

    i.      By what percentage will the machine depreciate annually?   (1 mark)

    ii.  Calculate the value of the machine after three years.   (1 mark)

  4. iii. At the end of which year will the machine's value first fall below $12 000?   (1 mark)

  1. At the end of which year will the value of the machine first be less using flat rate depreciation than it will be using reducing balance depreciation?  (2 marks)

Show Answers Only
  1. i.   
    ii. 
    iii.
  2. i.    
    ii. 
    iii.
Show Worked Solution
a.i.   
   

a.ii.  

 

a.iii.  

 

 

b.i.   
   


  

b.ii.  

 

 

b.iii.  

  


  

c.   

BUSINESS, FUR2 2006 VCAA 1 Answer

CORE*, FUR2 2007 VCAA 3

Khan paid $900 for a fax machine.

This price includes 10% GST (goods and services tax).

  1. Determine the price of the fax machine before GST was added. Write your answer correct to the nearest cent.  (1 mark)

  2. Khan will depreciate his $900 fax machine for taxation purposes.
  3. He considers two methods of depreciation.
  4. Flat rate depreciation
  5. Under flat rate depreciation the fax machine will be valued at $300 after five years.
    1. Calculate the annual depreciation in dollars.   (1 mark)
    2. ii. Determine the value of the fax machine after five years.   (1 mark)

Show Answers Only

  2. i.
    ii.  

Show Worked Solution

a.  

MARKER’S COMMENT: Reverse GST questions continue to cause problems for many students.

 
 

  
b.i.  

 

b.ii.  

CORE*, FUR2 2007 VCAA 1

Khan wants to buy some office furniture that is valued at $7000.

    1. A store requires 25% deposit. Calculate the deposit.   (1 mark)
    2. The balance is to be paid in 24 equal monthly instalments. No interest is charged.
    3. Determine the amount of each instalment. Write your answer in dollars and cents.   (1 mark)

Another store offers the same $7000 office furniture for $500 deposit and 36 monthly instalments of $220.

 

    1. Determine the total amount paid for the furniture at this store.   (1 mark)
    2. Calculate the annual flat rate of interest charged by this store.
    3. Write your answer as a percentage correct to one decimal place.   (2 marks)

A third store has the office furniture marked at $7000 but will give 15% discount if payment is made in cash at the time of sale.

  1. Calculate the cash price paid for the furniture after the discount is applied.   (1 mark) 

Show Answers Only

  1. i. 
  2. ii.
  3. i. 
  4. ii. 

Show Worked Solution

a.i.   
   

 
a.ii.  


 

b.i.   
   

 

b.ii.  

 

 
 

 

c.   
   
   

GRAPHS, FUR2 2007 VCAA 2

The Goldsmiths’ car can use either petrol or gas. 

The following equation models the fuel usage of petrol, , in litres per 100 km (L/100 km) when the car is travelling at an average speed of km/h.

The line    is drawn on the graph below for average speeds up to 110 km/h.

GRAPHS, FUR2 2007 VCAA 2

  1. Determine how many litres of petrol the car will use to travel 100 km at an average speed of 60 km/h.

     

    Write your answer correct to one decimal place.  (1 mark)

The following equation models the fuel usage of gas, , in litres per 100 km (L/100 km) when the car is travelling at an average speed of km/h.

  1. On the axes above, draw the line    for average speeds up to 110 km/h.  (1 mark)
  2. Determine the average speeds for which fuel usage of gas will be less than fuel usage of petrol.  (1 mark)

The Goldsmiths'’ car travels at an average speed of 85 km/h. It is using gas.

Gas costs 80 cents per litre.

  1. Determine the cost of the gas used to travel 100 km.

     

    Write your answer in dollars and cents.  (2 marks)

Show Answers Only
  2.  
    GRAPHS, FUR2 2007 VCAA 2 Answer
Show Worked Solution

a.  

 

 

b.  

GRAPHS, FUR2 2007 VCAA 2 Answer

 

c.   

MARKER’S COMMENT: Since fuel usage is less for gas, a speed of 75 km/hr was incorrect, as it was equal.

 

 

d.  

 

 

GRAPHS, FUR2 2007 VCAA 1

The Goldsmith family are going on a driving holiday in Western Australia.

On the first day, they leave home at 8 am and drive to Watheroo then Geraldton.

The distance––time graph below shows their journey to Geraldton.

GRAPHS, FUR2 2007 VCAA 1

At 9.30 am the Goldsmiths arrive at Watheroo.

They stop for a period of time.

  1. For how many minutes did they stop at Watheroo?  (1 mark)

After leaving Watheroo, the Goldsmiths continue their journey and arrive in Geraldton at 12 pm.

  1. What distance (in kilometres) do they travel between Watheroo and Geraldton?  (1 mark)
  2. Calculate the Goldsmiths'’ average speed (in km/h) when travelling between Watheroo and Geraldton.  (1 mark)

The Goldsmiths leave Geraldton at 1 pm and drive to Hamelin. They travel at a constant speed of 80 km/h for three hours. They do not make any stops.

  1. On the graph above, draw a line segment representing their journey from Geraldton to Hamelin.  (1 mark)

 

Show Answers Only
  4.  
    GRAPHS, FUR2 2007 VCAA 1 Answer
Show Worked Solution

a.  

 

b.  

 

c.  

 

 

d.    GRAPHS, FUR2 2007 VCAA 1 Answer

 

Calculus, MET2 2010 VCAA 1

  1. Part of the graph of the function    is shown on the axes below

     

         

    1. Find the rule and domain of  , the inverse function of  .   (3 marks)

    2. On the set of axes above sketch the graph of  . Label the axes intercepts with their exact values.   (3 marks)

    3. Find the values of , correct to three decimal places, for which  .   (2 marks)

    4. Calculate the area enclosed by the graphs of    and  . Give your answer correct to two decimal places.   (2 marks)

  2. The diagram below shows part of the graph of the function with rule
  3.         , where , and are real constants.
     

    • The graph has a vertical asymptote with equation  .
    • The graph has a y-axis intercept at 1.
    • The point on the graph has coordinates  , where is another real constant.
       

      VCAA 2010 1b

    1. State the value of .   (1 mark)

    2. Find the value of .   (1 mark)

    3. Show that  .   (2 marks)

    4. Show that the gradient of the tangent to the graph of at the point is  .   (1 mark)

    5. If the point    lies on the tangent referred to in part b.iv., find the exact value of .   (2 marks)

Show Answers Only
  1.   i.
  2.  ii. 
     
  3. iii.
  4. iv. ²
  5.   i.
  6.  ii.
  7. iii.
  8.  iv.
  9.   v.
Show Worked Solution

a.i.  

 

ii.  

 

 iii. 

 

 

  iv.  
    ²

 

b.i.  

 

  ii.  

 

iii.  

 

  iv.  
 
   
   

 

  v.  

♦♦ Mean mark 33%.
MARKER’S COMMENT: Many students worked out the equation of the tangent which was unnecessary and time consuming.

 

CORE*, FUR2 2008 VCAA 1

Michelle has a bank account that pays her simple interest.

The bank statement below shows the transactions on Michelle’s account for the month of July.
  


 

  1. What amount, in dollars, was deposited in cash on 11 July?   (1 mark)

Interest for this account is calculated on the minimum monthly balance at a rate of 3% per annum.

  1. Calculate the interest for July, correct to the nearest cent.   (2 marks)

Show Answers Only
Show Worked Solution
a.   
   

 

b.  

 
 
 

GRAPHS, FUR2 2008 VCAA 3

An event involves running for 10 km and cycling for 30 km.

Let    be the time taken (in minutes) to run 10 km

   be the time taken (in minutes) to cycle 30 km

Event organisers set constraints on the time taken, in minutes, to run and cycle during the event.

Inequalities 1 to 6 below represent all time constraints on the event.

Inequality 1:   Inequality 4:  
Inequality 2:   Inequality 5:  
Inequality 3:   Inequality 6:  

 

  1. Explain the meaning of Inequality 3 in terms of the context of this problem.  (1 mark)

 

The lines    and    are drawn on the graph below.

GRAPHS, FUR2 2008 VCAA 3

  1. On the graph above

     

    1. draw and label the lines    and    (2 marks)
    2. clearly shade the feasible region represented by Inequalities 1 to 6.  (1 mark)

One competitor, Jenny, took 100 minutes to complete the run.

  1. Between what times, in minutes, can she complete the cycling and remain within the constraints set for the event?  (1 mark)
  2. Competitors who complete the event in 90 minutes or less qualify for a prize. 

     

    Tiffany qualified for a prize.

     

    1. Determine the maximum number of minutes for which Tiffany could have cycled.  (1 mark)
    2. Determine the maximum number of minutes for which Tiffany could have run.  (1 mark)
Show Answers Only

  2.  i. & ii.
    GRAPHS, FUR2 2008 VCAA 3 Answer
Show Worked Solution

a.  

 

b.i. & ii.

GRAPHS, FUR2 2008 VCAA 3 Answer

 

c.   

♦♦ Mean mark of parts (c)-(d) (combined) was 19%.

 

d.i.   

 

 

 

d.ii.   

 

GRAPHS, FUR2 2008 VCAA 2

Tiffany decides to enter a charity event involving running and cycling. 

There is a $35 fee to enter.

  1. Write an equation that gives the total amount, dollars, collected from entry fees when there are competitors in the event.  (1 mark)

The event costs the organisers $50 625 plus $12.50 per competitor.

  1. Write an equation that gives the total cost, , in dollars, of the event when there are competitors.  (1 mark)
    1. Determine the number of competitors required for the organisers to break even.  (1 mark)

The number of competitors who entered the event was 8670.

  1. Determine the profit made by the organisers.  (1 mark)
Show Answers Only
Show Worked Solution

a.   

 

b.  

 

c.i.   

 

 

 

c.ii.  

 
 
 

GRAPHS, FUR2 2008 VCAA 1

Tiffany’s pulse rate (in beats/minute) during the first 60 minutes of a long-distance run is shown in the graph below.

GRAPHS, FUR2 2008 VCAA 1

  1. What was Tiffany’s pulse rate (in beats/minute) 15 minutes after she started her run?  (1 mark)
  2. By how much did Tiffany’s pulse rate increase over the first 60 minutes of her run?

     

    Write your answer in beats/minute.  (1 mark)

  3. The recommended maximum pulse rate for adults during exercise is determinded by subtracting the person’s age in years from 220.

     

    1. Write an equation in terms of the variables maximum pulse rate and age that can be used to determine a person’s recommended maximum pulse rate from his or her age.  (1 mark)

The target zone for aerobic exercise is between 60% and 75% of a person’s maximum pulse rate.

Tiffany is 20 years of age.

  1. Determine the values between which Tiffany’s pulse rate should remain so that she exercises within her target zone.

     

    Write your answers correct to the nearest whole number.  (1 mark)

Show Answers Only
Show Worked Solution

a.  

 

b.  

 

c.i.  

 

c.ii.  

 

 

CORE*, FUR2 2009 VCAA 3

The golf club’s social committee has $3400 invested in an account which pays interest at the rate of 4.4% per annum compounding quarterly.

  1. Show that the interest rate per quarter is 1.1%.   (1 mark)

  2. Determine the value of the $3400 investment after three years.

     

    Write your answer in dollars correct to the nearest cent.   (1 mark)

  3. Calculate the interest the $3400 investment will earn over six years.

     

    Write your answer in dollars correct to the nearest cent.   (2 marks)

Show Answers Only
Show Worked Solution

a.  


 

b.   

 
 
 

 

c.  

 

 

CORE*, FUR2 2009 VCAA 2

Rebecca will need to borrow $250 to buy a golf bag.

  1. If she borrows the $250 on her credit card, she will pay interest at the rate of 1.5% per month.

     

    Calculate the interest Rebecca will pay in the first month.

     

    Write your answer correct to the nearest cent.   (1 mark)

  2. If Rebecca borrows the $250 from the store’s finance company she will pay $6 interest per month.

     

    Calculate the annual flat interest rate charged. Write your answer as a percentage correct to one decimal place.   (1 mark)

Show Answers Only
Show Worked Solution

a.  


 

b.  

CORE*, FUR2 2009 VCAA 1

The recommended retail price of a golf bag is $500. Rebecca sees the bag discounted by $120 at a sale.

  1. What is the price of the golf bag after the $120 discount has been applied?   (1 mark)

  2. Find the discount as a percentage of the recommended retail price.   ( 1 mark)

Show Answers Only
Show Worked Solution
a.   
   

 

b.   
   

 

GRAPHS, FUR2 2009 VCAA 2

Luggage over 20 kg in weight is called excess luggage.

Fair Go Airlines charges for transporting excess luggage.

The charges for some excess luggage weights are shown in Table 2.

GRAPHS, FUR2 2009 VCAA 21

  1. Complete this graph by plotting the charge for excess luggage weight of 10 kg. Mark this point with a cross (×).  (1 mark)

    GRAPHS, FUR2 2009 VCAA 22

  2. A graph of the charge against (excess luggage weight)² is to be constructed.

     

    Fill in the missing (excess luggage weight)² value in Table 3 and plot this point with a cross (×) on the graph below.  (1 mark)

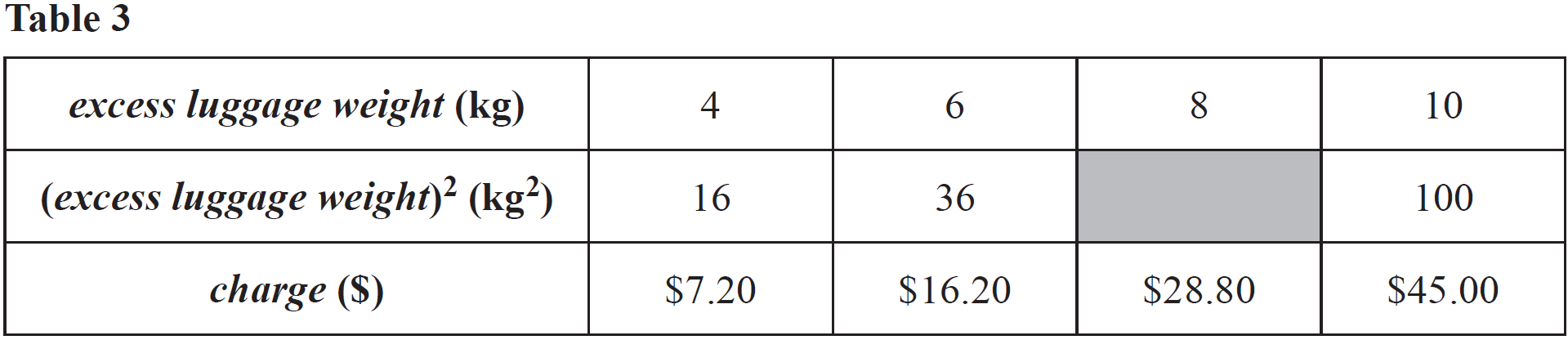
    GRAPHS, FUR2 2009 VCAA 23

GRAPHS, FUR2 2009 VCAA 24

  1. The graph above can be used to find the value of in the equation below.

     

          charge = × (excess luggage weight)²

     

    Find .  (1 mark)

  2. Calculate the charge for transporting 12 kg of excess luggage.

     

    Write your answer in dollars correct to the nearest cent.  (1 mark)

Show Answers Only
  1.  
    GRAPHS, FUR2 2009 VCAA 2 Answer
  2.  
    GRAPHS, FUR2 2009 VCAA 2 Answer1
    GRAPHS, FUR2 2009 VCAA 2 Anwer2
Show Worked Solution
a.   

GRAPHS, FUR2 2009 VCAA 2 Answer

 

b.    GRAPHS, FUR2 2009 VCAA 2 Answer1

GRAPHS, FUR2 2009 VCAA 2 Anwer2

 

c.  

 

d.  

GRAPHS, FUR2 2009 VCAA 1

Fair Go Airlines offers air travel between destinations in regional Victoria. 

Table 1 shows the fares for some distances travelled.

GRAPHS, FUR2 2009 VCAA 11

  1. What is the maximum distance a passenger could travel for $160?  (1 mark)

The fares for the distances travelled in Table 1 are graphed below.

GRAPHS, FUR2 2009 VCAA 13

  1. The fare for a distance longer than 400 km, but not longer than 550 km, is $280.

     

    Draw this information on the graph above.  (1 mark)

Fair Go Airlines is planning to change its fares.

A new fare will include a service fee of $40, plus 50 cents per kilometre travelled.

An equation used to determine this new fare is given by

fare = × distance.

  1. A passenger travels 300 km.

     

    How much will this passenger save on the fare calculated using the equation above compared to the fare shown in Table 1?  (1 mark)

  2. At a certain distance between 250 km and 400 km, the fare, when calculated using either the new equation or Table 1, is the same.

     

    What is this distance?  (2 marks)

  3. An equation connecting the maximum distance that may be travelled for each fare in Table 1 on page 16 can be written as

     

             fare = + × maximum distance.

     

    Determine and .  (2 marks)

Show Answers Only
  2.  
    GRAPHS, FUR2 2009 VCAA 1 Answer
Show Worked Solution

a.  

 

b.   

GRAPHS, FUR2 2009 VCAA 1 Answer

 

c.   
   

 

 

d.   

 

e.  

 

 

 

CORE*, FUR2 2010 VCAA 1

The cash price of a large refrigerator is $2000.

  1. A customer buys the refrigerator under a hire-purchase agreement.
  2. She does not pay a deposit and will pay $55 per month for four years.
    1. Calculate the total amount, in dollars, the customer will pay.   (1 mark)

    2. Find the total interest the customer will pay over four years.   (1 mark)

    3. Determine the annual flat interest rate that is applied to this hire-purchase agreement.
    4. Write your answer as a percentage.   (1 mark)

  3. Next year the cash price of the refrigerator will rise by 2.5%.
  4. The following year it will rise by a further 2.0%.
  5. Calculate the cash price of the refrigerator after these two price rises.   (1 mark)

Show Answers Only

  1. i. 
  2. ii. 
  3. iii.

Show Worked Solution

a.i.  


  

a.ii.   
   

 

a.iii.   
 
 
   

  
b.  

GRAPHS, FUR2 2010 VCAA 3

Let be the number of Softsleep pillows that are sold each week and be the number of Resteasy pillows that are sold each week.

A constraint on the number of pillows that can be sold each week is given by

Inequality 1:  

  1. Explain the meaning of Inequality 1 in terms of the context of this problem.  (1 mark)

Each week, Anne sells at least 30 Softsleep pillows and at least Resteasy pillows.

These constraints may be written as

Inequality 2:  

 

Inequality 3:  

The graphs of    and    are shown below.

GRAPHS, FUR2 2010 VCAA 3

  1. State the value of .  (1 mark)
  2. On the axes above

     

    1. draw the graph of    (1 mark)
    2. shade the region that satisfies Inequalities 1, 2 and 3.  (1 mark)
  3. Softsleep pillows sell for $65 each and Resteasy pillows sell for $50 each.

     

    What is the maximum possible weekly revenue that Anne can obtain?  (2 marks)

Anne decides to sell a third type of pillow, the Snorestop.

She sells two Snorestop pillows for each Softsleep pillow sold. She cannot sell more than 150 pillows in total each week.

  1. Show that a new inequality for the number of pillows sold each week is given by

     

          Inequality 4:  

     

     

    where      is the number of Softsleep pillows that are sold each week

     

        and      is the number of Resteasy pillows that are sold each week.  (1 mark)

Softsleep pillows sell for $65 each.

Resteasy pillows sell for $50 each.

Snorestop pillows sell for $55 each.

  1. Write an equation for the revenue, dollars, from the sale of all three types of pillows, in terms of the variables and .  (1 mark)
  2. Use Inequalities 2, 3 and 4 to calculate the maximum possible weekly revenue from the sale of all three types of pillow.  (2 marks) 
Show Answers Only

  3. i. & ii.
    GRAPHS, FUR2 2010 VCAA 3 Answer
Show Worked Solution

a.   

 

b.  

 

c.i. & ii.   

GRAPHS, FUR2 2010 VCAA 3 Answer

 

d.  

 

e.  

♦♦ Mean mark of parts (e)-(g) (combined) was 24%.

 

f.   
   
   

 

g.   

GRAPHS, FUR2 2010 VCAA 3 Answer1

GRAPHS, FUR2 2010 VCAA 1

Anne sells Softsleep pillows for $65 each.

  1. Write an equation for the revenue, dollars, that Anne receives from the sale of Softsleep pillows.  (1 mark)
  2. The cost, dollars, of making Softsleep pillows is given by

Find the cost of making 30 Softsleep pillows.  (1 mark)

The revenue, , from the sale of Softsleep pillows is graphed below.

 

GRAPHS, FUR2 2010 VCAA 1

  1. Draw the graph of    on the axes above.  (1 mark)
  2. How many Softsleep pillows will Anne need to sell in order to break even?  (1 mark)
Show Answers Only
  3.  
    GRAPHS, FUR2 2010 VCAA 1 Answer
Show Worked Solution

a.  

 

b.  

 

 

c.   

GRAPHS, FUR2 2010 VCAA 1 Answer

 

d.  

 

GEOMETRY, FUR2 2010 VCAA 1

In the plan below, the entry gate of an adventure park is located at point .

A canoeing activity is located at point .

The straight path is 40 metres long.

The bearing of from is 060°.

 

Geometry anad Trig, FUR2 2011 VCAA 1_1
 

  1. Write down the size of the angle that is marked in the plan above.  (1 mark)
  2. What is the bearing of the entry gate from the canoeing activity?  (1 mark)
  3. How many metres north of the entry gate is the canoeing activity?  (1 mark)

 is a 90 metre straight path between the canoeing activity and a water slide located at point .

is a straight path between the entry gate and the water slide.

The angle is 120°.

 

GEOMETRY, FUR2 2010 VCAA 12
 

    1. Find the area that is enclosed by the three paths, , and .

       

      Write your answer in square metres, correct to one decimal place.  (1 mark)

    2. Show that the length of path is 115.3 metres, correct to one decimal place.  (1 mark)

Straight paths and lead to the kiosk located at point .

These two paths are of equal length.

The angle is 10°.

 

GEOMETRY, FUR2 2010 VCAA 13

    1. Find the size of the angle .  (1 mark)
    2. Find the length of path , in metres, correct to one decimal place.  (1 mark)
Show Answers Only
    1. ²
Show Worked Solution
a.   
   

 

b.  

MARKER’S COMMENT: True bearings (3 figure) are preferred to quadrant bearings although S60°W was accepted.

 

c.   

GEOMETRY, FUR2 2010 VCAA 1 Answer1

 

 

d.i.   
   
   
    ²

 

d.ii.  

 
 
 

 

e.i.  

 

 

e.ii.  

 
 

CORE*, FUR2 2011 VCAA 1

Tony plans to take his family on a holiday.

The total cost of $3630 includes a 10% Goods and Services Tax (GST).

  1. Determine the amount of GST that is included in the total cost.  (1 mark)

During the holiday, the family plans to visit some theme parks.

The prices of family tickets for three theme parks are shown in the table below.

BUSINESS, FUR2 2011 VCAA 1

  1. What is the total cost for the family if it visits all three theme parks?  (1 mark)

If Tony purchases the Movie Journey family ticket online, the cost is discounted to $202.40

  1. Determine the percentage discount.  (1 mark)
Show Answers Only
Show Worked Solution

a.  

 
   
 

 

b.  

 

c.   
   
 
 

CORE*, FUR2 2012 VCAA 1

A club purchased new equipment priced at $8360. A 15% deposit was paid.

  1. Calculate the deposit.   (1 mark)

    1. Determine the amount of money that the club still owes on the equipment after the deposit is paid.   (1 mark)
    2. The amount owing will be fully repaid in 12 installments of $650.
    3. Determine the total interest paid.   (1 mark)

Show Answers Only

  2. i. 
  3. ii.

Show Worked Solution

a.   
   

 

b.i.   
   

 

b.ii.   
   

 

NETWORKS, FUR1 2006 VCAA 2 MC

The following directed graph represents a series of one-way streets with intersections numbered as nodes 1 to 8.
 

networks-fur1-2006-vcaa-2-mc-1
 

All intersections can be reached from

A.   intersection 4

B.   intersection 5

C.   intersection 6

D.   intersection 7

E.   intersection 8 

Show Answers Only

Show Worked Solution

NETWORKS, FUR1 2011 VCAA 6 MC

A store manager is directly in charge of five department managers.

Each department manager is directly in charge of six sales people in their department.

This staffing structure could be represented graphically by

A.   a tree.

B.   a circuit.

C.   an Euler path.

D.   a Hamiltonian path.

E.   a complete graph.

Show Answers Only

Show Worked Solution

MATRICES*, FUR1 2013 VCAA 9 MC

Alana, Ben, Ebony, Daniel and Caleb are friends. Each friend has a different age.

The arrows in the graph below show the relative ages of some, but not all, of the friends. For example, the arrow in the graph from Alana to Caleb shows that Alana is older than Caleb.
  

 
Using the information in the graph, it can be deduced that the second-oldest person in this group of friends is

A.   Alana

B.   Ben

C.   Caleb

D.   Daniel

E.   Ebony

Show Answers Only

Show Worked Solution

vcaa-networks-fur1-2013-9i

NETWORKS, FUR1 2013 VCAA 4 MC

Kate, Lexie, Mei and Nasim enter a competition as a team. In this competition, the team must complete four tasks, , as quickly as possible.

The table shows the time, in minutes, that each person would take to complete each of the four tasks.
 

     
 

If each team member is allocated one task only, the minimum time in which this team would complete the four tasks is

A.   

B.   

C.   

D.   

E.  

Show Answers Only

Show Worked Solution

vcaa-networks-fur1-2013-4i