SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Financial Maths, STD2 F4 2020 HSC 11 MC

An asset is depreciated using the declining-balance method with a rate of depreciation of 8% per half year. The asset was bought for $10 000.

What is the salvage value of the asset after 5 years?

  1.  $1749.01
  2.  $4182.12
  3.  $4343.88
  4.  $6590.82
Show Answers Only

Show Worked Solution

♦ Mean mark 43%.
COMMENT: 8% depreciation is applicable every 6 months here (n=10). Read carefully!

 
 
 

 

Measurement, STD2 M1 2020 HSC 5 MC

A plant stem is measured to be 16.0 cm, correct to one decimal place.

What is the percentage error in this measurement?

  1.  0.3125%
  2.  0.625%
  3.  3.125%
  4.  6.25%
Show Answers Only

Show Worked Solution

♦ Mean mark 41%.

 

 

Mechanics, EXT2 M1 EQ-Bank 4

A torpedo with a mass of 80 kilograms has a propeller system that delivers a force of on the torpedo, at maximum power. The water exerts a resistance on the torpedo proportional to the square of the torpedo's velocity .

  1. Explain why 
     
    where is a positive constant.  (1 mark)

  2. If the torpedo increases its velocity from    to  , show that the distance it travels in this time, , is given by
     
           (3 marks)

Show Answers Only
Show Worked Solution

i.   

 

ii.   
 
 
 
   

 


 


 

 
 

Mechanics, EXT2 M1 EQ-Bank 2

A particle with mass moves horizontally against a resistance force , equal to    where is the particle's velocity.

Initially, the particle is travelling in a positive direction from the origin at velocity .

  1. Show that the particle's displacement from the origin, , can be expressed as
     
           (2 marks)

     

  2. Show that the time, , when the particle is travelling at velocity , is given by
     
           (4 marks)

     

  3. Express as a function of , and hence find the limiting values of and .  (2 marks)

Show Answers Only
  2. `text(See Worked Solutions)“
Show Worked Solution

i.   

 

 


 

 
 

 

ii.   


 

     
     

 

 
 
 
 

 


 

 
 

 

iii.
 
 
 
 
 

 

Mechanics, EXT2 M1 EQ-Bank 1

A canon ball of mass 9 kilograms is dropped from the top of a castle at a height of metres above the ground.

The canon ball experiences a resistance force due to air resistance equivalent to  , where is the speed of the canon ball in metres per second. Let    and the displacement, metres at time seconds, be measured in a downward direction.

  1. Show the equation of motion is given by
     
           (1 mark)

  2. Show, by integrating using partial fractions, that
     
           (5 marks)

     

  3. If the canon hits the ground after 4 seconds, calculate the height of the castle, to the nearest metre.  (3 marks)

Show Answers Only
Show Worked Solution

i.   

 

ii.   
   

 

 


 


 


 

 
 
 

 

 

 

iii.   
 
 
 

 
 

 

 

 

 
 

Functions, 2ADV F2 EQ-Bank 13

The curve    is subject to the following transformations

    • Translated 2 units in the positive -direction
    • Dilated in the positive -direction by a factor of 4
    • Reflected in the -axis

The final equation of the curve is  .

  1.  Find the equation of the graph after the dilation.  (1 mark)

  2.  Find the values of    and  .  (2 marks)

Show Answers Only
Show Worked Solution

i.    

 

ii.   
   

 

 

COMMENT: Using “swap” terminology for reflections in the y-axis is simpler and more intelligible for students in our view.

 

 

 

 
 

 

Calculus, 2ADV C4 EQ-Bank 5

The velocity of a particle moving along the -axis at metres per second at seconds, is shown in the graph below.
 


 

Initially, the displacement is equal to 12 metres.

  1. Write an equation that describes the displacement, , at time seconds.  (2 marks)

  2. Draw a graph that shows the displacement of the particle,   metres from the origin, at a time seconds between    and  . Label the coordinates of the endpoints of your graph.  (2 marks)

Show Answers Only
  2.  

Show Worked Solution
i.   
 

 

 
 

 

 

ii. 


 

Statistics, 2ADV S3 EQ-Bank 1

A probability density function can be used to model the lifespan of a termite, , in weeks, is given by
 


 

  1. Show that the value of    is  .  (2 marks)

  2. Find the cumulative distribution function.  (2 marks)

  3. Find the probability that a termite's lifespan is greater than 5 weeks.  (1 mark)

Show Answers Only
Show Worked Solution
i.   
 
 
 
 

 

ii.   
   
   
   
   
   
   

 

 

iii.   
   
   
   

NETWORKS, FUR2-NHT 2019 VCAA 3

The zoo’s management requests quotes for parts of the new building works.

Four businesses each submit quotes for four different tasks.

Each business will be offered only one task.

The quoted cost, in $100 000, of providing the work is shown in Table 1 below.
  


 

The zoo’s management wants to complete the new building works at minimum cost.

The Hungarian algorithm is used to determine the allocation of tasks to businesses.

The first step of the Hungarian algorithm involves row reduction; that is, subtracting the smallest element in each row of Table 1 from each of the elements in that row.

The result of the first step is shown in Table 2 below.
 


 

The second step of the Hungarian algorithm involves column reduction; that is, subtracting the smallest element in each column of Table 2 from each of the elements in that column.

The results of the second step of the Hungarian algorithm are shown in Table 3 below. The values of Task 1 are given as and .
 


 

  1. Write down the values of and .   (1 mark)

  2. The next step of the Hungarian algorithm involves covering all the zero elements with horizontal or vertical lines. The minimum number of lines required to cover the zeros is three.

     

    Draw these three lines on Table 3 above.   (1 mark)

  3. An allocation for minimum cost is not yet possible.

     

    When all steps of the Hungarian algorithm are complete, a bipartite graph can show the allocation for minimum cost.

     

    Complete the bipartite graph below to show this allocation for minimum cost.   (1 mark)

     

  1. Business 4 has changed its quote for the construction of the pathways. The new cost is $1 000 000. The overall minimum cost of the building works is now reduced by reallocating the tasks.

     

    How much is this reduction?   (1 mark)

Show Answers Only
Show Worked Solution

a.   

 

b.  

 

c. 
 


 

 

d. 
 


 


 

 

CORE, FUR1-NHT 2019 VCAA 22-23 MC

Armand borrowed $12 000 to pay for a holiday.

He will be charged interest at the rate of 6.12% per annum, compounding monthly.

This loan will be repaid with monthly repayments of $500.
 

Part 1

After four months, the total interest that Armand will have paid is closest to

  1.   $231
  2.   $245
  3.   $255
  4.   $734
  5. $1796

 
Part 2

After eight repayments, Armand decided to increase the value of his monthly repayments.

He will make a number of monthly repayments of $850 and then one final repayment that will have a smaller value.

This final repayment has a value closest to

  1. $168
  2. $169
  3. $180
  4. $586
  5. $681
Show Answers Only

Show Worked Solution

 

 
 

 

 

 

 

 

 

 

GEOMETRY, FUR1-NHT 2019 VCAA 4 MC

A pizza in the shape of a circle has been cut into 12 equal slices.

The area of the top surfaces of one slice is 9.42 cm2, as shown shaded in the diagram below.
  

     
 

The perimeter of the top surfaces of one slice of pizza, in centimetres, is closest to

  1.   9.14
  2. 15.14
  3. 18.00
  4. 21.99
  5. 40.84
Show Answers Only

Show Worked Solution
 

 

 
 

 


 

MATRICES, FUR2-NHT 2019 VCAA 4

After 5.00 pm, tourists will start to arrive in Gillen and they will stay overnight.

As a result, the number of people in Gillen will increase and the television viewing habits of the tourists will also be monitored.

Assume that 50 tourists arrive every hour.

It is expected that 80% of arriving tourists will watch only during the hour that they arrive.

The remaining 20% of arriving tourists will not watch television during the hour that they arrive.

Let be the state matrix that shows the number of people in each category hours after 5.00 pm on this day.

The recurrence relation that models the change in the television viewing habits of this increasing number of people in Gillen hours after 5.00 pm on this day is shown below.

 

where


 

  1. Write down matrix .   (1 mark)

  2. How many people in Gillen are expected to watch at 7.00 pm on this day?   (2 marks)

Show Answers Only
  1.  
  2.  
Show Worked Solution

a.   

b.   
   
     
 

 

Calculus, MET1-NHT 2018 VCAA 9

Let diagram below shows a trapezium with vertices at    and  , where    is a real number and 

On the same axes as the trapezium, part of the graph of a cubic polynomial function is drawn. It has the rule  , where    is a non-zero real number and  .

  1. At the local maximum of the graph, .
  2. Find in terms of .   (3 marks)

The area between the graph of the function and the -axis is removed from the trapezium, as shown in the diagram.
 

  1. Show that the expression for the area of the shaded region is    square units.   (3 marks)

  2. Find the value of    for which the area of the shaded region is a maximum and find this maximum area.   (2 marks)

Show Answers Only
Show Worked Solution
a.     
 
 

 


 

 

 

 

b.   

 
 
 
 
 
 

 


 

 

 

c.   


 

 
 

Statistics, MET1-NHT 2018 VCAA 8

Let    be the random variable that represents the sample proportions of customers who bring their own shopping bags to a large shopping centre.

From a sample consisting of all customers on a particular day, an approximate 95% confidence interval for the proportion    of customers who bring their own shopping bags to this large shopping centre was determined to be  .

  1. Find the value of    that was used to obtain this approximate 95% confidence interval.   (1 mark)

  2. Use the fact that    to find the size of the sample from which this approximate 95% confidence interval was obtained.   (2 marks)

Show Answers Only

Show Worked Solution

a.   

 
 

 

b.   

Statistics, MET1-NHT 2018 VCAA 6

The discrete random variable has the probability mass function
 


 

  1. Show that  .  (2 marks)

  2. Find  .  (1 mark)

  3. Evaluate  .  (1 mark)

Show Answers Only

Show Worked Solution

a.    
 

 

 

b.   
 
 
 
 

 

c.   
 
 
 

CORE, FUR1-NHT 2019 VCAA 21 MC

Yazhen has a reducing balance loan.

Six lines of the amortisation table for Yazhen’s loan are shown below.

The interest rate for Yazhen’s loan increased after one of these six repayments had been made.

The first repayment made at the higher interest rate was repayment number

  1. 15
  2. 16
  3. 17
  4. 18
  5. 19
Show Answers Only

Show Worked Solution


 


 

Statistics, EXT1 S1 EQ-Bank 14

It is known that 65% of adults over the age of 60 have been tested for bowel cancer.

A random sample of 140 adults aged over 60 years is surveyed.

Using a normal approximation to the binomial distribution and the probability table attached, calculate the probability that at least 85 of the adults chosen have been tested for bowel cancer.   (3 marks)

Show Answers Only

Show Worked Solution

 
 
 

 

 
   

 

 
   

CORE, FUR2-NHT 2019 VCAA 8

A record producer gave the band $50 000 to write and record an album of songs.

This $50 000 was invested in an annuity that provides a monthly payment to the band.

The annuity pays interest at the rate of 3.12% per annum, compounding monthly.

After six months of writing and recording, the band has $32 667.68 remaining in the annuity.

  1. What is the value, in dollars, of the monthly payment to the band?   (1 mark)

  2. After six months of writing and recording, the band decided that it needs more time to finish the album.

     

    To extend the time that the annuity will last, the band will work for three more months without withdrawing a payment.

     

    After this, the band will receive monthly payments of $3800 for as long as possible.

     

    The annuity will end with one final monthly payment that will be smaller than all of the others.

     

    Calculate the total number of months that this annuity will last.   (2 marks)

Show Answers Only
Show Worked Solution

a.   

 
 
 
 
 
 

 

 

b.   

 
 
 
 
 
 

 

 

 
 
 
 
 
 

 

 

CORE, FUR2-NHT 2019 VCAA 7

Tisha plays drums in the same band as Marlon.

She would like to buy a new drum kit and has saved $2500.

  1. Tisha could invest this money in an account that pays interest compounding monthly.

     

    The balance of this investment after months, could be determined using the recurrence relation below
     
           
     
    Calculate the total interest that would be earned by Tisha's investment in the first five months.

     

    Round your answer to the nearest cent.   (2 marks)

Tisha could invest the $2500 in a different account that pays interest at the rate of 4.08% per annum, compounding monthly. She would make a payment of $150 into this account every month.

  1. Let be the value of Tisha's investment after months.

     

    Write down a recurrence relation, in terms of , and , that would model the change in the value of this investment.   (1 mark)

  2. Tisha would like to have a balance of $4500, to the nearest dollar, after 12 months.

     

    What annual interest rate would Tisha require?

     

    Round your answer to two decimal places.   (1 mark)

Show Answers Only
Show Worked Solution

a.   

 

 

b.   

 

c.   

 
 
 
 
 
 

 

CORE, FUR2-NHT 2019 VCAA 5

A random sample of 12 mammals drawn from a population of 62 types of mammals was categorized according to two variables.

likelihood of attack (1 = low, 2 = medium, 3 = high)

exposure to attack during sleep (1 = low, 2 = medium, 3 = high)

The data is shown in the following table.
 


 

  1. Use this data to complete the two-way frequency table below.   (1 mark)

      
         
     

The following two-way frequency table was formed from the data generated when the entire population of 62 types of mammals was similarly categorized.
 
     

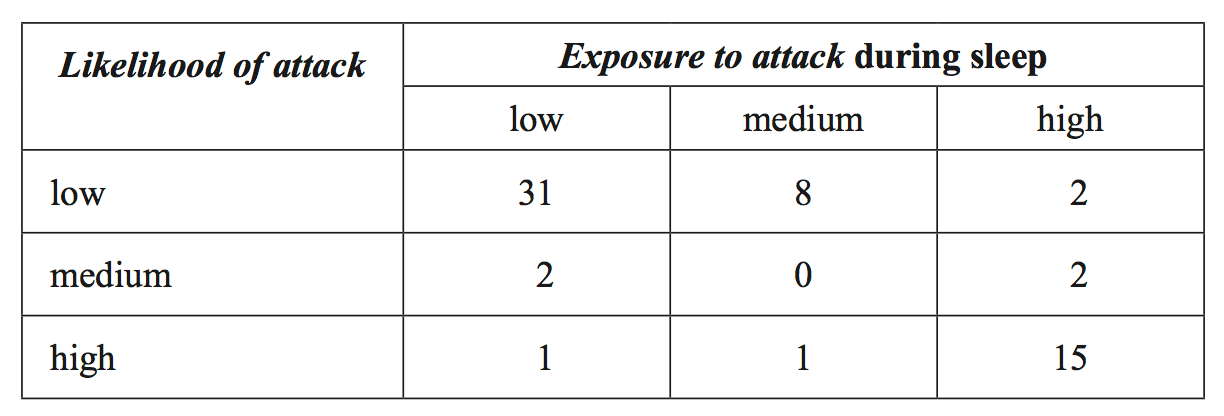
    1. How many of these 62 mammals had both a high likelihood of attack and a high exposure to attack during sleep?   (1 mark)

    2. Of those mammals that had a medium likelihood of attack, what percentage also had a low exposure to attack during sleep?   (1 mark)

    3. Does the information in the table above support the contention that likelihood of attack is associated with exposure to attack during sleep? justify your answer by quoting appropriate percentages. It is sufficient to consider only one category of likelihood of attack when justifying your answer.   (2 marks)

Show Answers Only

  1.  

    2.                      


    3.  

         
       

         
       

         

Show Worked Solution

a.     

 

b.    i.

ii.   
 

 

iii.

CORE, FUR2-NHT 2019 VCAA 4

The scatterplot below plots the variable life span, in years, against the variable sleep time, in hours, for a sample of 19 types of mammals.
 

On the assumption that the association between sleep time and life span is linear, a least squares line is fitted to this data with sleep time as the explanatory variable.

The equation of this least squares line is

life span = 42.1 – 1.90 × sleep time

The coefficient of determination is 0.416

  1. Draw the graph of the least squares line on the scatterplot above.   (1 mark)

  2. Describe the linear association between life span and sleep time in terms of strength and direction.   (2 marks)

  3. Interpret the slope of the least squares line in terms of life span and sleep time.   (2 marks)

  4. Interpret the coefficient of determination in terms of life span and sleep time.   (1 mark)

  5. The life of the mammal with a sleep time of 12 hours is 39.2 years.
  6. Show that, when the least squares line is used to predict the life span of this mammal, the residual is 19.9 years.   (2 marks)

Show Answers Only
  1.  

  2.  

  3.  

     

  4.  

Show Worked Solution

a.   
 


 

b.   


 

c.   

 

d.   


 

e.   
 

 

 
 

MATRICES, FUR1-NHT 2019 VCAA 7-8 MC

A farm contains four water sources, , , and .

Part 1

Cows on the farm are free to move between the four water sources.

The change in the number of cows at each of these water sources from week to week is shown in the transition diagram below.
 

Let be the state matrix for the location of the cows in week of 2019.

The state matrix for the location of the cows in week 23 of 2019 is
 

The state matrix for the location of the cows in week 24 of 2019 is

Of the cows expected to be at in week 24 of 2019, the percentage of these cows at in week 23 of 2019 is closest to

  1.   8%
  2.   9%
  3. 20%
  4. 22%
  5. 25%

 

Part 2

Sheep on the farm are also free to move between the four water sources.

The change in the number of sheep at each water source from week to week is shown in matrix below.
 


 

In the long term, 635 sheep are expected to be at each week.

In the long term, the number of sheep expected to be at each week is closest to

  1. 371
  2. 493
  3. 527
  4. 607
  5. 635
Show Answers Only

Show Worked Solution


 


 

 
 

 

 

 

 

GRAPHS, FUR1-NHT 2019 VCAA 7 MC

The shaded area in the graph below represents the feasible region for a linear programming problem.
 


 

The maximum value of the objective function  occurs at

  1. point only.
  2. any point along line segment  .
  3. any point along line segment  .
  4. any point along line segment  .
  5. any point along line segment  .
Show Answers Only

Show Worked Solution

 

 


 

GRAPHS, FUR1-NHT 2019 VCAA 4 MC

A farm has cows and sheep.

On this farm there are always at least twice as many sheep as cows.

The relationship between the number of cows and the number of sheep on this farm can be represented by the inequality

  1.  
  2.  
  3.  
  4.  
  5.  
Show Answers Only

Show Worked Solution

 

MATRICES, FUR1-NHT 2019 VCAA 5 MC

A population of birds feeds at two different locations, and , on an island.

The change in the percentage of the birds at each location from year to year can be determined from the transition matrix shown below.
 


 

In 2018, 55% of the birds fed at location .

In 2019, the percentage of the birds that are expected to feed at location is

  1. 32%
  2. 42%
  3. 48%
  4. 58%
  5. 62%
Show Answers Only

Show Worked Solution

NETWORKS, FUR1-NHT 2019 VCAA 7 MC

A graph has five vertices, and .

The adjacency matrix for this graph is shown below.
 


 

Which one of the following statements about this graph is not true?

  1. The graph is connected.
  2. The graph contains an Eulerian trail.
  3. The graph contains an Eulerian circuit.
  4. The graph contains a Hamiltonian cycle.
  5. The graph contains a loop and multiple edges.
Show Answers Only

Show Worked Solution

NETWORKS, FUR1-NHT 2019 VCAA 3 MC

Four students, Alice, Brad, Charli and Dexter, are working together on a school project.

This project has four parts.

Each of the students will complete only one part of the project.

The table below shows the time it would take each student to complete each part of the project, in minutes.
 

           Part 1               Part 2               Part 3               Part 4       
    Alice 5 5 3 5
    Brad 3 3 6 4
    Charli 6 5 4 3
    Dexter     4 5 6 5

  
The parts of this project must be completed one after the other.

Which allocation of student to part must occur for this project to be completed in the minimum time possible?

A.           Part 1               Part 2               Part 3               Part 4       
  Brad Dexter Alice Charli
         
B.    Part 1 Part 2 Part 3 Part 4
  Brad Dexter Charli Alice
         
C.    Part 1 Part 2 Part 3 Part 4
  Dexter Alice Charli Brad
         
D.    Part 1 Part 2 Part 3 Part 4
  Dexter Brad Alice Charli
         
E.    Part 1 Part 2 Part 3 Part 4
  Dexter Brad Charli Alice

 

Show Answers Only

Show Worked Solution

 

 

 

Calculus, MET2-NHT 2019 VCAA 5

Let    and  .

  1. Find  .   (1 mark)

  2. Find the coordinates of point  , where the tangent to the graph of  at  is parallel to the graph of  .   (2 marks)

  3. Show that the equation of the line that is perpendicular to the graph of    and goes through point  is  .   (1 mark)

Let be the point of intersection of the graphs of and  , as shown in the diagram below.
 

               
 

  1. Determine the coordinates of point .   (1 mark)

  2. The shaded region below is enclosed by the axes, the graphs of  and , and the line  .
     
     
               
     
    Find the area of the shaded region.   (2 marks)

Let    and  .

  1. The graphs of , and    are shown in the diagram below. The graphs of and touch but do not cross.
     
     
               
     

     Find the value of  .   (2 marks)

  2. Find the value of  , for which the tangent to the graph of at its -intercept and the tangent to the graph of at its -intercept are parallel.   (1 mark)

Show Answers Only
Show Worked Solution

a.   

 

b.   


 


 

 

c.   

 

d.   


 

 

e.
             
 

 
   
   

 

f.   


 

 

g.   


 

 


 

Calculus, MET2-NHT 2019 VCAA 4

A mining company has found deposits of gold between two points, and , that are located on a straight fence line that separates Ms Pot's property and Mr Neg's property. The distance between and is 4 units.

The mining company believes that the gold could be found on both Ms Pot's property and Mr Neg's property.

The mining company initially models he boundary of its proposed mining area using the fence line and the graph of 

where is the number of units from point in the direction of point and is the number of units perpendicular to the fence line, with the positive direction towards Ms Pot's property. The mining company will only mine from the boundary curve to the fence line, as indicated by the shaded area below.
 

  1. Determine the total number of square units that will be mined according to this model.   (2 marks)

The mining company offers to pay Mr Neg $100 000 per square unit of his land mined and Ms Pot $120 000 per square unit of her land mined.

  1. Determine the total amount of money that the mining company offers to pay.   (1 mark)

The mining company reviews its model to use the fence line and the graph of

       where .

  1. Find the value of    for which    for all .   (1 mark)

  2. Solve    for in terms of .   (2 marks)

Mr Neg does not want his property to be mined further than 4 units measured perpendicular from the fence line.

  1. Find the smallest value of , correct to three decimal places, for this condition to be met.   (2 marks)

  2. Find the value of for which the total area of land mined is a minimum.   (3 marks)

  3. The mining company offers to pay Ms Pot $120 000 per square unit of her land mined and Mr Neg $100 000 per square unit of his land mined.
  4. Determine the value of that will minimize the total cost of the land purchase for the mining company. Give your answer correct to three decimal places.   (2 marks)

Show Answers Only
Show Worked Solution
a.   
 
 

 

b.   
 

 
c.   

 
 
 

 
d.   

 
 

e.   


 


 

 

f.   

 

 

 

g.   

Statistics, MET2-NHT 2019 VCAA 3

Concerts at the Mathsland Concert Hall begin minutes after the scheduled starting time. is a random variable that is normally distributed with a mean of 10 minutes and a standard deviation of four minutes.

  1. What proportion of concerts begin before the scheduled starting time, correct to four decimal places?   (1 mark)

  2. Find the probability that a concert begins more than 15 minutes after the scheduled starting time, correct to four decimal places.   (1 mark)

If a concert begins more than 15 minutes after the scheduled starting time, the cleaner is given an extra payment of $200. If a concert begins up to 15 minutes after the scheduled starting time, the cleaner is given an extra payment of $100. If a concert begins at or before the scheduled starting time, there is no extra payment for the cleaner.

Let be the random variable that represents the extra payment for the cleaner, in dollars.

    1. Using your responses from part a. and part b., complete the following table, correct to three decimal places.   (1 mark)

       

    2. Calculate the expected value of the extra payment for the cleaner, to the nearest dollar.   (1 mark)

    3. Calculate the standard deviation of , correct to the nearest dollar.   (1 mark)

The owners of the Mathsland Concert Hall decide to review their operation. They study information from 1000 concerts at other similar venues, collected as a simple random sample. The sample value for the number of concerts that start more than 15 minutes after the scheduled starting time is 43.

    1. Find the 95% confidence interval for the proportion of the concerts that begin more than 15 minutes after the scheduled starting time. Give values correct to three decimal places.   (1 mark)

    2. Explain why this confidence interval suggests that the proportion of concerts that begin more than 15 minutes after the scheduled starting time at the Mathsland Concert Hall is different from the proportion at the venue in the sample.   (1 mark)

The owners of the Mathsland Concert Hall decide that concerts must not begin before the scheduled starting time. They also make changes to reduce the number of concerts that begin after the scheduled starting time. Following these changes, is the random variable that represents the number of minutes after the scheduled starting time that concerts begin. The probability density function for is
 


 

where is the the time, in minutes, after the scheduled starting time.

  1. Calculate the expected value of .   (2 marks) 
    1. Find the probability that a concert now begins more than 15 minutes after the scheduled starting time.   (1 mark)

    2. Find the probability that each of the next nine concerts begins more than 15 minutes after the scheduled starting time and the 10th concert begins more than 15 minutes after the scheduled starting time. Give your answer correct to four decimal places.   (2 marks)

    3. Find the probability that a concert begins up to 20 minutes after the scheduled starting time, given that it begins more than 15 minutes after the scheduled starting time. Give your answer correct to three decimal places.   (2 marks)

Show Answers Only

  3. i.   

     ii.   
     iii. 
  4. i.   
    ii.    

     

  5.  
  6. i.   
    ii.   
    iii.  

Show Worked Solution

a.   

 

 

b.   
 

 

c.i.

ii. 
 
 

 

iii. 
 

 

 
 
 
 

 

 
d.i. 

 

 
ii.   

 

e.   
 

 

f.i.   
 

 
ii. 
 

 
iii. 

 
 

 
 
 

Trigonometry, EXT1 T3 EQ-Bank 5

A particular energy wave can be modelled by the function

  1. Express this function in the form  .  (2 marks)

  2. Find the time the wave first attains its maximum value. Give your answer to one decimal place.  (2 marks)

Show Answers Only
Show Worked Solution

i.   

 

 

 


 

 

 

 

ii.   

 

 

Trigonometry, MET2-NHT 2019 VCAA 2

The wind speed at a weather monitoring station varies according to the function

where is the speed of the wind, in kilometres per hour (km/h), and    is the time, in minutes, after 9 am.

  1. What is the amplitude and the period of  ?   (2 marks)

  2. What are the maximum and minimum wind speeds at the weather monitoring station?   (1 mark)

  3. Find  , correct to four decimal places.   (1 mark)

  4. Find the average value of    for the first 60 minutes, correct to two decimal places.   (2 marks)

A sudden wind change occurs at 10 am. From that point in time, the wind speed varies according to the new function

                   

where    is the speed of the wind, in kilometres per hour, is the time, in minutes, after 9 am and  . The wind speed after 9 am is shown in the diagram below.
 

  1. Find the smallest value of , correct to four decimal places, such that    and    are equal and are both increasing at 10 am.   (2 marks)

  2. Another possible value of was found to be 31.4358

     

    Using this value of , the weather monitoring station sends a signal when the wind speed is greater than 38 km/h.

     

    i.  Find the value of at which a signal is first sent, correct to two decimal places.   (1 mark)

     

    ii. Find the proportion of one cycle, to the nearest whole percent, for which  .   (2 marks)

  3. Let    and  .
     
    The transformation    maps the graph of    onto the graph of  .

     

    State the values of  , , and , in terms of where appropriate.   (3 marks)

Show Answers Only

  2.  

  6. i.

     

    ii.

Show Worked Solution

a.   

 

b.   

 

c.   
 

 

d.   

 
 

 

e.   

 

f.i. 


 

f.ii. 

 
 

 

g.   

 


 


 

 

Graphs, MET2-NHT 2019 VCAA 1

Parts of the graphs of    and    are shown on the axes below.
 


 

The two graphs intersect at three points,  (–2, 0),  (1, 0)  and  (, ). The point  (, )  is not shown in the diagram above.

  1. Find the values of and .   (2 marks)

  2. Find the values of such that  .   (1 mark)

  3. State the values of for which
    1.    (1 mark)

    2.    (1 mark)

  4. Show that    for all  .   (1 mark)

  5. Find the values of such that    has exactly one negative solution.   (2 marks)

  6. Find the values of such that    has no solutions.   (1 mark)

Show Answers Only
  3. i. 
    ii.
  5.  
Show Worked Solution

a.   


 

 

 

b.   

 

c.i. 

c.ii. 

 

d.     
 

 

 
 
 

 

e.     
 

 

 

f.   

CORE, FUR1-NHT 2019 VCAA 12 MC

Which one of the following statements could be true when written as part of the results of a statistical investigation?

  1. The correlation coefficient between height (in centimetres) and foot length (in centimetres) was found to be 
  2. The correlation coefficient between height (below average, average, above average) and arm span (in centimetres) was found to be 
  3. The correlation coefficient between blood pressure (low, normal, high) and age (under 25, 25–49, over 50) was found to be 
  4. The correlation coefficient between the height of students of the same age (in centimetres) and the money they spent on snack food (in dollars) was found to be 
  5. The correlation coefficient between height of wheat (in centimetres) and grain yield (in tonnes) was found to be    and the coefficient of determination was found to be 
Show Answers Only

Show Worked Solution

CORE, FUR1-NHT 2019 VCAA 11 MC

The Human Development Index (HDI) and the mean number of children per woman for 13 countries are related.

This relationship is non-linear.

To linearise the data, a    transformation is applied to the response variable children.

A least squares line is then fitted to the linearised data.

The equation of this least squares line is

Using this equation, the mean number of children per woman for a country with a HDI of 95 is predicted to be closest to

  1.  0.4
  2.  1.5
  3.  2.6
  4.  2.9
  5.  3.1
Show Answers Only

Show Worked Solution
 
 

CORE, FUR1-NHT 2019 VCAA 5-7 MC

The birth weights of a large population of babies are approximately normally distributed with a mean of 3300 g and a standard deviation of 550 g.

Part 1

A baby selected at random from this population has a standardised weight of 

Which one of the following calculations will result in the actual birth weight of this baby?
 

 

Part 2

Using the 68–95–99.7% rule, the percentage of babies with a birth weight of less than 1650 g is closest to

  1. 0.14%
  2. 0.15%
  3. 0.17%
  4. 0.3%
  5. 2.5%

 

Part 3

A sample of 600 babies was drawn at random from this population.

Using the 68–95–99.7% rule, the number of these babies with a birth weight between 2200 g and 3850 g is closest to

  1. 111
  2. 113
  3. 185
  4. 408
  5. 489
Show Answers Only

Show Worked Solution

 

 

 
 

 

 
 


 


 


 

 
 

Probability, MET2-NHT 2019 VCAA 19 MC

A random sample of computer users was surveyed about whether the users had played a particular computer game. An approximate 95% confidence interval for the proportion of computer users who had played this game was calculated from this random sample to be (0.6668, 0.8147).

The number of computer users in the sample is closest to

  1.      5
  2.    33
  3.  135
  4.  150
  5.  180
Show Answers Only

Show Worked Solution


 

Functions, MET2-NHT 2019 VCAA 17 MC

The graph of the function is obtained from the graph of the function with rule    by a dilation of factor    from the -axis, a dilation of factor    from the -axis, a reflection in the  -axis and a translation of    units in the positive direction, in that order.

The range and period of are respectively

Show Answers Only

Show Worked Solution


 


 


 

 

Combinatorics, EXT1 A1 2019 MET1 8

A fair standard die is rolled 50 times. Let be a random variable with binomial distribution that represents the number of times the face with a six on it appears uppermost.

  1. Write down the expression for  , where  .  (1 mark)

  2. Show that  .  (2 marks)

Show Answers Only
Show Worked Solution

a. 

 

b.  
   
   
   

Statistics, EXT1 S1 2019 MET1 6

Jacinta tosses a coin five times.

  1. Assuming that the coin is fair and given that Jacinta observes a head on the first two tosses, find the probability that she observes a total of either four or five heads.  (2 marks)

  2. Albin suspects that a coin is not actually a fair coin and he tosses it 18 times.

     

    Albin observes a total of 12 heads from the 18 tosses.

  3. Let  = probability of obtaining a head.
  4. Find the range of in which 95% of observations are expected to lie within.  (2 marks)

Show Answers Only
  1.  
  2.  
Show Worked Solution

a.   

 
 

 

b.   

Calculus, 2ADV C3 2019 MET1 4

Given the function  ,

  1. State the domain and range of .  (1 mark)

  2. i.  Find the equation of the tangent to the graph of  at  .  (2 marks)

     

    ii. On the axes below, sketch the graph of the function  , labelling any asymptote with its equation.

     

        Also draw the tangent to the graph of    at  .  (4 marks)
     

Show Answers Only

  1.  

  2. i. 

     

    ii.

Show Worked Solution
a.  
 

 

b.i.  
 
 

 

 

b.ii.