SmarterEd

Aussie Maths & Science Teachers: Save your time with SmarterEd

Trigonometry, 2ADV* T1 2012 HSC 20 MC

Town `B` is 80 km due north of Town `A` and 59 km from Town `C`.

Town `A` is 31 km from Town `C`.
 

2012 20 mc
 

 What is the bearing of Town `C` from Town `B`?  

  1. `019^@`
  2. `122^@` 
  3. `161^@` 
  4. `341^@` 
Show Answers Only

`C`

Show Worked Solution

`text(Using the cosine rule:)`

`cos\ /_B` `= (a^2 + c^2 -b^2)/(2ac)`
  `= (59^2 + 80^2 -31^2)/(2 xx 59 xx 80)`
  `= 0.9449…`
`/_B` `= 19^@\  text((nearest degree))`

 

`:.\ text(Bearing of Town C from B) = 180-19= 161^@`

`=>  C`

Trigonometry, 2ADV T1 2018 HSC 12a

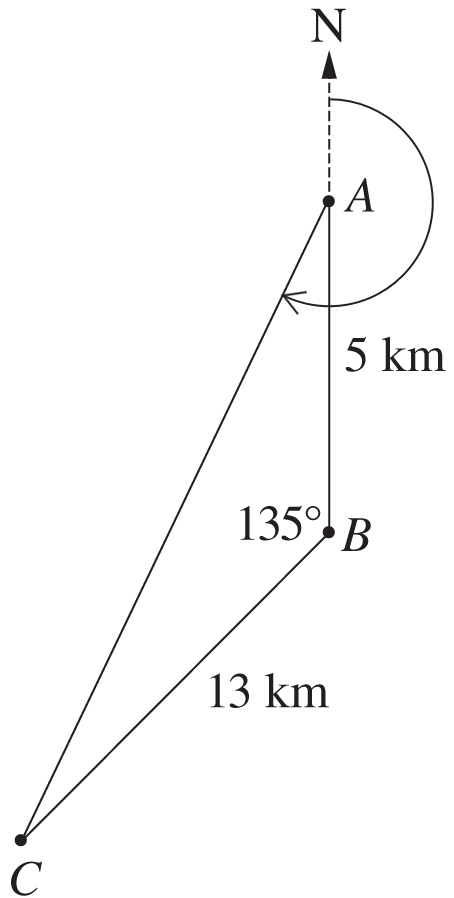
A ship travels from Port A on a bearing of 050° for 320 km to Port B. It then travels on a bearing of 120° for 190 km to Port C.
 


 

  1. What is the size of  `/_ ABC`?  (1 mark)

  2. What is the distance from Port A to Port C ? Answer to the nearest 10 kilometres.  (2 marks)

Show Answers Only
  1. `110^@`
  2. `420\ text(km)`
Show Worked Solution
i.  

`text(Let)\ \ D\ \ text(be south of)\ \ B`

`/_ ABD = 50^@ qquad text{(alternate angles)}`

`/_DBC = 60^@ qquad text{(180° in straight line)}`

`:. /_ ABC` `= 50 + 60`
  `= 110^@`

 

ii.  `text(Using the cosine rule:)`

`AC^2` `= AB^2 + BC^2 – 2 *AB*BC* cos /_ ABC`
  `= 320^2 + 190^2 – 2 xx 320 xx 190 xx cos 110^@`
  `= 180\ 089.64…`
`:. AC` `= 424.36…`
  `= 420\ text{km (nearest 10 km)}`

Algebra, STD2 A1 2018 HSC 28b

Solve the equation  `(2x)/5 + 1 = (3x + 1)/2`, leaving your answer as a fraction.  (3 marks)

Show Answers Only

`5/11`

Show Worked Solution

♦ Mean mark 35%.

`underbrace{(2x)/5 + 1}_text(multiply x10)` `=underbrace{(3x + 1)/2}_text(multiply x10)`
`4x + 10` `= 15x + 5`
`11x` `= 5`
`x` `= 5/11`

Statistics, STD2 S5 2018 HSC 27e

Joanna sits a Physics test and a Biology test.

  1. Joanna’s mark in the Physics test is 70. The mean mark for this test is 58 and the standard deviation is 8.

     

    Calculate the `z`-score for Joanna’s mark in this test.  (1 mark)

  2. In the Biology test, the mean mark is 64 and the standard deviation is 10.

     

    Joanna’s `z`-score is the same in both the Physics test and the Biology test.

     

    What is her mark in the Biology test?  (2 marks)

Show Answers Only
  1. `1.5`
  2. `79`
Show Worked Solution

i.   `x = 70, \ mu = 58, \ sigma = 8`

`:. ztext(-score)` `= (x – mu)/sigma`
  `= (70 – 58)/8`
  `= 1.5`

 

ii.    `1.5` `= (x – 64)/10`
  `x – 64` `= 15`
  `:. x` `= 79`

Statistics, STD2 S1 2018 HSC 26e

A cumulative frequency table for a data set is shown.

\begin{array} {|c|c|}
\hline
\ \ \ \ \ \ \ \textit{Score}\ \ \ \ \ \ \   & \ \ \ \ \ \textit{Cumulative}\ \ \ \ \  \\ & \textit{frequency} \\
\hline
\rule{0pt}{2.5ex} \text{1} \rule[-1ex]{0pt}{0pt} & 5 \\
\hline
\rule{0pt}{2.5ex} \text{2} \rule[-1ex]{0pt}{0pt} & 9 \\
\hline
\rule{0pt}{2.5ex} \text{3} \rule[-1ex]{0pt}{0pt} & 16 \\
\hline
\rule{0pt}{2.5ex} \text{4} \rule[-1ex]{0pt}{0pt} & 20 \\
\hline
\rule{0pt}{2.5ex} \text{5} \rule[-1ex]{0pt}{0pt} & 34 \\
\hline
\rule{0pt}{2.5ex} \text{6} \rule[-1ex]{0pt}{0pt} & 42 \\
\hline
\end{array}

What is the interquartile range of this data set?   (2 marks)

Show Answers Only

`2`

Show Worked Solution

`text(42 data points ⇒ median) = text(21st + 22nd)/2`

♦♦ Mean mark 27%.

`text(Q)_1` `= 11text(th data point) = 3`
`text(Q)_3` `= 32text(nd data point) = 5`

 

`:.\ text(IQR)` `= 5 – 3`
  `= 2`

Statistics, STD2 S1 2018 HSC 26d

The graph displays the mean monthly rainfall in Sydney and Perth.
 


 

  1. For how many months is the mean monthly rainfall higher in Perth than in Sydney?  (1 mark)

  2. For which of the two cities is the standard deviation of the mean monthly rainfall smaller? Justify your answer WITHOUT calculations.  (1 mark)

Show Answers Only
  1. `3`
  2. `text(The mean monthly rainfall of Sydney is in a)`
    `text(much tighter range than Perth.)`
    `:.\ text(Sydney has a smaller standard deviation.)`
Show Worked Solution

i.   `text(3 months (Jul, Aug and Sep))`

♦ Mean mark part (ii) 38%.
COMMENT: Extremely volatile result between parts with part (i) producing a 91% mean mark.

 

ii.    `text(The mean monthly rainfall of Sydney is in a)`

`text(much tighter range than Perth.)`

`:.\ text(Sydney has a smaller standard deviation.)`

Probability, STD2 S2 2018 HSC 26a

Jeremy rolled a biased 6-sided die a number of times. He recorded the results in a table.
  

\begin{array} {|l|c|c|c|c|c|c|}
\hline
\rule{0pt}{2.5ex} \text{Number} \rule[-1ex]{0pt}{0pt} & \ \ 1 \ \ & \ \ 2 \ \  & \ \ 3 \ \  & \ \ 4 \ \  & \ \ 5 \ \  & \ \ 6 \ \ \\
\hline
\rule{0pt}{2.5ex} \text{Frequency} \rule[-1ex]{0pt}{0pt} & \ \ 23 \ \ & \ \ 19 \ \  & \ \ 48 \ \  & \ \ 20 \ \  & \ \ 21 \ \  & \ \ 19 \ \ \\
\hline
\end{array} 

What is the relative frequency of rolling a 3?  (1 mark)

Show Answers Only

\(\dfrac{8}{25}\)

Show Worked Solution
♦ Mean mark 40%.

\(\text{Rel Freq}\) \(=\dfrac{\text{number of 3’s rolled}}{\text{total rolls}}\)
  \(=\dfrac{48}{150}\)
  \(=\dfrac{8}{25}\)

Functions, 2ADV F1 2018 HSC 3 MC

What is the `x`-intercept of the line  `x + 3y + 6 = 0`?

  1. `(-6, 0)`
  2. `(6, 0)`
  3. `(0, -2)`
  4. `(0, 2)`
Show Answers Only

`A`

Show Worked Solution

`x text(-intercept occurs when)\ y = 0:`

`x + 0 + 6` `= 0`
`x` `= -6`

 
`:. x text{-intercept is}\  (-6, 0)`

`=>  A`

Statistics, STD2 S5 2018 HSC 23 MC

A set of data is normally distributed with a mean of 48 and a standard deviation of 3.

Approximately what percentage of the scores lies between 39 and 45?

  1. 15.85%
  2. 31.7%
  3. 47.5%
  4. 49.85%
Show Answers Only

`A`

Show Worked Solution

`text(Given)\ \ mu = 48, \ sigma = 3`

♦ Mean mark 47%.

`ztext{-score (39)}` `= (x – mu)/sigma`
  `= (39 – 48)/3`
  `= −3`

 

`ztext{-score (45)}` `= (45 – 48)/3`
  `= −1`

 

 
`:.\ text(Scores between 39 and 45)`

`~~ 16text(%)`

`=>A`

`text(Note that % of scores below 39 is very small.)`

Probability, STD2 S2 2018 HSC 20 MC

During a year, the maximum temperature each day was recorded. The results are shown in the table.
  


  

From the days with a maximum temperature less than 25°C, one day is selected at random.

What is the probability, to the nearest percentage, that the selected day occurred during winter?

  1. 19%
  2. 25%
  3. 32%
  4. 77%
Show Answers Only

`text(C)`

Show Worked Solution
`text{P(winter day)}` `= (text(winter days < 25))/text(total days < 25) xx 100`
  `= 71/223 xx 100`
  `= 31.8…%`

`=>\ text(C)`

Financial Maths, STD2 F4 2018 HSC 19 MC

The table shows the compounded values of $1 at different interest rates over different periods.
 

 
Amy hopes to have $21 000 in 2 years to buy a car. She opens an account today which pays interest of 4% pa, compounded quarterly.

Using the table, which expression calculates the minimum single sum that Amy needs to invest today to ensure she reaches her savings goal?

  1. 21 000 × 1.0816
  2. 21 000 ÷ 1.0816
  3. 21 000 × 1.0829
  4. 21 000 ÷ 1.0829
Show Answers Only

`text(D)`

Show Worked Solution

`text(4% annual)`

♦♦ Mean mark 33%.

`=> (4%)/4 = 1text(% compounded quarterly)`

`=> n = 8`

`=>\  text(Factor) = 1.0829`

`:.\ text(Minimum sum) = 21\ 000 ÷ 1.0829`

`=>\ text(D)`

Statistics, STD2 S1 2018 HSC 11 MC

A set of data is summarised in this frequency distribution table.
 

 
Which of the following is true about the data?

  1. Mode = 7, median = 5.5
  2. Mode = 7, median = 6
  3. Mode = 9, median = 5.5
  4. Mode = 9, median = 6
Show Answers Only

`text(B)`

Show Worked Solution

`text{Mode = 7  (highest frequency of 9)}`

`text(Median = average of 15th and 16th data points.)`

`:.\ text(Median = 6)`

`=>\ text(B)`

Measurement, STD2 M6 2018 HSC 7 MC

The diagram shows the positions of towns `A`, `B` and `C`.

Town `A` is due north of town `B` and `angleCAB = 34°`
  


 

What is the bearing of town `C` from town `A`?

  1. 034°
  2. 146°
  3. 214°
  4. 326°
Show Answers Only

`C`

Show Worked Solution

`text(Bearing of Town)\ C\ text(from Town)\ A:`
 

`text(Bearing)` `= 180 + 34`
  `= 214^@`

 
`=>C`

Statistics, STD2 S1 2018 HSC 6 MC

A set of data is displayed in this dot plot.
 


 

Which of the following best describes this set of data?

  1. Symmetrical
  2. Positively skewed
  3. Negatively skewed
  4. Normally distributed
Show Answers Only

`text(C)`

Show Worked Solution

`text(Data is skewed.)`

♦ Mean mark 43% (a surprisingly poor result!)

`text(S)text(ince the “tail” is on the left had side, the)`

`text(data is negatively skewed.)`

`=>\ text(C)`

Statistics, STD2 S1 2018 HSC 3 MC

A survey asked the following question.

'How many brothers do you have?'

How would the responses be classified?

  1. Categorical, ordinal
  2. Categorical, nominal
  3. Numerical, discrete
  4. Numerical, continuous
Show Answers Only

`text(C)`

Show Worked Solution

`text(The number of brothers a person has is)`

`text(an exact whole number.)`

`:.\ text(Classification is numerical, discrete.)`

`=>\ text(C)`

Statistics, STD2 S1 2018 HSC 1 MC

A set of scores has the following five-number summary.

lower extreme = 2
lower quartile = 5
median = 6
upper quartile = 8
upper extreme = 9

What is the range?

  1. 2
  2. 3
  3. 6
  4. 7
Show Answers Only

`text(D)`

Show Worked Solution
`text(Range)` `=\ text(upper extreme − lower extreme)`
  `= 9 – 2`
  `= 7`

`=>\ text(D)`

Functions, 2ADV F1 2017 HSC 1 MC

What is the gradient of the line  `2x + 3y + 4 = 0`?

  1. `-2/3`
  2. `2/3`
  3. `-3/2`
  4. `3/2`
Show Answers Only

`A`

Show Worked Solution
`2x + 3y + 4` `= 0`
`3y` `= -2x-4`
`y` `= -2/3 x-4/3`
`:.\ text(Gradient)` `= -2/3`

 
`=>  A`

Measurement, STD2 M6 2017 HSC 30c

The diagram shows the location of three schools. School `A` is 5 km due north of school `B`, school `C` is 13 km from school `B` and `angleABC` is 135°.
 


 

  1. Calculate the shortest distance from school `A` to school `C`, to the nearest kilometre.  (2 marks)

  2. Determine the bearing of school `C` from school `A`, to the nearest degree.  (3 marks)

Show Answers Only
  1. `17\ text{km  (nearest km)}`
  2. `213^@`
Show Worked Solution

i.   `text(Using cosine rule:)`

`AC^2` `= AB^2 + BC^2 – 2 xx AB xx BC xx cos135^@`
  `= 5^2 + 13^2 – 2 xx 5 xx 13 xx cos135^@`
  `= 285.923…`
`:. AC` `= 16.909…`
  `= 17\ text{km  (nearest km)}`

 

ii.   

`text(Using sine rule, find)\ angleBAC:`

♦♦ Mean mark 31%.
`(sin angleBAC)/13` `= (sin 135^@)/17`
`sin angleBAC` `= (13 xx sin 135^@)/17`
  `= 0.5407…`
`angleBAC` `= 32.7^@`

 

`:. text(Bearing of)\ C\ text(from)\ A`

`= 180 + 32.7`

`= 212.7^@`

`= 213^@`

Statistics, STD2 S1 2017 HSC 30a

A set of data has a lower quartile (`Q_L`) of 10 and an upper quartile (`Q_U`) of 16.

What is the maximum possible range for this set of data if there are no outliers?  (2 marks)

Show Answers Only

`24`

Show Worked Solution

`IQR = 16 – 10 = 6`

♦♦ Mean mark 34%.

`text(If no outliers,)`

`text(Upper limit)` `= Q_U + 1.5 xx IQR`
  `= 16 + 1.5 xx 6`
  `= 25`
`text(Lower limit)` `= Q_L – 1.5 xx IQR`
  `= 10 – 1.5 xx 6`
  `= 1`

 

`:.\ text(Maximum range)` `= 25 – 1`
  `= 24`

Statistics, STD2 S5 2017 HSC 29d

All the students in a class of 30 did a test.

The marks, out of 10, are shown in the dot plot.
 


 

  1. Find the median test mark.  (1 mark)

  2. The mean test mark is 5.4. The standard deviation of the test marks is 4.22.

     

    Using the dot plot, calculate the percentage of the marks which lie within one standard deviation of the mean.  (2 marks)

  3. A student states that for any data set, 68% of the scores should lie within one standard deviation of the mean. With reference to the dot plot, explain why the student’s statement is NOT relevant in this context.  (1 mark)

Show Answers Only
  1. `6`
  2. `text(43%)`
  3. `text(The statement assumes the data is normally)`
    `text(distributed which is not the case here.)`
Show Worked Solution
♦ Mean mark 50%.
i.    `text(Median)` `= text(15th + 16th score)/2`
    `= (4 + 8)/2`
    `= 6`

 

ii.   `text(Lower limit) = 5.4 – 4.22 = 1.18`

♦♦ Mean mark 34%.

`text(Upper limit) = 5.4 + 4.22 = 9.62`

`:.\ text(Percentage in between)`

`= 13/30 xx 100`

`= 43.33…`

`= 43text{%  (nearest %)}`

 

iii.   `text(The statement assumes the data is normally)`

♦♦♦ Mean mark 13%.

`text(distributed which is not the case here.)`

Probability, STD2 S2 2017 HSC 29c

A group of Year 12 students was surveyed. The students were asked whether they live in the city or the country. They were also asked if they have ever waterskied.

The results are recorded in the table.
  


  

  1. A person is selected at random from the group surveyed. Calculate the probability that the person lives in the city and has never waterskied.  (2 marks)

  2. A newspaper article claimed that Year 12 students who live in the country are more likely to have waterskied than those who live in the city.

     

    Is this true, based on the survey results? Justify your answer with relevant calculations.  (2 marks)

Show Answers Only
  1. `125/176`
  2. `text(See Worked Solutions)`
Show Worked Solution
i.     `P` `= text(live in city, not skied)/text(total surveyed)`
    `= 2500/3520`
    `= 125/176`
♦ Mean mark part (ii) 47%.

 

ii.   `P(text(live in country, skied))` `= 70/((70 + 800))`
    `= 0.0804…`
    `= 8text(%)`

 

`P(text(live in city, skied))` `= 150/((150 + 2500))`
  `= 0.0566`
  `= 6text(%)`

 
`text(S)text(ince 8% > 6%, the statement is true.)`

Financial Maths, STD2 F5 2017 HSC 27c

A table of future value interest factors for an annuity of $1 is shown.
 


 

An annuity involves contributions of $12 000 per annum for 5 years. The interest rate is 4% per annum, compounded annually.

  1. Calculate the future value of this annuity.  (1 mark)

  2. Calculate the interest earned on this annuity.  (1 mark)

Show Answers Only
  1. `$64\ 995.60`
  2. `$4995.60`
Show Worked Solution

i.   `text(FV factor = 5.4163)`

`:.\ text(FV of Annuity)` `= 12\ 000 xx 5.4163`
  `= $64\ 995.60`
♦♦ Mean mark part (ii) 22%.
COMMENT: A very poorly answered question dealing with a core concept in this area.

 

ii.   `text(Interest earned)` `=\ text(FV − total repayments)`
    `= 64\ 995.60 – (5 xx 12\ 000)`
    `= $4995.60`

Statistics, STD2 S1 2017 HSC 27a

Jamal surveyed eight households in his street. He asked them how many kilolitres (kL) of water they used in the last year. Here are the results.

`220, 105, 101, 450, 37, 338, 151, 205`

  1. Calculate the mean of this set of data.  (1 mark)

  2. What is the standard deviation of this set of data, correct to one decimal place?  (1 mark)

Show Answers Only
  1. `200.875`
  2. `127.4\ \ text{(1 d.p.)}`
Show Worked Solution
i.   `text(Mean)` `= (220 + 105 + 101 + 450 + 37 + 338 + 151 + 205) ÷ 8`
    `= 200.875`
♦ Mean mark part (ii) 47%.
IMPORTANT: The population standard deviation is required here.

 

ii.   `text(Std Dev)` `= 127.357…\ \ text{(by calc)}`
    `= 127.4\ \ text{(1 d.p.)}`

Statistics, STD2 S5 2017 HSC 13 MC

The heights of Year 12 girls are normally distributed with a mean of 165 cm and a standard deviation of 5.5 cm.

What is the `z`-score for a height of 154 cm?

A.     `−2`

B.    `−0.5`

C.     `0.5`

D.     `2`

Show Answers Only

`text(A)`

Show Worked Solution
`ztext(-score)` `= (x – mu)/sigma`
  `= (154 – 165)/5.5`
  `= −2`

 
`=>A`

Statistics, STD2 S4 2017 HSC 12 MC

Which of the data sets graphed below has the largest positive correlation coefficient value?
 

A.      B.     
C.      D.     
Show Answers Only

\(C\)

Show Worked Solution

\(\text{Largest positive correlation occurs when both variables move}\)

\(\text{in tandem. The tighter the linear relationship, the higher the}\)

\(\text{correlation.}\)

\(\Rightarrow C\)

\(\text{(Note that B is negatively correlated)}\)

Financial Maths, STD2 F4 2017 HSC 10 MC

A single amount of $10 000 is invested for 4 years, earning interest at the rate of 3% per annum, compounded monthly.

Which expression will give the future value of the investment?

  1. `10\ 000 xx (1 + 0.03)^4`
  2. `10\ 000 xx (1 + 0.03)^48`
  3. `10\ 000 xx (1 + 0.03/12)^4`
  4. `10\ 000 xx (1 + 0.03/12)^48`
Show Answers Only

`D`

Show Worked Solution

`text(Compounding rate)\ = 3/100 ÷ 12= 0.03/12`

`text(Compounding periods)` `= 4 xx 12=48`

 
`:.\ text(FV) = 10\ 000 xx (1 + 0.03/12)^48`

\(\Rightarrow D\)

Algebra, STD2 A1 2017 HSC 9 MC

What is the value of  `x`  in the equation  `(5-x)/3 = 6`?

  1. `-13`
  2. `-3`
  3. `3`
  4. `13`
Show Answers Only

`A`

Show Worked Solution
`(5-x)/3` `= 6`
`5-x` `= 18`
`x` `= 5-18`
  `= -13`

`=>A`

Statistics, STD2 S1 2017 HSC 4 MC

A factory’s quality control department has tested every 50th item produced for possible defects.

What type of sampling has been used?

A.     Random

B.     Stratified

C.     Systematic

D.     Numerical

Show Answers Only

`C`

Show Worked Solution

`text(A systematic sample divides a population)`

`text(into equal sample sizes and then selects)`

`text(equally among them.)`

`=> C`

Probability, STD2 S2 2017 HSC 5 MC

In a survey of 200 randomly selected Year 12 students it was found that 180 use social media.

Based on this survey, approximately how many of 75 000 Year 12 students would be expected to use social media?

A.     60 000

B.     67 500

C.     74 980

D.     75 000

Show Answers Only

`B`

Show Worked Solution
`text(Expected number)` `= 180/200 xx 75\ 000`
  `= 67\ 500`

`=> B`

Algebra, STD2 A2 2017 HSC 3 MC

The graph shows the relationship between infant mortality rate (deaths per 1000 live births) and life expectancy at birth (in years) for different countries.
 

What is the life expectancy at birth in a country which has an infant mortality rate of 60?

  1. 68 years
  2. 69 years
  3. 86 years
  4. 88 years
Show Answers Only

\(A\)

Show Worked Solution

\(\text{When infant mortality rate is 60, life expectancy}\)

\(\text{at birth is 68 years (see below).}\)
 

\(\Rightarrow A\)

Statistics, STD2 S1 2017 HSC 1 MC

The box-and-whisker plot for a set of data is shown.
 

What is the median of this set of data?

  1. 15
  2. 20
  3. 30
  4. 35
Show Answers Only

`C`

Show Worked Solution

`text(Median = 30)`

`=> C`

Algebra, STD2 A2 2016 HSC 29e

The graph shows the life expectancy of people born between 1900 and 2000.
 


  1. According to the graph, what is the life expectancy of a person born in 1932?  (1 mark)

  2. With reference to the value of the gradient, explain the meaning of the gradient in this context.  (2 marks)

Show Answers Only
  1. `text(68 years)`
  2. `text(After 1900, life expectancy increases 0.25 years for each later year someone is born.)`
Show Worked Solution

i.    \(\text{68 years}\)

ii.    \(\text{Using (1900,60), (1980,80):}\)

\(\text{Gradient}\) \(= \dfrac{y_2-y_1}{x_2-x_1}\)
  \(= \dfrac{80-60}{1980-1900}\)
  \(= 0.25\)

 
\(\text{After 1900, life expectancy increases by 0.25 years for}\)

\(\text{each year later that someone is born.}\)

♦♦ Mean mark (ii) 33%.

Statistics, STD2 S1 2016 HSC 29c

The ages of members of a dance class are shown in the back-to-back stem-and-leaf plot.
 

2ug-2016-hsc-q29_2
 

Pat claims that the women who attend the dance class are generally older than the men.

Is Pat correct? Justify your answer by referring to the median and skewness of the two sets of data.  (3 marks)

Show Answers Only

`text(Women:)`

`text(The median is 55 in a data)`

`text(set that is negatively skewed.)`

`text(Men:)`

`text(The median is 45 in a data)`

`text(set that is positively skewed.)`

`:.\ text(Pat is correct.)`

Show Worked Solution

`text(Women:)`

♦ Mean mark 44%.

`text(The median is 55 in a data)`

`text(set that is negatively skewed.)`

`text(Men:)`

`text(The median is 45 in a data)`

`text(set that is positively skewed.)`

`:.\ text(Pat is correct.)`

Algebra, STD2 A4 2016 HSC 29b

The mass `M` kg of a baby pig at age `x` days is given by  `M = A(1.1)^x`  where `A` is a constant. The graph of this equation is shown.
 

2ug-2016-hsc-q29_1

  1. What is the value of `A`?   (1 mark)

  2. What is the daily growth rate of the pig’s mass? Write your answer as a percentage.   (1 mark)

Show Answers Only
  1. `1.5\ text(kg)`
  2. `10text(%)`
Show Worked Solution

i.   `text(When)\ x = 0,`

♦ Mean mark (i) 48%.
♦♦♦ Mean mark part (ii) 6%!
`1.5` `= A(1.1)^0`
`:. A` `= 1.5\ text(kg)`

 
ii.   `text(Daily growth rate)`

`= 0.1`

`= 10text(%)`

Financial Maths, STD2 F5 2016 HSC 28d

The table gives the contribution per period for an annuity with a future value of $1 at different interest rates and different periods of time. 
 

2ug-2016-hsc-q28_31
 

Margaret needs to save $75 000 over 6 years for a deposit on a new apartment. She makes regular quarterly contributions into an investment account which pays interest at 3% pa.

How much will Margaret need to contribute each quarter to reach her savings goal?  (2 marks)

Show Answers Only

`$2865`

Show Worked Solution

`text(Periods) = 6 xx 4 = 24`

♦ Mean mark 40%.

`text(Interest rate) = 1/4 xx 3 = 0.75text(%)`

`=>\ text(Table factor = 0.0382)`

`(text(i.e. 3.82 cents contributed per)`

  `text(quarter = $1 after 6 years))`

 

`:.\ text(Quarterly contribution)`

`= 75\ 000 xx 0.0382`

`= $2865`

Statistics, STD2 S1 2016 HSC 27c

The heights of 400 students were measured. The results are displayed in this cumulative frequency polygon.
 

2ug-2016-hsc-q27_2

 
Use the polygon to estimate the interquartile range.  (2 marks)

Show Answers Only

`36\ text(cm)`

Show Worked Solution

`text(See graph for values:)`

♦ Mean mark 46%.

2ug-2016-hsc-q27c-answer

`IQR` `= Q_3 – Q_1`
  `= 172 – 136`
  `= 36\ text(cm)`

Statistics, STD2 S1 2016 HSC 27b

A small population consists of three students of heights 153 cm, 168 cm and 174 cm. Samples of varying sizes can be taken from this population.

What is the mean of the mean heights of all the possible samples? Justify your answer.  (2 marks)

Show Answers Only

`165\ text(cm)`

Show Worked Solution

`text(If sample is 1 person,)`

♦ Mean mark 40%.

`text{Possible mean(s): 153, 168 or 174.}`
 

`text(If sample is 2 people,)`

`text{Possible mean(s):}quad(153 + 168)/2` `= 160.5`
`(153 + 174)/2` `= 163.5`
`(168 + 174)/2` `= 171`

 

`text(If sample is 3 people,)`

`text(Mean:)quad(153 + 168 + 174)/3 = 165`
 

`:.\ text(Mean of all mean heights)`

`= (153 + 168 + 174 + 160.5 + 163.5 + 171 + 165)/7`

`= 165\ text(cm)`

Measurement, STD2 M6 2016 HSC 25 MC

The diagram shows towns `A`, `B` and `C`. Town `B` is 40 km due north of town `A`. The distance from `B` to `C` is 18 km and the bearing of `C` from `A` is 025°. It is known that  `∠BCA`  is obtuse.
 

2ug-2016-hsc-25-mc

 
What is the bearing of `C` from `B`?

  1.    `070°`
  2.    `095°`
  3.    `110°`
  4.    `135°`
Show Answers Only

`=> D`

Show Worked Solution

`text(Using the sine rule,)`

♦ Mean mark 39%.
`(sin∠BCA)/40` `= (sin25^@)/18`
`sin angle BCA` `= (40 xx sin25^@)/18`
  `= 0.939…`
`angle BCA` `= 180 – 69.9quad(angleBCA > 90^@)`
  `= 110.1°`

 

`:. text(Bearing of)\ C\ text(from)\ B`

`= 110.1 + 25qquad(text(external angle of triangle))`

`= 135.1`

 
`=> D`

Probability, STD2 S2 2016 HSC 23 MC

A group of 485 people was surveyed. The people were asked whether or not they smoke. The results are recorded in the table.
 

A person is selected at random from the group.

What is the approximate probability that the person selected is a smoker OR is male?

  1. 33%
  2. 18%
  3. 68%
  4. 87%
Show Answers Only

`=> C`

Show Worked Solution

`P(text(Smoker or a male))`

`= (text(Total males + female smokers))/(text(Total surveyed))`

`= (264 + 68)/485`

`= 0.684…`
 

`=> C`

♦♦ Mean mark 34%.

Statistics, STD2 S1 2016 HSC 22 MC

The box-and-whisker plots show the results of a History test and a Geography test.
 

In History, 112 students completed the test. The number of students who scored above 30 marks was the same for the History test and the Geography test.

How many students completed the Geography test?

  1. 8
  2. 50
  3. 56
  4. 112
Show Answers Only

`=> C`

Show Worked Solution

`text{In History} \ => \  text{Q}_3 = 30\ \text{marks}`

`:.\ text{Scoring over 30}\ = 25text(%) xx 112 = 28\ \text{students}`
 

`text{In Geography} \ => \ text{Median}\ = 30\ \text{marks}`

`:.\ text{Students completing Geography}\ =2 xx 28 = 56\ \text{students}`

`=> C`

Statistics, STD2 S1 2016 HSC 21 MC

A grouped data frequency table is shown.

\begin{array} {|c|c|}
\hline
\rule{0pt}{2.5ex} \textit{Class Interval} \rule[-1ex]{0pt}{0pt} & \ \ \ \ \ \textit{Frequency}\ \ \ \ \  \\
\hline
\rule{0pt}{2.5ex} \text{1 – 5} \rule[-1ex]{0pt}{0pt} & 3 \\
\hline
\rule{0pt}{2.5ex} \text{6 – 10} \rule[-1ex]{0pt}{0pt} & 6 \\
\hline
\rule{0pt}{2.5ex} \text{11 – 15} \rule[-1ex]{0pt}{0pt} & 8 \\
\hline
\rule{0pt}{2.5ex} \text{16 – 20} \rule[-1ex]{0pt}{0pt} & 9 \\
\hline
\end{array}

What is the mean for this set of data?

  1.    6.5
  2.    10.5
  3.    11.9
  4.    12.4
Show Answers Only

`=> D`

Show Worked Solution

`text(Using the centre of each class interval:)`

♦ Mean mark 43%.
`text(Mean)` `= (3 xx 3 + 8 xx 6 + 13 xx 8 + 18 xx 9)/(3 + 6 + 8 + 9)`
  `= 12.42…`

`=> D`

Statistics, STD2 S1 2016 HSC 19 MC

A soccer referee wrote down the number of goals scored in 9 different games during the season.

`2,  \ 3,  \ 3,  \ 3,  \ 5,  \ 5,  \ 8,  \ 9,  \ ...`

The last number has been omitted. The range of the data is 10.

What is the five-number summary for this data set?

  1. `2, 3, 5, 8.5, 12`
  2. `2, 3, 5, 8.5, 10`
  3. `2, 3, 5, 8, 12`
  4. `2, 3, 5, 8, 10`
Show Answers Only

`=> A`

Show Worked Solution

`text{Since range is 10} \ => \ text{Last data point = 12}`

`text{Q}_1 = 3`

`text{Q}_3 = (8 + 9)/2 = 8.5`

`text(Median = 5)`

`=> A`

♦ Mean mark 46%.

Statistics, STD2 S5 2016 HSC 13 MC

The speed limit outside a school is 40 km/h. Year 11 students measured the speed of passing vehicles over a period of time. They found the set of data to be normally distributed with a mean speed of 36 km/h and a standard deviation of 2 km/h.

What percentage of the vehicles passed the school at a speed greater than 40 km/h?

  1. `text(2.5%)`
  2. `text(5%)`
  3. `text(47.5%)`
  4. `text(95%)`
Show Answers Only

`=> A`

Show Worked Solution
`z` `= (x – mu)/sigma`
  `= (40 – 36)/2`
  `= 2`

2ug-2016-hsc-13-mc-answer

`=> A`

Financial Maths, STD2 F4 2016 HSC 8 MC

The table shows the future value of an investment of $1000, compounding yearly, at varying interest rates for different periods of time.
 

2ug-2016-hsc-8-mc 

 
Based on the information provided, what is the future value of an investment of $2500 over 3 years at 4% pa?

  1.    $1124.86
  2.    $2812.15
  3.    $3624.86
  4.    $5312.15
Show Answers Only

`=> B`

Show Worked Solution

`text(Table factor) = 1124.86`

`:. FV` `= 2.5 xx 1124.86`
  `= $2812.15`

 
`=> B`

Statistics, STD2 S1 2016 HSC 7 MC

Which set of data is classified as categorical and nominal?

  1. blue, green, yellow
  2. small, medium, large
  3. 5.2 cm, 6 cm, 7.21 cm
  4. 4 people, 5 people, 9 people 
Show Answers Only

`A`

Show Worked Solution

`text(Categorical and nominal data is)`

♦♦ Mean mark 26%.

`text(qualitative and not ordered.)`

`=> A`

Statistics, STD2 S4 2016 HSC 3 MC

The graph shows a scatterplot for a set of data.
 

2ug-2016-hsc-3-mc 

 
Which of the following is the best approximation for the correlation coefficient of this set of data?

  1.    `−1`
  2.    `−0.3`
  3.    `0.3`
  4.    `1`
Show Answers Only

`B`

Show Worked Solution

`text(Correlation is negative and)`

`text(weak.)`

`=> B`

Statistics, STD2 S5 SM-Bank 2 MC

The pulse rates of a large group of 18-year-old students are approximately normally distributed with a mean of 75 beats/minute and a standard deviation of 11 beats/minute. 

The percentage of 18-year-old students with pulse rates less than 53 beats/minute or greater than 86 beats/minute is closest to

  1. `2.5text(%)` 
  2. `5text(%)` 
  3. `16text(%)` 
  4. `18.5text(%)` 
Show Answers Only

`D`

Show Worked Solution

`mu=75,\ \ \ sigma=11`

COMMENT: Two applications of the 68-95-99.7% rule are required. A good strategy is to first draw a normal curve and shade the required areas.
`z text{-score (53)}` `=(x-mu) /sigma`
   `=(53-75)/11`
   `= – 2`
`z text{-score (86)}` `= (86-75)/11`
  `=1`

core 2008 VCAA 6-7

`text(From the diagram, the % of students that have a)`

`z text(-score below – 2 or above 1)`

 `=2.5+16`

`=18.5 text(%)`

 `=>D`

Statistics, STD2 S5 SM-Bank 1 MC

The head circumference (in cm) of a population of infant boys is normally distributed with a mean of 49.5 cm and a standard deviation of 1.5 cm.

Four hundred of these boys are selected at random and each boy’s head circumference is measured.

The number of these boys with a head circumference of less than 48.0 cm is closest to 

  1. `3`
  2. `10`
  3. `64`
  4. `272`
Show Answers Only

`C`

Show Worked Solution

`mu=49.5,\ \ sigma=1.5`

`z text{-score (49.5)` `=(x-mu)/sigma`
  `=(48.0-49.5)/1.5`
  `=– 1`

 

`:.\ text(Number of boys with a head under 48.0 cm)`

`=16text(%) xx 400`

`=64`

`=>  C`

Financial Maths, STD2 F5 2015 HSC 30c

The table gives the present value interest factors for an annuity of $1 per period, for various interest rates `(r)` and numbers of periods `(N)`.

2015 30c

  1. Oscar plans to invest $200 each month for 74 months. His investment will earn interest at the rate of 0.0080 (as a decimal) per month.

     

    Use the information in the table to calculate the present value of this annuity.  (1 mark)

  2. Lucy is using the same table to calculate the loan repayment for her car loan. Her loan is `$21\ 500` and will be repaid in equal monthly repayments over 6 years. The interest rate on her loan is 10.8% per annum.

     

    Calculate the amount of each monthly repayment, correct to the nearest dollar.  (2 marks)

Show Answers Only
  1. `$11\ 136.89\ \ text{(nearest cent)}`
  2. `$407\ \ text{(nearest dollar)}`
Show Worked Solution

i.    `N = 74, r = 0.0080`

♦ Mean mark 48%.

`PVtext{(annuity) table factor}\ = 55.68446`

`:.PV\ text(of annuity)`

`= $200 xx 55.68446`

`= $11\ 136.892`

`= $11\ 136.89\ \ text{(nearest cent)}`

 

ii.  `text(Over 6 years)`

♦♦ Mean mark 33%.

`N = 6 xx 12 = 72\ text(months)`

`r = 10.8/12 = text(0.9%) = 0.009`

`PVtext{(annuity) table factor}\ =52.82118`
 

`text(Let)\ $M =\ text(monthly repayment)`

`text(Loan)\ = PV\ text(of annuity)`

`$21\ 500` `= M xx 52.82118`
 `:.\ M` `= $407.033…`
  `= $407\ \ text{(nearest dollar)}`

Statistics, STD2 S1 2015 HSC 29d

Data from 200 recent house sales are grouped into class intervals and a cumulative frequency histogram is drawn.
 

2UG 2015 29d1 
 

  1. Use the graph to estimate the median house price.   (1 mark)

  2. By completing the table, calculate the mean house price.   (3 marks)

\begin{array} {|c|c|}
\hline
\rule{0pt}{2.5ex} \text{Class Centre} \rule[-1ex]{0pt}{0pt} & \text{Frequency} \\ \text{(\$'000)} &  \\
\hline
\rule{0pt}{2.5ex}  \rule[-1ex]{0pt}{0pt} &  \\
\hline
\rule{0pt}{2.5ex}  \rule[-1ex]{0pt}{0pt} &  \\
\hline
\rule{0pt}{2.5ex}  \rule[-1ex]{0pt}{0pt} &  \\
\hline
\rule{0pt}{2.5ex}  \rule[-1ex]{0pt}{0pt} & \\
\hline
\end{array}

Show Answers Only
  1. `text(See Worked Solutions.)`
  2. `text(See Worked Solutions.)`
Show Worked Solution
i.   

2UG 2015 29d Answer

`text(From the graph, the estimated median)`

`text(house price = $392 500)`

♦♦♦ Mean marks of 9% for part (i) and 34% for part (ii)!

 
ii.

\begin{array} {|c|c|}
\hline
\rule{0pt}{2.5ex} \text{Class Centre} \rule[-1ex]{0pt}{0pt} & \text{Frequency} \\ \text{(\$’000)} &  \\
\hline
\rule{0pt}{2.5ex}  375 \rule[-1ex]{0pt}{0pt} & 30\\
\hline
\rule{0pt}{2.5ex}  385 \rule[-1ex]{0pt}{0pt} &  50 \\
\hline
\rule{0pt}{2.5ex}  395 \rule[-1ex]{0pt}{0pt} & 70 \\
\hline
\rule{0pt}{2.5ex}  405 \rule[-1ex]{0pt}{0pt} & 50 \\
\hline
\end{array}

 
`text(Mean house price ($’000))`

`= (375xx30 + 385xx50 + 395xx70 + 405xx50)/200`

`=$392`

`:. text(Mean house price is)\ $392\ 000`

Statistics, STD2 S4 2015 HSC 28e

The shoe size and height of ten students were recorded.

\begin{array} {|l|c|c|}
\hline \rule{0pt}{2.5ex} \text{Shoe size} \rule[-1ex]{0pt}{0pt} & \text{6} & \text{7} & \text{7} & \text{8} & \text{8.5} & \text{9.5} & \text{10} & \text{11} & \text{12} & \text{12} \\
\hline \rule{0pt}{2.5ex} \text{Height} \rule[-1ex]{0pt}{0pt} & \text{155} & \text{150} & \text{165} & \text{175} & \text{170} & \text{170} & \text{190} & \text{185} & \text{200} & \text{195} \\
\hline
\end{array}

  1. Complete the scatter plot AND draw a line of fit by eye.  (2 marks)
     
     
  2. Use the line of fit to estimate the height difference between a student who wears a size 7.5 shoe and one who wears a size 9 shoe.  (1 mark)

  3. A student calculated the correlation coefficient to be 1 for this set of data. Explain why this cannot be correct.  (1 mark)

Show Answers Only
  1. `text(See Worked Solutions.)`
  2. `13\ text{cm  (or close given LOBF drawn)}
  3. `text(A correlation co-efficient of 1 would)`
    `text(mean that all data points occur on the)`
    `text(line of best fit which clearly isn’t the case.)`
Show Worked Solution

i.    
      2UG 2015 28e Answer

ii.   `text{Shoe size 7½ gives a height estimate of 162 cm (see graph)}`

`text{Shoe size 9 gives a height estimate of 175 cm (see graph)}`

`:.\ text(Height difference)` `= 175-162`
  `= 13\ text{cm  (or close given LOBF drawn)}`

 

iii.   `text(A correlation co-efficient of 1 would mean)`

♦ Mean mark (c) 39%.

`text(that all data points occur on the line of best)`

`text(fit which clearly isn’t the case.)`

Statistics, STD2 S5 2015 HSC 28b

The results of two tests are normally distributed. The mean and standard deviation for each test are displayed in the table.
 

2015 28b

 
Kristoff scored 74 in Mathematics and 80 in English. He claims that he has performed better in English.

Is Kristoff correct? Justify your answer using appropriate calculations.  (2 marks)

Show Answers Only

`text(He is correct.)`

Show Worked Solution

`text(In Maths)`

♦ Mean mark 44%.
`ztext{-score(74)}` `= (x − mu)/sigma`
  `= (74 − 70)/6.5`
  `= 0.6153…`

 
`text(In English)`

`ztext{-score(80)}` `= (80 − 75)/8`
  `= 0.625`

 
`=>\ text(Kristoff’s)\ ztext(-score in English is higher than)`

`text(his)\ z text(-score in Maths.)`

`:.\ text(He is correct. He performed better in English.)`

Statistics, STD2 S1 2015 HSC 27d

In a small business, the seven employees earn the following wages per week:

\(\$300, \ \$490, \ \$520, \ \$590, \ \$660, \ \$680, \ \$970\)

  1.  Is the wage of $970 an outlier for this set of data? Justify your answer with calculations.  (3 marks)

  2.  Each employee receives a $20 pay increase.

     

     What effect will this have on the standard deviation?  (1 mark)

Show Answers Only

i.    \(\text{See Worked Solutions.} \)

ii.    \(\text{The standard deviation will remain the same.}\)

Show Worked Solution

i.    \(300, 490, 520, 590, 660, 680, 970\)

\(\text{Median}\) \(= 590\)
\(Q_1\) \(= 490\)
\(Q_3\) \(= 680\)
\(IQR\) \(= 680-490 = 190\)

 

\(\text{Outlier if \$970 is greater than:} \)

\(Q_3 + 1.5 x\times IQR = 680 + 1.5 \times 190 = \$965 \) 

\(\therefore\ \text{The wage \$970 per week is an outlier.}\)

♦ Mean mark (i) 39%.


ii.    \(\text{All values increase by \$20, but so too does the mean.} \)

\(\text{Therefore the spread about the new mean will not change} \)

\(\text{and therefore the standard deviation will remain the same.} \)

Algebra, STD2 A2 2015 HSC 27c

Ariana’s parents have given her an interest‑free loan of $4800 to buy a car. She will pay them back by paying `$x` immediately and `$y` every month until she has repaid the loan in full.

After 18 months Ariana has paid back $1510, and after 36 months she has paid back $2770.

This information can be represented by the following equations.

`x + 18y = 1510`

`x + 36y = 2770`

  1. Graph these equations below and use to solve simultaneously for the values of `x` and `y`.   (2 marks)

         

  2. How many months will it take Ariana to repay the loan in full? (2 marks)

Show Answers Only
  1. `x = 250, \ y = 70`
  2. `text(65 months)`
Show Worked Solution

i.

 
`:.\ text(Solution is)\ \ x = 250, \ y = 70`
 

ii.  `text(Let)\ \ A = text(the amount paid back after)\ n\ text(months)`

`A = 250 + 70n`

♦ Mean mark 44%.

`text(Find)\ n\ text(when)\ A = 4800`

`250 + 70n` `= 4800`
`70n` `= 4550`
`n` `= 65`

 

`:.\ text(It will take Ariana 65 months to repay)`

`text(the loan in full.)`

Probability, STD2 S2 2015 HSC 26e

The table shows the relative frequency of selecting each of the different coloured jelly beans from packets containing green, yellow, black, red and white jelly beans.

\begin{array} {|c|c|}
\hline
\rule{0pt}{2.5ex} \textit{Colour} \rule[-1ex]{0pt}{0pt} & \textit{Relative frequency} \\
\hline
\rule{0pt}{2.5ex} \text{Green} \rule[-1ex]{0pt}{0pt} & 0.32 \\
\hline
\rule{0pt}{2.5ex} \text{Yellow} \rule[-1ex]{0pt}{0pt} & 0.13 \\
\hline
\rule{0pt}{2.5ex} \text{Black} \rule[-1ex]{0pt}{0pt} & 0.14 \\
\hline
\rule{0pt}{2.5ex} \text{Red} \rule[-1ex]{0pt}{0pt} &  \\
\hline
\rule{0pt}{2.5ex} \text{White} \rule[-1ex]{0pt}{0pt} & 0.24 \\
\hline
\end{array}

  1. What is the relative frequency of selecting a red jelly bean?  (1 mark)

  2. Based on this table of relative frequencies, what is the probability of NOT selecting a black jelly bean?  (1 mark)

Show Answers Only
  1. \(0.17\)
  2. \(0.86\)
Show Worked Solution

i.  \(\text{Relative frequency of red}\)

\(= 1-(0.32 + 0.13 + 0.14 + 0.24)\)

\(= 1-0.83\)

\(= 0.17\)

 

ii.  \(P\text{(not selecting black)}\)

\(= 1-P\text{(selecting black)}\)

\(= 1-0.14\)

\(= 0.86\)

Financial Maths, STD2 F4 2015 HSC 26d

A family currently pays $320 for some groceries.

Assuming a constant annual inflation rate of 2.9%, calculate how much would be paid for the same groceries in 5 years’ time.  (2 marks)

Show Answers Only

`$369.17\ \ text{(nearest cent)}`

Show Worked Solution
`FV` `= PV(1 + r)^n`
  `= 320(1.029)^5`
  `= $369.1703…`
  `= $369.17\ \ text{(nearest cent)}`

Algebra, STD2 A1 2015 HSC 24 MC

Consider the equation  `(2x)/3-4 = (5x)/2 + 1`.

Which of the following would be a correct step in solving this equation?

  1. `(2x)/3-3 = (5x)/2`
  2. `(2x)/3 = (5x)/2 + 5`
  3. `2x-4 = (15x)/2 + 3`
  4. `(4x)/6-8 = 5x + 2`
Show Answers Only

`B`

Show Worked Solution
`(2x)/3-4` `= (5x)/2 + 1`
`(2x)/3` `= (5x)/2 + 5`

 
`=>B`