Let
Find
--- 4 WORK AREA LINES (style=lined) ---
Aussie Maths & Science Teachers: Save your time with SmarterEd
Let
Find
--- 4 WORK AREA LINES (style=lined) ---
The solution set of the equation
A.
B.
C.
D.
E.
The random variable
If the random variable
If
Let
Then
For the polynomial
The value of
A.
B.
C.
D.
E.
If
A.
B.
C.
D.
E.
The graph of the function
The graph of the function
The inverse of the function
The graph shown is
--- 1 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
On the same set of axes, draw the graph
--- 10 WORK AREA LINES (style=blank) ---
Let
--- 4 WORK AREA LINES (style=lined) ---
Find an anti-derivative of
--- 2 WORK AREA LINES (style=lined) ---
Differentiate with respect to
Let
--- 4 WORK AREA LINES (style=lined) ---
The rule for
--- 6 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
Find the value of
--- 3 WORK AREA LINES (style=lined) ---
Find an antiderivative of
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
| a. | ||
b.
--- 3 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
a.
b.
Differentiate with respect to
--- 6 WORK AREA LINES (style=lined) ---
Find
--- 2 WORK AREA LINES (style=lined) ---
Differentiate with respect to
--- 3 WORK AREA LINES (style=lined) ---
Differentiate
--- 2 WORK AREA LINES (style=lined) ---
Differentiate with respect to
--- 3 WORK AREA LINES (style=lined) ---
Let
Find the value of
--- 5 WORK AREA LINES (style=lined) ---
Let
Find the equation of the tangent to
--- 5 WORK AREA LINES (style=lined) ---
Solve the equation
Which expression is equivalent to
Let
Let
Evaluate
--- 4 WORK AREA LINES (style=lined) ---
The Bouncy Ball Company (BBC) makes tennis balls whose diameters are normally distributed with mean 67 mm and standard deviation 1 mm. The tennis balls are packed and sold in cylindrical tins that each hold four balls. A tennis ball fits into such a tin if the diameter of the ball is less than 68.5 mm.
--- 5 WORK AREA LINES (style=lined) ---
BBC management would like each ball produced to have diameter between 65.6 and 68.4 mm.
--- 5 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
BBC management wants engineers to change the manufacturing process so that 99% of all balls produced have diameter between 65.6 and 68.4 mm. The mean is to stay at 67 mm but the standard deviation is to be changed.
--- 6 WORK AREA LINES (style=lined) ---
a.
b.
c.i.
c.ii.
d.
Let
The graph of
--- 3 WORK AREA LINES (style=lined) ---
Find the length of
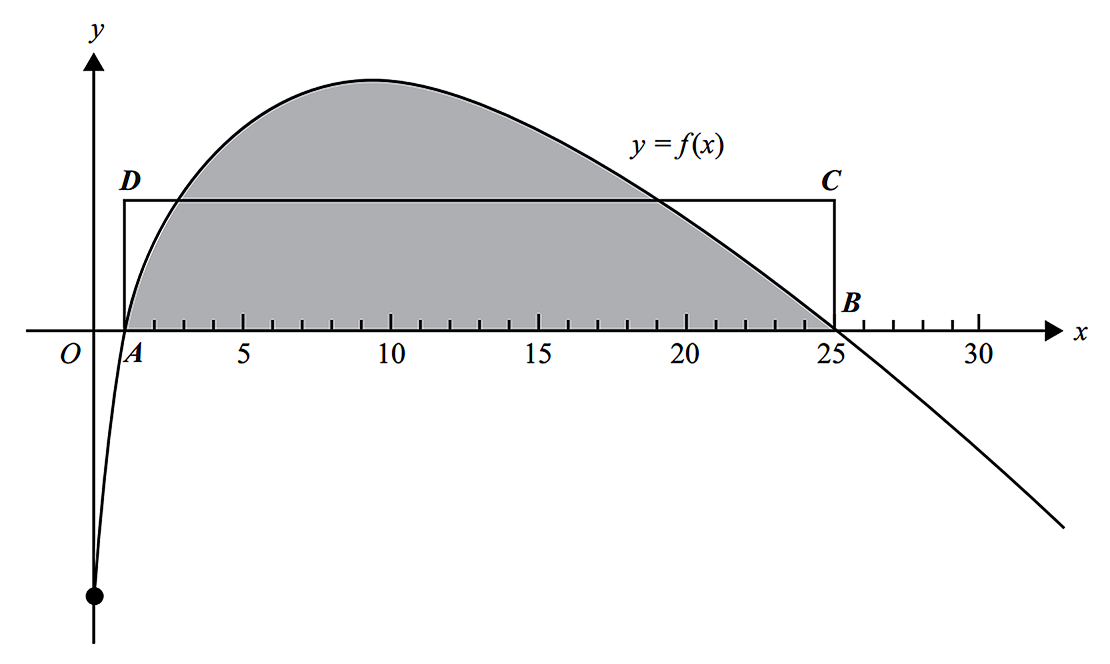
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
a.
b.
c.i.
c.ii.
For
The maximal domain
--- 1 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
a.i.
a.ii.
b.i.
b.ii.
c.i.
c.ii.
| d.i. | ||
d.ii.
If
A school has a class set of 22 new laptops kept in a recharging trolley. Provided each laptop is correctly plugged into the trolley after use, its battery recharges.
On a particular day, a class of 22 students uses the laptops. All laptop batteries are fully charged at the start of the lesson. Each student uses and returns exactly one laptop. The probability that a student does not correctly plug their laptop into the trolley at the end of the lesson is 10%. The correctness of any student’s plugging-in is independent of any other student’s correctness.
--- 5 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
The time for which a laptop will work without recharging (the battery life) is normally distributed, with a mean of three hours and 10 minutes and standard deviation of six minutes. Suppose that the laptops remain out of the recharging trolley for three hours.
--- 5 WORK AREA LINES (style=lined) ---
A supplier of laptops decides to take a sample of 100 new laptops from a number of different schools. For samples of size 100 from the population of laptops with a mean battery life of three hours and 10 minutes and standard deviation of six minutes,
--- 6 WORK AREA LINES (style=lined) ---
It is known that when laptops have been used regularly in a school for six months, their battery life is still normally distributed but the mean battery life drops to three hours. It is also known that only 12% of such laptops work for more than three hours and 10 minutes.
--- 5 WORK AREA LINES (style=lined) ---
The laptop supplier collects a sample of 100 laptops that have been used for six months from a number of different schools and tests their battery life. The laptop supplier wishes to estimate the proportion of such laptops with a battery life of less than three hours.
--- 2 WORK AREA LINES (style=lined) ---
The laptop supplier finds that, in a particular sample of 100 laptops, six of them have a battery life of less than three hours.
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
Consider the function
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
The diagram below shows part of the graph of
The tangent cuts the
--- 2 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
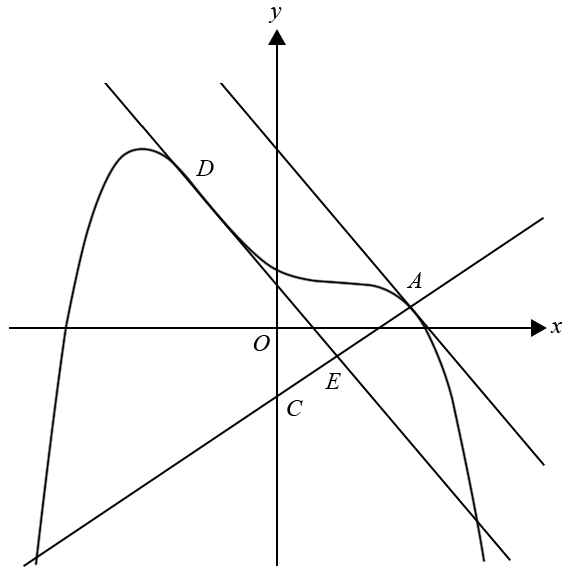
i. Find the coordinates of
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
Let
--- 4 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Find the value of
--- 8 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
a.
b.
c.
d.
e.
f.
A bag contains four white balls and six black balls. Three balls are drawn from the bag without replacement.
The probability that they are all black is
The function
If
A function
●
● the graph of
A.
B.
C.
D.
E.
If
The random variable,
If the random variable,
A box contains six red marbles and four blue marbles. Two marbles are drawn from the box, without replacement.
The probability that they are the same colour is
Which one of the following is the inverse function of
The average value of the function with rule
The inverse function of
The midpoint of the line segment joining
Spiro is saving for a car. He has an account with $3500 in it at the start of the year.
At the end of each month, Spiro adds another $180 to the account.
The account pays 3.6% interest per annum, compounded monthly.
--- 1 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
| a.i. | ||
| a.ii. | ||
| b. | ||
Joe buys a tractor under a buy-back scheme. This scheme gives Joe the right to sell the tractor back to the dealer through either a flat rate depreciation or unit cost depreciation.
--- 4 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
| a.i. | ||
| a.ii. |
| b.i. | ||
b.ii.