Draw the symbols which match the electronic components named in the table. (3 marks)
--- 0 WORK AREA LINES (style=lined) ---
Aussie Maths & Science Teachers: Save your time with SmarterEd
Nitric acid can be produced industrially using the process shown.
--- 6 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
a. Consider the equilibrium system in reactor 2:
→
→ Le Chatelier’s Principle states that the position of equilibrium will shift to the left (as per the equilibrium equation above) to counter the depletion of
→ This shift results in the further depletion of
b. Design improvements:
→ A catalyst could be used in Reactor 1 to lower the activation energy required for the reaction to occur. This would decrease the required temperature, making the process more energy efficient.
→ Water is disposed of in Separator 3 and is required as a reactant in Reactor 3. A design improvement would be to recycle (rather than dispose) this water for use in Reactor 3.
Other answers could include:
→ Capture the heat energy released from the cooler/condenser step between the processes in Reactor 1 and Reactor 2. This should be engineered so it can then be used in the Emissions control step, thus reducing the energy consumption of the overall nitric acid production.
→ The
a. Consider the equilibrium system in reactor 2:
→
→ Le Chatelier’s Principle states that the position of equilibrium will shift to the left (as per the equilibrium equation above) to counter the depletion of
→ This shift results in the further depletion of
b. Design improvements:
→ A catalyst could be used in Reactor 1 to lower the activation energy required for the reaction to occur. This would decrease the required temperature, making the process more energy efficient.
→ Water is disposed of in Separator 3 and is required as a reactant in Reactor 3. A design improvement would be to recycle (rather than dispose) this water for use in Reactor 3.
Other answers could include:
→ Capture the heat energy released from the cooler/condenser step between the processes in Reactor 1 and Reactor 2. This should be engineered so it can then be used in the Emissions control step, thus reducing the energy consumption of the overall nitric acid production.
→ The
Lung cancer can be linked to genetic causes. One of the genes frequently studied in lung cancer tissue is the Epidermal Growth Factor Receptor (EGFR) gene. It codes for EGFR protein, which is composed of one polypeptide chain.
--- 12 WORK AREA LINES (style=blank) ---
The structure of the EGFR protein includes a receptor and an enzyme component. The function of the protein is to help the cell to regulate cell division.
EGFR mutations are present in about 32% of cases of Non-Small Cell Lung Cancer (the most common type of lung cancer).
--- 8 WORK AREA LINES (style=lined) ---
i.
ii. → A mutation is a permanent change to any section of DNA.
→ It can include the change in a single nucleotide base, the deletion or insertion of a base, which will alter all codons proceeding it, or the translation, deletion or addition or entire segments of a chromosome.
→ If this occurred in the EGFR gene, this will be copied onto the mRNA, which will be transcribed into the EGFR protein.
→ The change of the removal or addition of certain amino acids within the polypeptide chain or even the change in a single amino acid will change the function of the protein or make it unusable at all. In rare cases, this could be beneficial, or a change in an amino acid will be insignificant and not change the proteins shape.
→ In most cases however, the mutation on the EFGR gene will render it useless, leading to uncontrolled cell division (cancer).
ii. → A mutation is a permanent change to any section of DNA.
→ It can include the change in a single nucleotide base, the deletion or insertion of a base, which will alter all codons proceeding it, or the translation, deletion or addition or entire segments of a chromosome.
→ If this occurred in the EGFR gene, this will be copied onto the mRNA, which will be transcribed into the EGFR protein.
→ The change of the removal or addition of certain amino acids within the polypeptide chain or even the change in a single amino acid will change the function of the protein or make it unusable at all. In rare cases, this could be beneficial, or a change in an amino acid will be insignificant and not change the proteins shape.
→ In most cases however, the mutation on the EFGR gene will render it useless, leading to uncontrolled cell division (cancer).
| The flow chart shows reactions involving five different organic compounds, | to |
| Draw the structure of each compound | to | in the corresponding space provided. (5 marks) |
--- 0 WORK AREA LINES (style=lined) ---
What is the structure of
Drawing out the condensed structural formula matches the structure in A.
A bar magnet is moved away from a stationary coil.
Which diagram correctly shows the direction of the induced current in the coil and the resulting magnetic polarity of the coil?
The current through the solenoid will produce a force that opposes the magnets motion (Lenz’s law).
So, there will be a north pole on the right hand side of the magnet. The right hand grip rule gives the direction of current.
The three major shopping centres in a large city, Eastmall
The total number of shoppers at each of the centres at 1.00 pm on a typical day is shown in matrix
Each of these centres has three major shopping areas: food
The proportion of shoppers in each of these three areas at 1.00 pm on a typical day is the same at all three centres and is given in matrix
`qquad qquad qquad P = [(0.48), (0.27), (0.25)] {:(F),(C),(M):}
If this were to happen, how many shoppers, in total, would be at Grandmall at this time? (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
The average daily amount spent, in dollars, by each shopper in each of the three areas at Grandmall in 2019 is shown in matrix
On one particular day, 135 shoppers spent the average daily amount on food, 143 shoppers spent the average daily amount on clothing and 131 shoppers spent the average daily amount on merchandise.
--- 3 WORK AREA LINES (style=lined) ---
| Area | food | clothing | merchandise |
| Expected change | increase by 5% | decrease by 15% | decrease by 1% |
The average daily amount, in dollars, expected to be spent in each area in 2020 can be determined by forming the matrix product
--- 3 WORK AREA LINES (style=lined) ---
a.
| b. | ||
| c.i. |
| c.ii. | |
d.
| e. | ||
The table below shows the mean age, in years, and the mean height, in centimetres, of 648 women from seven different age groups.
--- 1 WORK AREA LINES (style=lined) ---
A scatterplot displaying this data shows an association between the mean height and the mean age of these women. In an initial analysis of the data, a line is fitted to the data by eye, as shown.
--- 1 WORK AREA LINES (style=lined) ---
Using these two points, determine the equation of this line. Write the values of the intercept and the slope in the appropriate boxes below.
Round your answers to three significant figures. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
| mean height = |
|
+ |
|
× mean age |
The associated residual plot that was generated is shown below.
The residual plot indicates that the association between the mean height and the mean age of women is non-linear.
The data presented in the table in part a is repeated below. It can be linearised by applying an appropriate transformation to the variable mean age.
Apply an appropriate transformation to the variable mean age to linearise the data. Fit a least squares line to the transformed data and write its equation below.
Round the values of the intercept and the slope to four significant figures. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
| a. | ||
b.
c.
D.
The zoo’s management requests quotes for parts of the new building works.
Four businesses each submit quotes for four different tasks.
Each business will be offered only one task.
The quoted cost, in $100 000, of providing the work is shown in Table 1 below.
The zoo’s management wants to complete the new building works at minimum cost.
The Hungarian algorithm is used to determine the allocation of tasks to businesses.
The first step of the Hungarian algorithm involves row reduction; that is, subtracting the smallest element in each row of Table 1 from each of the elements in that row.
The result of the first step is shown in Table 2 below.
The second step of the Hungarian algorithm involves column reduction; that is, subtracting the smallest element in each column of Table 2 from each of the elements in that column.
The results of the second step of the Hungarian algorithm are shown in Table 3 below. The values of Task 1 are given as
--- 1 WORK AREA LINES (style=lined) ---
Draw these three lines on Table 3 above. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
When all steps of the Hungarian algorithm are complete, a bipartite graph can show the allocation for minimum cost.
Complete the bipartite graph below to show this allocation for minimum cost. (1 mark)
--- 4 WORK AREA LINES (style=lined) ---
How much is this reduction? (1 mark)
--- 6 WORK AREA LINES (style=lined) ---
A random sample of 12 mammals drawn from a population of 62 types of mammals was categorized according to two variables.
likelihood of attack (1 = low, 2 = medium, 3 = high)
exposure to attack during sleep (1 = low, 2 = medium, 3 = high)
The data is shown in the following table.
--- 0 WORK AREA LINES (style=lined) ---
The following two-way frequency table was formed from the data generated when the entire population of 62 types of mammals was similarly categorized.
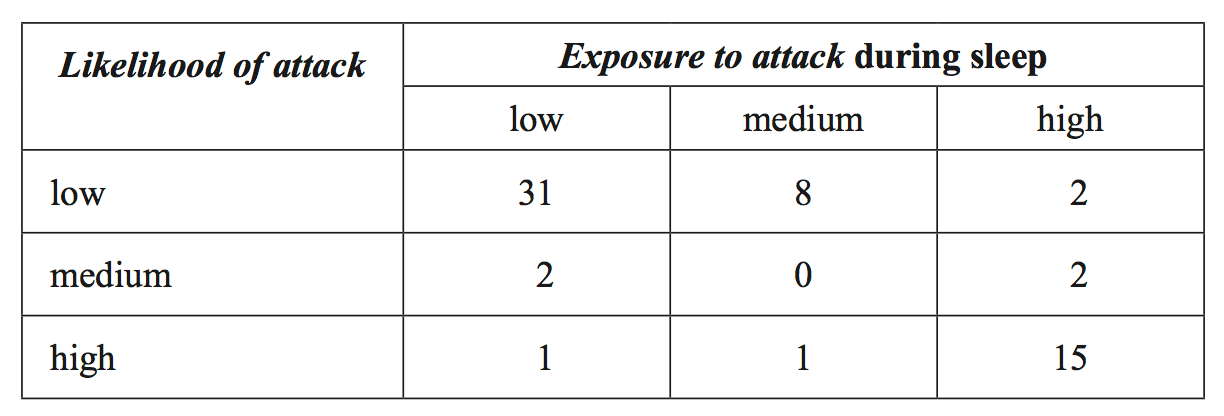
--- 5 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
a.
b. i.
| ii. |
|
iii.
The life span, in years, and gestation period, in days, for 19 types of mammals are displayed in the table below.
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
a.
b.
c.
The table below displays the average sleep time, in hours, for a sample of 19 types of mammals.
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
a.
b.
| c. |
|
| d. |
|
Members of the association will travel to a conference in cars and minibuses:
The constraints above can be represented by the following three inequalities.
A maximum of 60 people can attend the conference.
Use this information to write Inequality 4. (1 mark)
The graph below shows the four lines representing Inequalities 1 to 4.
Also shown on this graph are four of the integer points that satisfy Inequalities 1 to 4. Each of these integer points is marked with a cross (
Each car will cost $70 to hire and each minibus will cost $100 to hire.
The cost of hiring each minibus remained $100.
All original constraints apply.
If the increase in the cost of hiring each car is more than
Determine the value of
a.
| b. |
c.
d.
e.
The theme park has four locations, Air World
The number of visitors at each of the four locations is counted every hour.
By 10 am on Saturday the park had reached its capacity of 2000 visitors and could take no more visitors.
The park stayed at capacity until the end of the day
The state matrix,
--- 2 WORK AREA LINES (style=lined) ---
Let
The number of visitors expected at each location
--- 0 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Matrix
Matrix
--- 4 WORK AREA LINES (style=lined) ---
a.
| b. | ||
c.
| d. | |
|
|
The car park at a theme park has three areas,
The number of empty
--- 1 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
Drivers must pay a parking fee for each hour of parking.
Matrix
where matrix
Write down matrix
--- 2 WORK AREA LINES (style=lined) ---
The number of whole hours that each of the 110 cars had been parked was recorded at 1 pm. Matrix
Complete the matrix
--- 0 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
a.
b.
c.
d.
| e. | ||
The scatterplot below shows the atmospheric pressure, in hectopascals (hPa), at 3 pm (pressure 3 pm) plotted against the atmospheric pressure, in hectopascals, at 9 am (pressure 9 am) for 23 days in November 2017 at a particular weather station.
A least squares line has been fitted to the scatterplot as shown.
The equation of this line is
pressure 3 pm = 111.4 + 0.8894 × pressure 9 am
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
a.
| b. | ||
c.
| d. | ||
e.i.
| e.ii. | ||
| f.i. | |
|
|
f.ii.
Probability Table for Standard Normal Distribution
Negative z-scores
| z | 0.00 | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 | 0.06 | 0.07 | 0.08 | 0.09 |
| -3.4 | 0.0003 | 0.0003 | 0.0003 | 0.0003 | 0.0003 | 0.0003 | 0.0003 | 0.0003 | 0.0003 | 0.0002 |
| -3.3 | 0.0005 | 0.0005 | 0.0005 | 0.0004 | 0.0004 | 0.0004 | 0.0004 | 0.0004 | 0.0004 | 0.0003 |
| -3.2 | 0.0007 | 0.0007 | 0.0006 | 0.0006 | 0.0006 | 0.0006 | 0.0006 | 0.0005 | 0.0005 | 0.0005 |
| -3.1 | 0.0010 | 0.0009 | 0.0009 | 0.0009 | 0.0008 | 0.0008 | 0.0008 | 0.0008 | 0.0007 | 0.0007 |
| -3.0 | 0.0013 | 0.0013 | 0.0013 | 0.0012 | 0.0012 | 0.0011 | 0.0011 | 0.0011 | 0.0010 | 0.0010 |
| -2.9 | 0.0019 | 0.0018 | 0.0018 | 0.0017 | 0.0016 | 0.0016 | 0.0015 | 0.0015 | 0.0014 | 0.0014 |
| -2.8 | 0.0026 | 0.0025 | 0.0024 | 0.0023 | 0.0023 | 0.0022 | 0.0021 | 0.0021 | 0.0020 | 0.0019 |
| -2.7 | 0.0035 | 0.0034 | 0.0033 | 0.0032 | 0.0031 | 0.0030 | 0.0029 | 0.0028 | 0.0027 | 0.0026 |
| -2.6 | 0.0047 | 0.0045 | 0.0044 | 0.0043 | 0.0041 | 0.0040 | 0.0039 | 0.0038 | 0.0037 | 0.0036 |
| -2.5 | 0.0062 | 0.0060 | 0.0059 | 0.0057 | 0.0055 | 0.0054 | 0.0052 | 0.0051 | 0.0049 | 0.0048 |
| -2.4 | 0.0082 | 0.0080 | 0.0078 | 0.0075 | 0.0073 | 0.0071 | 0.0069 | 0.0068 | 0.0066 | 0.0064 |
| -2.3 | 0.0107 | 0.0104 | 0.0102 | 0.0099 | 0.0096 | 0.0094 | 0.0091 | 0.0089 | 0.0087 | 0.0084 |
| -2.2 | 0.0139 | 0.0136 | 0.0132 | 0.0129 | 0.0125 | 0.0122 | 0.0119 | 0.0116 | 0.0113 | 0.0110 |
| -2.1 | 0.0179 | 0.0174 | 0.0170 | 0.0166 | 0.0162 | 0.0158 | 0.0154 | 0.0150 | 0.0146 | 0.0143 |
| -2.0 | 0.0228 | 0.0222 | 0.0217 | 0.0212 | 0.0207 | 0.0202 | 0.0197 | 0.0192 | 0.0188 | 0.0183 |
| -1.9 | 0.0287 | 0.0281 | 0.0274 | 0.0268 | 0.0262 | 0.0256 | 0.0250 | 0.0244 | 0.0239 | 0.0233 |
| -1.8 | 0.0359 | 0.0351 | 0.0344 | 0.0336 | 0.0329 | 0.0322 | 0.0314 | 0.0307 | 0.0301 | 0.0294 |
| -1.7 | 0.0446 | 0.0436 | 0.0427 | 0.0418 | 0.0409 | 0.0401 | 0.0392 | 0.0384 | 0.0375 | 0.0367 |
| -1.6 | 0.0548 | 0.0537 | 0.0526 | 0.0516 | 0.0505 | 0.0495 | 0.0485 | 0.0475 | 0.0465 | 0.0455 |
| -1.5 | 0.0668 | 0.0655 | 0.0643 | 0.0630 | 0.0618 | 0.0606 | 0.0594 | 0.0582 | 0.0571 | 0.0559 |
| -1.4 | 0.0808 | 0.0793 | 0.0778 | 0.0764 | 0.0749 | 0.0735 | 0.0721 | 0.0708 | 0.0694 | 0.0681 |
| -1.3 | 0.0968 | 0.0951 | 0.0934 | 0.0918 | 0.0901 | 0.0885 | 0.0869 | 0.0853 | 0.0838 | 0.0823 |
| -1.2 | 0.1151 | 0.1131 | 0.1112 | 0.1093 | 0.1075 | 0.1056 | 0.1038 | 0.1020 | 0.1003 | 0.0985 |
| -1.1 | 0.1357 | 0.1335 | 0.1314 | 0.1292 | 0.1271 | 0.1251 | 0.1230 | 0.1210 | 0.1190 | 0.1170 |
| -1.0 | 0.1587 | 0.1562 | 0.1539 | 0.1515 | 0.1492 | 0.1469 | 0.1446 | 0.1423 | 0.1401 | 0.1379 |
| -0.9 | 0.1841 | 0.1814 | 0.1788 | 0.1762 | 0.1736 | 0.1711 | 0.1685 | 0.1660 | 0.1635 | 0.1611 |
| -0.8 | 0.2119 | 0.2090 | 0.2061 | 0.2033 | 0.2005 | 0.1977 | 0.1949 | 0.1922 | 0.1894 | 0.1867 |
| -0.7 | 0.2420 | 0.2389 | 0.2358 | 0.2327 | 0.2296 | 0.2266 | 0.2236 | 0.2206 | 0.2177 | 0.2148 |
| -0.6 | 0.2743 | 0.2709 | 0.2676 | 0.2643 | 0.2611 | 0.2578 | 0.2546 | 0.2514 | 0.2483 | 0.2451 |
| -0.5 | 0.3085 | 0.3050 | 0.3015 | 0.2981 | 0.2946 | 0.2912 | 0.2877 | 0.2843 | 0.2810 | 0.2776 |
| -0.4 | 0.3446 | 0.3409 | 0.3372 | 0.3336 | 0.3300 | 0.3264 | 0.3228 | 0.3192 | 0.3156 | 0.3121 |
| -0.3 | 0.3821 | 0.3783 | 0.3745 | 0.3707 | 0.3669 | 0.3632 | 0.3594 | 0.3557 | 0.3520 | 0.3483 |
| -0.2 | 0.4207 | 0.4168 | 0.4129 | 0.4090 | 0.4052 | 0.4013 | 0.3974 | 0.3936 | 0.3897 | 0.3859 |
| -0.1 | 0.4602 | 0.4562 | 0.4522 | 0.4483 | 0.4443 | 0.4404 | 0.4364 | 0.4325 | 0.4286 | 0.4247 |
| 0.0 | 0.5000 | 0.4960 | 0.4920 | 0.4880 | 0.4840 | 0.4801 | 0.4761 | 0.4721 | 0.4681 | 0.4641 |
Probability Table for Standard Normal Distribution
Positive z-scores
| z | 0.00 | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 | 0.06 | 0.07 | 0.08 | 0.09 |
| 0.0 | 0.5000 | 0.5040 | 0.5080 | 0.5120 | 0.5160 | 0.5199 | 0.5239 | 0.5279 | 0.5319 | 0.5359 |
| 0.1 | 0.5398 | 0.5438 | 0.5478 | 0.5517 | 0.5557 | 0.5596 | 0.5636 | 0.5675 | 0.5714 | 0.5753 |
| 0.2 | 0.5793 | 0.5832 | 0.5871 | 0.5910 | 0.5948 | 0.5987 | 0.6026 | 0.6064 | 0.6103 | 0.6141 |
| 0.3 | 0.6179 | 0.6217 | 0.6255 | 0.6293 | 0.6331 | 0.6368 | 0.6406 | 0.6443 | 0.6480 | 0.6517 |
| 0.4 | 0.6554 | 0.6591 | 0.6628 | 0.6664 | 0.6700 | 0.6736 | 0.6772 | 0.6808 | 0.6844 | 0.6879 |
| 0.5 | 0.6915 | 0.6950 | 0.6985 | 0.7019 | 0.7054 | 0.7088 | 0.7123 | 0.7157 | 0.7190 | 0.7224 |
| 0.6 | 0.7257 | 0.7291 | 0.7324 | 0.7357 | 0.7389 | 0.7422 | 0.7454 | 0.7486 | 0.7517 | 0.7549 |
| 0.7 | 0.7580 | 0.7611 | 0.7642 | 0.7673 | 0.7704 | 0.7734 | 0.7764 | 0.7794 | 0.7823 | 0.7852 |
| 0.8 | 0.7881 | 0.7910 | 0.7939 | 0.7967 | 0.7995 | 0.8023 | 0.8051 | 0.8078 | 0.8106 | 0.8133 |
| 0.9 | 0.8159 | 0.8186 | 0.8212 | 0.8238 | 0.8264 | 0.8289 | 0.8315 | 0.8340 | 0.8365 | 0.8389 |
| 1.0 | 0.8413 | 0.8438 | 0.8461 | 0.8485 | 0.8508 | 0.8531 | 0.8554 | 0.8577 | 0.8599 | 0.8621 |
| 1.1 | 0.8643 | 0.8665 | 0.8686 | 0.8708 | 0.8729 | 0.8749 | 0.8770 | 0.8790 | 0.8810 | 0.8830 |
| 1.2 | 0.8849 | 0.8869 | 0.8888 | 0.8907 | 0.8925 | 0.8944 | 0.8962 | 0.8980 | 0.8997 | 0.9015 |
| 1.3 | 0.9032 | 0.9049 | 0.9066 | 0.9082 | 0.9099 | 0.9115 | 0.9131 | 0.9147 | 0.9162 | 0.9177 |
| 1.4 | 0.9192 | 0.9207 | 0.9222 | 0.9236 | 0.9251 | 0.9265 | 0.9279 | 0.9292 | 0.9306 | 0.9319 |
| 1.5 | 0.9332 | 0.9345 | 0.9357 | 0.9370 | 0.9382 | 0.9394 | 0.9406 | 0.9418 | 0.9429 | 0.9441 |
| 1.6 | 0.9452 | 0.9463 | 0.9474 | 0.9484 | 0.9495 | 0.9505 | 0.9515 | 0.9525 | 0.9535 | 0.9545 |
| 1.7 | 0.9554 | 0.9564 | 0.9573 | 0.9582 | 0.9591 | 0.9599 | 0.9608 | 0.9616 | 0.9625 | 0.9633 |
| 1.8 | 0.9641 | 0.9649 | 0.9656 | 0.9664 | 0.9671 | 0.9678 | 0.9686 | 0.9693 | 0.9699 | 0.9706 |
| 1.9 | 0.9713 | 0.9719 | 0.9726 | 0.9732 | 0.9738 | 0.9744 | 0.9750 | 0.9756 | 0.9761 | 0.9767 |
| 2.0 | 0.9772 | 0.9778 | 0.9783 | 0.9788 | 0.9793 | 0.9798 | 0.9803 | 0.9808 | 0.9812 | 0.9817 |
| 2.1 | 0.9821 | 0.9826 | 0.9830 | 0.9834 | 0.9838 | 0.9842 | 0.9846 | 0.9850 | 0.9854 | 0.9857 |
| 2.2 | 0.9861 | 0.9864 | 0.9868 | 0.9871 | 0.9875 | 0.9878 | 0.9881 | 0.9884 | 0.9887 | 0.9890 |
| 2.3 | 0.9893 | 0.9896 | 0.9898 | 0.9901 | 0.9904 | 0.9906 | 0.9909 | 0.9911 | 0.9913 | 0.9916 |
| 2.4 | 0.9918 | 0.9920 | 0.9922 | 0.9925 | 0.9927 | 0.9929 | 0.9931 | 0.9932 | 0.9934 | 0.9936 |
| 2.5 | 0.9938 | 0.9940 | 0.9941 | 0.9943 | 0.9945 | 0.9946 | 0.9948 | 0.9949 | 0.9951 | 0.9952 |
| 2.6 | 0.9953 | 0.9955 | 0.9956 | 0.9957 | 0.9959 | 0.9960 | 0.9961 | 0.9962 | 0.9963 | 0.9964 |
| 2.7 | 0.9965 | 0.9966 | 0.9967 | 0.9968 | 0.9969 | 0.9970 | 0.9971 | 0.9972 | 0.9973 | 0.9974 |
| 2.8 | 0.9974 | 0.9975 | 0.9976 | 0.9977 | 0.9977 | 0.9978 | 0.9979 | 0.9979 | 0.9980 | 0.9981 |
| 2.9 | 0.9981 | 0.9982 | 0.9982 | 0.9983 | 0.9984 | 0.9984 | 0.9985 | 0.9985 | 0.9986 | 0.9986 |
| 3.0 | 0.9987 | 0.9987 | 0.9987 | 0.9988 | 0.9988 | 0.9989 | 0.9989 | 0.9989 | 0.9990 | 0.9990 |
| 3.1 | 0.9990 | 0.9991 | 0.9991 | 0.9991 | 0.9992 | 0.9992 | 0.9992 | 0.9992 | 0.9993 | 0.9993 |
| 3.2 | 0.9993 | 0.9993 | 0.9994 | 0.9994 | 0.9994 | 0.9994 | 0.9994 | 0.9995 | 0.9995 | 0.9995 |
| 3.3 | 0.9995 | 0.9995 | 0.9995 | 0.9996 | 0.9996 | 0.9996 | 0.9996 | 0.9996 | 0.9996 | 0.9997 |
| 3.4 | 0.9997 | 0.9997 | 0.9997 | 0.9997 | 0.9997 | 0.9997 | 0.9997 | 0.9997 | 0.9997 | 0.9998 |
Table 3 shows the yearly average traffic congestion levels in two cities, Melbourne and Sydney, during the period 2008 to 2016. Also shown is a time series plot of the same data.
The time series plot for Melbourne is incomplete.
--- 0 WORK AREA LINES (style=lined) ---
--- 0 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
iii. Use the least squares line to predict when the percentage congestion level in Sydney will be 43%. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
The yearly average traffic congestion level data for Melbourne is repeated in Table 4 below.
--- 1 WORK AREA LINES (style=lined) ---
--- 0 WORK AREA LINES (style=lined) ---
| congestion level = |
|
+ |
|
× year |
Explain why, quoting the values of appropriate statistics. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
The data in Table 1 relates to the impact of traffic congestion in 2016 on travel times in 23 cities in the United Kingdom (UK).
The four variables in this data set are:
--- 1 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
--- 0 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
Traffic congestion can lead to an increase in travel times in cities. The dot plot and boxplot below both show the increase in travel time due to traffic congestion, in minutes per day, for the 23 UK cities.
--- 1 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
The points
The line
The
The point
i.
ii.
| `= M_(RS)= ((at)/2, (−at^2)/2)“ |
Let
State the values of
--- 5 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
The functions of
--- 3 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
ii. Describe the transformation that maps the graph of
--- 3 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
a.
b.
c.
d.
e.
f.
g.i.
g.ii.
h.
i.i.
i.ii.
a.
b.
c.
| d. | ||
e.
f.
| g.i. | ||
| g.ii. | ||
h.
i.i
i.ii
Sammy visits a giant Ferris wheel. Sammy enters a capsule on the Ferris wheel from a platform above the ground. The Ferris wheel is rotating anticlockwise. The capsule is attached to the Ferris wheel at point
Sammy exits the capsule after one complete rotation of the Ferris wheel.
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
As the Ferris wheel rotates, a stationary boat at
--- 3 WORK AREA LINES (style=lined) ---
Part of the path of
--- 2 WORK AREA LINES (style=lined) ---
As the Ferris wheel continues to rotate, the boat at
--- 10 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
| a. | ||||
b.
c.
| |
|
| d. |
| e. |
| f. |
| g. | ||
| h. |
A permutation matrix,
Matrix
| A. |
|
B. |
|
| C. |
|
D. |
|
| E. |
|
The shaded area in the graph below shows the feasible region for a linear programming problem.
The objective function is given by
Which one of the following statements is not true?
The annual fee for membership of a car club, in dollars, based on years of membership of the club is shown in the step graph below.
In the Martin family:
• Hayley has been a member of the club for four years
• John has been a member of the club for 20 years
• Sharon has been a member of the club for 25 years.
What is the total fee for membership of the car club for the Martin family?
The graph below shows the volume of water in a water tank between 7 am and 5 pm on one day.
Which one of the following statements is true?
Consider the following graph.
The adjacency matrix for this graph, with some elements missing, is shown below.
This adjacency matrix contains 16 elements when complete.
Of the 12 missing elements
Which one of the following graphs contains a loop?
| A. | B. |
| C. | D. |
| E. | |
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---

--- 5 WORK AREA LINES (style=lined) ---

--- 5 WORK AREA LINES (style=lined) ---

The point
Find the exact values of
--- 6 WORK AREA LINES (style=lined) ---
Let
--- 7 WORK AREA LINES (style=lined) ---
--- 7 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
Find the values of
--- 5 WORK AREA LINES (style=lined) ---

Let
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
A travel company is studying the choice between air (
Matrix
Let
Matrix
Matrix
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
In 2016, the number of customers studied was increased to 1360.
Matrix
--- 2 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
a.
b.
c.
d.
e.i.
| e.ii. | ||
Part 1
The histogram below shows the distribution of the number of billionaires per million people for 53 countries.
Using this histogram, the percentage of these 53 countries with less than two billionaires per million people is closest to
Part 2
The histogram below shows the distribution of the number of billionaires per million people for the same 53 countries as in Part 1, but this time plotted on a
Based on this histogram, the number of countries with one or more billionaires per million people is
10 000 trout eggs, 1000 baby trout and 800 adult trout are placed in a pond to establish a trout population.
In establishing this population
From year to year, this situation can be represented by the transition matrix
The initial state matrix for this trout population,
Let
--- 3 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 3 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
The rule
--- 4 WORK AREA LINES (style=lined) ---
--- 6 WORK AREA LINES (style=lined) ---
a.i.
a.ii.
b.i.
b.ii.
c.i.
c.ii.
d.
e.i.
e.ii.
A school has three computer classes, A, B and C. There are 15 students in each class.
Each student is given a mark out of 100 based on their performance in a test.
Matrix
Two other matrices,
Which one of the following matrix expressions can be used to generate a matrix that displays the mean mark obtained for each class?
A.
B.
C.
D.
E.
| |
|
|
|
|
| |
|
The diagram shows a triangular piece of land
The owner of the land wants to build a straight fence to divide the land into two pieces of equal area. Let
Let
--- 2 WORK AREA LINES (style=lined) ---
--- 5 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
(You may assume that the value of
--- 5 WORK AREA LINES (style=lined) ---
| i. | ||
ii.
| |
| iii. | ||
| |
iv.
A rectangular playing surface is to be constructed so that the length is 6 metres more than the width.
--- 2 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
A graph comparing the area of the playing surface to its length is shown.

--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Company

Use the horizontal axis to represent the area and the vertical axis to represent the cost. (2 marks)
--- 8 WORK AREA LINES (style=lined) ---
Justify your answer with suitable calculations. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
The dot plot below shows the distribution of the time, in minutes, that 50 people spent waiting to get help from a call centre.
Which one of the following boxplots best represents the data?
The following graph indicates
--- 2 WORK AREA LINES (style=lined) ---
(1) If 2.5% of girls of the same age are taller than Rachel, how tall is she? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
(2) Rachel does not grow any taller. At age 15 ½, what percentage of girls of the same age will be taller than Rachel? (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
--- 1 WORK AREA LINES (style=lined) ---
For adults (18 years and older), the Body Mass Index is given by
The medically accepted healthy range for
--- 4 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
(2) Give ONE reason why this equation is not suitable for predicting heights of girls older than 12. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
(2)
(2)
| i. |
| ii. | (1) | |
| (2) | ||
| iii. | |
| iv. | |
| v. | (1) | |
| (2) | ||
An aerial diagram of a swimming pool is shown.
The swimming pool is a standard length of 50 metres but is not in the shape of a rectangle.
(i) Given
1 cm =
(ii) If the length of a carpark next to the pool measured 5 cm (not shown), how long would it be in real life? (1 mark)
(iii) In the diagram of the swimming pool, the five widths are measured to be:
The average depth of the pool is 1.2 m
Calculate the approximate volume of the swimming pool, in cubic metres. In your calculations, use TWO applications of Simpson’s Rule. (3 marks)
(i)
(ii)
(iii)
Part of the floor plan of a house is shown. The plan is drawn to scale.
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
| i. | |
| ii. | |
| iii. | |
| |
|
Moivre’s manufacturing company produces cans of Magic Beans. The can has a diameter of 10 cm and a height of 10 cm.
What is the maximum number of cans that can be packed into one of these boxes? (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
--- 4 WORK AREA LINES (style=lined) ---
Is Monica correct? Justify your answer with suitable calculations. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
The company wants to produce a can with a volume of 1570 cm³, using the least amount of metal. Monica is given the job of determining the dimensions of the can to be produced. She considers the following graphs.
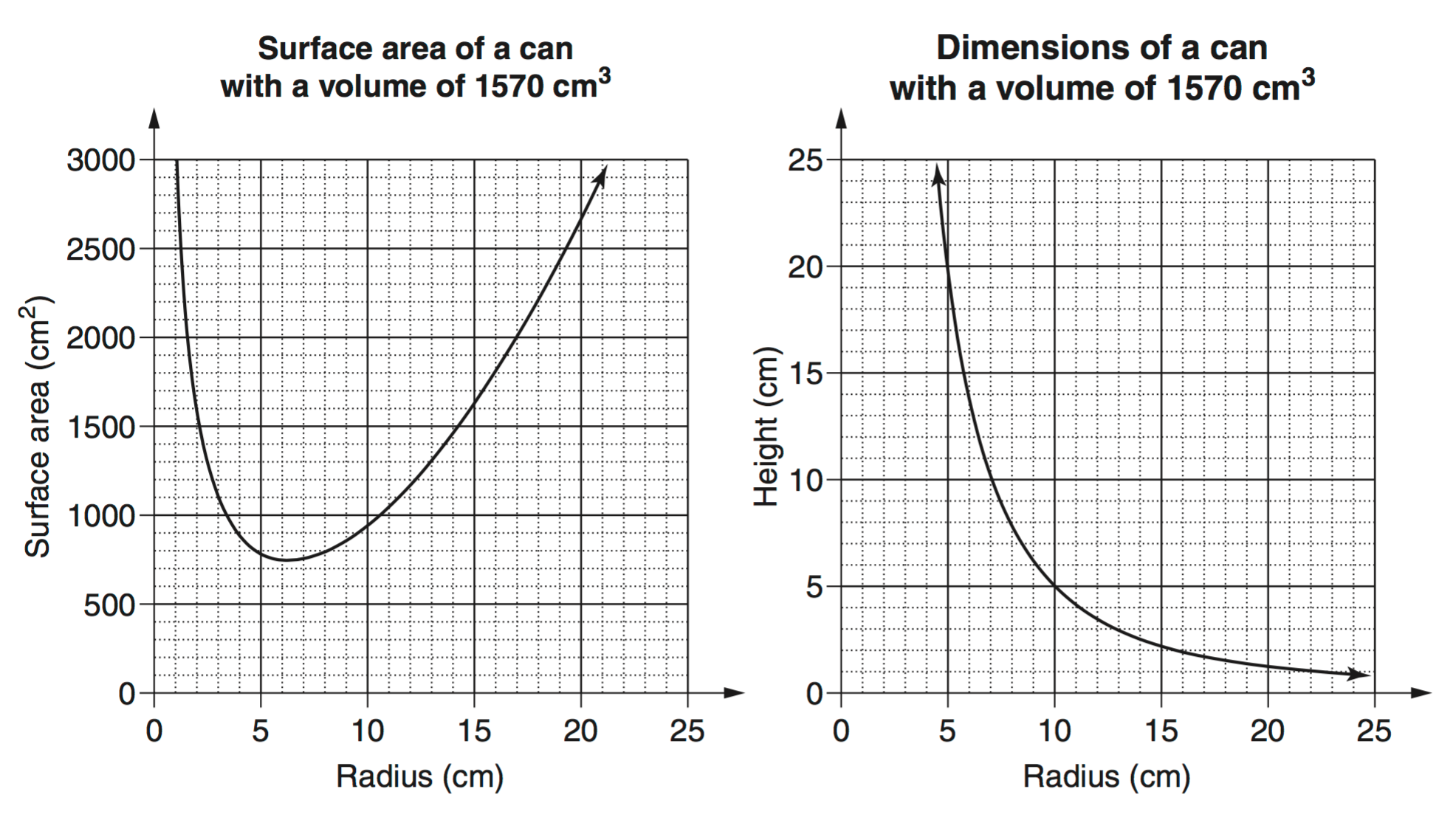
--- 5 WORK AREA LINES (style=lined) ---
| i. | ||
| ii. | ||
| iii. | ||
| iv. |  |
The table shows monthly home loan repayments with interest rate changes from February to October 2009.
--- 3 WORK AREA LINES (style=lined) ---
Xiang’s bank approves loans for customers if their loan repayments are no more than 30% of their monthly gross salary.
Xiang’s monthly gross salary is $6500.
If she had applied for the loan in October 2009, would her bank have approved her loan?
Justify your answer with suitable calculations. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
Graphs of their loan balances are shown.

Identify TWO differences between the graphs and provide a possible explanation for each difference, making reference to interest rates and/or loan repayments. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
| i. | ||
| ii. |
iii.
A new shopping centre has opened near a primary school. A survey is conducted to determine the number of motor vehicles that pass the school each afternoon between 2.30 pm and 4.00 pm.
The results for 60 days have been recorded in the table and are displayed in the cumulative frequency histogram.
--- 1 WORK AREA LINES (style=lined) ---
What problem could arise from the change in the median number of motor vehicles passing the school before and after the opening of the new shopping centre?
Briefly recommend a solution to this problem. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
| i. | ||
| ii. |  |
iii.
| iv. | |
Wind turbines, such as those shown, are used to generate power.
In theory, the power that could be generated by a wind turbine is modelled using the equation
| where | |
--- 1 WORK AREA LINES (style=lined) ---
In practice, the actual power generated by a wind turbine is only 40% of the theoretical power.
--- 2 WORK AREA LINES (style=lined) ---
The graph shows both the theoretical power generated and the actual power generated by a particular wind turbine.
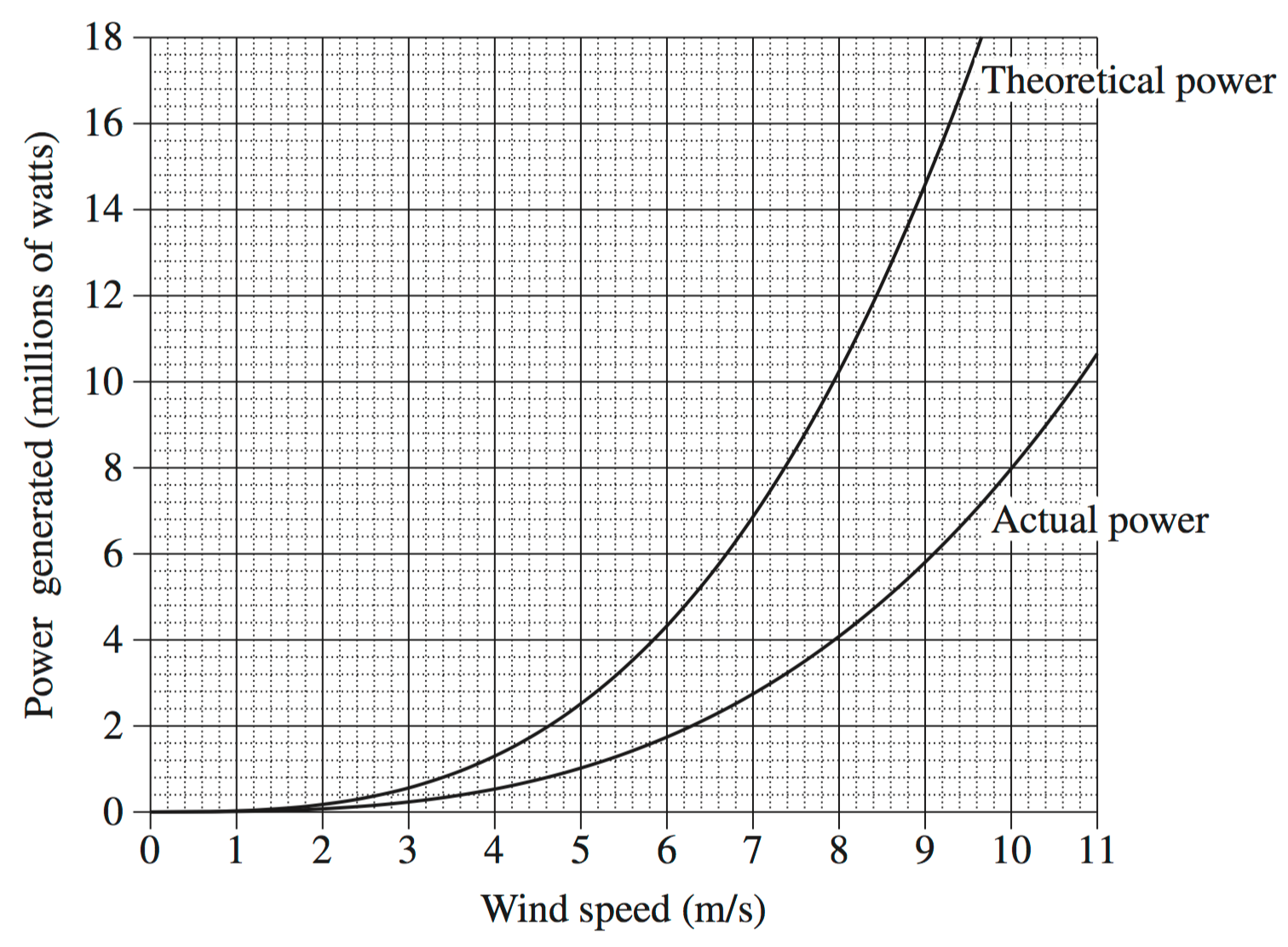
--- 1 WORK AREA LINES (style=lined) ---
A particular farm requires at least 4.4 million watts of actual power in order to be self-sufficient.
--- 1 WORK AREA LINES (style=lined) ---
A more accurate formula to calculate the power (
| where | |
Each blade of a particular wind turbine has a length of 43 metres.The turbine operates at a wind speed of 8 m/s.
--- 6 WORK AREA LINES (style=lined) ---
| i. | |
| ii. |
| iii. | |
| iv. |
| v. |
A golf ball is hit from point
The path of the golf ball is modelled using the equation
where
The graph of this equation is drawn below.
--- 1 WORK AREA LINES (style=lined) ---
What horizontal distance does the ball travel in the period between these two occasions? (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
--- 2 WORK AREA LINES (style=lined) ---
Find all values of
--- 4 WORK AREA LINES (style=lined) ---
i.
ii.
iii.
iv.