CHEMISTRY, M4 EQ-Bank 4
The chemical equation for the combustion of butane
Given that the standard enthalpy of formation of
--- 6 WORK AREA LINES (style=lined) ---
CHEMISTRY, M4 EQ-Bank 1
The chemical equation for the combustion of butanol
- Define what the term 'standard enthalpy of formation' means. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Use the table data to calculate the standard enthalpy of formation of butanol. (3 marks)
--- 6 WORK AREA LINES (style=blank) ---
CHEMISTRY, M4 EQ-Bank 17
The decomposition of a metal carbonate is represented by the following equation:
The following data was recorded:
- Calculate the Gibbs free energy at 350 K. (2 marks)
--- 4 WORK AREA LINES (style=blank) ---
- Determine if the reaction is spontaneous at this temperature. (1 mark)
--- 2 WORK AREA LINES (style=blank) ---
- Discuss how both enthalpy and entropy influence the spontaneity of this reaction and predict the temperature range in which the reaction will be spontaneous. (4 marks)
--- 8 WORK AREA LINES (style=blank) ---
v1 Algebra, STD2 A2 2009 HSC 24d
A factory makes both cloth and leather lounges. In any week
• the total number of cloth lounges and leather lounges that are made is 400
• the maximum number of leather lounges made is 270
• the maximum number of cloth lounges made is 325.
The factory manager has drawn a graph to show the numbers of leather lounges (
- Find the equation of the line
. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Explain why this line is only relevant between
and for this factory. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- The profit per week,
, can be found by using the equation . Compare the profits at
and . (2 marks)
--- 6 WORK AREA LINES (style=lined) ---
v1 Algebra, STD2 A2 2010 HSC 27c
The graph shows tax payable against taxable income, in thousands of dollars.
- Use the graph to find the tax payable on a taxable income of
. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- Use suitable points from the graph to show that the gradient of the section of the graph marked
is . (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- How much of each dollar earned between
and is payable in tax? Give your answer correct to the nearest whole number. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Write an equation that could be used to calculate the tax payable,
, in terms of the taxable income, , for taxable incomes between and . (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
v1 Algebra, STD2 A2 2007 HSC 18 MC
v1 Algebra, STD2 A1 2020 HSC 13 MC
When Stuart stops drinking alcohol at 11:30 pm, he has a blood alcohol content (BAC) of 0.08625.
The number of hours required for a person to reach zero BAC after they stop consuming alcohol is given by the formula:
At what time on the next day should Stuart expect his BAC to be 0.05?
- 1:33 am
- 1:55 am
- 2:15 am
- 5:15 am
v1 Algebra, STD2 A1 2007 HSC 28b
EXAMCOPY Functions, MET2 2022 VCAA 4
Consider the function
Part of the graph of
- State the range of
. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- i. Find
. (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
- ii. State the maximal domain over which
is strictly increasing. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Show that
. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- Find the domain and the rule of
, the inverse of . (3 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Let
be the function , where and . - The inverse function of
is defined by . - The area of the regions bound by the functions
and can be expressed as a function, . - The graph below shows the relevant area shaded.
- You are not required to find or define
.
- Determine the range of values of
such that . (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Explain why the domain of
does not include all values of . (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
CHEMISTRY, M8 2022 VCE 5*
A chemist uses spectroscopy to identify an unknown organic molecule, Molecule
The
The infra-red (IR) spectrum of Molecule
- Name the functional group that produces the peak at 168 ppm in the
spectrum on the first image, which is consistent with the IR spectrum shown above. Justify your answer with reference to the IR spectrum. (2 marks)
--- 2 WORK AREA LINES (style=lined) ---
The mass spectrum of Molecule
- The molecular mass of Molecule
is 108.5 - Explain the presence of the peak at 110 m/z. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
The
- The
spectrum consists of two singlet peaks. - What information does this give about the molecule? (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
- Draw a structural formula for Molecule
that is consistent with the information provided in parts a–c. (2 marks)
--- 5 WORK AREA LINES (style=blank) ---
CHEMISTRY, M8 2021 VCE 16 MC
Which one of the following statements about IR spectroscopy is correct?
- IR radiation changes the spin state of electrons.
- Bond wave number is influenced only by bond strength.
- An IR spectrum can be used to determine the purity of a sample.
- In an IR spectrum, high transmittance corresponds to high absorption.
CHEMISTRY, M8 2023 VCE 7-2*
The infrared (IR) spectrum of the molecule 3-methyl-2-butanone is shown below.
Explain why different frequencies of infrared radiation can be absorbed by the same molecule as shown in the spectrum above. (3 marks)
--- 7 WORK AREA LINES (style=lined) ---
CHEMISTRY, M8 2013 VCE 2
The strength of the eggshell of birds is determined by the calcium carbonate,
The percentage of calcium carbonate in the eggshell can be determined by gravimetric analysis.
0.412 g of clean, dry eggshell was completely dissolved in a minimum volume of dilute hydrochloric acid.
An excess of a basic solution of ammonium oxalate,
The suspension was filtered and the crystals were then dried to constant mass.
0.523 g of
- Write a balanced equation for the formation of the calcium oxalate monohydrate precipitate. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- Determine the percentage, by mass, of calcium carbonate in the eggshell. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
CHEMISTRY, M7 2018 VCE 1a
Organic compounds are numerous and diverse due to the nature of the carbon atom. There are international conventions for the naming and representation of organic compounds.
- Draw the structural formula of 2-methyl-propan-2-ol. (1 mark)
--- 5 WORK AREA LINES (style=lined) ---
- Give the molecular formula of but-2-yne. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- Give the IUPAC name of the compound that has the structural formula shown above. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
CHEMISTRY, M8 2016 VCE 6
Brass is an alloy of copper and zinc.
To determine the percentage of copper in a particular sample of brass, an analyst prepared a number of standard solutions of copper
The calibration curve obtained is shown below.
- A 0.198 g sample of the brass was dissolved in acid and the solution was made up to 100.00 mL in a volumetric flask. The absorbance of this test solution was found to be 0.13
- Calculate the percentage by mass of copper in the brass sample. (3 marks)
--- 5 WORK AREA LINES (style=lined) ---
- If the analyst had made up the solution of the brass sample to 20.00 mL instead of 100.00 mL, would the result of the analysis have been equally reliable? Why? (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
- Name another analytical technique that could be used to verify the result from part a. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
PHYSICS, M8 2019 VCE 17
Students are comparing the diffraction patterns produced by electrons and X-rays, in which the same spacing of bands is observed in the patterns, as shown schematically in Figure 18. Note that both patterns shown are to the same scale.
The electron diffraction pattern is produced by 3.0 × 10
- Explain why electrons can produce the same spacing of bands in a diffraction pattern as X-rays. (3 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Calculate the frequency of X-rays that would produce the same spacing of bands in a diffraction pattern as for the electrons. Show your working. (4 marks)
--- 6 WORK AREA LINES (style=lined) ---
PHYSICS, M5 2019 VCE 8
A 250 g toy car performs a loop in the apparatus shown in Figure 8.
The car starts from rest at point
- Calculate the value of
. Show your working. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
- Calculate the magnitude of the normal reaction force on the car by the track when it is at point
. Show your working. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
- Explain why the car does not fall from the track at point
, when it is upside down. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
PHYSICS, M6 2019 VCE 6
A home owner on a large property creates a backyard entertainment area. The entertainment area has a low-voltage lighting system. To operate correctly, the lighting system requires a voltage of 12 V. The lighting system has a resistance of 12
- Calculate the power drawn by the lighting system. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
To operate the lighting system, the home owner installs an ideal transformer at the house to reduce the voltage from 240 V to 12 V. The home owner then runs a 200 m long heavy-duty outdoor extension lead, which has a total resistance of 3
- The lights are a little dimmer than expected in the entertainment area.
- Give one possible reason for this and support your answer with calculations. (4 marks)
--- 7 WORK AREA LINES (style=lined) ---
- Using the same equipment, what changes could the home owner make to improve the brightness of the lights? Explain your answer. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
PHYSICS, M6 2019 VCE 1
A particle of mass
- Is the charge
positive or negative? Give a reason for your answer. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- Explain why the path of the particle is an arc of a circle while the particle is in the magnetic field. (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
PHYSICS, M7 2020 VCE 11
An astronaut has left Earth and is travelling on a spaceship at 0.800
- How long will the trip take according to a clock that the astronaut is carrying on his spaceship? Show your working. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Is the trip time measured by the astronaut in part a. a proper time? Explain your reasoning. (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
PHYSICS, M6 2020 VCE 6
Two Physics students hold a coil of wire in a constant uniform magnetic field, as shown in Figure 5a. The ends of the wire are connected to a sensitive ammeter. The students then change the shape of the coil by pulling each side of the coil in the horizontal direction, as shown in Figure 5b. They notice a current register on the ammeter.
- Will the magnetic flux through the coil increase, decrease or stay the same as the students change the shape of the coil? (1 mark)
--- 2 WORK AREA LINES (style=blank) ---
- Explain, using physics principles, why the ammeter registered a current in the coil and determine the direction of the induced current. (3 marks
--- 6 WORK AREA LINES (style=lined) ---
- The students then push each side of the coil together, as shown in Figure 6a, so that the coil returns to its original circular shape, as shown in Figure 6b, and then changes to the shape shown in Figure 6c.
- Describe the direction of any induced currents in the coil during these changes. Give your reasoning. (2 marks)
--- 6 WORK AREA LINES (style=lined) ---
PHYSICS, M5 2020 VCE 4*
The Ionospheric Connection Explorer (ICON) space weather satellite, constructed to study Earth's ionosphere, was launched in October 2019. ICON will study the link between space weather and Earth's weather at its orbital altitude of 600 km above Earth's surface. Assume that ICON's orbit is a circular orbit.
- Calculate the orbital radius of the ICON satellite. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Calculate the orbital period of the ICON satellite correct to three significant figures. Show your working. (4 marks)
--- 8 WORK AREA LINES (style=lined) ---
- Explain how the ICON satellite maintains a stable circular orbit without the use of propulsion engines. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
PHYSICS, M6 2020 VCE 3
Electron microscopes use a high-precision electron velocity selector consisting of an electric field,
Electrons travelling at the required velocity,
- Show that the velocity of an electron that travels straight through the aperture to point
is given by = . (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Calculate the magnitude of the velocity,
, of an electron that travels straight through the aperture to point if = 500 kV m and = 0.25 T. Show your working. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- i. At which of the points –
, or – in Figure 2 could electrons travelling faster than arrive? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- ii. Explain your answer to part c.i. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
CHEMISTRY, M3 EQ-Bank 12
A student stirs 2.80 g of silver
- Write a balanced chemical equation for the reaction. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Calculate the theoretical mass of precipitate that will be formed. (3 marks)
--- 8 WORK AREA LINES (style=lined) ---
The student weighed a piece of filter paper, filtered out the precipitate and dried it thoroughly in an incubator. The final precipitate mass was higher than predicted in (b).
- Identify one scientific reason why the precipitate mass was too high and suggest an improvement to the experimental method which would eliminate this error. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
CHEMISTRY, M3 EQ-Bank 11
A student stirs 2.45 g of copper
- Write a balanced chemical equation for the reaction. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- Calculate the theoretical mass of precipitate that will be formed. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
The student weighed a piece of filter paper, filtered out the precipitate and dried it thoroughly in an incubator. The final precipitate mass was higher than predicted in (b).
- Identify one scientific reason why the precipitate mass was too high and suggest an improvement to the experimental method which would eliminate this error. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
Calculus, MET2 2023 VCE SM-Bank 1
The function
- Sketch the graph of
on the axes below. Label the vertical asymptote with its equation, and label any axial intercepts, stationary points and endpoints in coordinate form, correct to three decimal places. (3 marks)
--- 0 WORK AREA LINES (style=lined) ---
- i. Find the equation of the tangent to the graph of
at the point where . (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- ii. Sketch the graph of the tangent to the graph of
at on the axes in part a. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
Newton's method is used to find an approximate
- Find the value of
. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- Find the horizontal distance between
and the closest -intercept of , correct to four decimal places. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- i. Find the value of
, where , such that an initial estimate of gives the same value of as found in part . Give your answer correct to three decimal places. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- ii. Using this value of
, sketch the tangent to the graph of at the point where on the axes in part a. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
CHEMISTRY, M2 EQ-Bank 2
During a laboratory experiment, a gas is collected in a sealed syringe. Initially, the gas has a volume of 5.0 litres and a pressure of 1.0 atmosphere.
- Calculate the new pressure inside the syringe when the volume is decreased to 3.0 litres, assuming no temperature change. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- After reaching the pressure calculated in part a, the volume is further decreased so that the pressure inside the syringe doubles. Calculate the final volume of the gas. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Discuss two potential experimental errors that could affect the accuracy of the observed results compared to the theoretical predictions of Boyle's Law. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
CHEMISTRY, M1 EQ-Bank 33
Explain why the boiling points of hydrogen halides
Include in your answer the types of intermolecular forces involved and how molecular mass affects these boiling points. (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
Calculus, SPEC2 2022 VCAA 3
A particle moves in a straight line so that its distance,
- i. Express the differential equation in the form
. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- ii. Hence, show that
. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- The graph of
has a horizontal asymptote.
-
- Write down the equation of this asymptote. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- Sketch the graph of
and the horizontal asymptote on the axes below. Using coordinates, plot and label the point where , giving the value of correct to two decimal places. (2 marks) --- 3 WORK AREA LINES (style=lined) ---
- Write down the equation of this asymptote. (1 mark)
- Find the speed of the particle when
. Give your answer in metres per second, correct to two decimal places. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
Two seconds after the first particle passed through
Its distance
- Verify that the particles are the same distance from
when . (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- Find the ratio of the speed of the first particle to the speed of the second particle when the particles are at the same distance from
. Give your answer as in simplest form, where and are positive integers. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
Calculus, MET2 2022 VCAA 5
Consider the composite function
Use the following table of values for
- Find the value of
. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
The derivative of
- Show that
. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Find the equation of the tangent to
at . (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Find the average value of the derivative function
between and . (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
- Find four solutions to the equation
for the interval . (3 marks)
--- 8 WORK AREA LINES (style=lined) ---
Functions, MET2 2022 VCAA 4
Consider the function
Part of the graph of
- State the range of
. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- i. Find
. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- ii. State the maximal domain over which
is strictly increasing. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- Show that
. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- Find the domain and the rule of
, the inverse of . (3 marks)
--- 6 WORK AREA LINES (style=lined) ---
- Let
be the function , where and . - The inverse function of
is defined by . - The area of the regions bound by the functions
and can be expressed as a function, . - The graph below shows the relevant area shaded.
- You are not required to find or define
. - i. Determine the range of values of
such that . (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- ii. Explain why the domain of
does not include all values of . (1 mark
--- 2 WORK AREA LINES (style=lined) ---
Probability, MET2 2022 VCAA 3
Mika is flipping a coin. The unbiased coin has a probability of
Let
Mika flips the coin five times.
-
- Find
. (1 mark) --- 2 WORK AREA LINES (style=lined) ---
- Find
(1 mark) --- 2 WORK AREA LINES (style=lined) ---
- Find
, correct to three decimal places. (2 marks) --- 4 WORK AREA LINES (style=lined) ---
- Find the expected value and the standard deviation for
. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Find
The height reached by each of Mika's coin flips is given by a continuous random variable,
where
-
- State the value of the definite integral
--- 5 WORK AREA LINES (style=lined) ---
- Given that
--- 5 WORK AREA LINES (style=lined) ---
-
The ceiling of Mika's room is 3 m above the floor. The minimum distance between the coin and the ceiling is a continuous random variable,
- The function
- Find the values of
--- 5 WORK AREA LINES (style=lined) ---
- State the value of the definite integral
- Mika's sister Bella also has a coin. On each flip, Bella's coin has a probability of
- Bella flips her coin 25 times in order to estimate
- Let
- Is the random variable
--- 2 WORK AREA LINES (style=lined) ---
- If
--- 6 WORK AREA LINES (style=lined) ---
- Bella knows that she can decrease the width of a 95% confidence interval by using a larger sample of coin flips.
- If
--- 2 WORK AREA LINES (style=lined) ---
- Is the random variable
Calculus, SPEC2 2022 VCAA 14 MC
A particle moving in a straight line with constant acceleration has a velocity of 7 ms
The velocity of the particle, in metres per second, at the midpoint of
PHYSICS, M6 2020 VCE 7 MC
An ideal transformer has an input DC voltage of 240 V, 2000 turns in the primary coil and 80 turns in the secondary coil.
The output voltage is closest to
- 0 V
- 9.6 V
- 6.0 × 10
- 3.8 × 10
Calculus, MET2 2022 VCAA 2
On a remote island, there are only two species of animals: foxes and rabbits. The foxes are the predators and the rabbits are their prey.
The populations of foxes and rabbits increase and decrease in a periodic pattern, with the period of both populations being the same, as shown in the graph below, for all
One point of minimum fox population, (20, 700), and one point of maximum fox population, (100, 2500), are also shown on the graph.
The graph has been drawn to scale.
The population of rabbits can be modelled by the rule
- i. State the initial population of rabbits. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- ii. State the minimum and maximum population of rabbits. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- iii. State the number of weeks between maximum populations of rabbits. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
The population of foxes can be modelled by the rule
- Show that
--- 4 WORK AREA LINES (style=lined) ---
- Find the maximum combined population of foxes and rabbits. Give your answer correct to the nearest whole number. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- What is the number of weeks between the periods when the combined population of foxes and rabbits is a maximum? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
The population of foxes is better modelled by the transformation of
- Find the average population during the first 300 weeks for the combined population of foxes and rabbits, where the population of foxes is modelled by the transformation of
--- 8 WORK AREA LINES (style=lined) ---
Over a longer period of time, it is found that the increase and decrease in the population of rabbits gets smaller and smaller.
The population of rabbits over a longer period of time can be modelled by the rule
- Find the average rate of change between the first two times when the population of rabbits is at a maximum. Give your answer correct to one decimal place. (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
- Find the time, where
--- 3 WORK AREA LINES (style=lined) ---
- Over time, the rabbit population approaches a particular value.
- State this value. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
Calculus, MET2 2022 VCAA 1
The diagram below shows part of the graph of
- State the equation of the axis of symmetry of the graph of
--- 2 WORK AREA LINES (style=lined) ---
- State the derivative of
--- 2 WORK AREA LINES (style=lined) ---
The tangent to
- Find the equation of the tangent to
--- 6 WORK AREA LINES (style=lined) ---
The diagram below shows part of the graph of
- i. Find the equation of the line perpendicular to the tangent passing through point
--- 5 WORK AREA LINES (style=lined) ---
- ii. The line perpendicular to the tangent at point
- Find the area enclosed by this line and the curve
--- 8 WORK AREA LINES (style=lined) ---
- Another parabola is defined by the rule
- A tangent to
- Find the value of
--- 8 WORK AREA LINES (style=lined) ---
Probability, MET2 2022 VCAA 20 MC
A soccer player kicks a ball with an angle of elevation of
The horizontal distance that the ball travels before landing is given by the function
The probability that the ball travels more than 40 m horizontally before landing is closest to
- 0.969
- 0.937
- 0.226
- 0.149
- 0.027
Calculus, SPEC2 2022 VCAA 10 MC
Consider the curve given by
The equation of the tangent to this curve at the point
Calculus, MET2 2023 VCAA 3
- State the value of
--- 1 WORK AREA LINES (style=lined) ---
- The derivative,
- Find the real number
--- 2 WORK AREA LINES (style=lined) ---
-
i. Let
--- 3 WORK AREA LINES (style=lined) ---
--- 8 WORK AREA LINES (style=lined) ---
Let
- Find the coordinates of the point of inflection for
--- 2 WORK AREA LINES (style=lined) ---
- Find the largest interval of
- Give your answer correct to two decimal places. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Apply Newton's method, with an initial estimate of
- Write the estimates
--- 0 WORK AREA LINES (style=lined) ---
- For the function
--- 3 WORK AREA LINES (style=lined) ---
- There is a positive real number
- Find this value of
--- 5 WORK AREA LINES (style=lined) ---
PHYSICS, M6 2021 VCE 5
Figure 5 shows a stationary electron (e
- Explain why the magnetic field does not exert a force on the electron. Justify your answer with an appropriate formula. (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
The switch is now closed.
- Determine the magnitude and the direction of any electric force now acting on the electron. Show your working. (3 marks)
--- 5 WORK AREA LINES (style=lined) ---
-
Ravi and Mia discuss what they think will happen regarding the size and the direction of the magnetic force on the electron after the switch is closed.
Ravi says that there will be a magnetic force of constant magnitude, but it will be continually changing direction.
Mia says that there will be a constantly increasing magnetic force, but it will always be acting in the same direction.
Evaluate these two statements, giving clear reasons for your answer. (4 marks)
--- 8 WORK AREA LINES (style=lined) ---
PHYSICS, M6 2021 VCE 2
A schematic side view of one design of an audio loudspeaker is shown in Figure 2. It uses a current carrying coil that interacts with permanent magnets to create sound by moving a cone in and out.
Figure 3 shows a schematic view of the loudspeaker from the position of the eye shown in Figure 2. The direction of the current is clockwise, as shown.
- Draw four magnetic field lines on Figure 3, showing the direction of each field line using an arrow. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
- Which one of the following gives the direction of the force acting on the current carrying coil shown in Figure 3? (1 mark)
| A. | left | B. | right |
| C. | up the page | D. | down the page |
| E. | into the page | F. | out of the page |
--- 1 WORK AREA LINES (style=lined) ---
- The current carrying coil has a radius of 5.0 cm and 20 turns of wire, and it carries a clockwise current
- Calculate the magnitude of the force,
--- 6 WORK AREA LINES (style=lined) ---
Functions, MET2 2023 VCAA 2
The following diagram represents an observation wheel, with its centre at point
Consider the function
- Show that
--- 5 WORK AREA LINES (style=lined) ---
- Find the average height of a pod on the wheel as it travels from point
- Give your answer in metres, correct to two decimal places. (2 marks)
--- 3 WORK AREA LINES (style=lined) ---
- Find the average rate of change, in metres per minute, of the height of a pod on the wheel as it travels from point
--- 3 WORK AREA LINES (style=lined) ---
After 15 minutes, the wheel stops moving and remains stationary for 5 minutes. After this, it continues moving at double its previous speed for another 7.5 minutes.
The height above the ground of a pod that was initially at point
where
- i.State the values of
--- 2 WORK AREA LINES (style=lined) ---
ii. Find all possible values of--- 4 WORK AREA LINES (style=lined) ---
iii. Sketch the graph of the piecewise function
Calculus, MET2 2023 VCAA 1
Let
- State the coordinates of all axial intercepts of
--- 2 WORK AREA LINES (style=lined) ---
- Find the coordinates of the stationary points of
--- 3 WORK AREA LINES (style=lined) ---
-
- Let
- Find the values of
--- 3 WORK AREA LINES (style=lined) ---
- Let
-
- Write down an expression using definite integrals that gives the area of the regions bound by
--- 4 WORK AREA LINES (style=lined) ---
- Hence, find the total area of the regions bound by
--- 2 WORK AREA LINES (style=lined) ---
- Write down an expression using definite integrals that gives the area of the regions bound by
- Let
- Find the possible values of
--- 9 WORK AREA LINES (style=lined) ---
PHYSICS, M5 2022 VCE 2
There are over 400 geostationary satellites above Earth in circular orbits. The period of orbit is one day (86 400 seconds). Each geostationary satellite remains stationary in relation to a fixed point on the equator. Figure 2 shows an example of a geostationary satellite that is in orbit relative to a fixed point,
- Explain why geostationary satellites must be vertically above the equator to remain stationary relative to Earth's surface. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Using
--- 8 WORK AREA LINES (style=lined) ---
- Calculate the speed of an orbiting geostationary satellite. (3 marks)
--- 7 WORK AREA LINES (style=lined) ---
Complex Numbers, SPEC2 2023 VCAA 2
Let
- Verify that
--- 2 WORK AREA LINES (style=lined) ---
- List the other roots of
--- 2 WORK AREA LINES (style=lined) ---
- On the Argand diagram below, plot and label the points that represent all the roots of
--- 0 WORK AREA LINES (style=blank) ---
- i. On the Argand diagram below, sketch the ray that originates at the real root of
--- 0 WORK AREA LINES (style=lined) ---
- ii. Find the equation of this ray in the form
--- 3 WORK AREA LINES (style=lined) ---
- Verify that the equation
-
--- 4 WORK AREA LINES (style=lined) ---
- i. Express
--- 3 WORK AREA LINES (style=lined) ---
- ii. Given that
use De Moivre's theorem to show that
-
-
--- 8 WORK AREA LINES (style=lined) ---
-
Probability, MET2 2023 VCAA 4
A manufacturer produces tennis balls.
The diameter of the tennis balls is a normally distributed random variable
- Find
--- 1 WORK AREA LINES (style=lined) ---
- Find the minimum diameter of a tennis ball that is larger than 90% of all tennis balls produced.
- Give your answer in centimetres, correct to two decimal places. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
Tennis balls are packed and sold in cylindrical containers. A tennis ball can fit through the opening at the top of the container if its diameter is smaller than 6.95 cm.
- Find the probability that a randomly selected tennis ball can fit through the opening at the top of the container.
- Give your answer correct to four decimal places. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- In a random selection of 4 tennis balls, find the probability that at least 3 balls can fit through the opening at the top of the container.
- Give your answer correct to four decimal places. (2 marks)
--- 2 WORK AREA LINES (style=lined) ---
A tennis ball is classed as grade A if its diameter is between 6.54 cm and 6.86 cm, otherwise it is classed as grade B.
- Given that a tennis ball can fit through the opening at the top of the container, find the probability that it is classed as grade A.
- Give your answer correct to four decimal places. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- The manufacturer would like to improve processes to ensure that more than 99% of all tennis balls produced are classed as grade A.
- Assuming that the mean diameter of the tennis balls remains the same, find the required standard deviation of the diameter, in centimetres, correct to two decimal places. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
- An inspector takes a random sample of 32 tennis balls from the manufacturer and determines a confidence interval for the population proportion of grade A balls produced.
- The confidence interval is (0.7382, 0.9493), correct to four decimal places.
- Find the level of confidence that the population proportion of grade A balls is within the interval, as a percentage correct to the nearest integer. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
A tennis coach uses both grade A and grade B balls. The serving speed, in metres per second, of a grade A ball is a continuous random variable,
- Find the probability that the serving speed of a grade A ball exceeds 50 metres per second.
- Give your answer correct to four decimal places. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Find the exact mean serving speed for grade A balls, in metres per second. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
The serving speed of a grade B ball is given by a continuous random variable,
A transformation maps the graph of
- If the mean serving speed for a grade B ball is
--- 5 WORK AREA LINES (style=lined) ---
Calculus, MET2 2023 VCAA 5
- Complete a possible sequence of transformations to map
• Dilation of factor--- 2 WORK AREA LINES (style=lined) ---
Two functions
- Give the domain and range for the inverse of
--- 3 WORK AREA LINES (style=lined) ---
Shown below is the graph of
The intersection points between the graphs of
-
- Find the coordinates of
--- 2 WORK AREA LINES (style=lined) ---
- Find the coordinates of
-
- Find the area of the region bound by the graphs of
- Give your answer correct to two decimal places. (2 marks)
--- 4 WORK AREA LINES (style=lined) ---
- Find the area of the region bound by the graphs of
Let
- The turning point of
- Find the value of
--- 2 WORK AREA LINES (style=lined) ---
Let
The rule for the inverse of
- What is the smallest value of
- Give your answer correct to two decimal places. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
It is possible for the graphs of
- Find the area of the region bound by the graphs of
- Give your answer correct to two decimal places. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
Calculus, MET2 2023 VCAA 11 MC
Two functions,
The gradient of the graph
Calculus, MET1 2022 VCAA 8
Part of the graph of
- State the value of
--- 2 WORK AREA LINES (style=lined) ---
- Evaluate
--- 6 WORK AREA LINES (style=lined) ---
- Consider the average value of the function
- Find the value of
--- 6 WORK AREA LINES (style=lined) ---
Calculus, MET1 2022 VCAA 7
A tilemaker wants to make square tiles of size 20 cm × 20 cm.
The front surface of the tiles is to be painted with two different colours that meet the following conditions:
- Condition 1 - Each colour covers half the front surface of a tile.
- Condition 2 - The tiles can be lined up in a single horizontal row so that the colours form a continuous pattern.
An example is shown below.
There are two types of tiles: Type A and Type B.
For Type A, the colours on the tiles are divided using the rule
The corners of each tile have the coordinates (0,0), (20,0), (20,20) and (0,20), as shown below.
- i. Find the area of the front surface of each tile. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
ii. Find the value of
--- 2 WORK AREA LINES (style=lined) ---
Type B tiles, an example of which is shown below, are divided using the rule
- Show that a Type B tile meets Condition 1. (3 marks)
--- 8 WORK AREA LINES (style=lined) ---
- Determine the endpoints of
--- 8 WORK AREA LINES (style=lined) ---
Graphs, MET1 2022 VCAA 6
The graph of
- On the axes above, draw the graph of
--- 0 WORK AREA LINES (style=lined) ---
- Find all values of
--- 5 WORK AREA LINES (style=lined) ---
- Let
- The graph of
-
- Find the value for
--- 2 WORK AREA LINES (style=lined) ---
- Find the smallest positive value for
--- 2 WORK AREA LINES (style=lined) ---
- Hence, or otherwise, state the domain,
--- 2 WORK AREA LINES (style=lined) ---
- Find the value for
Calculus, MET1 2023 VCAA 9
The shapes of two walking tracks are shown below.
Track 1 is described by the function
Track 2 is defined by the function
The unit of length is kilometres.
- Given that
--- 4 WORK AREA LINES (style=lined) ---
- Verify that
- Give the co-ordinates of
--- 8 WORK AREA LINES (style=lined) ---
- A theme park is planned whose boundaries will form the triangle
- Find the maximum possible area of the theme park, in km². (3 marks)
--- 12 WORK AREA LINES (style=lined) ---
Statistics, MET1 2023 VCAA 6
Let
From a sample of randomly selected households in a given suburb, an approximate 95% confidence interval for the proportion
- Find the value of
--- 2 WORK AREA LINES (style=lined) ---
Use
- Find the size of the sample from which this 95% confidence interval was obtained. (2 marks)
--- 5 WORK AREA LINES (style=lined) ---
- A larger sample of households is selected, with a sample size four times the original sample.
- The sample proportion of households having solar panels installed is found to be the same.
- By what factor will the increased sample size affect the width of the confidence interval? (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
Probability, MET1 2023 VCAA 8
Suppose that the queuing time,
for some
- Show that
--- 5 WORK AREA LINES (style=lined) ---
- Find
--- 6 WORK AREA LINES (style=lined) ---
- What is the probability that a person has to queue for more than two minutes, given that they have already queued for one minute? (3 marks)
--- 12 WORK AREA LINES (style=blank) ---
Networks, GEN2 2023 VCAA 14
One of the landmarks in state
This project involves 12 activities,
The table below shows the 12 activities that need to be completed for the renovation project.
It also shows the earliest start time (EST), the duration, and the immediate predecessors for the activities.
The immediate predecessor(s) for activity
- Write down the immediate predecessor(s) for activity
--- 1 WORK AREA LINES (style=lined) ---
- What is the earliest start time, in days, for activity
--- 1 WORK AREA LINES (style=lined) ---
- How many activities have a float time of zero? (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
The managers of the project are able to reduce the time, in days, of six activities.
These reductions will result in an increase in the cost of completing the activity.
The maximum decrease in time of any activity is two days.
- If activities
- What will be the maximum reduction time, in days? (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- The managers of the project have a maximum budget of $15 000 to reduce the time for several activities to produce the maximum reduction in the project's overall completion time.
- Complete the table below, showing the reductions in individual activity completion times that would achieve the earliest completion time within the $ 15 000 budget. (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
Networks, GEN2 2023 VCAA 13
The state
The edges on the graph represent the roads between the landmarks.
The numbers on each edge represent the length, in kilometres, along each road.
Three friends, Eden, Reynold and Shyla, meet at landmark
- Eden would like to visit landmark
- What is the minimum distance Eden could travel from
--- 1 WORK AREA LINES (style=lined) ---
- Reynold would like to visit all the landmarks and return to
- Write down a route that Reynold could follow to minimise the total distance travelled. (1 mark)
--- 1 WORK AREA LINES (style=lined) ---
- Shyla would like to travel along all the roads.
- To complete this journey in the minimum distance, she will travel along two roads twice.
- Shyla will leave from landmark
- Complete the following by filling in the boxes provided.
- The two roads that will be travelled along twice are the roads between: (1 mark)
--- 0 WORK AREA LINES (style=lined) ---
Recursion and Finance, GEN2 2023 VCAA 7
Arthur takes out a new loan of $60 000 to pay for an overseas holiday.
Interest on this loan compounds weekly.
The balance of the loan, in dollars, after
- Show that the interest rate for this loan is 7.8% per annum. (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
- Determine the value of
- Arthur makes interest-only repayments (1 mark)
--- 2 WORK AREA LINES (style=lined) ---
-
Arthur fully repays the loan in five years. Round your answer to the nearest cent. (1 mark)
--- 3 WORK AREA LINES (style=lined) ---
- Arthur makes interest-only repayments (1 mark)
- Arthur decides that the value of
- If Arthur fully repays the loan with exactly three more years of repayments, what new value of
--- 3 WORK AREA LINES (style=lined) ---
- For what value of
--- 2 WORK AREA LINES (style=lined) ---
Networks, GEN1 2022 VCAA 4 MC
Consider the graph below.
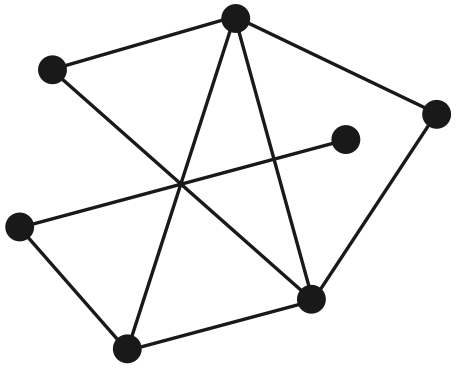
The number of edges that need to be removed for this graph to be planar is
- 0
- 1
- 2
- 3
- 4
Matrices, GEN1 2022 VCAA 8 MC
Two types of computers - laptops
Matrix
Matrix
A calculation that determines the total time that it would take each of Henry, Irvine or Jean, working alone, to service all the laptops and desktops in all four departments is
- « Previous Page
- 1
- 2
- 3
- 4
- 5
- …
- 24
- Next Page »